No CrossRef data available.
Article contents
EXCEPTIONAL SIMPLE REAL LIE ALGEBRAS 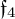 $\mathfrak {f}_4$ AND
$\mathfrak {f}_4$ AND 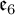 $\mathfrak {e}_6$ VIA CONTACTIFICATIONS
$\mathfrak {e}_6$ VIA CONTACTIFICATIONS
Published online by Cambridge University Press: 03 July 2024
Abstract
In Cartan’s PhD thesis, there is a formula defining a certain rank 8 vector distribution in dimension 15, whose algebra of authomorphism is the split real form of the simple exceptional complex Lie algebra 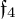 $\mathfrak {f}_4$. Cartan’s formula is written in the standard Cartesian coordinates in
$\mathfrak {f}_4$. Cartan’s formula is written in the standard Cartesian coordinates in 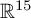 $\mathbb {R}^{15}$. In the present paper, we explain how to find analogous formulae for the flat models of any bracket generating distribution
$\mathbb {R}^{15}$. In the present paper, we explain how to find analogous formulae for the flat models of any bracket generating distribution 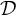 $\mathcal D$ whose symbol algebra
$\mathcal D$ whose symbol algebra  $\mathfrak {n}({\mathcal D})$ is constant and 2-step graded,
$\mathfrak {n}({\mathcal D})$ is constant and 2-step graded, 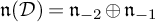 $\mathfrak {n}({\mathcal D})=\mathfrak {n}_{-2}\oplus \mathfrak {n}_{-1}$.
$\mathfrak {n}({\mathcal D})=\mathfrak {n}_{-2}\oplus \mathfrak {n}_{-1}$.
The formula is given in terms of a solution to a certain system of linear algebraic equations determined by two representations 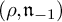 $(\rho ,\mathfrak {n}_{-1})$ and
$(\rho ,\mathfrak {n}_{-1})$ and  $(\tau ,\mathfrak {n}_{-2})$ of a Lie algebra
$(\tau ,\mathfrak {n}_{-2})$ of a Lie algebra 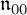 $\mathfrak {n}_{00}$ contained in the
$\mathfrak {n}_{00}$ contained in the 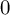 $0$th order Tanaka prolongation
$0$th order Tanaka prolongation 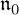 $\mathfrak {n}_0$ of
$\mathfrak {n}_0$ of 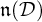 $\mathfrak {n}({\mathcal D})$.
$\mathfrak {n}({\mathcal D})$.
Numerous examples are provided, with particular emphasis put on the distributions with symmetries being real forms of simple exceptional Lie algebras  $\mathfrak {f}_4$ and
$\mathfrak {f}_4$ and 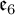 $\mathfrak {e}_6$.
$\mathfrak {e}_6$.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press
Footnotes
The research was funded from the Norwegian Financial Mechanism 2014-2021 with project registration number 2019/34/H/ST1/00636





