Hostname: page-component-848d4c4894-cjp7w Total loading time: 0 Render date: 2024-06-27T08:54:52.704Z Has data issue: false hasContentIssue false
  • English
  • Français

THE TWO-ARC-TRANSITIVE GRAPHS OF SQUARE-FREE ORDER ADMITTING ALTERNATING OR SYMMETRIC GROUPS

Part of: Permutation groups

Published online by Cambridge University Press:  29 March 2017

GAI XIA WANG  and
ZAI PING LU
GAI XIA WANG*
Affiliation:
Department of Applied Mathematics, AnHui University of Technology, Ma’an shan 243002, PR China email wgx075@163.com
ZAI PING LU
Affiliation:
Center for Combinatorics, LPMC, Nankai University, Tianjin 300071, PR China email lu@nankai.edu.cn
*
✉wgx075@163.com
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 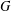$G$ be a finite group with $\mathsf{soc}(G)=\text{A}_{c}$ for 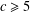$c\geq 5$. A characterization of the subgroups with square-free index in 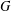$G$ is given. Also, it is shown that a $(G,2)$-arc-transitive graph of square-free order is isomorphic to a complete graph, a complete bipartite graph with a matching deleted or one of $11$ other graphs.

Keywords

symmetric graphtwo-arc-transitive graphautomorphism group

MSC classification

Primary: 20B15: Primitive groups
Secondary: 20B30: Symmetric groups
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 104 , Issue 1 , February 2018 , pp. 127 - 144
Copyright
© 2017 Australian Mathematical Publishing Association Inc. 

Footnotes

This work is supported by National Natural Science Foundation of China (11371204) and NNSFC (11601005).

References

Baddeley, R. W., ‘Two-arc transitive graphs and twisted wreath products’, J. Algebraic Combin. 2 (1993), 215–237.Google Scholar
Biggs, N., Algebraic Graph Theory (Cambridge University Press, New York, 1992).Google Scholar
Cheng, Y. and Oxley, J., ‘On weakly symmetric graphs of order twice a prime’, J. Combin. Theory B 42 (1987), 196–211.CrossRefGoogle Scholar
Conder, M. and Dobcsányi, P., ‘Trivalent symmetric graphs on up to 768 vertices’, J. Combin. Math. Combin. Comput. 40 (2002), 41–63.Google Scholar
Conway, J. H., Curtis, R. T., Norton, S. P., Parker, R. A. and Wilson, R. A., Atlas of Finite Groups (Oxford University Press, Eynsham, 1985).Google Scholar
Dixon, J. D. and Mortimer, B., Permutation Groups (Springer, New York–Berlin–Heidelberg, 1996).Google Scholar
Fang, X. G. and Praeger, C. E., ‘Finite two-arc transitive graphs admitting a Suzuki simple group’, Comm. Algebra 27 (1999), 3727–3754.Google Scholar
Fang, X. G. and Praeger, C. E., ‘Finite two-arc transitive graphs admitting a Ree simple group’, Comm. Algebra 27 (1999), 3755–3769.Google Scholar
Gardiner, A., ‘Arc transitivity in graphs’, Quart. J. Math. Oxford (2) 24 (1973), 399–407.Google Scholar
Hassani, A., Iranmanesh, M. and Praeger, C. E., ‘On vertex-imprimitive graphs of order a product of three distinct odd primes’, J. Combin. Math. Combin. Comput. 28 (1998), 187–213.Google Scholar
Hassani, A., Nochefranca, L. R. and Praeger, C. E., ‘Two-arc transitive graphs admitting a two-dimensional projective linear group’, J. Group Theory 2 (1999), 335–353.Google Scholar
Iranmanesh, M. A. and Praeger, C. E., ‘On non-Cayley vertex-transitive graphs of order a product of three primes’, J. Combin. Theory B 81 (2001), 1–19.Google Scholar
Ivanov, A. A. and Praeger, C. E., ‘On finite affine 2-arc transitive graphs. Algebraic combinatorics’, Eur. J. Combin. 14(5) (1993), 421–444.Google Scholar
Kantor, W. M., ‘Homogeneous designs and geometric lattices’, J. Combin. Theory A 38 (1985), 66–74.Google Scholar
Li, C. H., ‘A family of quasiprimitive 2-arc-transitive graphs which have non-quasiprimitive full automorphism groups’, Eur. J. Combin. 19 (1998), 499–502.Google Scholar
Li, C. H., ‘On automorphism groups of quasiprimitive 2-arc transitive graphs’, J. Algebric Combin. 28 (2008), 261–270.Google Scholar
Li, C. H., Liu, Z. and Lu, Z. P., ‘The edge-transitive tetravalent Cayley graphs of square-free order’, Discrete Math. 312 (2012), 1952–1967.Google Scholar
Li, C. H., Lu, Z. P. and Marušič, D., ‘On primitive permutation groups with small suborbits and their orbital graphs’, J. Algebra 279 (2004), 749–770.Google Scholar
Li, C. H., Lu, Z. P. and Wang, G. X., ‘Vertex-transitive cubic graphs of square-free order’, J. Graph Theory 75 (2014), 1–19.Google Scholar
Li, C. H. and Seress, A., ‘The primitive permutation groups of square-free degree’, Bull. Lond. Math. Soc. 35 (2003), 635–644.Google Scholar
Li, C. H. and Seress, A., ‘On vertex-primitive non-Cayley graphs of square-free order’, Des. Codes Cryptogr. 34 (2005), 265–281.Google Scholar
Marušič, D. and Potočnik, P., ‘Classifying 2-arc-transitive graphs of order a product of two primes’, Discrete Math. 244 (2002), 331–338.Google Scholar
Miller, A. A. and Praeger, C. E., ‘Non-Cayley vertex-transitive graphs of order twice the product of two odd primes’, J. Algebraic Combin. 3 (1994), 77–111.Google Scholar
Praeger, C. E., ‘An O’Nan-Scott theorem for finite quasiprimitive permutation groups and an application to 2-arc transitive graphs’, J. Lond. Math. Soc. 47 (1992), 227–239.Google Scholar
Praeger, C. E., ‘On a reduction theorem for finite bipartite 2-arc transitive graphs’, Australas. J. Combin. 7 (1993), 21–36.Google Scholar
Praeger, C. E. and Wang, J., ‘On primitive representations of finite alternating and symmetric groups with a 2-transitive subconstituent’, J. Algebra 180 (1996), 808–833.Google Scholar
Praeger, C. E., Wang, R. J. and Xu, M. Y., ‘Symmetric graphs of order a product of two distinct primes’, J. Combin. Theory Ser. B 58 (1993), 299–318.Google Scholar
Praeger, C. E. and Xu, M. Y., ‘Vertex-primitive graphs of order a product of two distinct primes’, J. Combin. Theory Ser. B 59 (1993), 245–266.Google Scholar
Sabidussi, G., ‘Vertex-transitive graphs’, Monatsh. Math. 68 (1964), 426–438.Google Scholar
Seress, A., ‘On vertex-transitive, non-Cayley graphs of order pqr ’, Discrete Math. 182 (1998), 279–292.Google Scholar
Turner, J. M., ‘Point-symmetric graphs with a prime number of points’, J. Combin. Theory 3(2) (1967), 136–145.Google Scholar
Wang, R. J. and Xu, M. Y., ‘A classification of symmetric graph of order 3p ’, J. Combin. Theory B 58 (1993), 197–216.Google Scholar
Weiss, R., ‘Groups with a (B, N)-pair and locally transitive graphs’, J. Nagoya Math. 74 (1979), 1–21.Google Scholar
Weiss, R., ‘ s-transitive graphs, Algebraic methods in graph theory’, Colloq. Soc. Janos Bolyai 25 (1981), 827–847.Google Scholar
Weiss, R., ‘The nonexistence of 8-transitive graphs’, Combinatorica 1 (1981), 309–311.Google Scholar