I investigate the relationships between three hierarchies of reflection principles for a forcing class 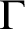$\Gamma $ : the hierarchy of bounded forcing axioms, of 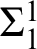$\Sigma ^1_1$ -absoluteness, and of Aronszajn tree preservation principles. The latter principle at level 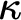$\kappa $ says that whenever T is a tree of height $\omega _1$ and width 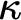$\kappa $ that does not have a branch of order type 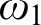$\omega _1$ , and whenever 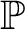${\mathord {\mathbb P}}$ is a forcing notion in 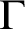$\Gamma $ , then it is not the case that 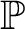${\mathord {\mathbb P}}$ forces that T has such a branch. 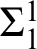$\Sigma ^1_1$ -absoluteness serves as an intermediary between these principles and the bounded forcing axioms. A special case of the main result is that for forcing classes that don’t add reals, the three principles at level 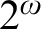$2^\omega $ are equivalent. Special attention is paid to certain subclasses of subcomplete forcing, since these are natural forcing classes that don’t add reals.