Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-17T16:21:28.542Z Has data issue: false hasContentIssue false
  • English
  • Français

Microscale deformation of (001) and (100) rutile single crystals under spherical nanoindentation

Published online by Cambridge University Press:  24 November 2011

Sandip Basu ,
Omar A. Elshrief ,
Robert Coward ,
Babak Anasori  and
Michel W. Barsoum
Sandip Basu
Affiliation:
Department of Mechanical Engineering, Texas A&M University, College Station, Texas 77843
Omar A. Elshrief
Affiliation:
Department of Materials Science and Engineering, Drexel University, Philadelphia, Pennsylvania 19104
Robert Coward
Affiliation:
Department of Materials Science and Engineering, Drexel University, Philadelphia, Pennsylvania 19104
Babak Anasori
Affiliation:
Department of Materials Science and Engineering, Drexel University, Philadelphia, Pennsylvania 19104
Michel W. Barsoum*
Affiliation:
Department of Materials Science and Engineering, Drexel University, Philadelphia, Pennsylvania 19104
*
a)Address all correspondence to this author. e-mail: barsoumw@drexel.edu
Get access

Abstract

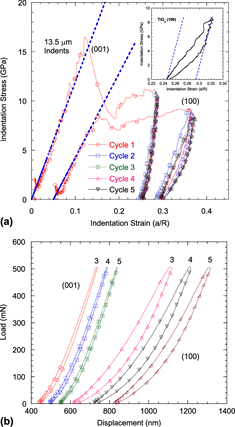
Herein rutile (TiO2) single-crystal surfaces, with (001) and (100) orientations, were indented with hemispherical indenters with radii of 13.5, 5, and 1.4 μm. By converting the load–displacement data to nanoindentation (NI) stress–strain curves, together with microscopic post-indentation observations, we conclude that in the (001) orientation, plastic deformation occurs by the activation of all four {101}<10> slip systems. In the (100) orientation, only two of the four {101}<10 > slip systems, along with {100}<00> slip, are activated. Because the four {101}<10> slip systems in the (001) orientation intersect, the surface is harder and exhibits higher hardening rates after the nucleation of dislocations. The latter are manifested by pop-ins, some of which are large. The pop-in stresses are adequately described by Weibull statistics and were significantly higher for the (001) orientation. The elastic moduli, determined from spherical NI stiffness versus contact radii plots, were 349 ± 5 and 229 ± 4 GPa for (001) and (100) orientations, respectively. Fully spontaneous reversible, stress–strain hysteretic curves—only manifest in the (100) orientation—are attributed to the to-and-fro motion of dislocations comprising incipient kink bands in the {100}<00> slip system.

Keywords

NanoindentationStress–strain relationshipDislocations
Type
Articles
Information
Journal of Materials Research , Volume 27 , Issue 1: Focus Issue: Instrumented Indentation , 14 January 2012 , pp. 53 - 63
Copyright
Copyright © Materials Research Society 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

1.Diebold, U.: Structure and properties of TiO2 surfaces: A brief review. Appl. Phys. A Mater. Sci. Process. 76, 681 (2003).CrossRefGoogle Scholar
2.Park, N-G., Lagemaat, J.d., and Frank, A.J.: Comparison of dye-sensitized rutile- and anatase-based TiO2 solar cells. J. Phys. Chem. B 104, 8989 (2000).CrossRefGoogle Scholar
3.Matsuura, S.: New developments and applications of gas sensors in Japan. Sens. Actuators, B 13–14, 7 (1993).CrossRefGoogle Scholar
4.Okimura, K., Maeda, N., and Shibata, A.: Characteristics of rutile TiO2 films prepared by r.f. magnetron sputtering at a low temperature. Thin Solid Films 281–282, 427 (1996).CrossRefGoogle Scholar
5.Feng, B., Chen, J.Y., Qi, S.K., He, L., Zhao, J.Z., and Zhang, X.D.: Characterization of surface oxide films on titanium and bioactivity. J. Mater. Sci.- Mater. Med. 13, 457 (2002).CrossRefGoogle ScholarPubMed
6.Kadoshima, M., Hiratani, M., Shimamoto, Y., Torii, K., Miki, H., Kimura, S., and Nabatame, T.: Rutile-type TiO2 thin film for high-k gate insulator. Thin Solid Films 424, 224 (2003).CrossRefGoogle Scholar
7.Hirthe, W.M. and Brittain, J.O.: Dislocations in rutile as revealed by the etch-pit technique. J. Am. Ceram. Soc. 45, 546 (1962).CrossRefGoogle Scholar
8.Ashbee, K.H.G. and Smallman, R.E.: The plastic deformation of titanium dioxide single crystals. Proc. R. Soc. London, Ser.A 274, 195 (1963).Google Scholar
9.Blanchin, M.G., Bursill, L.A., and Lafage, C.: Deformation and microstructure of rutile. Proc. R. Soc. London, Ser. A 429, 175 (1990).Google Scholar
10.Li, H. and Bradt, R.C.: Knoop microhardness anisotropy of single crystal rutile. J. Am. Ceram. Soc. 73, 1360 (1990).CrossRefGoogle Scholar
11.Li, H. and Bradt, R.C.: The microhardness indentation load/size effect in rutile and cassiterite single crystals. J. Mater. Sci. 28, 917 (1993).CrossRefGoogle Scholar
12.Basu, S. and Barsoum, M.W.: Deformation micromechanisms of ZnO single crystals as determined from spherical nanoindentation stress-strain curves. J. Mater. Res. 22, 2470 (2007).CrossRefGoogle Scholar
13.Mayo, M.J., Siegel, R.W., Narayanasamy, A., and Nix, W.D.: Mechanical properties of nanophase TiO2 as determined by nanoindentation. J. Mater. Res. 5, 1073 (1990).CrossRefGoogle Scholar
14.Kurosaki, K., Setoyama, D., Matsunaga, J., and Yamanaka, S.: Nanoindentation tests for TiO2, MgO, and YSZ single crystals. J. Alloy. Comp. 386, 261 (2005).CrossRefGoogle Scholar
15.Olofinjana, A.O., Bell, J.M., and Jamting, A.K.: Evaluation of the mechanical properties of sol-gel-deposited titania films using ultra-micro-indentation method. Wear 241, 174 (2000).CrossRefGoogle Scholar
16.Basu, S., Moseson, A., and Barsoum, M.W.: On the determination of spherical nanoindentation stress-strain curves. J. Mater. Res. 21, 2628 (2006).CrossRefGoogle Scholar
17.Moseson, A.J., Basu, S., and Barsoum, M.W.: Determination of the effective zero point of contact for spherical nanoindentation. J. Mater. Res. 23, 204 (2008).CrossRefGoogle Scholar
18.Basu, S., Barsoum, M.W., and Kalidindi, S.R.: Sapphire: A kinking nonlinear elastic solid. J. Appl. Phys. 99, 063501 (2006).CrossRefGoogle Scholar
19.Basu, S., Zhou, A., and Barsoum, M.W.: Reversible dislocation motion under contact loading in LiNbO3 single crystal. J. Mater. Res. 23, 1334 (2008).CrossRefGoogle Scholar
20.Basu, S., Zhou, A., and Barsoum, M.W.: On spherical nanoindentations, kinking nonlinear elasticity of mica single crystals and their geological implications. J. Struct. Geol. 31, 791 (2009).CrossRefGoogle Scholar
21.Barsoum, M.W., Murugaiah, A., Kalidindi, S.R., and Zhen, T.: Kinking nonlinear elastic solids, nanoindentations and geology. Phys. Rev. Lett. 92, 255508 (2004).CrossRefGoogle ScholarPubMed
22.Buchs, R., Basu, S., Elshrief, O., Coward, R., and Barsoum, M.W.: Spherical nanoindentation and vickers microhardness study of the deformation of poled BaTiO3 single crystals. J. Appl. Phys. 105, 093540 (2009).CrossRefGoogle Scholar
23.Basu, S., Barsoum, M.W., Williams, A.D., and Moustakas, T.D.: Spherical nanoindentation and deformation mechanisms in free-standing GaN films. J. Appl. Phys. 101, 083522 (2007).CrossRefGoogle Scholar
24.Sneddon, I.N.: The relaxation between load and penetration in the axisymmetric boussinesq problem for a punch of arbitrary profile. Int. J. Eng. Sci. 3, 47 (1965).CrossRefGoogle Scholar
25.Barsoum, M.W., Zhen, T., Kalidindi, S.R., Radovic, M., and Murugahiah, A.: Fully reversible, dislocation-based compressive deformation of Ti3SiC2 to 1 GPa. Nat. Mater. 2, 107 (2003).CrossRefGoogle ScholarPubMed
26.Barsoum, M.W., Murugaiah, A., Kalidindi, S.R., and Gogotsi, Y.: Kink bands, nonlinear elasticity and nanoindentations in graphite. Carbon 42, 1435 (2004).CrossRefGoogle Scholar
27.Barsoum, M.W., Zhen, T., Zhou, A., Basu, S., and Kalidindi, S.R.: Microscale modeling of kinking nonlinear elastic solids. Phys. Rev. B 71, 134101 (2005).CrossRefGoogle Scholar
28.Zhou, A., Basu, S., and Barsoum, M.W.: Kinking nonlinear elasticity, damping, micro- and macroyielding of hexagonal close-packed metals. Acta Mater. 56, 60 (2008).CrossRefGoogle Scholar
29.Barsoum, M.W. and Basu, S.: Kinking nonlinear elastic solids, in Encyclopedia of Materials: Science and Technology, edited by Buschow, K.H.J., Cahn, R.W., Flemings, M.C., Ilschner, B., Kramer, E.J., Mahajan, S., and Veyssiere, P. (Elsevier, Oxford, 2010).Google Scholar
30.Murugaiah, A., Barsoum, M.W., Kalidindi, S.R., and Zhen, T.: Spherical nanoindentations in Ti3SiC2. J. Mater. Res. 19, 1139 (2004).CrossRefGoogle Scholar
31.Zhou, A.G., Barsoum, M.W., Basu, S., Kalidindi, S.R., and El-Raghy, T.: Incipient and regular kink bands in dense and porous Ti2AlC. Acta Mater. 54, 1631 (2006).CrossRefGoogle Scholar
32.Frank, F.C. and Stroh, A.N.: On the theory of kinking. Proc. Phys. Soc. 65, 811 (1952).CrossRefGoogle Scholar
33.Hertz, H.: Miscellaneous Papers by H. Hertz (Macmillan, London, 1896).Google Scholar
34.Wachtman, J.B., Tefft, W.E., and Lam, D.G.: Elastic constants of rutile (TiO2). J. Res. Nat. Bur. Stand. 66A, 465 (1962).CrossRefGoogle Scholar
35.Chang, E. and Graham, E.K.: The elastic constants of cassiterite SnO2 and their pressure and temperature dependence. J. Geophys. Res. 80, 2595 (1975).CrossRefGoogle Scholar
36.Isaak, D.G., Carnes, J.D., Anderson, O.L., Cynn, H., and Hake, E.: Elasticity of TiO2 rutile to 1800 K. Phys. Chem. Miner. 26, 31 (1998).CrossRefGoogle Scholar
37.Lorenz, D., Zeckzer, A., Hilpert, U., Grau, P., Johansen, H., and Leipner, H.S.: Pop-in effect as homogeneous nucleation of dislocations during nanoindentation. Phys. Rev. B 67, 172101 (2003).CrossRefGoogle Scholar
38.Schuh, C.A., Mason, J.K., and Lund, A.C.: Quantitative insight into dislocation nucleation from high-temperature nanoindentation experiments. Nat. Mater. 4, 617 (2005).CrossRefGoogle ScholarPubMed
39.Morris, J.R., Bei, H., Pharr, G.M., and George, E.P.: Size effects and stochastic behavior of nanoindentation pop in. Phys. Rev. Lett. 106, 165502 (2011).CrossRefGoogle ScholarPubMed
40.Ashbee, K.H.G. and Smallman, R.E.: Stress-strain behavior of titanium dioxide (rutile) single crystals. J. Am. Ceram. Soc. 46, 211 (1963).CrossRefGoogle Scholar
41.Zhou, A.G. and Barsoum, M.W.: Kinking nonlinear elastic deformation of Ti3AlC2, Ti2AlC, Ti3Al(C0.5, N0.5)2 and Ti2Al(C0.5, N0.5). J. Alloy. Comp. 498, 62 (2010).CrossRefGoogle Scholar
42.Anasori, B.: Spherical nanoindentation study of the deformation micromechanisms of LiTaO3 single crystals. J. Appl. Phys. 110, 023516 (2011).CrossRefGoogle Scholar
43.Ma, X.G., Liang, P., Miao, L., Bie, S.W., Zhang, C.K., Xu, L., and Jiang, J.J.: Pressure-induced phase transition and elastic properties of TiO2 polymorphs. Phys. Status Solidi B 246, 2132 (2009).CrossRefGoogle Scholar