Hostname: page-component-77c89778f8-vsgnj Total loading time: 0 Render date: 2024-07-17T08:58:55.989Z Has data issue: false hasContentIssue false
  • English
  • Français

Spectral evolution of weakly nonlinear random waves: kinetic description versus direct numerical simulations

Published online by Cambridge University Press:  12 April 2018

Sergei Y. Annenkov  and
Victor I. Shrira Open the ORCID record for Victor I. Shrira [Opens in a new window]
Sergei Y. Annenkov*
Affiliation:
School of Computing and Mathematics, Keele University, Keele ST5 5BG, UK
Victor I. Shrira
Affiliation:
School of Computing and Mathematics, Keele University, Keele ST5 5BG, UK
*
†Email address for correspondence: s.annenkov@keele.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

Kinetic equations are widely used in many branches of science to describe the evolution of random wave spectra. To examine the validity of these equations, we study numerically the long-term evolution of water wave spectra without wind input using three different models. The first model is the classical kinetic (Hasselmann) equation (KE). The second model is the generalised kinetic equation (gKE), derived employing the same statistical closure as the KE but without the assumption of quasistationarity. The third model, which we refer to as the DNS-ZE, is a direct numerical simulation algorithm based on the Zakharov integrodifferential equation, which plays the role of the primitive equation for a weakly nonlinear wave field. It does not employ any statistical assumptions. We perform a comparison of the spectral evolution of the same initial distributions without forcing, with/without a statistical closure and with/without the quasistationarity assumption. For the initial conditions, we choose two narrow-banded spectra with the same frequency distribution and different degrees of directionality. The short-term evolution (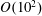$O(10^{2})$ wave periods) of both spectra has been previously thoroughly studied experimentally and numerically using a variety of approaches. Our DNS-ZE results are validated both with existing short-term DNS by other methods and with available laboratory observations of higher-order moment (kurtosis) evolution. All three models demonstrate very close evolution of integral characteristics of the spectra, approaching with time the theoretical asymptotes of the self-similar stage of evolution. Both kinetic equations give almost identical spectral evolution, unless the spectrum is initially too narrow in angle. However, there are major differences between the DNS-ZE and gKE/KE predictions. First, the rate of angular broadening of initially narrow angular distributions is much larger for the gKE and KE than for the DNS-ZE, although the angular width does appear to tend to the same universal value at large times. Second, the shapes of the frequency spectra differ substantially (even when the nonlinearity is decreased), the DNS-ZE spectra being wider than the KE/gKE ones and having much lower spectral peaks. Third, the maximal rates of change of the spectra obtained with the DNS-ZE scale as the fourth power of nonlinearity, which corresponds to the dynamical time scale of evolution, rather than the sixth power of nonlinearity typical of the kinetic time scale exhibited by the KE. The gKE predictions fall in between. While the long-term DNS show excellent agreement with the KE predictions for integral characteristics of evolving wave spectra, the striking systematic discrepancies for a number of specific spectral characteristics call for revision of the fundamentals of the wave kinetic description.

JFM classification

Waves/Free-surface Flows: Surface gravity waves Waves/Free-surface Flows: Waves/Free-surface Flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 844 , 10 June 2018 , pp. 766 - 795
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Annenkov, S. Y. & Shrira, V. I. 2001 On the predictability of evolution of surface gravity and gravity–capillary waves. Physica D 152–153, 665–675.Google Scholar
Annenkov, S. Y. & Shrira, V. I. 2006a Role of non-resonant interactions in the evolution of nonlinear random water wave fields. J. Fluid Mech. 561, 181–207.10.1017/S0022112006000632Google Scholar
Annenkov, S. Y. & Shrira, V. I. 2006b Direct numerical simulation of downshift and inverse cascade for water wave turbulence. Phys. Rev. Lett. 96, 204501.10.1103/PhysRevLett.96.204501Google Scholar
Annenkov, S. Y. & Shrira, V. I. 2009 ‘Fast’ nonlinear evolution in wave turbulence. Phys. Rev. Lett. 102, 024502.10.1103/PhysRevLett.102.024502Google Scholar
Annenkov, S. Y. & Shrira, V. I. 2011 Evolution of wave turbulence under ‘gusty’ forcing. Phys. Rev. Lett. 107, 114502.10.1103/PhysRevLett.107.114502Google Scholar
Annenkov, S. Y. & Shrira, V. I. 2013 Large-time evolution of statistical moments of a wind wave field. J. Fluid Mech. 726, 517–546.10.1017/jfm.2013.243Google Scholar
Annenkov, S. Y. & Shrira, V. I. 2015 Modelling the impact of squall on wind waves with the generalized kinetic equation. J. Phys. Oceanogr. 45, 807–812.10.1175/JPO-D-14-0182.1Google Scholar
Annenkov, S. Y. & Shrira, V. I. 2016 Modelling transient sea states with the generalised kinetic equation. In Rogue and Shock Waves in Nonlinear Dispersive Media, pp. 159–178. Springer.10.1007/978-3-319-39214-1_6Google Scholar
Ardhuin, F., Chapron, B. & Collard, F. 2009 Observation of swell dissipation across oceans. Geophys. Res. Lett. 36, L06607.10.1029/2008GL037030Google Scholar
Badulin, S. I., Pushkarev, A. N., Resio, D. & Zakharov, V. E. 2005 Self-similarity of wind-driven seas. Nonlinear Process. Geophys. 12, 891–945.10.5194/npg-12-891-2005Google Scholar
Badulin, S. I. & Zakharov, V. E. 2017 Ocean swell within the kinetic equation for water waves. Nonlinear Process. Geophys. 24, 237–253.10.5194/npg-24-237-2017Google Scholar
Benney, D. J. & Saffman, P. G. 1966 Nonlinear interactions of random waves in a dispersive medium. Proc. R. Soc. Lond. A 289, 301–320.Google Scholar
Caulliez, G. & Collard, F. 1999 Three-dimensional evolution of wind waves from gravity–capillary to short gravity range. Eur. J. Mech. (B/Fluids) 18, 389–402.10.1016/S0997-7546(99)80036-3Google Scholar
Cavaleri, L. et al. 2007 Wave modelling – the state of the art. Prog. Oceanogr. 75, 603–674.10.1016/j.pocean.2007.05.005Google Scholar
Dommermuth, D. G. & Yue, D. K. P. 1987 A high-order spectral method for the study of nonlinear gravity waves. J. Fluid Mech. 184, 267–288.10.1017/S002211208700288XGoogle Scholar
Gagnaire-Renou, E., Benoit, M. & Badulin, S. I. 2011 On weakly turbulent scaling of wind sea in simulations of fetch-limited growth. J. Fluid Mech. 669, 178–213.10.1017/S0022112010004921Google Scholar
Gramstad, O. & Babanin, A. 2014 Implementing new nonlinear term in third generation wave models. In ASME 2014 33rd International Conference on Ocean, Offshore and Arctic Engineering, pp. V04BT02A057-V04BT02A057. American Society of Mechanical Engineers.Google Scholar
Gramstad, O. & Stiassnie, M. 2013 Phase-averaged equation for water waves. J. Fluid Mech. 718, 280–303.10.1017/jfm.2012.609Google Scholar
Hasselmann, K. 1962 On the non-linear energy transfer in a gravity-wave spectrum Part 1. General theory. J. Fluid Mech. 12, 481–500.10.1017/S0022112062000373Google Scholar
Hasselmann, K. et al. 1973 Measurements of wind wave growth and swell decay during the Joint North Sea Wave Project (JONSWAP). Deutches Hydrographisches Institut.Google Scholar
Hwang, P. A., Wang, D. W., Walsh, E. J., Krabill, W. B. & Swift, R. N. 2000 Airborne measurements of the wavenumber spectra of ocean surface waves. Part II: directional distribution. J. Phys. Oceanogr. 30, 2768–2787.10.1175/1520-0485(2001)031<2768:AMOTWS>2.0.CO;22.0.CO;2>Google Scholar
Hwung, H. H., Chiang, W. S. & Hsiao, S. C. 2007 Observations on the evolution of wave modulation. Proc. R. Soc. Lond. A 463, 85–112.10.1098/rspa.2006.1759Google Scholar
Janssen, P. A. E. M. 2003 Nonlinear four-wave interactions and freak waves. J. Phys. Oceanogr. 33, 863–884.10.1175/1520-0485(2003)33<863:NFIAFW>2.0.CO;22.0.CO;2>Google Scholar
Janssen, P. A. E. M. 2004 The Interaction of Ocean Waves and Wind. Cambridge University Press.10.1017/CBO9780511525018Google Scholar
Janssen, P. A. E. M. 2009 On some consequences of the canonical transformation in the Hamiltonian theory of water waves. J. Fluid Mech. 637, 1–44.10.1017/S0022112009008131Google Scholar
Krasitskii, V. P. 1994 On reduced Hamiltonian equations in the nonlinear theory of water surface waves. J. Fluid Mech. 272, 1–20.10.1017/S0022112094004350Google Scholar
Lvov, Y. V., Nazarenko, S. & Pokorni, B. 2006 Discreteness and its effect on water-wave turbulence. Physica D 218, 24–35.Google Scholar
Nazarenko, S., Lukaschuk, S., McLelland, S. & Denissenko, P. 2010 Statistics of surface gravity wave turbulence in the space and time domains. J. Fluid Mech. 642, 395–420.10.1017/S0022112009991820Google Scholar
Nazarenko, S. V. 2011 Wave Turbulence. Springer.10.1007/978-3-642-15942-8Google Scholar
Newell, A. C. & Rumpf, B. 2011 Wave turbulence. Annu. Rev. Fluid Mech. 43, 59–78.10.1146/annurev-fluid-122109-160807Google Scholar
Newell, A. C. & Rumpf, B. 2013 Wave turbulence: a story far from over. In Advances in Wave Turbulence, World Scientific Series on Nonlinear Science, 83, pp. 1–51. World Scientific.Google Scholar
Olagnon, M., Prevosto, M., Van Iseghem, S., Ewans, K. & Forristall, G. Z.2004 WASP (West Africa Swell Project) Final Report and Appendices. 200 $+$ 192 pp. Available at http://archimer.ifremer.fr/doc/00114/22537/.Google Scholar
Onorato, M., Cavaleri, L., Fouques, S., Gramstad, O., Janssen, P. A. E. M., Monbaliu, J., Osborne, A. R., Pakozdi, C., Serio, M., Stansberg, C. T. et al. 2009 Statistical properties of mechanically generated surface gravity waves: a laboratory experiment in a three-dimensional wave basin. J. Fluid Mech. 627, 235–257.10.1017/S002211200900603XGoogle Scholar
Pushkarev, A. N. & Zakharov, V. E. 2000 Turbulence of capillary waves – theory and numerical simulation. Physica D 135, 98–116.Google Scholar
Resio, D. & Perrie, W. 1991 A numerical study of nonlinear energy fluxes due to wave–wave interactions. Part 1. Methodology and basic results. J. Fluid Mech. 223, 603–629.10.1017/S002211209100157XGoogle Scholar
Resio, D. T., Vincent, L. & Ardag, D. 2016 Characteristics of directional wave spectra and implications for detailed-balance wave modeling. Ocean Model. 103, 38–52.10.1016/j.ocemod.2015.09.009Google Scholar
Shemer, L., Sergeeva, A. & Liberzon, D. 2010 Effect of the initial spectrum on the spatial evolution of statistics of unidirectional nonlinear random waves. J. Geophys. Res. 115, C12039.10.1029/2010JC006326Google Scholar
Shrira, V. I. & Annenkov, S. Y. 2013 Towards a new picture of wave turbulence. In Advances in Wave Turbulence, World Scientific Series on Nonlinear Science, 83, pp. 239–281. World Scientific.10.1142/9789814366946_0007Google Scholar
Tanaka, M. 2001 Verification of Hasselmann’s energy transfer among surface gravity waves by direct numerical simulations of primitive equations. J. Fluid Mech. 444, 199–221.10.1017/S0022112001005389Google Scholar
Toba, Y. 1973 Local balance in the air–sea boundary processes. Part III. On the spectrum of wind waves. J. Oceanogr. Soc. Japan 29, 209–220.10.1007/BF02108528Google Scholar
Toffoli, A., Gramstad, O., Trulsen, K., Monbaliu, J., Bitner-Gregersen, E. & Onorato, M. 2010 Evolution of weakly nonlinear random directional waves: laboratory experiments and numerical simulations. J. Fluid Mech. 664, 313–336.10.1017/S002211201000385XGoogle Scholar
Trulsen, K. & Dysthe, K. B. 1996 A modified nonlinear Schrödinger equation for broader bandwidth gravity waves on deep water. Wave Motion 24, 281–289.10.1016/S0165-2125(96)00020-0Google Scholar
van Vledder, G. P. 2006 The WRT method for the computation of non-linear four-wave interactions in discrete spectral wave models. Coast. Engng 53, 223–242.10.1016/j.coastaleng.2005.10.011Google Scholar
Xiao, W., Liu, Y., Wu, G. & Yue, D. K. 2013 Rogue wave occurrence and dynamics by direct simulations of nonlinear wave-field evolution. J. Fluid Mech. 720, 357–392.10.1017/jfm.2013.37Google Scholar
Zakharov, V. E. 1968 Stability of periodic waves of finite amplitude on the surface of a deep fluid. J. Appl. Mech. Tech. Phys. (USSR) 9, 86–94.Google Scholar
Zakharov, V. E., Korotkevich, A. O., Pushkarev, A. & Resio, D. 2007 Coexistence of weak and strong wave turbulence in a swell propagation. Phys. Rev. Lett. 99, 164501.10.1103/PhysRevLett.99.164501Google Scholar
Zakharov, V. E., L’vov, V. S. & Falkovich, G. 1992 Kolmogorov Spectra of Turbulence I: Wave Turbulence. Springer.10.1007/978-3-642-50052-7Google Scholar
Zavadsky, A., Liberzon, D. & Shemer, L. 2013 Statistical analysis of the spatial evolution of the stationary wind wave field. J. Phys. Oceanogr. 43, 65–79.10.1175/JPO-D-12-0103.1Google Scholar