Hostname: page-component-76fb5796d-9pm4c Total loading time: 0 Render date: 2024-04-27T08:16:26.223Z Has data issue: false hasContentIssue false
  • English
  • Français

On the response of convectively unstable flows to oscillatory forcing with application to liquid sheets

Published online by Cambridge University Press:  19 April 2012

N. S. Barlow ,
S. J. Weinstein  and
B. T. Helenbrook
N. S. Barlow*
Affiliation:
Department of Chemical and Biological Engineering, University at Buffalo, State University of New York, Buffalo, NY 14260, USA
S. J. Weinstein
Affiliation:
Department of Chemical and Biomedical Engineering, Rochester Institute of Technology, Rochester, NY 14623, USA
B. T. Helenbrook
Affiliation:
Department of Mechanical and Aeronautical Engineering, Clarkson University, Potsdam, NY 13699, USA
*
†Email address for correspondence: barlow.nate@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

The complete integral solution is found for the convectively unstable and oscillatory-forced linear Klein–Gordon equation as a function of spatial variable, , and time, . A comparison of the integral solution with series solutions of the Klein–Gordon equation elucidates salient features of both the transient and long-time spatially growing solutions. A rigorous method is developed for identifying the key 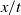rays associated with saddle points that can be used to characterize the transition between transient temporally growing and long-term spatially growing waves. This method effectively combines the procedure given by Gordillo & Pérez-Saborid (Phys. Fluids, vol. 14, 2002, pp. 4329–4343) for determining the 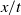ray at which the forced spatial growth response affects the observed waveform and competes with the transient response, with an established methodology for identifying the leading and trailing edge rays of an impulse response. The method is applied to a linearized system describing an oscillatory-forced liquid sheet and asymptotic predictions are obtained. Series solutions are used to validate these predictions. We establish that the portion of the solution responsible for spatial growth in the signalling problem is correctly identified by Gordillo & Pérez-Saborid (Phys. Fluids, vol. 14, 2002, pp. 4329–4343), and that this interpretation is in contrast with the classical literature. The approach provided here can be applied in multiple ways to study a convectively unstable oscillatory-forced medium. In cases where numerical or series solutions are readily available, the proposed method is used to extract key features of the solution. In cases where only the forced long time behaviour is needed, the dispersion relation is used to extract: (i) the time required to see the forced solution; (ii) the amplitude, phase and spatial growth of the forced solution; and (iii) the breadth of the transient.

JFM classification

Instability: Absolute/convective instability Flow Control: Instability control Interfacial Flows (free surface): Thin films
Type
Papers
Information
Journal of Fluid Mechanics , Volume 699 , 25 May 2012 , pp. 115 - 152
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Barlow, N. S., Helenbrook, B. T. & Lin, S. P. 2011 Transience to instability in a liquid sheet. J. Fluid Mech. 666, 358–390.Google Scholar
2. Barlow, N. S., Helenbrook, B. T., Lin, S. P. & Weinstein, S. J. 2010 An interpretation of absolutely and convectively unstable waves using series solutions. Wave Motion 47, 564–582.Google Scholar
3. Bender, C. M. & Orszag, S. A. 1978 Advanced Mathematical Methods for Scientists and Engineers I: Asymptotic Methods and Perturbation Theory. McGraw-Hill.Google Scholar
4. Bers, A. 1983 Space–time evolution of plasma instabilities-absolute and convective. In Handbook of Plasma Physics, vol. 1: Basic Plasma Physics 1 (ed. Galeev, A. A. & Sagdeev, R. Z. ), pp. 451–517. North-Holland, ch. 3.2.Google Scholar
5. Bleistein, N. 1984 Mathematical Methods for Wave Phenomena. Academic.Google Scholar
6. Bleistein, N. & Handelsman, R. A. 1975 Asymptotic Expanions of Integrals. Holt, Rinehart, and Winston.Google Scholar
7. Clarke, A., Weinstein, S. J., Moon, A. G. & Simister, E. A. 1997 Time-dependent equations governing the shape of a two-dimensional liquid curtain. Part 2. Experiment. Phys. Fluids 9 (12), 3637–3644.CrossRefGoogle Scholar
8. Gaster, M. 1968 Growth of disturbances in both space and time. J. Fluid Mech. 11 (4), 723–727.Google Scholar
9. Gordillo, J. M. & Pérez-Saborid, M. 2002 Transient effects in the signaling problem. Phys. Fluids 14 (12), 4329–4343.CrossRefGoogle Scholar
10. Huerre, P. 1987 Spatio-temporal instabilities in closed and open flows. In Instabilities and Non-equilibrium Structures (ed. Tirapegui, E. & Villarroel, D. ), vol. 1, pp. 141–177. D. Reidel.CrossRefGoogle Scholar
11. Huerre, P. 2000 Open shear flow instabilities. In Perspectives in Fluid Dynamics (ed. Batchelor, G. K., Moffatt, H. K. & Worster, M. G. ). Cambridge University Press.Google Scholar
12. Huerre, P. & Monkewitz, P. A. 1990 Local and global instabilities in spatially developing flows. Annu. Rev. Fluid Mech. 22, 473–537.Google Scholar
13. Lighthill, M. J. 1962 Introduction to Fourier Analysis and Generalised Functions. Cambridge University Press.Google Scholar
14. Lighthill, M. J. 1978 Waves in Fluids. Cambridge University Press.Google Scholar
15. Lin, S. P. 2003 Breakup of Liquid Sheets and Jets. Cambridge University Press.CrossRefGoogle Scholar
16. Lin, S. P. & Jiang, W. Y. 2003 Absolute and convective instability of a radially expanding liquid sheet. Phys. Fluids 15 (6), 1745–1754.Google Scholar
17. Monkewitz, P. A. 1990 The role of absolute and convective instability in predicting the behavior of fluid systems. Eur. J. Mech. (B/Fluids) 9 (5), 395–413.Google Scholar
18. Morse, P. M. & Feshbach, H. 1953 Methods of Theoretical Physics. McGraw-Hill Book Company.Google Scholar
19. Rayleigh, Lord 1896 Theory of Sound, vol. 2, 2nd edn. Macmillan and Co.Google Scholar
20. Squire, H. B. 1953 Investigation of the instability of a moving liquid film. Brit. J. Appl. Phys. 4, 167–169.CrossRefGoogle Scholar
21. Taylor, G. I. 1959 The dynamics of thin sheets of fluid. Part II. Waves on fluid sheets. Proc. R. Soc. Lond. A 253, 296–312.Google Scholar
22. Weinberger, H. F. 1965 A First Course in Partial Differential Equations. Xerox College.Google Scholar
23. Weinstein, S. J., Clarke, A., Moon, A. G. & Simister, E. A. 1997 Time-dependent equations governing the shape of a two-dimensional liquid curtain. Part 1. Theory. Phys. Fluids 9 (12), 3625–3636.CrossRefGoogle Scholar
24. Weinstein, S. J. & Ruschak, K. J. 2004 Coating flows. Annu. Rev. Fluid Mech. 36 (29–53).CrossRefGoogle Scholar
25. Whitham, G. B. 1974 Linear and Nonlinear Waves. Wiley-Interscience.Google Scholar