Hostname: page-component-76fb5796d-wq484 Total loading time: 0 Render date: 2024-04-27T02:34:00.993Z Has data issue: false hasContentIssue false
  • English
  • Français

Maximum amplification of enstrophy in three-dimensional Navier–Stokes flows

Published online by Cambridge University Press:  23 April 2020

Di Kang ,
Dongfang Yun  and
Bartosz Protas Open the ORCID record for Bartosz Protas [Opens in a new window]
Di Kang
Affiliation:
Department of Mathematics and Statistics, McMaster University, Hamilton, ONL8S 4K1, Canada
Dongfang Yun
Affiliation:
Department of Mathematics and Statistics, McMaster University, Hamilton, ONL8S 4K1, Canada
Bartosz Protas*
Affiliation:
Department of Mathematics and Statistics, McMaster University, Hamilton, ONL8S 4K1, Canada
*
†Email address for correspondence: bprotas@mcmaster.ca
Get access Rights & Permissions [Opens in a new window]

Abstract

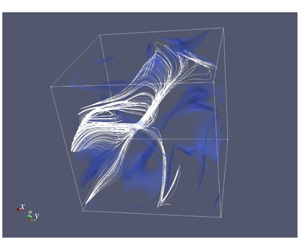
This investigation concerns a systematic search for potentially singular behaviour in three-dimensional (3-D) Navier–Stokes flows. Enstrophy serves as a convenient indicator of the regularity of solutions to the Navier Stokes system – as long as this quantity remains finite, the solutions are guaranteed to be smooth and satisfy the equations in the classical (pointwise) sense. However, there are no estimates available with finite a priori bounds on the growth of enstrophy and, hence, the regularity problem for the 3-D Navier–Stokes system remains open. In order to quantify the maximum possible growth of enstrophy, we consider a family of optimization problems in which initial conditions with prescribed enstrophy ${\mathcal{E}}_{0}$ are sought such that the enstrophy in the resulting Navier–Stokes flow is maximized at some time $T$. Such problems are solved computationally using a large-scale adjoint-based gradient approach derived in the continuous setting. By solving these problems for a broad range of values of ${\mathcal{E}}_{0}$ and $T$, we demonstrate that the maximum growth of enstrophy is in fact finite and scales in proportion to ${\mathcal{E}}_{0}^{3/2}$ as ${\mathcal{E}}_{0}$ becomes large. Thus, in such a worst-case scenario the enstrophy still remains bounded for all times and there is no evidence for formation of singularity in finite time. We also analyse properties of the Navier–Stokes flows leading to the extreme enstrophy values and show that this behaviour is realized by a series of vortex reconnection events.

JFM classification

Mathematical Foundations: Variational methods Vortex Flows: Vortex interactions
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 893 , 25 June 2020 , A22
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adams, R. A. & Fournier, J. F. 2005 Sobolev Spaces. Elsevier.Google Scholar
Ayala, D.2014 Extreme vortex states and singularity formation in incompressible flows. PhD thesis, McMaster University. Available at: http://hdl.handle.net/11375/15453.Google Scholar
Ayala, D., Doering, C. R. & Simon, T. M. 2018 Maximum palinstrophy amplification in the two-dimensional Navier–Stokes equations. J. Fluid Mech. 837, 839–857.CrossRefGoogle Scholar
Ayala, D. & Protas, B. 2011 On maximum enstrophy growth in a hydrodynamic system. Physica D 240, 1553–1563.Google Scholar
Ayala, D. & Protas, B. 2014a Maximum palinstrophy growth in 2D incompressible flows. J. Fluid Mech. 742, 340–367.CrossRefGoogle Scholar
Ayala, D. & Protas, B. 2014b Vortices, maximum growth and the problem of finite-time singularity formation. Fluid Dyn. Res. 46 (3), 031404.Google Scholar
Ayala, D. & Protas, B. 2017 Extreme vortex states and the growth of enstrophy in 3D incompressible flows. J. Fluid Mech. 818, 772–806.CrossRefGoogle Scholar
Beale, J. T., Kato, T. & Majda, A. 1984 Remarks on the breakdown of smooth solutions for the 3-D Euler equations. Commun. Math. Phys. 94 (1), 61–66.CrossRefGoogle Scholar
Berger, M. S. 1977 Nonlinearity and Functional Analysis. Academic Press.Google Scholar
Bewley, T. R. 2009 Numerical Renaissance. Renaissance Press.Google Scholar
Biryuk, A. È. 2001 Spectral properties of solutions of the burgers equation with small dissipation. Funct. Anal. Applics 35 (1), 1–12.CrossRefGoogle Scholar
Brachet, M. E. 1991 Direct simulation of three-dimensional turbulence in the Taylor–Green vortex. Fluid Dyn. Res. 8, 1–8.CrossRefGoogle Scholar
Brachet, M. E., Meiron, D. I., Orszag, S. A., Nickel, B. G., Morf, R. H. & Frisch, U. 1983 Small-scale structure of the Taylor–Green vortex. J. Fluid Mech. 130, 411–452.CrossRefGoogle Scholar
Bustamante, M. D. & Brachet, M. 2012 Interplay between the Beale–Kato–Majda theorem and the analyticity-strip method to investigate numerically the incompressible Euler singularity problem. Phys. Rev. E 86, 066302.Google ScholarPubMed
Bustamante, M. D. & Kerr, R. M. 2008 3-D Euler about a 2D symmetry plane. Physica D 237, 1912–1920.Google Scholar
Caffarelli, L., Kohn, R. & Nirenberg, L. 1982 Partial regularity of suitable weak solutions of the Navier–Stokes equations. Commun. Pure Appl. Maths 35 (6), 771–831.CrossRefGoogle Scholar
Campolina, C. S. & Mailybaev, A. A. 2018 Chaotic blowup in the 3D incompressible euler equations on a logarithmic lattice. Phys. Rev. Lett. 121, 064501.CrossRefGoogle ScholarPubMed
Chernyshenko, S. I., Goulart, P., Huang, D. & Papachristodoulou, A. 2014 Polynomial sum of squares in fluid dynamics: a review with a look ahead. Phil. Trans. R. Soc. Lond. A 372 (2020), 20130350.Google ScholarPubMed
Davidson, P. A. 2004 Turbulence. An Introduction for Scientists and Engineers. Oxford University Press.Google Scholar
Doering, C. R. 2009 The 3-D Navier–Stokes problem. Annu. Rev. Fluid Mech. 41, 109–128.CrossRefGoogle Scholar
Doering, C. R. & Constantin, P. 1992 Energy dissipation in shear driven turbulence. Phys. Rev. Lett. 69, 1648–1651.CrossRefGoogle ScholarPubMed
Doering, C. R. & Gibbon, J. D. 1995 Applied Analysis of the Navier–Stokes Equations. Cambridge University Press.CrossRefGoogle Scholar
Donzis, D. A., Gibbon, J. D., Gupta, A., Kerr, R. M., Pandit, R. & Vincenzi, D. 2013 Vorticity moments in four numerical simulations of the 3D Navier–Stokes equations. J. Fluid Mech. 732, 316–331.CrossRefGoogle Scholar
Elgindi, T. M. & Jeong, I. 2019 Finite-time singularity formation for strong solutions to the axi-symmetric 3D Euler equations. Ann. PDE 5, 16.CrossRefGoogle Scholar
Fantuzzi, G. & Goluskin, D.2019 Bounding extreme events in nonlinear dynamics using convex optimization. arXiv:1907.10997.Google Scholar
Fefferman, C. L.2000 Existence and smoothness of the Navier–Stokes equation. Available at: http://www.claymath.org/sites/default/files/navierstokes.pdf. Clay Millennium Prize Problem Description.Google Scholar
Feng, H. & Šverák, V. 2015 On the Cauchy problem for axi-symmetric vortex rings. Arch. Rat. Mech. Anal. 215 (1), 89–123.CrossRefGoogle Scholar
Foias, C. & Temam, R. 1989 Gevrey class regularity for the solutions of the Navier–Stokes equations. J. Funct. Anal. 87, 359–369.CrossRefGoogle Scholar
Frigo, M. & Johnson, S. G. 2003 FFTW User’s Manual. Massachusetts Institute of Technology, Cambridge, MA.Google Scholar
Gibbon, J. D. 2019 Weak and strong solutions of the 3D Navier–Stokes equations and their relation to a chessboard of convergent inverse length scales. J. Nonlinear Sci. 29 (1), 215–228.CrossRefGoogle Scholar
Gibbon, J. D., Bustamante, M. & Kerr, R. M. 2008 The three-dimensional Euler equations: singular or non-singular? Nonlinearity 21, 123–129.CrossRefGoogle Scholar
Gibbon, J. D., Donzis, D., Gupta, A., Kerr, R. M., Pandit, R. & Vincenzi, D. 2014 Regimes of nonlinear depletion and regularity in the 3D Navier–Stokes equations. Nonlinearity 27 (10), 2605–2625.CrossRefGoogle Scholar
Goluskin, D. 2018 Bounding averages rigorously using semidefinite programming: mean moments of the Lorenz system. J. Nonlinear Sci. 28 (2), 621–651.CrossRefGoogle Scholar
Goluskin, D. & Fantuzzi, G. 2019 Bounds on mean energy in the Kuramoto–Sivashinsky equation computed using semidefinite programming. Nonlinearity 32 (5), 1705–1730.CrossRefGoogle Scholar
Grafke, T., Homann, H., Dreher, J. & Grauer, R. 2008 Numerical simulations of possible finite-time singularities in the incompressible Euler equations: comparison of numerical methods. Physica D 237, 1932–1936.Google Scholar
Gunzburger, M. D. 2003 Perspectives in Flow Control and Optimization. SIAM.Google Scholar
Hou, T. Y. 2009 Blow-up or no blow-up? A unified computational and analytic approach to 3D incompressible Euler and Navier–Stokes equations. Acta Numerica 18, 277–346.CrossRefGoogle Scholar
Hou, T. Y. & Li, R. 2007 Computing nearly singular solutions using pseudo-spectral methods. J. Comput. Phys. 226, 379–397.CrossRefGoogle Scholar
Hussain, F. & Duraisamy, K. 2011 Mechanics of viscous vortex reconnection. Phys. Fluids 23 (2), 021701.CrossRefGoogle Scholar
Jaque, R. S. & Fuentes, O. V. 2017 Reconnection of orthogonal cylindrical vortices. Eur. J. Mech. (B/Fluids) 62, 51–56.CrossRefGoogle Scholar
Kerr, R. M. 1993 Evidence for a singularity of the three-dimensional, incompressible Euler equations. Phys. Fluids A 5, 1725–1746.CrossRefGoogle Scholar
Kerr, R. M. 2013a Bounds for Euler from vorticity moments and line divergence. J. Fluid Mech. 729, R2.CrossRefGoogle Scholar
Kerr, R. M. 2013b Swirling, turbulent vortex rings formed from a chain reaction of reconnection events. Phys. Fluids 25, 065101.CrossRefGoogle Scholar
Kerr, R. M. 2018 Enstrophy and circulation scaling for Navier–Stokes reconnection. J. Fluid Mech. 839, R2.CrossRefGoogle Scholar
Kiselev, A. 2010 Regularity and blow up for active scalars. Math. Model. Nat. Phenom. 5, 225–255.CrossRefGoogle Scholar
Kiselev, A., Nazaraov, F. & Shterenberg, R. 2008 Blow up and regularity for fractal Burgers equation. Dyn. Partial Differ. Equ. 5, 211–240.CrossRefGoogle Scholar
Kiselev, A. A. & Ladyzhenskaya, O. A. 1957 On the existence and uniqueness of the solution of the nonstationary problem for a viscous, incompressible fluid. Izv. Akad. Nauk SSSR Ser. Mat 21 (5), 655–680.Google Scholar
Kreiss, H. & Lorenz, J. 2004 Initial-Boundary Value Problems and the Navier–Stokes Equations, Classics in Applied Mathematics, vol. 47. SIAM.CrossRefGoogle Scholar
Leray, J. 1934 Sur le mouvement d’un liquide visqu’eux emplissant l’espace. Acta Mathematica 63 (1), 193–248.CrossRefGoogle Scholar
Lu, L.2006 Bounds on the enstrophy growth rate for solutions of the 3D Navier–Stokes equations. PhD thesis, University of Michigan, Ann Arbor, MI.Google Scholar
Lu, L. & Doering, C. R. 2008 Limits on enstrophy growth for solutions of the three-dimensional Navier–Stokes equations. Indiana Univ. Math. J. 57, 2693–2727.CrossRefGoogle Scholar
Luenberger, D. 1969 Optimization by Vector Space Methods. John Wiley & Sons.Google Scholar
Luo, G. & Hou, T. Y. 2014a Potentially singular solutions of the 3D axisymmetric Euler equations. Proc. Natl Acad. Sci. USA 111 (36), 12968–12973.CrossRefGoogle Scholar
Luo, G. & Hou, T. Y. 2014b Toward the finite-time blowup of the 3D incompressible Euler equations: a numerical investigation. SIAM Multiscale Model. Simul. 12 (4), 1722–1776.CrossRefGoogle Scholar
Matsumoto, T., Bec, J. & Frisch, U. 2008 Complex-space singularities of 2D Euler flow in Lagrangian coordinates. Physica D 237, 1951–1955.Google Scholar
Melander, M. V. & Hussain, F. 1989 Cross-linking of two antiparallel vortex tubes. Phys. Fluids A 1 (4), 633–636.CrossRefGoogle Scholar
Miller, E. 2020 A regularity criterion for the Navier–Stokes equation involving only the middle eigenvalue of the strain tensor. Arch Rat. Mech. Anal 235 (1), 99–139.CrossRefGoogle Scholar
Moffatt, H. K. & Kimura, Y. 2019a Towards a finite-time singularity of the Navier–Stokes equations. Part 1. Derivation and analysis of dynamical system. J. Fluid Mech. 861, 930–967.CrossRefGoogle Scholar
Moffatt, H. K. & Kimura, Y. 2019b Towards a finite-time singularity of the Navier–Stokes equations. Part 2. Vortex reconnection and singularity evasion. J. Fluid Mech. 870, R1.CrossRefGoogle Scholar
Nocedal, J. & Wright, S. J. 1999 Numerical Optimization. Springer.CrossRefGoogle Scholar
Ohkitani, K. 2008 A miscellany of basic issues on incompressible fluid equations. Nonlinearity 21, 255–271.CrossRefGoogle Scholar
Ohkitani, K. 2016 Late formation of singularities in solutions to the Navier–Stokes equations. J. Phys. A 49 (1), 015502.Google Scholar
Ohkitani, K. & Constantin, P. 2008 Numerical study of the Eulerian–Lagrangian analysis of the Navier–Stokes turbulence. Phys. Fluids 20, 1–11.CrossRefGoogle Scholar
Orlandi, P., Pirozzoli, S., Bernardini, M. & Carnevale, G. F. 2014 A minimal flow unit for the study of turbulence with passive scalars. J. Turbul. 15, 731–751.CrossRefGoogle Scholar
Orlandi, P., Pirozzoli, S. & Carnevale, G. F. 2012 Vortex events in Euler and Navier–Stokes simulations with smooth initial conditions. J. Fluid Mech. 690, 288–320.CrossRefGoogle Scholar
Pelinovsky, D. 2012 Sharp bounds on enstrophy growth in the viscous Burgers equation. Proc. R. Soc. Lond. A 468, 3636–3648.CrossRefGoogle Scholar
Pelz, R. B. 2001 Symmetry and the hydrodynamic blow-up problem. J. Fluid Mech. 444, 299–320.CrossRefGoogle Scholar
Poças, D. & Protas, B. 2018 Transient growth in stochastic Burgers flows. J. Discrete Continuous Dyn. Syst. B 23, 2371–2391.CrossRefGoogle Scholar
Press, W. H., Flannery, B. P., Teukolsky, S. A. & Vetterling, W. T. 1986 Numerical Recipes. Cambridge University Press.Google Scholar
Prodi, G. 1959 Un teorema di unicità per le equazioni di Navier–Stokes. Ann. Math. Pura Appl. 48 (1), 173–182.CrossRefGoogle Scholar
Protas, B. 2008 Adjoint-based optimization of PDE systems with alternative gradients. J. Comput. Phys. 227, 6490–6510.CrossRefGoogle Scholar
Protas, B., Bewley, T. & Hagen, G. 2004 A comprehensive framework for the regularization of adjoint analysis in multiscale PDE systems. J. Comput. Phys. 195, 49–89.CrossRefGoogle Scholar
Pumir, A. & Siggia, E. 1990 Collapsing solutions to the 3D Euler equations. Phys. Fluids A 2, 220–241.CrossRefGoogle Scholar
Robinson, J. C., Rodrigo, J. L. & Sadowski, W. 2016 The Three-Dimensional Navier–Stokes Equations: Classical Theory. Cambridge University Press.CrossRefGoogle Scholar
Schumacher, J., Eckhardt, B. & Doering, C. R. 2010 Extreme vorticity growth in Navier–Stokes turbulence. Phys. Lett. A 374 (6), 861–865.CrossRefGoogle Scholar
Serrin, J. 1962 On the interior regularity of weak solutions of the Navier–Stokes equations. Arch. Rat. Mech. Anal. 9 (1), 187–195.CrossRefGoogle Scholar
Siegel, M. & Caflisch, R. E. 2009 Calculation of complex singular solutions to the 3D incompressible Euler equations. Physica D 238, 2368–2379.Google Scholar
Tao, T. 2016 Finite time blowup for an averaged three-dimensional Navier–Stokes equation. J. Am. Math. 29, 601–674.CrossRefGoogle Scholar
Tobasco, I., Goluskin, D. & Doering, C. R. 2018 Optimal bounds and extremal trajectories for time averages in nonlinear dynamical systems. Phys. Lett. A 382 (6), 382–386.CrossRefGoogle Scholar
Yun, D. & Protas, B. 2018 Maximum rate of growth of enstrophy in solutions of the fractional Burgers equation. J. Nonlinear Sci. 28 (1), 395–422.CrossRefGoogle Scholar

Kang et al. supplementary movie 1

Time evolution of the vorticity components (blue) $\widetilde{\omega}_1$, (red) \widetilde{\omega}_2$ and (green) $\widetilde{\omega}_3$ in the solution of the Navier-Stokes system (2.1) with the optimal asymmetric initial condition $\widetilde{\mathbf{u}}_{0;\mathcal{E}_0,T}$ obtained by solving the finite-time optimization problem 3,1 for the initial enstrophy $\mathcal{E}_0 = 500$ and $\widetilde{T}_{\mathcal{E}_0} = 0.17$, cf. figure 13. The animation covers the time interval $[0,widetilde{T}_{\mathcal{E}_0}]$.

Download Kang et al. supplementary movie 1(Video)
Video 9.2 MB

Kang et al. supplementary movie 2

Time evolution of the vorticity components (blue) $\widetilde{\omega}_1$, (red) $\widetilde{\omega}_2$ and (green) $\widetilde{\omega}_3$ in the solution of the Navier-Stokes system (2.1) with the optimal asymmetric initial condition $\widetilde{\mathbf{u}}_{0;\mathcal{E}_0,T}$ obtained by solving the finite-time optimization problem 3,1 for the initial enstrophy $\mathcal{E}_0 = 300$ and $\widetilde{T}_{\mathcal{E}_0} = 0.21$. The animation covers the time interval $[0,widetilde{T}_{\mathcal{E}_0}]$.

Download Kang et al. supplementary movie 2(Video)
Video 9.7 MB

Kang et al. supplementary movie 3

Time evolution of the vorticity components (blue) $\widetilde{\omega}_1$, (red) $\widetilde{\omega}_2$ and (green) $\widetilde{\omega}_3$ in the solution of the Navier-Stokes system (2.1) with the optimal asymmetric initial condition $\widetilde{\mathbf{u}}_{0;\mathcal{E}_0,T}$ obtained by solving the finite-time optimization problem 3,1 for the initial enstrophy $\mathcal{E}_0 = 1000$ and $\widetilde{T}_{\mathcal{E}_0} = 0.12$. The animation covers the time interval $[0,widetilde{T}_{\mathcal{E}_0}]$.

Download Kang et al. supplementary movie 3(Video)
Video 7 MB