Hostname: page-component-848d4c4894-wzw2p Total loading time: 0 Render date: 2024-05-09T18:33:32.742Z Has data issue: false hasContentIssue false
  • English
  • Français

Energy fluxes in quasi-equilibrium flows

Published online by Cambridge University Press:  17 December 2019

Alexandros Alexakis Open the ORCID record for Alexandros Alexakis [Opens in a new window]  and
Marc-Etienne Brachet Open the ORCID record for Marc-Etienne Brachet [Opens in a new window]
Alexandros Alexakis*
Affiliation:
Laboratoire de Physique de l’École Normale Supérieure, ENS, Université PSL, CNRS, Sorbonne Université, Université de Paris, F-75005Paris, France
Marc-Etienne Brachet
Affiliation:
Laboratoire de Physique de l’École Normale Supérieure, ENS, Université PSL, CNRS, Sorbonne Université, Université de Paris, F-75005Paris, France
*
†Email address for correspondence: alexakis@phys.ens.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

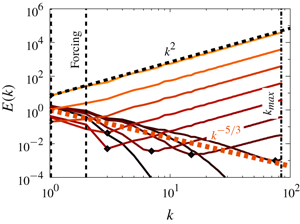
We examine the relation between the absolute equilibrium state of the spectrally truncated Euler equations (TEE) predicted by Kraichnan (J. Fluid Mech., vol. 59 (4), 1973, pp. 745–752) to the forced and dissipated flows of the spectrally truncated Navier–Stokes (TNS) equations. In both of these idealized systems, a finite number of Fourier modes is kept contained inside a sphere of radius $k_{max}$, but, while the first conserves energy, in the second, energy is injected by a body-force $\boldsymbol{f}$ and dissipated by the viscosity $\unicode[STIX]{x1D708}$. For the TNS system, stochastically forced with energy injection rate ${\mathcal{I}}_{{\mathcal{E}}}$, we show, using an asymptotic expansion of the Fokker-Planck equation, that in the limit of small $k_{max}\unicode[STIX]{x1D702}$ (where $\unicode[STIX]{x1D702}=(\unicode[STIX]{x1D708}^{3}/{\mathcal{I}}_{{\mathcal{E}}})^{1/4}$, the Kolmogorov length scale) the flow approaches the absolute equilibrium solution of Kraichnan with an effective ‘temperature’ such that there is a balance between the energy injection and the energy dissipation rate. We further investigate the TNS system using direct numerical simulations in periodic cubic boxes of size $2\unicode[STIX]{x03C0}/k_{0}$. The simulations verify the predictions of the model for small values of $k_{max}\unicode[STIX]{x1D702}$. For intermediate values of $k_{max}\unicode[STIX]{x1D702}$, a transition from the quasi-equilibrium ‘thermal’ state to Kolmogorov turbulence is observed. In particular, we demonstrate that, at steady state, the TNS system reproduces the Kolmogorov energy spectrum if $k_{max}\unicode[STIX]{x1D702}\gg 1$. As $k_{max}\unicode[STIX]{x1D702}$ becomes smaller, then a bottleneck effect appears, taking the form of the equipartition spectrum $E(k)\propto k^{2}$ at small scales. As $k_{max}\unicode[STIX]{x1D702}$ is decreased even further, so that $k_{max}\unicode[STIX]{x1D702}\ll (k_{0}/k_{max})^{11/4}$, the equipartition spectrum occupies all scales approaching the asymptotic equilibrium solutions found before. If the forcing is applied at small scales and the dissipation acts only at large scales, then the equipartition spectrum appears at all scales for all values of $\unicode[STIX]{x1D708}$. In both cases, a finite forward or inverse flux is present even for the cases where the flow is close to the equilibrium state solutions. However, unlike the classical turbulence, where an energy cascade develops with a mean energy flux that is large compared to its fluctuations, the quasi-equilibrium state has a mean flux of energy that is subdominant to the large flux fluctuations observed.

JFM classification

Turbulent Flows: Homogeneous turbulence Turbulent Flows: Turbulence theory
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 884 , 10 February 2020 , A33
Copyright
© 2019 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alexakis, A. 2017 Helically decomposed turbulence. J. Fluid Mech. 812, 752–770.CrossRefGoogle Scholar
Alexakis, A. & Biferale, L. 2018 Cascades and transitions in turbulent flows. Phys. Rep. 767–769, 1–101.CrossRefGoogle Scholar
Alexakis, A. & Brachet, M.-E. 2019 On the thermal equilibrium state of large scale flows. J. Fluid Mech. 872, 594–625.CrossRefGoogle Scholar
Benavides, S. J. & Alexakis, A. 2017 Critical transitions in thin layer turbulence. J. Fluid Mech. 822, 364–385.CrossRefGoogle Scholar
Biferale, L., Cencini, M., De Pietro, M., Gallavotti, G. & Lucarini, V. 2018 Equivalence of nonequilibrium ensembles in turbulence models. Phys. Rev. E 98 (1), 012202.Google ScholarPubMed
Biferale, L., Musacchio, S. & Toschi, F. 2012 Inverse energy cascade in three-dimensional isotropic turbulence. Phys. Rev. Lett. 108 (16), 164501.CrossRefGoogle ScholarPubMed
Bouchet, F. & Venaille, A. 2012 Statistical mechanics of two-dimensional and geophysical flows. Phys. Rep. 515 (5), 227–295.CrossRefGoogle Scholar
Cambon, C. & Jacquin, L. 1989 Spectral approach to non-isotropic turbulence subjected to rotation. J. Fluid Mech. 202, 295–317.CrossRefGoogle Scholar
Cameron, A., Alexakis, A. & Brachet, M.-É. 2017 Effect of helicity on the correlation time of large scales in turbulent flows. Phys. Rev. Fluids 2 (11), 114602.CrossRefGoogle Scholar
Chen, Q., Chen, S. & Eyink, G. L. 2003 The joint cascade of energy and helicity in three-dimensional turbulence. Phys. Fluids 15 (2), 361–374.CrossRefGoogle Scholar
Cichowlas, C., Bonaïti, P., Debbasch, F. & Brachet, M. 2005 Effective dissipation and turbulence in spectrally truncated Euler flows. Phys. Rev. Lett. 95 (26), 264502.CrossRefGoogle ScholarPubMed
Craya, A. 1958 Contributiona l’analyse de la turbulence associéea des vitesses moyennes. pub. Sci. Tech. du Ministere de l’Air (France) (345).Google Scholar
Dallas, V., Fauve, S. & Alexakis, A. 2015 Statistical equilibria of large scales in dissipative hydrodynamic turbulence. Phys. Rev. Lett. 115 (20), 204501.CrossRefGoogle ScholarPubMed
Deusebio, E., Boffetta, G., Lindborg, E. & Musacchio, S. 2014 Dimensional transition in rotating turbulence. Phys. Rev. E 90 (2), 023005.Google ScholarPubMed
Donzis, D. A. & Sreenivasan, K. R. 2010 The bottleneck effect and the Kolmogorov constant in isotropic turbulence. J. Fluid Mech. 657, 171–188.CrossRefGoogle Scholar
Falkovich, G. 1994 Bottleneck phenomenon in developed turbulence. Phys. Fluids 6 (4), 1411–1414.CrossRefGoogle Scholar
Frisch, U., Kurien, S., Pandit, R., Pauls, W., Ray, S. S., Wirth, A. & Zhu, J.-Z. 2008 Hyperviscosity, Galerkin truncation, and bottlenecks in turbulence. Phys. Rev. Lett. 101 (14), 144501.CrossRefGoogle ScholarPubMed
Gallavotti, G. 1996 Equivalence of dynamical ensembles and Navier–Stokes equations. Phys. Lett. A 223 (1–2), 91–95.CrossRefGoogle Scholar
Herring, J. R. 1974 Approach of axisymmetric turbulence to isotropy. Phys. Fluids 17 (5), 859–872.CrossRefGoogle Scholar
Hopf, E. 1952 Statistical hydromechanics and functional calculus. J. Rat. Mech. Anal. 1, 87–123.Google Scholar
Kraichnan, R. H. 1967 Inertial ranges in two-dimensional turbulence. Phys. Fluids 10 (7), 1417–1423.CrossRefGoogle Scholar
Kraichnan, R. H. 1973 Helical turbulence and absolute equilibrium. J. Fluid Mech. 59 (4), 745–752.CrossRefGoogle Scholar
Krstulovic, G., Mininni, P. D., Brachet, M. E. & Pouquet, A. 2009 Cascades, thermalization, and eddy viscosity in helical Galerkin truncated Euler flows. Phys. Rev. E 79 (5), 056304.Google ScholarPubMed
Lee, T. D. 1952 On some statistical properties of hydrodynamical and magneto-hydrodynamical fields. Q. Appl. Maths 10 (1), 69–74.CrossRefGoogle Scholar
Lesieur, M.1972 Décomposition d’un champ de vitesse non divergent en ondes d’hélicité. Tech. Rep. Observatoire de Nice.Google Scholar
Lohse, D. & Müller-Groeling, A. 1995 Bottleneck effects in turbulence: scaling phenomena in r versus p space. Phys. Rev. Lett. 74 (10), 1747.CrossRefGoogle Scholar
Martinez, D. O., Chen, S., Doolen, G. D., Kraichnan, R. H., Wang, L.-P. & Zhou, Y. 1997 Energy spectrum in the dissipation range of fluid turbulence. J. Plasma Phys. 57 (1), 195–201.CrossRefGoogle Scholar
Mininni, P. D., Rosenberg, D., Reddy, R. & Pouquet, A. 2011 A hybrid MPI–OpenMP scheme for scalable parallel pseudospectral computations for fluid turbulence. Parallel Comput. 37 (6–7), 316–326.CrossRefGoogle Scholar
Moffatt, H. K. 2014 Note on the triad interactions of homogeneous turbulence. J. Fluid Mech. 741, R3.CrossRefGoogle Scholar
Naso, A., Chavanis, P.-H. & Dubrulle, B. 2010 Statistical mechanics of two-dimensional Euler flows and minimum enstrophy states. Eur. Phys. J. B 77 (2), 187–212.CrossRefGoogle Scholar
Orszag, S. A. 1977 Statistical theory of turbulence. In Fluid Dynamics, Les Houches 1973 (ed. Balian, R. & Peube, J. L.), Gordon and Breach.Google Scholar
Orszag, S. A. & Patterson, G. S. 1977 Numerical simulation of turbulence. In Statistical Models and Turbulence (ed. Rosenblatt, M. & Van Atta, C.), Lecture Notes in Physics, vol. 12. Springer.Google Scholar
Robert, R. & Sommeria, J. 1991 Statistical equilibrium states for two-dimensional flows. J. Fluid Mech. 229, 291–310.CrossRefGoogle Scholar
Sahoo, G., Alexakis, A. & Biferale, L. 2017 Discontinuous transition from direct to inverse cascade in three-dimensional turbulence. Phys. Rev. Lett. 118 (16), 164501.CrossRefGoogle ScholarPubMed
Seshasayanan, K., Benavides, S. J. & Alexakis, A. 2014 On the edge of an inverse cascade. Phys. Rev. E 90 (5), 051003.Google ScholarPubMed
Shukla, V., Dubrulle, B., Nazarenko, S., Krstulovic, G. & Thalabard, S. 2019 Phase transition in time-reversible Navier–Stokes equations. Phys. Rev. E 100, 043104.Google ScholarPubMed
Shukla, V., Fauve, S. & Brachet, M. 2016 Statistical theory of reversals in two-dimensional confined turbulent flows. Phys. Rev. E 94 (6), 061101.Google ScholarPubMed
Sozza, A., Boffetta, G., Muratore-Ginanneschi, P. & Musacchio, S. 2015 Dimensional transition of energy cascades in stably stratified forced thin fluid layers. Phys. Fluids 27 (3), 035112.CrossRefGoogle Scholar
Sreenivasan, K. R. 1995 On the universality of the Kolmogorov constant. Phys. Fluids 7 (11), 2778–2784.CrossRefGoogle Scholar
Waleffe, F. 1992 The nature of triad interactions in homogeneous turbulence. Phys. Fluids 4 (2), 350–363.CrossRefGoogle Scholar