Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-21T13:58:47.314Z Has data issue: false hasContentIssue false
  • English
  • Français

Cross-stream stereoscopic particle image velocimetry of a modified turbulent boundary layer over directional surface pattern

Published online by Cambridge University Press:  23 January 2017

Kevin Kevin ,
J. P. Monty ,
H. L. Bai ,
G. Pathikonda ,
B. Nugroho ,
J. M. Barros ,
K. T. Christensen  and
N. Hutchins
Kevin Kevin*
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Parkville, Victoria 3010, Australia
J. P. Monty
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Parkville, Victoria 3010, Australia
H. L. Bai
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Parkville, Victoria 3010, Australia Department of Civil and Environmental Engineering, Hong Kong University of Science and Technology, Clear Water Bay, Kowloon, Hong Kong
G. Pathikonda
Affiliation:
Department of Mechanical Science and Engineering, University of Illinois at Urbana-Champaign, Urbana, IL 61801, USA Department of Aerospace and Mechanical Engineering, University of Notre Dame, Notre Dame, IN 46556, USA
B. Nugroho
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Parkville, Victoria 3010, Australia
J. M. Barros
Affiliation:
Department of Mechanical Science and Engineering, University of Illinois at Urbana-Champaign, Urbana, IL 61801, USA Department of Mechanical Engineering, United States Naval Academy, Annapolis, MD 21402, USA
K. T. Christensen
Affiliation:
Department of Mechanical Science and Engineering, University of Illinois at Urbana-Champaign, Urbana, IL 61801, USA Department of Aerospace and Mechanical Engineering, University of Notre Dame, Notre Dame, IN 46556, USA International Institute for Carbon Neutral Energy Research (WPI-I2CNER), Kyushu University, Fukuoka 819-0395, Japan
N. Hutchins
Affiliation:
Department of Mechanical Engineering, University of Melbourne, Parkville, Victoria 3010, Australia
*
†Email address for correspondence: kevin.kevin@unimelb.edu.au
Get access Rights & Permissions [Opens in a new window]

Abstract

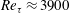A turbulent boundary layer developed over a herringbone patterned riblet surface is investigated using stereoscopic particle image velocimetry in the cross-stream plane at $Re_{\unicode[STIX]{x1D70F}}\approx 3900$. The three velocity components resulting from this experiment reveal a pronounced spanwise periodicity in all single-point velocity statistics. Consistent with previous hot-wire studies over similar-type riblets, we observe a weak time-average secondary flow in the form of 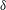$\unicode[STIX]{x1D6FF}$-filling streamwise vortices. The observed differences in the surface and secondary flow characteristics, compared to other heterogeneous-roughness studies, may suggest that different mechanisms are responsible for the flow modifications in this case. Observations of instantaneous velocity fields reveal modified and rearranged turbulence structures. The instantaneous snapshots also suggest that the time-average secondary flow may be an artefact arising from superpositions of much stronger instantaneous turbulent events enhanced by the surface texture. In addition, the observed instantaneous secondary motions seem to have promoted a free-stream-engulfing behaviour in the outer layer, which would indicate an increase turbulent/non-turbulent flow mixing. It is overall demonstrated that the presence of large-scale directionality in transitional surface roughness can cause a modification throughout the entire boundary layer, even when the roughness height is 0.5 % of the layer thickness.

JFM classification

Boundary Layers: Boundary layer structure Turbulent Flows: Turbulence control Turbulent Flows: Turbulent boundary layers
Type
Papers
Information
Journal of Fluid Mechanics , Volume 813 , 25 February 2017 , pp. 412 - 435
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Acharya, M., Bornstein, J. & Escudier, M. P. 1986 Turbulent boundary layers on rough surfaces. Exp. Fluids 4 (1), 33–47.CrossRefGoogle Scholar
Adrian, R. J., Christensen, K. T. & Liu, Z. C. 2000a Analysis and interpretation of instantaneous turbulent velocity fields. Exp. Fluids 29 (3), 275–290.CrossRefGoogle Scholar
Adrian, R. J., Meinhart, C. D. & Tomkins, C. D. 2000b Vortex organization in the outer region of the turbulent boundary layer. J. Fluid Mech. 422, 1–54.Google Scholar
del Álamo, J. C., Jiménez, J., Zandonade, P. & Moser, R. D. 2006 Self-similar vortex clusters in the turbulent logarithmic region. J. Fluid Mech. 561, 329–358.Google Scholar
Anders, J. B. 1990 Outer-layer manipulators for turbulent drag reduction. Visc. Drag Reduc. Boundary Layers 123, 263–284.Google Scholar
Anderson, W., Barros, J. M., Christensen, K. T. & Awasthi, A. 2015 Numerical and experimental study of mechanisms responsible for turbulent secondary flows in boundary layer flows over spanwise heterogeneous roughness. J. Fluid Mech. 768, 316–347.Google Scholar
Barros, J. M. & Christensen, K. T. 2014 Observations of turbulent secondary flows in a rough-wall boundary layer. J. Fluid Mech. 748, R1.CrossRefGoogle Scholar
Bechert, D. W. & Bartenwerfer, M. 1989 The viscous flow on surfaces with longitudinal ribs. J. Fluid Mech. 206, 105–129.Google Scholar
Bechert, D. W., Bruse, M. & Hage, W. 2000 Experiments with three-dimensional riblets as an idealized model of shark skin. Exp. Fluids 28 (5), 403–412.Google Scholar
Bechert, D. W., Bruse, M., Hage, W., der Hoeven, J. G. T. Van & Hoppe, G. 1997 Experiments on drag-reducing surfaces and their optimization with an adjustable geometry. J. Fluid Mech. 338, 59–87.Google Scholar
Chan, L., MacDonald, M., Chung, D., Hutchins, N. & Ooi, A. 2015 A systematic investigation of roughness height and wavelength in turbulent pipe flow in the transitionally rough regime. J. Fluid Mech. 771, 743–777.Google Scholar
Chauhan, K., Philip, J., de Silva, C. M., Hutchins, N. & Marusic, I. 2014 The turbulent/non-turbulent interface and entrainment in a boundary layer. J. Fluid Mech. 742, 119–151.CrossRefGoogle Scholar
Chen, H., Rao, F., Shang, X., Zhang, D. & Hagiwara, I. 2014 Flow over bio-inspired 3d herringbone wall riblets. Exp. Fluids 55 (3), 1–7.Google Scholar
Choi, K. S. 1989 Near wall structure of turbulent boundary layer with riblets. J. Fluid Mech. 208, 417–458.Google Scholar
Coceal, O., Thomas, T. G., Castro, I. P. & Belcher, S. E. 2006 Mean flow and turbulence statistics over groups of urban-like cubical obstacles. Boundary-Layer Meteorol. 121 (3), 491–519.Google Scholar
Eitel-Amor, G., Örlü, R. & Schlatter, P. 2014 Simulation and validation of a spatially evolving turbulent boundary layer up to Re 𝜃 = 8300. Intl J. Heat Fluid Flow 47, 57–69.Google Scholar
Finnigan, J. J. 2000 Turbulence in plant canopies. Annu. Rev. Fluid Mech. 32 (1), 519–571.Google Scholar
Flack, K. A. & Schultz, M. P. 2010 Review of hydraulic roughness scales in the fully rough regime. J. Fluids Engng 132 (4), 041203.Google Scholar
Flack, K. A., Schultz, M. P. & Shapiro, T. A. 2005 Experimental support for Townsend’s Reynolds number similarity hypothesis on rough walls. Phys. Fluids 17 (3), 035102.CrossRefGoogle Scholar
García-Mayoral, R. & Jiménez, J. 2011 Hydrodynamic stability and breakdown of the viscous regime over riblets. J. Fluid Mech. 678, 317–347.CrossRefGoogle Scholar
Granville, P. S.1958 The frictional resistance and turbulent boundary layer of rough surfaces. Tech. Rep. 1024.Google Scholar
Hinze, J. 1967 Secondary currents in wall turbulence. Phys. Fluids (Suppl.) 10, S122–S125.Google Scholar
Hinze, J. O. 1973 Experimental investigation on secondary currents in the turbulent flow through a straight conduit. Appl. Sci. Res. 28 (1), 453–465.Google Scholar
Hutchins, N., Hambleton, W. T. & Marusic, I. 2005 Inclined cross-stream stereo particle image velocimetry measurements in turbulent boundary layers. J. Fluid Mech. 541, 21–54.Google Scholar
Hutchins, N. & Marusic, I. 2007 Evidence of very long meandering features in the logarithmic region of turbulent boundary layers. J. Fluid Mech. 579, 1–28.Google Scholar
Jiménez, J. 2004 Turbulent flows over rough walls. Annu. Rev. Fluid Mech. 36, 173–196.CrossRefGoogle Scholar
Kamrin, K., Bazant, M. Z. & Stone, H. A. 2010 Effective slip boundary conditions for arbitrary periodic surfaces: the surface mobility tensor. J. Fluid Mech. 658, 409–437.Google Scholar
Kim, K. C. & Adrian, R. J. 1999 Very large-scale motion in the outer layer. Phys. Fluids 11 (2), 417–422.Google Scholar
Koeltzsch, K., Dinkelacker, A. & Grundmann, R. 2002 Flow over convergent and divergent wall riblets. Exp. Fluids 33 (2), 346–350.CrossRefGoogle Scholar
Lee, J. H., Kevin, Monty, J. P. & Hutchins, N. 2016 Validating under-resolved turbulence intensities for PIV experiments in canonical wall-bounded turbulence. Exp. Fluids.CrossRefGoogle Scholar
Luchini, P., Manzo, F. & Pozzi, A. 1991 Resistance of a grooved surface to parallel flow and cross-flow. J. Fluid Mech. 228, 87–109.Google Scholar
Mejia-Alvarez, R. & Christensen, K. T. 2013 Wall-parallel stereo particle-image velocimetry measurements in the roughness sublayer of turbulent flow overlying highly irregular roughness. Phys. Fluids 25 (1), 115109.Google Scholar
Monty, J. P., Stewart, J. A., Williams, R. C. & Chong, M. S. 2007 Large-scale features in turbulent pipe and channel flows. J. Fluid Mech. 589, 147–156.Google Scholar
Moody, L. F. 1944 Friction factors for pipe flow. Trans. ASME 66 (8), 671–684.Google Scholar
Napoli, E., Armenio, V. & Marchis, M. D. 2008 The effect of the slope of irregularly distributed roughness elements on turbulent wall-bounded flows. J. Fluid Mech. 613, 385–394.Google Scholar
Nugroho, B., Gnanamanickam, E. P., Kevin, Monty, J. P. & Hutchins, N.2014 Roll-modes generated in turbulent boundary layers with passive surface modifications. AIAA paper.Google Scholar
Nugroho, B., Hutchins, N. & Monty, J. P. 2013 Large-scale spanwise periodicity in a turbulent boundary layer induced by highly ordered and directional surface roughness. Intl J. Heat Fluid Flow 41, 90–102.Google Scholar
Perry, A. E. & Li, J. D. 1990 Experimental support for the attached-eddy hypothesis in zero-pressure gradient turbulent boundary layers. J. Fluid Mech. 218, 405–438.Google Scholar
Perry, A. E., Schofield, W. H. & Joubert, P. N. 1969 Rough wall turbulent boundary layers. J. Fluid Mech. 37 (02), 383–413.Google Scholar
Prandtl, L. & Schlichting, H.1955 The resistance law for rough plates, translated by P. S. Granville. Tech. Rep. 258.Google Scholar
Raupach, M. R. & Shaw, R. H. 1982 Averaging procedures for flow within vegetation canopies. Boundary-Layer Meteorol. 22 (1), 79–90.Google Scholar
Schubauer, G. B. & Spangenberg, W. G. 1960 Forced mixing in boundary layers. J. Fluid Mech. 8, 10–32.Google Scholar
Shockling, M. A., Allen, J. J. & Smits, A. J. 2006 Roughness effects in turbulent pipe flow. J. Fluid Mech. 564, 267–285.Google Scholar
de Silva, C. M., Gnanamanickam, E. P., Atkinson, C., Buchmann, N. A., Hutchins, N., Soria, J. & Marusic, I. 2014 High spatial range velocity measurements in high Reynolds number turbulent boundary layer. Phys. Fluids 26 (2), 025117.Google Scholar
Soloff, S. M., Adrian, R. J. & Liu, Z. C. 1997 Distortion compensation for generalized stereoscopic particle image velocimetry. Meas. Sci. Technol. 8 (12), 1441–1454.Google Scholar
Tomkins, C. D. & Adrian, R. J. 2003 Spanwise structure and scale growth in turbulent boundary layers. J. Fluid Mech. 490, 37–74.Google Scholar
Vanderwel, C. & Ganapathisubramani, B. 2015 Effects of spanwise spacing on large-scale secondary flows in rough-wall turbulent boundary layers. J. Fluid Mech. 774, R2.Google Scholar
Vermaas, D. A., Uijttewaal, W. S. J. & Hoitink, A. J. F. 2011 Lateral transfer of streamwise momentum caused by a roughness transition across a shallow channel. Water Resour. Res. 47 (2), W02530.Google Scholar
Volino, R. J., Schultz, M. P. & Flack, K. A. 2009 Turbulence structure in a boundary layer with two-dimensional roughness. J. Fluid Mech. 635, 75–101.CrossRefGoogle Scholar
Walsh, M. J. 1990 Effect of detailed surface geometry on riblet drag reduction performance. J. Aircraft. 27 (6), 572–573.Google Scholar
Wang, Z. Q. & Cheng, N. S. 2006 Time-mean structure of secondary flows in open channel with longitudinal bedforms. Adv. Water Resour. 29 (11), 521–542.Google Scholar
Willingham, D., Anderson, W., Christensen, K. T. & Barros, J. M. 2014 Turbulent boundary layer flow over transverse aerodynamic roughness transitions: induced mixing and flow characterization. Phys. Fluids 26 (2), 025117.Google Scholar
Wu, Y. & Christensen, K. T. 2010 Spatial structure of a turbulent boundary layer with irregular surface roughness. J. Fluid Mech. 655, 380–418.CrossRefGoogle Scholar