No CrossRef data available.
Article contents
Coulomb-driven electroconvection turbulence in two-dimensional cavity
Published online by Cambridge University Press: 31 January 2024
Abstract
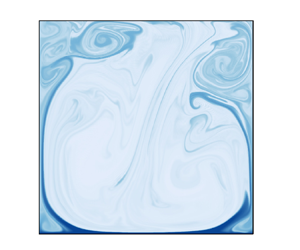
A comprehensive direct numerical simulation of electroconvection (EC) turbulence caused by strong unipolar charge injection in a two-dimensional cavity is performed. The EC turbulence has strong fluctuations and intermittency in the closed cavity. Several dominant large-scale structures are found, including two vertical main rolls and a single primary roll. The flow mode significantly influences the charge transport efficiency. A nearly  $Ne \sim T^{1/2}$ scaling stage is observed, and the optimal
$Ne \sim T^{1/2}$ scaling stage is observed, and the optimal  $Ne$ increment is related to the mode with two vertical rolls, while the single roll mode decreases the charge transport efficiency. As the flow strength increases, EC turbulence transitions from an electric force-dominated mode to an inertia-dominated mode. The former utilizes the Coulomb force more effectively and allocates more energy to convection. The vertical mean profiles of charge, electric field and energy budget provide intuitive information on the spatial energy distribution. With the aid of the energy-box technique, a detailed energy transport evolution is illustrated with changing electric Rayleigh numbers. This exploration of EC turbulence can help explain more complicated electrokinetic turbulence mechanisms and the successful utilization of Fourier mode decomposition and energy-box techniques is expected to benefit future EC studies.
$Ne$ increment is related to the mode with two vertical rolls, while the single roll mode decreases the charge transport efficiency. As the flow strength increases, EC turbulence transitions from an electric force-dominated mode to an inertia-dominated mode. The former utilizes the Coulomb force more effectively and allocates more energy to convection. The vertical mean profiles of charge, electric field and energy budget provide intuitive information on the spatial energy distribution. With the aid of the energy-box technique, a detailed energy transport evolution is illustrated with changing electric Rayleigh numbers. This exploration of EC turbulence can help explain more complicated electrokinetic turbulence mechanisms and the successful utilization of Fourier mode decomposition and energy-box techniques is expected to benefit future EC studies.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press





