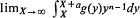Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
DALEY, D. J.
1970.
The Non-existence of Stationary Infinite Newtonian Universes and a Multi-dimensional Model of Shot Noise.
Nature,
Vol. 227,
Issue. 5261,
p.
935.
Stützer, Hans Jürgen
1975.
Sekundärgrößen unbegrenzt teilbarer Punktprozesse I.
Mathematische Nachrichten,
Vol. 70,
Issue. 1,
p.
283.
Daley, D. J.
1981.
The absolute convergence of weighted sums of dependent sequences of random variables.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 58,
Issue. 2,
p.
199.
Orsingher, Enzo
and
Battaglia, Francesco
1982.
Probability Distributions and Level Crossings of Shot Noise Models.
Stochastics,
Vol. 8,
Issue. 1,
p.
45.
Schmidt, Volker
1983.
Stationary processes with piecewise monotonous trajectories.
Mathematische Nachrichten,
Vol. 113,
Issue. 1,
p.
93.
Lane, John A.
1984.
The central limit theorem for the Poisson shot-noise process.
Journal of Applied Probability,
Vol. 21,
Issue. 02,
p.
287.
Schmidt, Volkeb
1985.
Poisson bounds for moments of shot noise processes.
Statistics,
Vol. 16,
Issue. 2,
p.
253.
Heinrich, Lothar
and
Schmidt, Volker
1985.
Normal convergence of multidimensional shot noise and rates of this convergence.
Advances in Applied Probability,
Vol. 17,
Issue. 4,
p.
709.
Schmidt, V
1985.
On finiteness and continuity of shot noise processes.
Optimization,
Vol. 16,
Issue. 6,
p.
921.
Hsing, Tailen
1987.
On the intensity of crossings by a shot noise process.
Advances in Applied Probability,
Vol. 19,
Issue. 3,
p.
743.
Schmidt, V.
1987.
Qualitative and asymptotic properties of stochastic integrals related to random marked point processes.
Optimization,
Vol. 18,
Issue. 5,
p.
737.
Bassan, B.
and
Bona, E.
1988.
Biomathematics and Related Computational Problems.
p.
423.
Hsing, Tailen
and
Teugels, J. L.
1989.
Extremal properties of shot noise processes.
Advances in Applied Probability,
Vol. 21,
Issue. 03,
p.
513.
Hawkins, D.L.
and
Hsing, Tailen
1989.
A sequential procedure for monitoring the mean of a shot noise process.
Sequential Analysis,
Vol. 8,
Issue. 3,
p.
237.
Giraitis, L.
Molchanov, S. A.
and
Surgailis, D.
1993.
New Directions in Time Series Analysis.
Vol. 46,
Issue. ,
p.
153.
van Lieshout, M. N. M.
and
Molchanov, I. S.
1998.
Shot noise weighted processes: a new family of spatial point processes.
Communications in Statistics. Stochastic Models,
Vol. 14,
Issue. 3,
p.
715.
Kella, Offer
and
Whitt, Ward
1999.
Linear stochastic fluid networks.
Journal of Applied Probability,
Vol. 36,
Issue. 01,
p.
244.
Kella, Offer
and
Whitt, Ward
1999.
Linear stochastic fluid networks.
Journal of Applied Probability,
Vol. 36,
Issue. 1,
p.
244.
Лебедев, Алексей Викторович
and
Lebedev, Alexey Viktorovich
2003.
Максимумы субэкспоненциальных полей дробового шума с конечным радиусом влияния.
Математические заметки,
Vol. 73,
Issue. 2,
p.
258.
Dousse, O.
Baccelli, F.
and
Thiran, P.
2003.
Impact of interferences on connectivity in ad hoc networks.
Vol. 3,
Issue. ,
p.
1724.
 where {tj} is a homogeneous Poisson process in Euclidean n-space En and the a.e. finite Em-valued function f(·) satisfies |f(t)| = g(t) (all |t | = t), g(t) ↓ 0 for all sufficiently large t → ∞, and with either m = 1, or m = n and f(t)/g(t) =t/t. The convergence of the sum at (*) is shown to depend on
where {tj} is a homogeneous Poisson process in Euclidean n-space En and the a.e. finite Em-valued function f(·) satisfies |f(t)| = g(t) (all |t | = t), g(t) ↓ 0 for all sufficiently large t → ∞, and with either m = 1, or m = n and f(t)/g(t) =t/t. The convergence of the sum at (*) is shown to depend on