Hostname: page-component-848d4c4894-4rdrl Total loading time: 0 Render date: 2024-06-17T15:48:02.373Z Has data issue: false hasContentIssue false
  • English
  • Français

Multiplicity of Boardman strata and deformations of map germs

Published online by Cambridge University Press:  18 May 2009

J. J. Nuño Ballesteros  and
M. J. Saia
J. J. Nuño Ballesteros
Affiliation:
Departament de Geometria I Topologia, Universitat de ValènciaCampus de Burjassot, 46100 Burjassot, Spain email: nuno@uv.es
M. J. Saia
Affiliation:
Instituto de Geociências E Ciencias Exatas, Universidade Estadual Paulista Campus de Rio Claro, Caixa Postal 178, 13500-230, Rio Claro, SP, Brazil email: mjsaia@rcb000.uesp.ansp.br
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We define algebraically for each map germ f:Kn,0→Kp, 0 and for each Boardman symbol i=(i1,…,ik) a number ci(f) which is 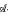-invariant. If f is finitely determined, this number is the generalization of the Milnor number of f when p = 1, the number of cusps of f when n = p = 2, or the number of cross caps when n = 2, p = 3. We study some properties of this number and prove that, in some particular cases, this number can be interpreted geometrically as the number of Σi points that appear in a generic deformation of f. In the last part, we compute this number in the case that the map germ is a projection and give some applications to catastrophe map germs.

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 40 , Issue 1 , March 1998 , pp. 21 - 32
Copyright
Copyright © Glasgow Mathematical Journal Trust 1998

References

REFERENCES

1.Boardman, J., Singularities of differentiable maps, Inst. Hautes Études Sci. Publ. Math. 33 (1967), 21–57.CrossRefGoogle Scholar
2.Fukuda, T. and Ishikawa, G., On the number ofcusps of stable perturbations of a plane-to-plane singularity, Tokyo J. Math., 10 (1987), 375–384.CrossRefGoogle Scholar
3.Fulton, W., Intersection theory (Springer-Verlag, 1984).CrossRefGoogle Scholar
4.Guffney, T. and Mond, D., Cusps and double folds of germs of analytic maps ℂ2→ℂ2, J. London Math. Soc. 2 43 (1991), 185–192.CrossRefGoogle Scholar
5.Mather, J. N., Stable map-germs and algebraic geometry, in Manifolds-Amsterdam, Lect. Notes in Math. 197 (Springer-Verlag, 1971) 176–193.Google Scholar
6.Matsumura, H., Commutative ring theory (Cambridge Studies in Advanced Math. 8, Cambridge University Press, 1986).Google Scholar
7.Mond, D., Vanishing cycles for analytic maps, in Singularity Theory and its Applications, Lecture Notes in Math. 1462 (Springer-Verlag, 1991) 221–234.CrossRefGoogle Scholar
8.Morin, B., Formes canoniques des singularités d'une application différentiate, C. R. Acad. Sci. Paris 260 (1965), 5662–5665, 6503–6506.Google Scholar
9.Morin, B., Calcul jacobien, Ann. Sci. Ecole Norm. Sup. 8 (1975), 1–98.CrossRefGoogle Scholar
10.Mumford, D., Algebraic Geometry I Complex Projective Varieties, A series of Comprehensive Studies in Mathematics 221 (Springer-Verlag, Berlin, Heidelberg, 1976).Google Scholar
11.Trotman, D. J. A. and Zeeman, E. C., The classification of elementary catastrophes of codimension ≤5, in Structural Stability, the Theory of Catastrophes and Applications in the Sciences, Seattle 1975, Lecture Notes in Math. 525 (Springer-Verlag, 1976).Google Scholar
12.Wall, C. T. C., Finite determinacy of smooth map-germs, Bull. London Math. Soc. 13 (1981), 481–539.CrossRefGoogle Scholar