Hostname: page-component-848d4c4894-wzw2p Total loading time: 0 Render date: 2024-04-30T20:52:51.743Z Has data issue: false hasContentIssue false
  • English
  • Français

THE GENERALISED LIÉNARD EQUATIONS

Published online by Cambridge University Press:  01 September 2009

A. AGHAJANI  and
A. MORADIFAM
A. AGHAJANI
Affiliation:
School of Mathematics, Iran University of Science and Technology, Tehran, Iran e-mail: aghajani@iust.ac.ir
A. MORADIFAM
Affiliation:
Department of Mathematics, University of British Columbia, Vancouver BC, Canada e-mail: a.moradi@math.ubc.ca
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper we present sufficient conditions for all trajectories of the system 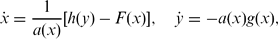to cross the vertical isocline h(y) = F(x), which is very important in the global asymptotic stability of the origin, oscillation theory and existence of periodic solutions. Also we give sufficient conditions for all trajectories which start at a point on the curve h(y) = F(x), to cross the y-axis which is closely connected with the existence of homoclinic orbits, stability of the zero solution, oscillation theory and the centre problem. The obtained results extend and improve some of the authors' previous results and some other theorems in the literature.

Keywords

34C3734D0534C05
Type
Research Article
Information
Glasgow Mathematical Journal , Volume 51 , Issue 3 , September 2009 , pp. 605 - 617
Copyright
Copyright © Glasgow Mathematical Journal Trust 2009

References

REFERENCES

1.Aghajani, A. and Moradifam, A., Some sufficient conditions for the intersection with the vertical isocline in the Liînard plane, Appl. Math. Lett. 19 (2006), 491–497.Google Scholar
2.Aghajani, A. and Moradifam, A., Intersection with the vertical isocline in the Liénard plane, Nonlin. Anal. 68 (2007), 3475–3484.CrossRefGoogle Scholar
3.Aghajani, A. and Moradifam, A., On the homoclinic orbits of the generalized Liénard equations, Appl. Math. Lett. 20 (2007), 345–351.CrossRefGoogle Scholar
4.Aghajani, A. and Moradifam, A., Oscillation of solutions of second-order nonlinear differential equations of Euler type, J. Math. Anal. Appl. 326 (2007), 1076–1089.CrossRefGoogle Scholar
5.Ding, C.-M., The homoclinic orbits in the Liînard plane, J. Math. Anal. Appl. 191 (1995), 26–39.CrossRefGoogle Scholar
6.Filippov, A. F., A sufficient condition for the existence of a stable limit cycle for an equation of the second order, Mat. Sb. (N.S.) 30 (1952), 171–180.Google Scholar
7.Graef, J. R., On the generalized Liénard equation with negative damping, J. Diff. Eq. 12 (1972), 34–62.CrossRefGoogle Scholar
8.Gyllenberg, M. and Ping, Y., The generalized Liénard systems, Discrete Cont. Dyn. Syst. 8 (2002), 1043–1057.CrossRefGoogle Scholar
9.Hara, T., Notice on the Vinograd type theorems for Liénard system, Nonlin. Anal. 22 (1994), 1437–1443.CrossRefGoogle Scholar
10.Hara, T. and Sugie, J., When all trajectories in the Liénard plane cross the vertical isocline? Nonlin. Diff Eq. Appl. 2 (4) (1995), 527–551.CrossRefGoogle Scholar
11.Hara, T. and Yoneyama, T., On the global center of generalized Liénard equation and its application to stability problems, Funkcial. Ekvac. 28 (1985), 171–192.Google Scholar
12.Hesaaraki, M. and Moradifam, A., Intersection with the vertical isocline in the generalized Liénard equations, J. Math. Anal. Appl. 334 (2007), 787–798.CrossRefGoogle Scholar
13.Hirsch, M. W. and Smale, S., Differential equations, dynamical systems, and linear algebra (Academic Press, New York, 1974).Google Scholar
14.Jiang, J. F., The global stability of a class of second order differential equations, Nonlin. Anal. 28 (1997), 855–870.Google Scholar
15.LaSale, J. P. and Lefschetz, S., Stability by Liapunov's direct method (Academic Press, New York, 1961).Google Scholar
16.Lefschetz, S., Differential equations: Geometric theory (Dover, New York, 1977).Google Scholar
17.Sansone, G. and Conti, R., NonLinear differential equations, Macmillan (New York, 1964).Google Scholar
18.Sugie, J., Homoclinic orbits in generalized Liénard systems, J. Math. Anal. Appl. 309 (2005), 211–226.Google Scholar
19.Sugie, J., Liénard dynamics with an open limit orbit, Nonlin. Diff. Eq. Appl. 8 (2001), 83–97.CrossRefGoogle Scholar
20.Sugie, J. and Hara, T., Existence and non-existence of homoclinic trajectories of the Liénard system, Discrete Cont. Dyn. Syst. 2 (1996), 237–254.CrossRefGoogle Scholar
21.Villari, G. and Zanolin, F., On a dynamical system in the Liénard plane: Necessary and sufficient conditions for the intersection with the vertical isocline and applications, Funkcial. Ekvac. 33 (1990), 19–38.Google Scholar
22.Yan, P. and Jiang, J. F., Concerning the center of the generalized Liénard systems, J. Syst. Sci. Math. Scis. 19 (1999), 353–358.Google Scholar
23.Zhang, Z. F., Ding, T. R., Huang, W. Z. and Dong, Z. X., Qualitative theory of ordinary differential equations, Translation of Mathematics Monographs vol. 101 (American Mathematical Society, Providence, RI, 1992).Google Scholar
24.Zhou, Y.-R. and Wang, X.-R., On the conditions of a center of the Liénard equation, J. Math. Anal. Appl. 180 (1993), 43–59.Google Scholar