Hostname: page-component-76fb5796d-vvkck Total loading time: 0 Render date: 2024-04-26T21:10:59.040Z Has data issue: false hasContentIssue false
  • English
  • Français

Global boundedness of a class of multilinear Fourier integral operators

Part of: Miscellaneous topics - Partial differential equations Spaces and algebras of analytic functions Harmonic analysis in several variables

Published online by Cambridge University Press:  22 February 2021

Salvador Rodríguez-López ,
David Rule  and
Wolfgang Staubach
Salvador Rodríguez-López
Affiliation:
Department of Mathematics, Stockholm University, SE-106 91Stockholm, Sweden; E-mail: s.rodriguez-lopez@math.su.se
David Rule
Affiliation:
Department of Mathematics, Linköping University, SE-581 83Linköping, Sweden; E-mail: david.rule@liu.se
Wolfgang Staubach
Affiliation:
Department of Mathematics, Stockholm University, SE-106 91Stockholm, Sweden; E-mail: s.rodriguez-lopez@math.su.se Department of Mathematics, Uppsala University, SE-751 06Uppsala, Sweden; E-mail: wulf@math.uu.se

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We establish the global regularity of multilinear Fourier integral operators that are associated to nonlinear wave equations on products of 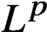$L^p$ spaces by proving endpoint boundedness on suitable product spaces containing combinations of the local Hardy space, the local BMO and the $L^2$ spaces.

MSC classification

Primary: 35S30: Fourier integral operators 42B20: Singular and oscillatory integrals (Calderón-Zygmund, etc.) 30H10: Hardy spaces
Secondary: 30H35: BMO-spaces 42B25: Maximal functions, Littlewood-Paley theory 42B35: Function spaces arising in harmonic analysis
Type
Analysis
Information
Forum of Mathematics, Sigma , Volume 9 , 2021 , e14
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s), 2021. Published by Cambridge University Press

References

Bernicot, F. and Germain, P., ‘Bilinear oscillatory integrals and boundedness for new bilinear multipliers’, Adv. Math. 225(4) (2010), 1739–1785.CrossRefGoogle Scholar
Bernicot, F. and Germain, P., ‘Bilinear dispersive estimates via space-time resonances I: The one-dimensional case’, Anal. PDE 6(3) (2013), 687–722.CrossRefGoogle Scholar
Bernicot, F. and Germain, P., ‘Bilinear dispersive estimates via space time resonances, dimensions two and three’, Arch. Ration. Mech. Anal. 214(2) (2014), 617–669.CrossRefGoogle Scholar
Carleson, L., ‘An interpolation problem for bounded analytic functions’, Amer. J. Math. 80 (1958), 921–930.CrossRefGoogle Scholar
Coifman, R. and Meyer, Y., Au delà des opérateurs pseudo-différentiels, Astérisque, 57 (Société Mathématique de France, Paris, 1978).Google Scholar
Dos Santos Ferreira, D. and Staubach, W., Global and Local Regularity for Fourier Integral Operators on Weighted and Unweighted Spaces, Mem. Amer. Math. Soc., 229(1074) (American Mathematical Society, Providence, RI, 2014).Google Scholar
Fefferman, C., ‘A note on spherical summation multipliers’, Israel J. Math. 15 (1973), 44–52.CrossRefGoogle Scholar
Germain, P., Masmoudi, N., and Shatah, J., ‘Global solutions for 3D quadratic Schrödinger equations’, Int. Math. Res. Not. IMRN 3 (2009), 414–432.Google Scholar
Germain, P., Masmoudi, N. and Shatah, J., ‘Global solutions for the gravity water waves equation in dimension 3’, C. R. Math. Acad. Sci. Paris 347(15-6) (2009), 897–902 (in English, with English and French summaries).CrossRefGoogle Scholar
Germain, P., Masmoudi, N. and Shatah, J., ‘Global solutions for 2D quadratic Schrödinger equations’, J. Math. Pures Appl. (9) 97(5) (2012), 505–543 (in English, with English and French summaries).CrossRefGoogle Scholar
Goldberg, D., ‘A local version of real Hardy spaces’, Duke Math. J. 46(1) (1979), 27–42.CrossRefGoogle Scholar
Grafakos, L., Classical Fourier Analysis, third edn, Graduate Texts in Mathematics, 249 (Springer, New York, 2014).Google Scholar
Grafakos, L. and Mastyło, M., ‘Analytic families of multilinear operators’, Nonlinear Anal. 107 (2014), 47–62.CrossRefGoogle Scholar
Hassell, A., Portal, P. and Rozendaal, J., ‘Off-singularity bounds and Hardy spaces for Fourier integral operators’, Trans. Amer. Math. Soc. 373 (2020), 5773–5832.CrossRefGoogle Scholar
Israelsson, A., Rodríguez-López, S. and Staubach, W., ‘Local and global estimates for hyperbolic equations in Besov-Lipschitz and Triebel-Lizorkin spaces’ (2019). To appear in Analysis & PDE.Google Scholar
Krantz, S. G., ‘Fractional integration on Hardy spaces’, Studia Math. 73(2) (1982), 87–94.CrossRefGoogle Scholar
Mendez, M. and Mitrea, M., ‘The Banach envelopes of Besov and Triebel-Lizorkin spaces and applications to partial differential equations’, J. Fourier Anal. Appl. 6(5) (2000), 503–531.CrossRefGoogle Scholar
Miyachi, A., ‘On some singular Fourier multipliers’, J. Fac. Sci. Univ. Tokyo Sect. IA Math. 28(2) (1981), 267–315.Google Scholar
Peetre, J., ‘On spaces of Triebel-Lizorkin type’, Ark. Mat. 13 (1975), 123–130.CrossRefGoogle Scholar
Peloso, M. M. and Secco, S. ‘Boundedness of Fourier integral operators on Hardy spaces’, Proc. Edinb. Math. Soc. (2) 51(2) (2008), 443–463.CrossRefGoogle Scholar
Rodríguez-López, S., Rule, D. and Staubach, W., ‘A Seeger-Sogge-Stein theorem for bilinear Fourier integral operators’, Adv. Math. 264 (2014), 1–54.CrossRefGoogle Scholar
Ruzhansky, M. and Sugimoto, M., ‘A local-to-global boundedness argument and Fourier integral operators’, J. Math. Anal. Appl. 473(2) (2019), 892–904.CrossRefGoogle Scholar
Seeger, A., Sogge, C. D. and Stein, E. M., ‘Regularity properties of Fourier integral operators’, Ann. of Math. (2) 134(2) (1991), 231–251.CrossRefGoogle Scholar
Stein, E. M., Harmonic Analysis: Real-Variable Methods, Orthogonality, and Oscillatory Integrals, Princeton Mathematical Series, 43 (Princeton University Press, Princeton, NJ, 1993). With the assistance of Timothy S. Murphy; Monographs in Harmonic Analysis, III.Google Scholar
Stein, E. M. and Weiss, G., Introduction to Fourier Analysis on Euclidean Spaces, Princeton Mathematical Series, 32 (Princeton University Press, Princeton, NJ, 1971).Google Scholar
Triebel, H., Theory of Function Spaces, Monographs in Mathematics, 78 (Birkhäuser Verlag, Basel, 1983).CrossRefGoogle Scholar