Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Croisille, Jean‐Pierre
and
Greff, Isabelle
2002.
Some nonconforming mixed box schemes for elliptic problems.
Numerical Methods for Partial Differential Equations,
Vol. 18,
Issue. 3,
p.
355.
Sarghini, F.
Coppola, G.
and
de Felice, G.
2002.
A new high‐order finite volume element method with spectral‐like resolution.
International Journal for Numerical Methods in Fluids,
Vol. 40,
Issue. 3-4,
p.
487.
Chatzipantelidis, P.
Makridakis, Ch.
and
Plexousakis, M.
2003.
A-posteriori error estimates for a finite volume method for the Stokes problem in two dimensions.
Applied Numerical Mathematics,
Vol. 46,
Issue. 1,
p.
45.
El Alaoui, Linda
and
Ern, Alexandre
2004.
Residual and hierarchicalaposteriorierror estimates for nonconforming mixed finite element methods.
ESAIM: Mathematical Modelling and Numerical Analysis,
Vol. 38,
Issue. 6,
p.
903.
Croisille, Jean-Pierre
and
Greff, Isabelle
2005.
An efficient box-scheme for convection–diffusion equations with sharp contrast in the diffusion coefficients.
Computers & Fluids,
Vol. 34,
Issue. 4-5,
p.
461.
El Alaoui, Linda
and
Ern, Alexandre
2006.
Nonconforming finite element methods with subgrid viscosity applied to advection‐diffusion‐reaction equations.
Numerical Methods for Partial Differential Equations,
Vol. 22,
Issue. 5,
p.
1106.
Croisille, J.-P.
2006.
A Hermitian Box-scheme for One-dimensional Elliptic Equations – Application to Problems with High Contrasts in the Ellipticity.
Computing,
Vol. 78,
Issue. 4,
p.
329.
Droniou, Jérôme
and
Eymard, Robert
2006.
A mixed finite volume scheme for anisotropic diffusion problems on any grid.
Numerische Mathematik,
Vol. 105,
Issue. 1,
p.
35.
Kim, Kwang Y.
2006.
New mixed finite volume methods for second order eliptic problems.
ESAIM: Mathematical Modelling and Numerical Analysis,
Vol. 40,
Issue. 1,
p.
123.
Ern, Alexandre
and
Guermond, Jean-Luc
2006.
Evaluation of the condition number in linear systems arising in finite element approximations.
ESAIM: Mathematical Modelling and Numerical Analysis,
Vol. 40,
Issue. 1,
p.
29.
Perot, J.B.
and
Subramanian, V.
2007.
A discrete calculus analysis of the Keller Box scheme and a generalization of the method to arbitrary meshes.
Journal of Computational Physics,
Vol. 226,
Issue. 1,
p.
494.
Tabatabaei, Amir Hossein A.E.
Shakour, Elham
and
Dehghan, Mehdi
2007.
Some implicit methods for the numerical solution of Burgers’ equation.
Applied Mathematics and Computation,
Vol. 191,
Issue. 2,
p.
560.
Mishev, Ilya D.
and
Chen, Qian‐Yong
2007.
A mixed finite volume method for elliptic problems.
Numerical Methods for Partial Differential Equations,
Vol. 23,
Issue. 5,
p.
1122.
Greff, Isabelle
2007.
Nonconforming Box‐Schemes for Elliptic Problems on Rectangular Grids.
SIAM Journal on Numerical Analysis,
Vol. 45,
Issue. 3,
p.
946.
Ju, Lili
and
Du, Qiang
2009.
A finite volume method on general surfaces and its error estimates.
Journal of Mathematical Analysis and Applications,
Vol. 352,
Issue. 2,
p.
645.
El Alaoui, Linda
2009.
An adaptive finite volume box scheme for solving a class of nonlinear parabolic equations.
Applied Mathematics Letters,
Vol. 22,
Issue. 3,
p.
291.
Dhawan, S.
Kapoor, S.
Kumar, S.
and
Rawat, S.
2012.
Contemporary review of techniques for the solution of nonlinear Burgers equation.
Journal of Computational Science,
Vol. 3,
Issue. 5,
p.
405.
Kwak, Do Y.
2012.
A New Class of Higher Order Mixed Finite Volume Methods for Elliptic Problems.
SIAM Journal on Numerical Analysis,
Vol. 50,
Issue. 4,
p.
1941.
Ranjan, R.
and
Pantano, C.
2013.
A collocated method for the incompressible Navier–Stokes equations inspired by the Box scheme.
Journal of Computational Physics,
Vol. 232,
Issue. 1,
p.
346.
Liu, Huipo
Wang, Shuanghu
and
Han, Hongbin
2016.
Error Analysis and Adaptive Methods of Least Squares Nonconforming Finite Element for the Transport Equations.
Advances in Applied Mathematics and Mechanics,
Vol. 8,
Issue. 5,
p.
871.
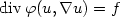 . The first scheme, which has been
introduced in [CITE], is a generalization in two dimensions
of Keller's box-scheme. The second scheme is the dual of the first
one, and is a cell-centered
scheme for u and the flux φ. For the first scheme, the two trial
finite element spaces are
the nonconforming space of Crouzeix-Raviart
for the primal unknown u and the div-conforming
space of Raviart-Thomas for the flux φ. The two test spaces are
the functions constant per cell both for the conservative and for the flux
equations.
We prove an optimal second order error estimate for
the box scheme and
we emphasize the link between this
scheme and the post-processing of Arnold
and Brezzi of the classical mixed method.
. The first scheme, which has been
introduced in [CITE], is a generalization in two dimensions
of Keller's box-scheme. The second scheme is the dual of the first
one, and is a cell-centered
scheme for u and the flux φ. For the first scheme, the two trial
finite element spaces are
the nonconforming space of Crouzeix-Raviart
for the primal unknown u and the div-conforming
space of Raviart-Thomas for the flux φ. The two test spaces are
the functions constant per cell both for the conservative and for the flux
equations.
We prove an optimal second order error estimate for
the box scheme and
we emphasize the link between this
scheme and the post-processing of Arnold
and Brezzi of the classical mixed method.




