Article contents
Local Lipschitz continuity of solutions of non-linear elliptic differential-functional equations
Published online by Cambridge University Press: 20 July 2007
Abstract
The object of this paper is to prove existence and regularity results for non-linear elliptic differential-functional equations of the form $\textrm{div}\,a(\nabla u)+F[u](x)=0,$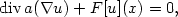 over the functions $u\in W^{1,1}(\Omega)$
over the functions $u\in W^{1,1}(\Omega)$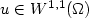 that assume given boundary values ϕ on ∂Ω. The vector field $a:{\mathbb R}^n\to {\mathbb R}^n$
that assume given boundary values ϕ on ∂Ω. The vector field $a:{\mathbb R}^n\to {\mathbb R}^n$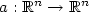 satisfies an ellipticity condition and for a fixed x, F[u](x) denotes a non-linear functional of u. In considering the same problem, Hartman and Stampacchia [Acta Math.115 (1966) 271–310] have obtained existence results in the space of uniformly Lipschitz continuous functions when ϕ satisfies the classical bounded slope condition. In a variational context, Clarke [Ann. Sc. Norm. Super. Pisa Cl. Sci.4 (2005) 511–530] has introduced a new type of hypothesis on the boundary condition ϕ: the lower (or upper) bounded slope condition. This condition, which is less restrictive than the previous one, is satisfied if ϕ is the restriction to ∂Ω of a convex function. We show that if a and F satisfy hypotheses similar to those of Hartman and Stampacchia, the lower bounded slope condition implies the existence of solutions in the space of locally Lipschitz continuous functions on Ω.
satisfies an ellipticity condition and for a fixed x, F[u](x) denotes a non-linear functional of u. In considering the same problem, Hartman and Stampacchia [Acta Math.115 (1966) 271–310] have obtained existence results in the space of uniformly Lipschitz continuous functions when ϕ satisfies the classical bounded slope condition. In a variational context, Clarke [Ann. Sc. Norm. Super. Pisa Cl. Sci.4 (2005) 511–530] has introduced a new type of hypothesis on the boundary condition ϕ: the lower (or upper) bounded slope condition. This condition, which is less restrictive than the previous one, is satisfied if ϕ is the restriction to ∂Ω of a convex function. We show that if a and F satisfy hypotheses similar to those of Hartman and Stampacchia, the lower bounded slope condition implies the existence of solutions in the space of locally Lipschitz continuous functions on Ω.
- Type
- Research Article
- Information
- ESAIM: Control, Optimisation and Calculus of Variations , Volume 13 , Issue 4 , October 2007 , pp. 707 - 716
- Copyright
- © EDP Sciences, SMAI, 2007
References
- 2
- Cited by




