An a priori Campanato type regularity condition is established for a class of W1X local minimisers $\overline{u}$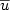 of the general variational integral $ \int_{\Omega} F(\nabla u(x))\,{\rm d}x$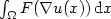 where $\Omega \subset \mathbb{R}^n$ 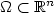is an open bounded domain, F is of class C2, F is strongly quasi-convex and satisfies the growth condition$ F(\xi)\leq c(1+|\xi|^p)$ 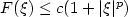for a p > 1 and where the corresponding Banach spaces X are the Morrey-Campanato space $\mathcal{L}^{p,\mu} (\Omega,\mathbb{R}^{N\times n})$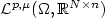, µ < n, Campanato space $\mathcal{L}^{p,n}(\Omega,\mathbb{R}^{N\times n})$ and the space of bounded mean oscillation $ {\rm BMO} \Omega,\mathbb{R}^{N\times n})$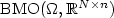. The admissible maps $u\colon \Omega \to \mathbb{R}^N$ are of Sobolev class W1,p, satisfying a Dirichlet boundary condition, and to help clarify the significance of the above result the sufficiency condition for W1BMO local minimisers is extended from Lipschitz maps to this admissible class.