Hostname: page-component-77c89778f8-9q27g Total loading time: 0 Render date: 2024-07-19T22:11:22.654Z Has data issue: false hasContentIssue false
  • English
  • Français

Hausdorff dimension of invariant measure of circle diffeomorphisms with a break point

Published online by Cambridge University Press:  07 September 2017

KONSTANTIN KHANIN  and
SAŠA KOCIĆ
KONSTANTIN KHANIN
Affiliation:
Department of Mathematics, University of Toronto, 40 St. George Street, Toronto, ON, Canada M5S 2E4 email khanin@math.utoronto.ca
SAŠA KOCIĆ
Affiliation:
Department of Mathematics, University of Mississippi, PO Box 1848, University, MS 38677-1848, USA email skocic@olemiss.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove that, for almost all irrational 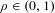$\unicode[STIX]{x1D70C}\in (0,1)$, the Hausdorff dimension of the invariant measure of a 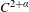$C^{2+\unicode[STIX]{x1D6FC}}$-smooth 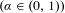$(\unicode[STIX]{x1D6FC}\in (0,1))$ circle diffeomorphism with a break of size 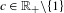$c\in \mathbb{R}_{+}\backslash \{1\}$, with rotation number $\unicode[STIX]{x1D70C}$, is zero. This result cannot be extended to all irrational rotation numbers.

Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 39 , Issue 5 , May 2019 , pp. 1331 - 1339
Copyright
© Cambridge University Press, 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Cunha, K. and Smania, D.. Rigidity for piecewise smooth homeomorphisms on the circle. Adv. Math. 250 (2014), 193–226.Google Scholar
Furstenberg, H.. Strict ergodicity and transformation of the torus. Amer. J. Math. 83(4) (1961), 573–601.Google Scholar
Herman, M. R.. Sur la conjugaison différentiable des difféomorphismes du cercle a des rotations. Publ. Math. Inst. Hautes Études Sci. 49 (1979), 5–234.Google Scholar
Khanin, K. and Kocić, S.. Renormalization conjecture and rigidity theory for circle diffeomorphisms with breaks. Geom. Funct. Anal. 24 (2014), 2002–2028.Google Scholar
Khanin, K., Kocić, S. and Mazzeo, E.. C 1 -rigidity of circle diffeomorphisms with breaks for almost all rotation numbers. Ann. Sci. Éc. Norm. Supér. (4) 50 (2017), 1163–1203.Google Scholar
Khanin, K. and Teplinsky, A.. Herman’s theory revisited. Invent. Math. 178 (2009), 333–344.Google Scholar
Khanin, K. M. and Vul, E. B.. Circle homeomorphisms with weak discontinuities. Adv. Sov. Math. 3 (1991), 57–98.Google Scholar
Kocić, S.. Generic rigidity of circle diffeomorphisms with breaks. Comm. Math. Phys. 344(2) (2016), 427–445.Google Scholar
Marmi, S., Moussa, P. and Yoccoz, J.-C.. Linearization of generalized interval exchange maps. Ann. Math. 176(3) (2012), 1583–1646.Google Scholar
Pesin, Y.. Dimension Theory in Dynamical Systems: Contemporary Views and Applications. The University of Chicago Press, Chicago, IL, 1998.Google Scholar
Sadovskaya, V.. Dimensional characteristics of invariant measures for circle diffeomorphisms. Ergod. Th. & Dynam. Sys. 29 (2009), 1979–1992.Google Scholar
Yoccoz, J.-C.. Conjugaison differentiable des difféomorphismes du cercle donc le nombre de rotation vérifie une condition Diophantienne. Ann. Sci. Éc. Norm. Supér. (4) 17 (1984), 333–361.Google Scholar
Young, L.-S.. Dimension, entropy, and Lyapunov exponents. Ergod. Th. & Dynam. Sys. 2 (1982), 109–124.Google Scholar