No CrossRef data available.
Article contents
Dynamical decompositions of  $\unicode[STIX]{x1D6FD}X\setminus X$
$\unicode[STIX]{x1D6FD}X\setminus X$
Published online by Cambridge University Press: 22 July 2015
Abstract
Let 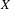 $X$ be a locally compact,
$X$ be a locally compact,  $\unicode[STIX]{x1D70E}$-compact, non-compact Hausdorff space and let
$\unicode[STIX]{x1D70E}$-compact, non-compact Hausdorff space and let 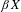 $\unicode[STIX]{x1D6FD}X$ be the Stone-Čech compactification of
$\unicode[STIX]{x1D6FD}X$ be the Stone-Čech compactification of 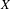 $X$. Let
$X$. Let 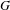 $G$ be a countably infinite discrete group continuously acting on
$G$ be a countably infinite discrete group continuously acting on 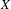 $X$, and suppose that, for every
$X$, and suppose that, for every 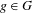 $g\in G$,
$g\in G$, 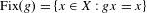 $\text{Fix}(g)=\{x\in X:gx=x\}$ is compact. The action of
$\text{Fix}(g)=\{x\in X:gx=x\}$ is compact. The action of 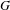 $G$ on
$G$ on 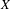 $X$ induces the action on
$X$ induces the action on 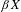 $\unicode[STIX]{x1D6FD}X$, and so on
$\unicode[STIX]{x1D6FD}X$, and so on  $X^{\ast }=\unicode[STIX]{x1D6FD}X\setminus X$. Let
$X^{\ast }=\unicode[STIX]{x1D6FD}X\setminus X$. Let  ${\mathcal{D}}$ denote the finest decomposition of
${\mathcal{D}}$ denote the finest decomposition of 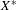 $X^{\ast }$ into closed invariant subsets such that the corresponding quotient space of
$X^{\ast }$ into closed invariant subsets such that the corresponding quotient space of 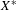 $X^{\ast }$ is Hausdorff. Such a decomposition can be defined for any action of
$X^{\ast }$ is Hausdorff. Such a decomposition can be defined for any action of 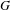 $G$ on a compact Hausdorff space. Applying it to every member of
$G$ on a compact Hausdorff space. Applying it to every member of 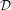 ${\mathcal{D}}$ gives us a decomposition
${\mathcal{D}}$ gives us a decomposition  ${\mathcal{D}}^{2}$ of
${\mathcal{D}}^{2}$ of 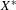 $X^{\ast }$, then
$X^{\ast }$, then 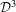 ${\mathcal{D}}^{3}$, and so on. We show that (1)
${\mathcal{D}}^{3}$, and so on. We show that (1)  ${\mathcal{D}}^{\unicode[STIX]{x1D714}_{1}}$ is the coarsest decomposition of
${\mathcal{D}}^{\unicode[STIX]{x1D714}_{1}}$ is the coarsest decomposition of  $X^{\ast }$ into closed invariant topologically transitive subsets, (2) there is a dense subset of points
$X^{\ast }$ into closed invariant topologically transitive subsets, (2) there is a dense subset of points 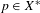 $p\in X^{\ast }$ such that
$p\in X^{\ast }$ such that 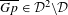 $\overline{Gp}\in {\mathcal{D}}^{2}\setminus {\mathcal{D}}$, in particular,
$\overline{Gp}\in {\mathcal{D}}^{2}\setminus {\mathcal{D}}$, in particular, 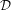 ${\mathcal{D}}$ is non-trivial and
${\mathcal{D}}$ is non-trivial and 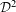 ${\mathcal{D}}^{2}$ is finer than
${\mathcal{D}}^{2}$ is finer than 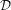 ${\mathcal{D}}$, and (3) for every ordinal
${\mathcal{D}}$, and (3) for every ordinal 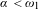 $\unicode[STIX]{x1D6FC}<\unicode[STIX]{x1D714}_{1}$, there is
$\unicode[STIX]{x1D6FC}<\unicode[STIX]{x1D714}_{1}$, there is 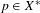 $p\in X^{\ast }$ such that
$p\in X^{\ast }$ such that 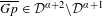 $\overline{Gp}\in {\mathcal{D}}^{\unicode[STIX]{x1D6FC}+2}\setminus {\mathcal{D}}^{\unicode[STIX]{x1D6FC}+1}$, so all the decompositions
$\overline{Gp}\in {\mathcal{D}}^{\unicode[STIX]{x1D6FC}+2}\setminus {\mathcal{D}}^{\unicode[STIX]{x1D6FC}+1}$, so all the decompositions 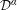 ${\mathcal{D}}^{\unicode[STIX]{x1D6FC}}$,
${\mathcal{D}}^{\unicode[STIX]{x1D6FC}}$, 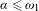 $\unicode[STIX]{x1D6FC}\leq \unicode[STIX]{x1D714}_{1}$, are distinct.
$\unicode[STIX]{x1D6FC}\leq \unicode[STIX]{x1D714}_{1}$, are distinct.
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press, 2015




