Hostname: page-component-7479d7b7d-qs9v7 Total loading time: 0 Render date: 2024-07-08T22:01:34.991Z Has data issue: false hasContentIssue false
  • English
  • Français

Ziegler's Indecomposability Criterion

Published online by Cambridge University Press:  20 November 2018

Ivo Herzog
Ivo Herzog*
Affiliation:
The Ohio State University at Lima, Lima, OH 45804, USA e-mail: herzog.23@osu.edu
Rights & Permissions [Opens in a new window]

Abstract.

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Ziegler’s Indecomposability Criterion is used to prove that a totally transcendental, i.e., $\sum $-pure injective, indecomposable left module over a left noetherian ring is a directed union of finitely generated indecomposable modules. The same criterion is also used to give a sufficient condition for a pure injective indecomposable module 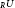$_{R}U$ to have an indecomposable local dual 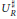$U_{R}^{\#}.$

Keywords

16G1003C60pure injective indecomposable modulelocal dualgeneric moduleamalgamation
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 56 , Issue 3 , 01 September 2013 , pp. 564 - 569
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Crawley-Boevey, W., Modules of finite length over their endomorphism rings. In: Representations of algebras and related topics (Kyoto, 1990), London Math. Soc. Lecture Note Ser., 168, Cambridge University Press, Cambridge, 1992, pp. 127–184.Google Scholar
[2] Herzog, I., Elementary duality of modules. Trans. Amer. Math. Soc. 340 (1993), no. 1, 37–69. http://dx.doi.org/10.2307/2154545 Google Scholar
[3] Herzog, I., The Ziegler spectrum of a locally coherent Grothendieck category. Proc. London Math. Soc. (3) 74 (1997), no. 3, 503–558. http://dx.doi.org/10.1112/S002461159700018X Google Scholar
[4] Hrushovski, E., A New strongly minimal set. Stability in model theory, III (Trento, 1991). Ann. Pure Appl. Logic 62 (1993), no. 2, 147–166. http://dx.doi.org/10.1016/0168-0072(9390171-9) Google Scholar
[5] Prest, M., Duality and pure-semisimple rings. J. London Math. Soc. (2) 38 (1988), no. 3, 403–409.Google Scholar
[6] Prest, M., Purity, spectra and localization. Encyclopedia of Mathematics and its Applications, 121, Cambridge University Press, Cambridge, 2009.Google Scholar
[7] Ziegler, M., Model theory of modules. Ann. Pure Appl. Logic 26 (1984), no. 2, 149–213. http://dx.doi.org/10.1016/0168-0072(84)90014-9 Google Scholar
[8] Zimmermann-Huisgen, B. and Zimmermann, W., On the sparsity of representations of rings of pure global dimension zero. Trans. Amer. Math. Soc. 320 (1990), no. 2, 695–711. http://dx.doi.org/10.2307/2001697 Google Scholar