Hostname: page-component-8448b6f56d-m8qmq Total loading time: 0 Render date: 2024-04-24T20:54:48.623Z Has data issue: false hasContentIssue false
  • English
  • Français

Some Normal Numbers Generated by Arithmetic Functions

Published online by Cambridge University Press:  20 November 2018

Paul Pollack  and
Joseph Vandehey
Paul Pollack
Affiliation:
Department of Mathematics, Boyd Graduate Studies Research Center, University of Georgia, Athens, GA 30602, USA. e-mail: pollack@uga.edu e-mail: vandehey@uga.edu
Joseph Vandehey
Affiliation:
Department of Mathematics, Boyd Graduate Studies Research Center, University of Georgia, Athens, GA 30602, USA. e-mail: pollack@uga.edu e-mail: vandehey@uga.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 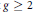$g\,\ge \,2$. A real number is said to be $g$-normal if its base $g$ expansion contains every finite sequence of digits with the expected limiting frequency. Let $\varphi$ denote Euler’s totient function, let $\sigma$ be the sum-of-divisors function, and let $\lambda$ be Carmichael’s lambda-function. We show that if $f$ is any function formed by composing $\varphi$, $\sigma$, or $\lambda$, then the number

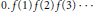$$0.f\left( 1 \right)f\left( 2 \right)f\left( 3 \right)\,.\,.\,.$$

obtained by concatenating the base $g$ digits of successive $f$-values is $g$-normal. We also prove the same result if the inputs 1,2,3.... are replaced with the primes 2, 3, 5.... The proof is an adaptation of a method introduced by Copeland and Erdõs in 1946 to prove the 10-normality of 0:235711131719...

Keywords

11K1611A6311N2511N37normal numberEuler functionsum-of-divisors functionCarmichael lambda-functionChampernowne’s number
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 58 , Issue 1 , 01 March 2015 , pp. 160 - 173
Copyright
Copyright © Canadian Mathematical Society 2015

References

[1] Bassily, N. L., Kátai, I., and Wijsmuller, M., On the prime power divisors of the iterates of the Euler-' function. Publ. Math. Debrecen 55 (1999), no. 1–2. 17–32. Google Scholar
[2] Besicovitch, A. S., The asymptotic distribution of the numerals in the decimal representation of the squares of the natural numbers. Math. Z. 39 (1935), no. 1, 146–156. http://dx.doi.org/10.1007/BF01201350 Google Scholar
[3] Borel, E., Les probabilit´es d´enombrables et leurs applications arithm´etiques. Supplemento di rend. circ. Mat. Palermo 27 (1909), 247–271. Google Scholar
[4] Champernowne, D. G., The construction of decimals normal in the scale of ten. J. London Math. Soc. 3 (1933), 254–260. http://dx.doi.org/10.1112/jlms/s1-8.4.254 Google Scholar
[5] Copeland, A. H. and P. Erdʺos, Note on normal numbers. Bull. Amer. Math. Soc. 52 (1946), 857–860. http://dx.doi.org/10.1090/S0002-9904-1946-08657-7 Google Scholar
[6] Davenport, H. andErdʺos, P., Note on normal decimals. Canad. J. Math. 4 (1952), 58–63. http://dx.doi.org/10.4153/CJM-1952-005-3 Google Scholar
[7] De Koninck, J.-M. and Kátai, I., On a problem on normal numbers raised by Igor Shparlinski. Bull. Aust. Math. Soc. 84 (2011), no. 2, 337–349. http://dx.doi.org/10.1017/S0004972711002322 Google Scholar
[8] De Koninck, J.-M. and Kátai, I., Using large prime divisors to construct normal numbers. Ann. Univ. Sci. Budapest. Sect. Comput. 39 (2013), 45–62. Google Scholar
[9] Erdʺos, P., Granville, A., Pomerance, C., and Spiro, C., On the normal behavior of the iterates of some arithmetic functions. In: Analytic number theory (Allerton Park, IL, 1989), Progr. Math., 85, Birkhäuser Boston, Boston, MA, 1990, pp. 165–204..Google Scholar
[10] Friedlander, J. B., Pomerance, C., and Shparlinski, I. E., Period of the power generator and small values of Carmichael's function. Math. Comp.70 (2001), no. 236, 1591–1605.(electronic); corrigendum 71 (2002), 1803–1806. http://dx.doi.org/10.1090/S0025-5718-00-01282-5 Google Scholar
[11] Hardy, G. H. and Wright, E. M., An introduction to the theory of numbers. Sixth ed., Oxford University Press, Oxford, 2008.Google Scholar
[12] Kurlberg, P. and Rudnick, Z., On quantum ergodicity for linear maps of the torus. Comm. Math. Phys. 222 (2001), no. 1, 201–227. http://dx.doi.org/10.1007/s002200100501 Google Scholar
[13] Luca, F. and Pollack, P., An arithmetic function arising from Carmichael's conjecture. J. Th´eor. Nombres Bordeaux 23 (2011), no. 3, 697–714. http://dx.doi.org/10.5802/jtnb.783 Google Scholar
[14] Luca, F. and Pomerance, C., Irreducible radical extensions and Euler-function chains. In: Combinatorial number theory, de Gruyter, Berlin, 2007, pp. 351–361..Google Scholar
[15] Madritsch, M. G., Thuswaldner, J. M., and Tichy, R. F., Normality of numbers generated by the values of entire functions. J. Number Theory 128 (2008), no. 5, 1127–1145. http://dx.doi.org/10.1016/j.jnt.2007.04.005 Google Scholar
[16] Nakai, Y. and Shiokawa, I., Normality of numbers generated by the values of polynomials at primes. Acta Arith. 81 (1997), no. 4, 345–356. Google Scholar
[17] Pollack, P. and Thompson, L., Practical pretenders. Publ.Math. Debrecen 82 (2013), no. 3–4. 651–667. http://dx.doi.org/10.5486/PMD.2013.5428 Google Scholar
[18] Vandehey, J., The normality of digits in almost constant additive functions. Monatsh. Math. 171 (2013), no. 3–4. 481–497. http://dx.doi.org/10.1007/s00605-012-0453-2 Google Scholar