No CrossRef data available.
Article contents
p-Radial Exceptional Sets and Conformal Mappings
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
For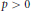 
$p\,>\,0$ and for a given set 
$E$ of type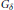 
${{G}_{\delta }}$ in the boundary of the unit disc 
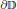$\partial \mathbb{D}$ we construct a holomorphic function 
$f\,\in \,\mathbb{O}\left( \mathbb{D} \right)$ such that
$${{\int_{\mathbb{D}\backslash \left[ 0,\,1 \right]E}{\left| f \right|}}^{p}}\,d{{\mathfrak{L}}^{2}}\,<\,\infty \,\text{and}\,E\,=\,{{E}^{p}}\left( f \right)\,=\,\{\,z\,\in \,\partial \mathbb{D}\,:\,\int _{0}^{1}\,{{\left| f\left( tz \right) \right|}^{p}}\,dt\,=\,\infty \}.$$
In particular if a set $E$ has a measure equal to zero, then a function 
$f$ is constructed as integrable with power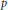 
$p$ on the unit disc 
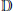$\mathbb{D}$.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2007
References
[1] Globevnik, J., Holomorphic functions which are highly nonintegrable at the boundary. Israel J. Math.
115(2000), 195–203.Google Scholar
[2] Jakóbczak, P., The exceptional sets for functions from the Bergman space. J. Port. Math.
50(1993), no. 1, 115–128.Google Scholar
[3] Jakóbczak, P., The exceptional sets for holomorphic functions in Hartogs domains. Complex Variables Theory Appl.
32(1997), no. 1, 89–97.Google Scholar
[4] Jakóbczak, P., Highly nonintegrable functions in the unit ball. Israel J. Math
97(1997), 175–181.Google Scholar
[5] Jakóbczak, P., Exceptional sets of slices for functions from the Bergman space in the ball. Canad. Math. Bull.
44(2001), no. 2, 150–159.Google Scholar
[6] Kot, P., Description of simple exceptional sets in the unit ball. Czechoslovak Math. J.
54(129)(2004), no. 1, 55–63.Google Scholar
[7] Kot, P., Maximum sets of semicontinuous functions. Potential Anal.
23(2005), no. 4, 323–356.Google Scholar
[8] Kot, P., Exceptional sets in Hartogs domains. Canad. Math. Bull
48(2005), no. 4, 580–586.Google Scholar
[9] Kot, P., Exceptional sets in convex domains. J. Convex Anal.
12(2005), no. 2, 351–364.Google Scholar
[10] Pommerenke, C., Boundary Behavior of Conformal Maps. Grundlehren der Mathematischen Wissenschaften 299, Springer-Verlag, Berlin, 1992.Google Scholar
You have
Access