Article contents
How the Roots of a Polynomial Vary with its Coefficients: A Local Quantitative Result
Published online by Cambridge University Press: 20 November 2018
Abstract
A well-known result, due to Ostrowski, states that if 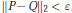 ${{\left\| P-Q \right\|}_{2}}\,<\,\varepsilon $, then the roots
${{\left\| P-Q \right\|}_{2}}\,<\,\varepsilon $, then the roots  $({{x}_{j}})$ of
$({{x}_{j}})$ of  $P$ and
$P$ and 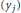 $({{y}_{j}})$ of
$({{y}_{j}})$ of 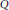 $Q$ satisfy
$Q$ satisfy 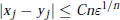 $\left| {{x}_{j}}\,-\,{{y}_{j}} \right|\,\le \,Cn{{\varepsilon }^{1/n}}$, where
$\left| {{x}_{j}}\,-\,{{y}_{j}} \right|\,\le \,Cn{{\varepsilon }^{1/n}}$, where  $n$ is the degree of
$n$ is the degree of  $P$ and
$P$ and 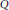 $Q$. Though there are cases where this estimate is sharp, it can still be made more precise in general, in two ways: first by using Bombieri’s norm instead of the classical
$Q$. Though there are cases where this estimate is sharp, it can still be made more precise in general, in two ways: first by using Bombieri’s norm instead of the classical 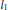 ${{l}_{1}}$ or
${{l}_{1}}$ or 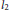 ${{l}_{2}}$ norms, and second by taking into account the multiplicity of each root. For instance, if
${{l}_{2}}$ norms, and second by taking into account the multiplicity of each root. For instance, if  $x$ is a simple root of
$x$ is a simple root of  $P$, we show that
$P$, we show that 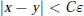 $\left| x\,-\,y \right|\,<\,C\varepsilon $ instead of
$\left| x\,-\,y \right|\,<\,C\varepsilon $ instead of  ${{\varepsilon }^{1/n}}$. The proof uses the properties of Bombieri’s scalar product andWalsh Contraction Principle.
${{\varepsilon }^{1/n}}$. The proof uses the properties of Bombieri’s scalar product andWalsh Contraction Principle.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1999
References
- 13
- Cited by




