No CrossRef data available.
Article contents
Some results on various types of compactness of weak* Dunford–Pettis operators on Banach lattices
Published online by Cambridge University Press: 23 October 2023
Abstract
We study the relationship between weak* Dunford–Pettis and weakly (resp. M-weakly, order weakly, almost M-weakly, and almost L-weakly) operators on Banach lattices. The following is one of the major results dealing with this matter: If E and F are Banach lattices such that F is Dedekind 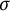 $\sigma $-complete, then each positive weak* Dunford–Pettis operator
$\sigma $-complete, then each positive weak* Dunford–Pettis operator 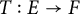 $T:E\rightarrow F$ is weakly compact if and only if one of the following assertions is valid: (a) the norms of
$T:E\rightarrow F$ is weakly compact if and only if one of the following assertions is valid: (a) the norms of 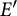 $E^{\prime }$ and F are order continuous; (b) E is reflexive; and (c) F is reflexive.
$E^{\prime }$ and F are order continuous; (b) E is reflexive; and (c) F is reflexive.
Keywords
MSC classification
- Type
- Article
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press on behalf of The Canadian Mathematical Society





