Hostname: page-component-77c89778f8-vpsfw Total loading time: 0 Render date: 2024-07-17T04:46:34.852Z Has data issue: false hasContentIssue false
  • English
  • Français

A Sufficient Condition that an Operator Algebra be Self-Adjoint

Published online by Cambridge University Press:  20 November 2018

Heydar Radjavi  and
Peter Rosenthal
Heydar Radjavi
Affiliation:
Pahlavi University, Shiraz, Iran
Peter Rosenthal
Affiliation:
University of Toronto, Toronto, Ontario
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

It is well-known, and easily verified, that each of the following assertions implies the preceding ones.

  1. (i) Every operator has a non-trivial invariant subspace.

  2. (ii) Every commutative operator algebra has a non-trivial invariant subspace,

  3. (iii) Every operator other than a multiple of the identity has a non-trivial hyperinvariant subspace.

  4. (iv) The only transitive operator algebra on 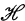is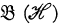

Note. Operator means bounded linear operator on a complex Hilbert space 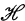, operator algebra means weakly closed algebra of operators containing the identity, subspace means closed linear manifold, a non-trivial subspace is a subspace other than {0} and 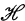, a. hyperinvariant subspace for A is a subspace invariant under every operator which commutes with A, a transitive operator algebra is one without any non-trivial invariant subspaces and 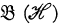denotes the algebra of all operators on .

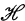Type
Research Article
Information
Canadian Journal of Mathematics , Volume 23 , Issue 4 , August 1971 , pp. 588 - 597
Copyright
Copyright © Canadian Mathematical Society 1971

References

1. Aronszajn, N. and Smith, K. T., Invariant subspaces of completely continuous operators, Ann. of Math. 60 (1954), 345–350.Google Scholar
2. Arveson, W. B., A density theorem for operator algebras, Duke Math. J. 84 (1967), 635–647.Google Scholar
3. Arveson, W. B. and Feldman, J., A note on invariant subspaces, Michigan Math. J. 15 (1968), 61–64.Google Scholar
4. Cater, F., Real and complex vector spaces (Saunders, London, 1966).Google Scholar
5. Chandler, Davis, Heydar, Radjavi and Peter, Rosenthal, On operator algebras and invariant subspaces, Can. J. Math. 21 (1969), 1178–1181.Google Scholar
6. Jacques, Dixmier, Les algèbres d'opérateurs dans Vespace Hilbertian, 2nd edition (Gauthier-Villars, Paris, 1969).Google Scholar
7. Douglas, R. G. and Carl, Pearcy, On a topology for invariant subspaces, J. Functional Analysis 2 (1968), 323–341.Google Scholar
8. Douglas, R. G. and Carl, Pearcy, E.yperinvariant subspaces and transitive algebras (to appear).Google Scholar
9. Halmos, P. R., Introduction to Hilbert Space, 2nd edition (Chelsea, New York, 1957).Google Scholar
10. Henry, Helson, Lectures on invariant subspaces (Academic Press, New York, 1964).Google Scholar
11. Hoover, T. B., Hyperinvariant subspaces for n-normal operators (to appear in Acta Sci. Math. (Szeged)).Google Scholar
12. Jacobson, N., Lectures in abstract algebra, Volume 2 (Van Nostrand, Princeton, 1953).Google Scholar
13. Kadison, R. V. and Singer, I., Triangular operator algebras, Amer. J. Math. 82 (1960), 227–259.Google Scholar
14. Kitano, K., Invariant subspaces of some non self-adjoint operators, Töhoku Math. J. 2nd series 20 (1968), 313–322.Google Scholar
15. Naimark, M. A., Normed Rings (Noordhoff, Groningen, The Netherlands, 1959).Google Scholar
16. Nordgren, Eric A., Transitive operator algebras, J. Math. Anal. Appl. 82 (1970), 639–643.Google Scholar
17. Eric, Nordgren, Heydar, Radjavi and Peter, Rosenthal, On density of transitive algebras, Acta. Sci. Math. (Szeged) 30 (1969), 175–179.Google Scholar
18. Heydar, Radjavi and Peter, Rosenthal, On invariant subspaces and reflexive algebras, Amer. J. Math. 91 (1969), 683–692.Google Scholar
19. Heydar, Radjavi and Peter, Rosenthal, Hyperinvariant subspaces for spectral and n-normal operators (to appear in Acta Sci. Math. (Szeged)).Google Scholar
20. Rickart, C. E., General theory of Banach algebras (Van Nostrand, Princeton, 1960).Google Scholar
21. Peter, Rosenthal, A note on unicellular operators, Proc. Amer. Math. Soc. 19 (1968), 505–506.Google Scholar
22. Peter, Rosenthal, Completely reducible operators, Proc. Amer. Math. Soc. 19 (1968), 826–830.Google Scholar
23. Peter, Rosenthal, Weakly closed maximal triangular algebras are hyperreducible, Proc. Amer. Math. Soc. 24 (1970), 220.Google Scholar
24. Sarason, D. E., Invariant subspaces and unstarred operator algebras, Pacific J. Math. 17 (1966), 511–517.Google Scholar
25. -Nagy, B. Sz. and Foias, C., Analyse harmonique des opérateurs de Vespace de Hilbert (Masson et. C , Academiai Kiado, Hungary, 1967).Google Scholar