Hostname: page-component-7bb8b95d7b-5mhkq Total loading time: 0 Render date: 2024-10-04T19:24:55.230Z Has data issue: false hasContentIssue false
  • English
  • Français

Splitting, Bounding, and AlmostDisjointness Can Be Quite Different

Published online by Cambridge University Press:  20 November 2018

Vera Fischer  and
Diego Alejandro Mejia
Vera Fischer
Affiliation:
Institut für Diskrete Mathematik und Geometrie, Technishe Universität Wien, Wiedner Hauptstrasse 810/104, 1040 Wien, Austria e-mail: vera.fischer@univie.ac.at, diego.mejia@shizuoka.ac.jp
Diego Alejandro Mejia
Affiliation:
Institut für Diskrete Mathematik und Geometrie, Technishe Universität Wien, Wiedner Hauptstrasse 810/104, 1040 Wien, Austria e-mail: vera.fischer@univie.ac.at, diego.mejia@shizuoka.ac.jp
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We prove the consistency of

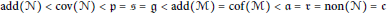$$~~\text{add}\left( \mathcal{N} \right)<\operatorname{cov}\left( \mathcal{N} \right)<\mathfrak{p}\text{=}\mathfrak{s}\text{=}\mathfrak{g}< \text{add}\left( \mathcal{M} \right)=\text{cof}\left( \mathcal{M} \right)<\mathfrak{a}=\mathfrak{r}=\text{non}\left( N \right)=\mathfrak{c}$$
with $\text{ZFC}$, where each of these cardinal invariants assume arbitrary uncountable regular values.

Keywords

03E1703E3503E40Cardinal characteristics of the continuumsplittingbounding numbermaximal almost-disjoint familiestemplate forcing iterationsisomorphism-of-names
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 69 , Issue 3 , 01 June 2017 , pp. 502 - 531
Copyright
Copyright © Canadian Mathematical Society 2017

References

[BaJ95] Bartoszynski, T. and Judah, H., Set theory: on the structure of the real line. A K Peters, Wellesley, MA, 1995.Google Scholar
[BD85] Baumgartner, J. E. and Dordal, P., Adjoining dominating functions. J. Symbolic Logic 50(1985), no. 1, 94–101.http://dx.doi.org/10.2307/2273792 Google Scholar
[Bla89] Blass, A., Applications of superperfect forcing and its relatives. In: Set theory and its applications (Toronto, ON, 1987), Lecture Notes in Math., 1401, Springer, Berlin, 1989, pp. 18–40.http://dx.doi.org/10.1007/BFb0097329 Google Scholar
[Bla10] Blass, A., Combinatorial cardinal characteristics of the continuum. In: Handbook of set theory, Vols. 1, 2, 3, Springer, Dordrecht, 2010, pp. 395–489. http://dx.doi.Org/10.1007/97-8-1-4020-5764-9_7 Google Scholar
[Bre91] Brendle, J., Larger cardinals in Cichoń's diagram. J. Symbolic Logic 56(1991), no. 3, 795–810.http://dx.doi.org/10.2307/2275049 Google Scholar
[Bre97] Brendle, J., Mob families and mad families. Arch. Math. Logic 37(1997), no. 3,183–197.http://dx.doi.Org/10.1007/s001530050091 Google Scholar
[BreO2] Brendle, J., Mad families and iteration theory. In: Logic and algebra, Contemp. Math., 302, American Mathematical Society, Providence, RI, 2002, pp. 1–31.http://dx.doi.Org/10.1090/conm/302/05083 Google Scholar
[BreO3] Brendle, J., The almost-disjointness number may have countable cofinality. Trans. Amer. Math. Soc. 355(2003), no. 7, 2633–2649. http://dx.doi.org/10.1090/S0002-9947-03-03271-9 Google Scholar
[BreO5] Brendle, J., Templates and iterations. Luminy 2002 lecture notes. Kyōto Daigaku Sūrikaiseki Kenkyūsho Kōkyūroku, 2005, pp. 1–12.Google Scholar
[BreO7] Brendle, J. , Mad families and ultrafilters. Acta Univ. Carolin. Math. Phys. 48(2007), no. 2,19–35.Google Scholar
[BreFll] Brendle, J. and Fischer, V., Mad families, splitting families and large continuum. J. Symbolic Logic 76(2011), no. 1, 198–208. http://dx.doi.Org/10.2178/jsl/1294170995 Google Scholar
[BreR14] Brendle, J. and Raghavan, D., Bounding, splitting, and almost disjointness. Ann. Pure Appl. Logic 165(2014), no. 2, 631–651.http://dx.doi.Org/10.1016/j.apal.2013.09.002 Google Scholar
[FS08] Fischer, V. and Steprāns, J., The consistency of b = K and s = K+. Fund. Math. 201(2008), no. 3, 283–293. http://dx.doi.org/10.4064/fm201-3-5 Google Scholar
[FT15] Fischer, V. and Törnquist, A., Template iterations and maximal cofinitary groups. Fund. Math. 230(2015), no. 3, 205–236.http://dx.doi.org/10.4064/fm230-3-1 Google Scholar
[Gol93] Goldstern, M., Tools for your forcing construction. In: Set theory of the reals (Ramat Gan,1991), Israel Math. Conf. Proa, 6, Bar-Ilan Univ., Ramat Gan, 1993, pp. 305–360.Google Scholar
[JeO3] Jech, T., Set theory. The third millenium ed., Springer Monographs in Mathematics, Springer-Verlag, Berlin, 2003.Google Scholar
[JS90] Judah, H. and Shelah, S., The Kunen-Miller chart (Lebesgue measure, the Baire property, Laver reals and preservation theorems for forcing). J. Symbolic Logic 55(1990), 909–927.http://dx.doi.org/10.2307/2274464 Google Scholar
[Kam89] Kamburelis, A., Iterations of Boolean algebras with measure. Arch. Math. Logic 29(1989), no. 1, 21–28.http://dx.doi.org/10.1007/BF01630808 Google Scholar
[Kunll] Kunen, K., Set theory. Studies in Logic, 34, College Publications, London, 2011.Google Scholar
[Mejl3] Mejia, D. A., Matrix iterations and Cichon's diagram. Arch. Math. Logic 52(2013), no. 3-4, 261–278.http://dx.doi.org/10.1007/sOO153-012-0315-6 Google Scholar
[Mejl5] Mejia, D. A. , Template iterations with non-definable ccc forcing notions. Ann. Pure Appl. Logic 166(2015), no. 11, 1071–1109.http://dx.doi.Org/10.1016/j.apal.2015.06.001 Google Scholar
[She84] Shelah, S., On cardinal invariants of the continuum. In: Axiomatic set theory (Boulder, Colo., 1983), Contemp. Math., 31, American Mathematical Society, Providence, RI, 1984, pp. 183–207.http://dx.doi.org/10.1090/conm/031/763901 Google Scholar
[SheO4] Shelah, S., Two cardinal invariants of the continuum (∂ < a) and FS linearly ordered iterated forcing. Acta Math. 192(2004), no. 2,187–223.http://dx.doi.org/10.1007/BF02392740 Google Scholar