Hostname: page-component-76fb5796d-vvkck Total loading time: 0 Render date: 2024-04-25T13:12:20.941Z Has data issue: false hasContentIssue false
  • English
  • Français

Slim Exceptional Sets for Sums of Cubes

Published online by Cambridge University Press:  20 November 2018

Trevor D. Wooley
Trevor D. Wooley*
Affiliation:
Department of Mathematics, University of Michigan, East Hall, 525 East University Avenue, Ann Arbor, Michigan 48109-1109, U.S.A., email: wooley@math.lsa.umich.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We investigate exceptional sets associated with various additive problems involving sums of cubes. By developing a method wherein an exponential sum over the set of exceptions is employed explicitly within the Hardy-Littlewood method, we are better able to exploit excess variables. By way of illustration, we show that the number of odd integers not divisible by 9, and not exceeding $X$, that fail to have a representation as the sum of 7 cubes of prime numbers, is 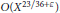$O\left( {{X}^{23/36+\varepsilon }} \right)$. For sums of eight cubes of prime numbers, the corresponding number of exceptional integers is 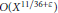$O\left( {{X}^{11/36+\varepsilon }} \right)$.

Keywords

11P3211P0511P55Waring’s problemexceptional sets
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 54 , Issue 2 , 01 April 2002 , pp. 417 - 448
Copyright
Copyright © Canadian Mathematical Society 2002

References

[1] Baker, R. C. and Harman, G., On the distribution of αpk modulo one. Mathematika 38 (1991), 170–184.Google Scholar
[2] Boklan, K. D., A reduction technique in Waring's problem, I. Acta Arith. 65 (1993), 147–161.Google Scholar
[3] Brüdern, J., OnWaring's problem for cubes.Math. Proc. Cambridge Philos. Soc. 109 (1991), 229–256.Google Scholar
[4] Brüdern, J., Kawada, K. and Wooley, T. D., Additive representation in thin sequences, I: Waring's problem for cubes. Ann. Sci. École Norm. Sup. (4), in press.Google Scholar
[5] Brüdern, J., Kawada, K. and Wooley, T. D., Additive representation in thin sequences, III: asymptotic formulae. Acta Arith., to appear.Google Scholar
[6] Brüdern, J. and Wooley, T. D., On Waring's problem for cubes and smooth Weyl sums. Proc. London Math. Soc. (3) 82 (2001), 89–109.Google Scholar
[7] Davenport, H., On Waring's problem for cubes. Acta Math. 71 (1939), 123–143.Google Scholar
[8] Friedlander, J. B., Integers free from large and small primes. Proc. London Math. Soc. (3) 33 (1976), 565–576.Google Scholar
[9] Hall, R. and Tenenbaum, G., Divisors. Cambridge University Press, Cambridge, 1988.Google Scholar
[10] Harcos, G., Waring's problem with small prime factors. Acta Arith. 80 (1997), 165–185.Google Scholar
[11] Harman, G., Trigonometric sums over primes II. Glasgow Math. J. 24 (1983), 23–37.Google Scholar
[12] Heath-Brown, D. R., The density of rational points on cubic surfaces. Acta Arith. 79 (1997), 17–30.Google Scholar
[13] Heath-Brown, D. R., The circle method and diagonal cubic forms. Philos. Trans. Roy. Soc. London Ser. A 356 (1998), 673–699.Google Scholar
[14] Hooley, C., On the representations of a number as the sum of two cubes. Math. Z. 82 (1963), 259–266.Google Scholar
[15] Hooley, C., On the representations of a number as the sum of four cubes. Proc. London Math. Soc. (3) 36 (1978), 117–140.Google Scholar
[16] Hooley, C., On Hypothesis K* in Waring's problem. In: Sieve methods, exponential sums and their applications in number theory (Cardiff, 1995), London Math. Soc. Lecture Note Ser. 237, Cambridge University Press, Cambridge, 1997, 175–185. Google Scholar
[17] Hua, L.-K., On the representation of numbers as the sums of the powers of primes. Math. Z. 44 (1938), 335–346.Google Scholar
[18] Kawada, K. and Wooley, T. D., On the Waring-Goldbach problem for fourth and fifth powers. Proc. London Math. Soc. (3) 83 (2001), 1–50.Google Scholar
[19] Parsell, S. T., The density of rational lines on cubic hypersurfaces. Trans. Amer. Math. Soc. 352 (2000), 5045–5062.Google Scholar
[20] Ren, Xiumin, The Waring-Goldbach problem for cubes. Acta Arith. 94 (2000), 287–301.Google Scholar
[21] Vaughan, R. C., On Waring's problem for cubes. J. Reine Angew. Math. 365 (1986), 122–170.Google Scholar
[22] Vaughan, R. C., The Hardy-Littlewood method. 2nd. edition, Cambridge University Press, Cambridge, 1997.Google Scholar
[23] Wooley, T. D., Breaking classical convexity in Waring's problem: sums of cubes and quasi-diagonal behaviour. Invent. Math. 122 (1995), 421–451.Google Scholar
[24] Wooley, T. D., Sums of three cubes. Mathematika, in press.Google Scholar
[25] Wooley, T. D., Slim exceptional sets for sums of four squares. Proc. London Math. Soc. (3), to appear.Google Scholar