Hostname: page-component-848d4c4894-mwx4w Total loading time: 0 Render date: 2024-06-27T04:33:27.878Z Has data issue: false hasContentIssue false
  • English
  • Français

Meridional rank and bridge number of knotted 2-spheres

Published online by Cambridge University Press:  27 December 2023

Jason Joseph  and
Puttipong Pongtanapaisan
Jason Joseph
Affiliation:
North Carolina School of Science and Mathematics, Morganton, NC 28655, United States e-mail: jason.joseph@ncssm.edu
Puttipong Pongtanapaisan*
Affiliation:
School of Mathematical and Statistical Sciences, Arizona State University, Tempe, AZ 85281, United States
*
e-mail: ppongtan@asu.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

The meridional rank conjecture asks whether the bridge number of a knot in $S^3$ is equal to the minimal number of meridians needed to generate the fundamental group of its complement. In this paper, we investigate the analogous conjecture for knotted spheres in 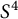$S^4$. Towards this end, we give a construction to produce classical knots with quotients sending meridians to elements of any finite order in Coxeter groups and alternating groups, which detect their meridional ranks. We establish the equality of bridge number and meridional rank for these knots and knotted spheres obtained from them by twist-spinning. On the other hand, we show that the meridional rank of knotted spheres is not additive under connected sum, so that either bridge number also collapses, or meridional rank is not equal to bridge number for knotted spheres.

Keywords

Meridional rankbridge number2-knotsknotted surfacesCoxeter groups
Type
Article
Information
Canadian Journal of Mathematics , First View , pp. 1 - 18
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author was supported by the Max Planck Institute for Mathematics and the NSF grant DMS-1664567 during part of this research and is currently supported by the NSF-RTG grant NSF DMS-1745670. Research conducted for this paper is supported by the Pacific Institute for the Mathematical Sciences (PIMS). The research and findings may not reflect those of the Institute.

References

Annin, S. and Maglione, J., Economical generating sets for the symmetric and alternating groups consisting of cycles of a fixed length . J. Algebra Appl. 11(2012), no. 06, 1250110.CrossRefGoogle Scholar
Baader, S., Blair, R., and Kjuchukova, A., Coxeter groups and meridional rank of links . Math. Ann. 379(2020), 1533–1551.CrossRefGoogle Scholar
Baader, S., Blair, R., Kjuchukova, A., and Misev, F., The bridge number of arborescent links with many twigs. Algebr. Geom. Topol. 23(2023), no. 1, 75–85.Google Scholar
Baader, S. and Kjuchukova, A., Symmetric quotients of knot groups and a filtration of the Gordian graph . Math. Proc. Cambridge Philos. Soc. 169(2020), 141–148.CrossRefGoogle Scholar
Blair, R., Kjuchukova, A., Velazquez, R., and Villanueva, P., Wirtinger systems of generators of knot groups . Comm. Anal. Geom. 28(2020), no. 2, 243–262.CrossRefGoogle Scholar
Brunner, A. M., Geometric quotients of link groups . Topology Appl. 48(1992), no. 3, 245–262.CrossRefGoogle Scholar
Burde, G. and Zieschang, H., Knots, Walter de Gruyter, Berlin, 2002.CrossRefGoogle Scholar
Freedman, M. H., The disk theorem for four-dimensional manifolds . In Proceedings of the International Congress of Mathematicians, Vol. 1, 2 (Warsaw, 1983). PWN, Warsaw, 1984, pp. 647–663.Google Scholar
Gompf, R. E. and Stipsicz, A. I., $4$ -manifolds and Kirby calculus, Graduate Studies in Mathematics, 20, American Mathematical Society, Providence, RI, 1999.CrossRefGoogle Scholar
Joseph, J. M., Klug, M. R., Ruppik, B. M., and Schwartz, H. R., Unknotting numbers of 2-spheres in the 4-sphere . J. Topol. 14(2021), no. 4, 1321–1350.CrossRefGoogle Scholar
Kadokami, T. and Yang, Z., An integral invariant from the knot group . Osaka J. Math. 47(2010), no. 4, 965–976.Google Scholar
Kanenobu, T., Weak unknotting number of a composite $2$ -knot . J. Knot Theory Ramifications. 5(1996), no. 2, 161–166.CrossRefGoogle Scholar
Litherland, R. A., Deforming twist-spun knots . Trans. Amer. Math. Soc. 250(1979), 311–331.CrossRefGoogle Scholar
Livingston, C., Knot theory, Carus Mathematical Monographs, 24, Mathematical Association of America, Washington, DC, 1993.CrossRefGoogle Scholar
Maeda, T., On a composition of knot groups ii: Algebraic bridge index . In Mathematics seminar notes. Vol. 5. 1977, pp. 457–464.Google Scholar
Rolfsen, D., Knots and links, Mathematics Lecture Series, No. 7, Publish or Perish, Inc., Berkeley, CA, 1976.Google Scholar
Scharlemann, M., Smooth spheres in R 4 with four critical points are standard . Invent. Math. 79(1985), no. 1, 125–141.CrossRefGoogle Scholar
Schubert, H., Knoten mit zwei Brücken . Math. Z. 65(1956), 133–170.CrossRefGoogle Scholar
Silver, D. S. and Whitten, W., Knot group epimorphisms . J. Knot Theory Ramifications 15(2006), no. 02, 153–166.CrossRefGoogle Scholar
Viro, O. J., Local knotting of submanifolds . Mat.-Sb. 19(1973), no. 2, 166.CrossRefGoogle Scholar
Weidmann, R., On the rank of amalgamated products and product knot groups . Math. Ann. 312(1998), no. 4, 761–771.CrossRefGoogle Scholar
Zeeman, E. C., Twisting spun knots . Trans. Amer. Math. Soc. 115(1965), 471–495.CrossRefGoogle Scholar