Hostname: page-component-5c6d5d7d68-vt8vv Total loading time: 0.001 Render date: 2024-08-20T01:01:37.386Z Has data issue: false hasContentIssue false
  • English
  • Français

The L(r, t) Summability Transform

Published online by Cambridge University Press:  20 November 2018

Robert E. Powell
Robert E. Powell*
Affiliation:
Lehigh University
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In a recent article Cheney and Sharma (1) studied the linear operator Pn defined by

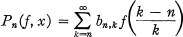
where

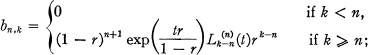
here Lj(n)(t) denotes the Laguerre polynomial of degree j. Cheney and Sharma proved that if f is continuous on [0, 1], then Pn(f, x) converges uniformly to f(x) on [0, a] where 0 < a < 1.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 18 , 1966 , pp. 1251 - 1260
Copyright
Copyright © Canadian Mathematical Society 1966

References

1. Cheney, E. W. and Sharma, A., Bernstein power series, Can. J. Math., 16 (1964), 241–252.Google Scholar
2. Cowling, V. F., Summability and analytic continuation, Proc. Amer. Math. Soc., 1 (1950), 536–542.Google Scholar
3. Cowling, V. F. and King, J. P., On the Taylor and Lototsky summability of series of Legendre polynomials, J. Analyse Math., 10 (1962-63), 139–152.Google Scholar
4. Jakimovski, Amnon, Analytic continuation and summability of Legendre polynomials, Quart. J. Math. Oxford, Ser. 2, 15 (1964), 289–302.Google Scholar
5. Laush, G., Relations among the Weierstrass methods of summability, Doctoral Dissertation, Cornell University, Ithaca, N.Y. (1949).Google Scholar
6. Lorentz, G. G., Bernstein polynomials (Toronto, 1953). pp. 117-120.Google Scholar
7. Szegö, Gabor, Orthogonal polynomials (Providence, 1959). pp. 96–97.Google Scholar
8. Whittaker, E. T. and Watson, G. N., A course of modern analysis (Cambridge, 1952). p. 321.Google Scholar