No CrossRef data available.
Article contents
Counterexample to a Conjecture on Positive Definite Functions
Published online by Cambridge University Press: 20 November 2018
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Cooper [1] called a complex-valued function f on the real line Rpositive definite for F, where F is a set of complex-valued functions on R, if the integral
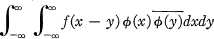
exists as a Lebesgue integral and is nonnegative for every ϕ in F. Let us denote by P(F) the set of functions positive definite for F, by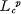 
the set of functions in LP(R) with compact support, and by 
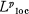the set of functions which are locally in LP(R), i.e., 
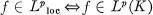for every compact subset K of R. Cooper showed that 
for any p ≧ 2 and that each function in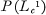 
is essentially bounded and hence equal almost everywhere to an ordinary continuous positive definite function in the sense of Bochner.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1972
References
1.
Cooper, J. L. B., Positive definite functions of a real variable, Proc. London Math. Soc. (3)
10 (1960), 53–66.Google Scholar
2.
Gelfand, I. M. and Shilov, G. E., Generalized functions, Vol. 1 (Academic Press, New York, 1964).Google Scholar
3.
Richard, O'Neil, Convolution operators and L﹛p, q) spaces, Duke Math. J.
80 (1963), 129–142.Google Scholar
4.
James, Stewart, Unbounded positive definite functions, Can. J. Math.
21 (1969), 1309–1318.Google Scholar
5.
James, Stewart, Functions with a finite number of negative squares (to appear in Can. Math. Bull. 3 (1972)).Google Scholar
You have
Access