Hostname: page-component-848d4c4894-4rdrl Total loading time: 0 Render date: 2024-07-05T08:22:54.816Z Has data issue: false hasContentIssue false
  • English
  • Français

Complex Uniform Convexity and Riesz Measures

Published online by Cambridge University Press:  20 November 2018

Gordon Blower  and
Thomas Ransford
Gordon Blower
Affiliation:
Department of Mathematics and Statistics, Lancaster University, Lancaster LA1 4YF, England, United Kingdom e-mail: g.blower@lancaster.ac.uk
Thomas Ransford
Affiliation:
Département de mathématiques et de statistique, Université Laval, Québec, Québec, G1K 7P4 e-mail: Thomas.Ransford@mat.ulaval.ca
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

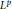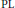The norm on a Banach space gives rise to a subharmonic function on the complex plane for which the distributional Laplacian gives a Riesz measure. This measure is calculated explicitly here for Lebesgue ${{L}^{p}}$ spaces and the von Neumann-Schatten trace ideals. Banach spaces that are $q$-uniformly $\text{PL}$-convex in the sense of Davis, Garling and Tomczak-Jaegermann are characterized in terms of the mass distribution of this measure. 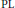This gives a new proof that the trace ideals ${{c}^{p}}$ are 2-uniformly $\text{PL}$-convex for 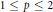$1\,\le \,p\,\le \,2$.

Keywords

46B2046L52subharmonic functionsBanach spacesSchatten trace ideals
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 56 , Issue 2 , 01 April 2004 , pp. 225 - 245
Copyright
Copyright © Canadian Mathematical Society 2004

References

[1] Ando, T., Concavity of certain maps on positive definite matrices and applications to Hadamard products. Linear Algebra Appl. 26(1979), 203–241.Google Scholar
[2] Arregui, J. L. and Blasco, O., Convolution of three functions by means of bilinear maps and applications. Illinois J. Math. 43(1999), 264–280.Google Scholar
[3] Aupetit, B., On log-subharmonicity of singular values of matrices. Studia Math. 122(1997), 195–200.Google Scholar
[4] Ball, K., Carlen, E. A., and Lieb, E. H., Sharp uniform convexity and smoothness inequalities for trace norms. Invent. Math. 115(1994), 463–482.Google Scholar
[5] Bergh, J. and Löfström, J., Interpolation spaces: an introduction. Springer, Berlin, 1976.Google Scholar
[6] Blasco, O., On the area integral for H 1 (σ p ), 1 ≤ p ≤ 2. Bull. Polish Acad. Sci. Math. 44(1996), 285–292.Google Scholar
[7] Blasco, O. and Pavlovi ć, M., Complex convexity and vector-valued Littlewood-Paley inequalities. Bull. London Math. Soc. 35(2003), 749–758.Google Scholar
[8] Bourgain, J., Vector-valued singular integrals and the H 1 – BMO duality. Probability theory and harmonic analysis., (eds., Chao, J. A. and Woyczynski, W. A.), Marcel Dekker, New York, 1986, 1–19 Google Scholar
[9] Carlen, E. A. and Lieb, E. H., Optimal hypercontractivity for Fermi fields and related non-commutative integration inequalities. Comm. Math. Phys. 155(1993), 27–46.Google Scholar
[10] Coifman, R. R. and Semmes, S., Interpolation of Banach spaces, Perron processes and Yang-Mills. Amer. J. Math. 115(1993), 243–278.Google Scholar
[11] Donoghue, W. F. Jr., Monotone Matrix Functions and Analytic Continuation. Springer, Berlin, 1974.Google Scholar
[12] Davis, W. J., Garling, D. J. H. and Tomczak-Jaegermann, N., The complex convexity of quasi-normed linear spaces. J. Funct. Anal. 55(1984), 110–150.Google Scholar
[13] Haagerup, U. and Pisier, G., Factorization of analytic functions with values in noncommutative L 1 -spaces and applications. Canad. J. Math. 41(1989), 882–906.Google Scholar
[14] Horn, R. A. and Johnson, C. R., Topics in Matrix Analysis. Cambridge University Press, Cambridge, 1991.Google Scholar
[15] Klimek, M., Pluripotential theory. London Math. Soc., Oxford, 1991.Google Scholar
[16] Lelong, P., Fonction de Green pluricomplexe et lemmes de Schwarz dans les espaces de Banach. J. Math. Pures Appl. (9) 68(1989), 319–347.Google Scholar
[17] Lieb, E. H., Convex trace functions and the Wigner-Yanase-Dyson conjecture. Adv. Math. 11(1973), 267–288.Google Scholar
[18] Mazur, S., Über schwache Konvergenz in den Raüme (Lp). Studia Math. 4(1933), 128–133.Google Scholar
[19] Pisier, G., Interpolation between Hp spaces and non-commutative generalizations 1. Pacific J. Math. 155(1992), 341–368.Google Scholar
[20] Radó, T., Subharmonic functions. Chelsea Publishing Company, New York, 1949.Google Scholar
[21] Ransford, T., Potential theory in the complex plane. London Math. Soc. Stud. Texts 28, Cambridge University Press, 1995.Google Scholar
[22] Simon, B., Trace ideals and their applications. London Math. Soc. Lecture Notes 35, Cambridge University Press, 1979.Google Scholar
[23] White, M. C., Analytic multivalued functions and symmetrically normed ideals. Ph.D.Thesis, Cambridge, 1989.Google Scholar
[24] Widder, D. V., The Laplace transform. Princeton, Princeton University Press, Princeton N.J., 1941.Google Scholar
[25] Xu, Q.-H., Convexité uniforme et inégalités de martingales. Math. Ann. 287(1990), 193–211.Google Scholar
[26] Xu, Q.-H., Littlewood-Paley theory for functions with values in uniformly convex spaces. J. Reine Angew. Math. 504(1998), 195–226.Google Scholar