Hostname: page-component-848d4c4894-8bljj Total loading time: 0 Render date: 2024-06-27T11:14:32.977Z Has data issue: false hasContentIssue false
  • English
  • Français

Structure of the kernels associated with invariant subspaces of the Bergman shift

Published online by Cambridge University Press:  17 April 2009

George Chailos
George Chailos
Affiliation:
University of Tennessee, Knoxville TN 37920, United States of America, e-mail: chailos@math.utk.eduIntercollege, Makedonitissas Ave, 1700 Nicosia, Cyprus, e-mail: chailos.g@intercollege.ac.cy
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this article we consider index 1 invariant subspaces M of the operator of multiplication by ζ(z) = z, Mζ, on the Bergman space of the unit disc . It turns out that there is a positive sesquianalytic kernel lλ defined on  ×  which determines M uniquely. Here we study the boundary behaviour and some of the basic properties of the kernel lλ. Among other things, we show that if the lower zero set of M, 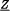(M), is nonempty, the kernel lλ for fixed λ ∈  has a meromorphic continuation across 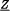\(M), where  is the unit circle. Furthermore we consider some special type of kernels lλ and by studying their structure we obtain information for the invariant subspaces related to them. Lastly, and after introducing the general for the invariant subspaces related to them. Lastly, and after introducing the general vector valued setting, we discuss some analogous results for the case of , where m is a positive integer.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 67 , Issue 3 , June 2003 , pp. 445 - 457
Copyright
Copyright © Australian Mathematical Society 2003

References

[1]Aleman, A. and Richter, S., ‘Some sufficient conditions for the division property of invariant subspaces of weighted Bergman Spaces’, J. Funct. Anal. 144 (1997), 542–555.CrossRefGoogle Scholar
[2]Aleman, A., Richter, S. and Ross, W., ‘Pseudocontinuations and the backward shift’, Indiana Univ. Math. J. 47 (1998), 223–276.CrossRefGoogle Scholar
[3]Aleman, A., Richter, S. and Sundberg, C., ‘Beurling' Theorem for the Bergman Space’, Acta Math. 177 (1996), 275–310.CrossRefGoogle Scholar
[4]Aronszajn, N., ‘Theory of reproducing kernels’, Trans. Amer. Math. Soc. 68 (1950), 337–404.CrossRefGoogle Scholar
[5]Chailos, G., On reproducing kernels and invariant subspaces of the Bergman shift, (Ph.D. dissertation) (University of Tennessee, Knoxville, 2002).Google Scholar
[6]Hedenmalm, H., ‘Spectral properties of invariant subspaces in the Bergman space’, J. Funct. Anal. 116 (1993), 441–448.CrossRefGoogle Scholar
[7]Hedenmalm, H., Korenblum, B. and Zhu, K., Theory of Bergman Spaces, Graduate Texts in Mathematics 199 (Springer-Verlag, New York, 2000).CrossRefGoogle Scholar
[8]Horowitz, C., ‘Factorization theorems for functions in the Bergman Spaces’, Duke Math. J. 44 (1977), 201–213.CrossRefGoogle Scholar
[9]McCullough, S. and Richter, S., ‘Bergman-type reproducing kernels, contractive divisors and dilations’, J. Funct. Anal. (to appear).Google Scholar
[10]McDonald, G. and Sundberg, C., ‘Toeplitz operators on the disc’, Indiana University Math. J. 28 (1979), 595–641.CrossRefGoogle Scholar
[11]Richter, S., ‘Unitary equivalence of invariant subspaces of Bergmen spaces and Dirichlet spaces’, Pacific J. Math. 133 (1988), 151–156.CrossRefGoogle Scholar