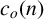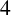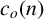1 Introduction
A partition
![]() $\lambda$
of a nonnegative integer n is a finite weakly decreasing sequence of positive integers
$\lambda$
of a nonnegative integer n is a finite weakly decreasing sequence of positive integers
![]() $\lambda _1\geq \lambda _2\geq \cdots \geq \lambda _r$
such that
$\lambda _1\geq \lambda _2\geq \cdots \geq \lambda _r$
such that
![]() $\sum _{i=1}^r\lambda _i=n$
. The
$\sum _{i=1}^r\lambda _i=n$
. The
![]() $\lambda _i$
for
$\lambda _i$
for
![]() $1\leq i\leq r$
are called the parts of the partition
$1\leq i\leq r$
are called the parts of the partition
![]() $\lambda $
. Let
$\lambda $
. Let
![]() $p(n)$
denote the number of partitions of n. In 1919, Ramanujan [Reference Ramanujan8] discovered three remarkable congruences enjoyed by
$p(n)$
denote the number of partitions of n. In 1919, Ramanujan [Reference Ramanujan8] discovered three remarkable congruences enjoyed by
![]() $p(n)$
, namely,
$p(n)$
, namely,
In 1944, Dyson [Reference Dyson7] introduced the notion of the rank, and further conjectured that this partition statistic could provide a combinatorial interpretation for (1.1) and (1.2). Dyson’s conjecture was later confirmed by Atkin and Swinnerton-Dyer [Reference Atkin and Swinnerton-Dyer4] in 1954. Unfortunately, this partition statistic cannot interpret (1.3) combinatorially. Therefore, Dyson further conjectured that there exists another statistic, which he named the ‘crank’, providing a combinatorial interpretation of (1.3). This partition statistic was discovered by Andrews and Garvan [Reference Andrews and Garvan3] in 1988. For a partition
![]() $\lambda $
, let
$\lambda $
, let
![]() $l(\lambda )$
denote the largest part of
$l(\lambda )$
denote the largest part of
![]() $\lambda $
, let
$\lambda $
, let
![]() $\omega (\lambda )$
and
$\omega (\lambda )$
and
![]() $\mu (\lambda )$
denote the number of ones in
$\mu (\lambda )$
denote the number of ones in
![]() $\lambda $
and the number of parts of
$\lambda $
and the number of parts of
![]() $\lambda $
that are larger than
$\lambda $
that are larger than
![]() $\omega (\lambda )$
, respectively. The crank is defined by
$\omega (\lambda )$
, respectively. The crank is defined by
 $$ \begin{align*} \textrm{crank}(\lambda)= \begin{cases} l(\lambda) \quad &\textrm{if }\omega(\lambda)=0,\\ \omega(\lambda)-\mu(\lambda) \quad &\textrm{if }\omega(\lambda)>0. \end{cases} \end{align*} $$
$$ \begin{align*} \textrm{crank}(\lambda)= \begin{cases} l(\lambda) \quad &\textrm{if }\omega(\lambda)=0,\\ \omega(\lambda)-\mu(\lambda) \quad &\textrm{if }\omega(\lambda)>0. \end{cases} \end{align*} $$
Let
![]() $c_o(n)$
denote the number of partitions of n with odd crank. The generating function of
$c_o(n)$
denote the number of partitions of n with odd crank. The generating function of
![]() $c_o(n)$
is given by
$c_o(n)$
is given by
 $$ \begin{align*} \sum_{n=0}^\infty c_o(n)q^n=\dfrac{1}{2} {\bigg(\dfrac{1}{(q;q)_\infty}-\dfrac{(q;q)_\infty^3}{(q^2;q^2)_\infty^2}\bigg)}. \end{align*} $$
$$ \begin{align*} \sum_{n=0}^\infty c_o(n)q^n=\dfrac{1}{2} {\bigg(\dfrac{1}{(q;q)_\infty}-\dfrac{(q;q)_\infty^3}{(q^2;q^2)_\infty^2}\bigg)}. \end{align*} $$
Throughout the rest of this paper, we always assume that q is a complex number such that
![]() $|q|<1$
and adopt the following customary notation:
$|q|<1$
and adopt the following customary notation:
 $$ \begin{align*} (a;q)_\infty &:=\prod_{k=0}^\infty(1-aq^k),\\ (a_1,a_2,\ldots,a_m;q)_\infty &:=(a_1;q)_\infty(a_2;q)_\infty\cdots(a_m;q)_\infty. \end{align*} $$
$$ \begin{align*} (a;q)_\infty &:=\prod_{k=0}^\infty(1-aq^k),\\ (a_1,a_2,\ldots,a_m;q)_\infty &:=(a_1;q)_\infty(a_2;q)_\infty\cdots(a_m;q)_\infty. \end{align*} $$
Recently, Banerjee and Dastidar [Reference Banerjee and Dastidar5] considered some arithmetic properties of
![]() $c_o(n)$
. By means of q-series manipulations, Banerjee and Dastidar [Reference Banerjee and Dastidar5, (1.10)] proved that for any
$c_o(n)$
. By means of q-series manipulations, Banerjee and Dastidar [Reference Banerjee and Dastidar5, (1.10)] proved that for any
![]() $n\geq 0$
,
$n\geq 0$
,
Based on computer experiments, they conjectured a congruence modulo
![]() $4$
satisfied by
$4$
satisfied by
![]() $c_o(n)$
.
$c_o(n)$
.
Conjecture 1.1. We have
![]() $c_o(2n)\equiv 0 \pmod {4}$
for any
$c_o(2n)\equiv 0 \pmod {4}$
for any
![]() $n\geq 0$
.
$n\geq 0$
.
Banerjee and Dastidar [Reference Banerjee and Dastidar5] verified that Conjecture 1.1 holds for any
![]() $1\leq n\leq 2000$
. By using some q-series techniques, we not only confirm the above congruence modulo
$1\leq n\leq 2000$
. By using some q-series techniques, we not only confirm the above congruence modulo
![]() $4$
, but also establish another congruence modulo
$4$
, but also establish another congruence modulo
![]() $8$
.
$8$
.
Theorem 1.2. For any
![]() $n\geq 0$
,
$n\geq 0$
,
2 Proof of Theorem 1.2
To prove (1.4) and (1.5), we need the following three auxiliary identities.
Lemma 2.1 [Reference Andrews, Berndt, Chan, Kim and Malik2, Lemma 4.1]
We have
 $$ \begin{align} \dfrac{1}{(q;q)_\infty}=\dfrac{1}{(q^2;q^2)_\infty^2} ((-q^6,-q^{10},q^{16};q^{16})_\infty+q(-q^2,-q^{14},q^{16};q^{16})_\infty). \end{align} $$
$$ \begin{align} \dfrac{1}{(q;q)_\infty}=\dfrac{1}{(q^2;q^2)_\infty^2} ((-q^6,-q^{10},q^{16};q^{16})_\infty+q(-q^2,-q^{14},q^{16};q^{16})_\infty). \end{align} $$
Lemma 2.2 (Jacobi’s identity [Reference Berndt6, Theorem 1.3.9])
 $$ \begin{align} (q;q)_\infty^3=\sum_{n=0}^\infty(-1)^n(2n+1)q^{n(n+1)/2}. \end{align} $$
$$ \begin{align} (q;q)_\infty^3=\sum_{n=0}^\infty(-1)^n(2n+1)q^{n(n+1)/2}. \end{align} $$
Lemma 2.3 (Jacobi’s triple product identity [Reference Andrews and Berndt1, Lemma 1.2.2])
 $$ \begin{align} \sum_{n=-\infty}^\infty a^{n(n+1)/2}b^{n(n-1)/2}=(-a,-b,ab;ab)_\infty,\quad|ab|<1. \end{align} $$
$$ \begin{align} \sum_{n=-\infty}^\infty a^{n(n+1)/2}b^{n(n-1)/2}=(-a,-b,ab;ab)_\infty,\quad|ab|<1. \end{align} $$
Now we are in a position to prove Theorem 1.2.
Proof of Theorem 1.2
Define the sequence
![]() $\{A(n)\}_{n\geq 0}$
by
$\{A(n)\}_{n\geq 0}$
by
 $$ \begin{align} \sum_{n=0}^\infty A(n)q^n=\dfrac{1}{(q;q)_\infty}-\dfrac{(q;q)_\infty^3}{(q^2;q^2)_\infty^2}. \end{align} $$
$$ \begin{align} \sum_{n=0}^\infty A(n)q^n=\dfrac{1}{(q;q)_\infty}-\dfrac{(q;q)_\infty^3}{(q^2;q^2)_\infty^2}. \end{align} $$
Therefore, (1.4) and (1.5) are equivalent respectively to
and
However, from (2.2),
 $$ \begin{align*} (q;q)_\infty^3 &=\sum_{n=0}^\infty(-1)^n(2n+1)q^{n(n+1)/2}\\ &=\sum_{n=0}^\infty(-1)^{8n}(16n+1)q^{4n(8n+1)}+\sum_{n=0}^\infty(-1)^{8n+1}(16n+3)q^{(4n+1)(8n+1)}\\ &\quad+\sum_{n=0}^\infty(-1)^{8n+2}(16n+5)q^{(4n+1)(8n+3)}+\sum_{n=0}^\infty(-1)^{8n+3}(16n+7)q^{(4n+2)(8n+3)}\\ &\quad+\sum_{n=0}^\infty(-1)^{8n+4}(16n+9)q^{(4n+2)(8n+5)}+\sum_{n=0}^\infty(-1)^{8n+5}(16n+11)q^{(4n+3)(8n+5)}\\ &\quad+\sum_{n=0}^\infty(-1)^{8n+6}(16n+13)q^{(4n+3)(8n+7)}+\sum_{n=0}^\infty(-1)^{8n+7}(16n+15)q^{(4n+4)(8n+7)},\end{align*} $$
$$ \begin{align*} (q;q)_\infty^3 &=\sum_{n=0}^\infty(-1)^n(2n+1)q^{n(n+1)/2}\\ &=\sum_{n=0}^\infty(-1)^{8n}(16n+1)q^{4n(8n+1)}+\sum_{n=0}^\infty(-1)^{8n+1}(16n+3)q^{(4n+1)(8n+1)}\\ &\quad+\sum_{n=0}^\infty(-1)^{8n+2}(16n+5)q^{(4n+1)(8n+3)}+\sum_{n=0}^\infty(-1)^{8n+3}(16n+7)q^{(4n+2)(8n+3)}\\ &\quad+\sum_{n=0}^\infty(-1)^{8n+4}(16n+9)q^{(4n+2)(8n+5)}+\sum_{n=0}^\infty(-1)^{8n+5}(16n+11)q^{(4n+3)(8n+5)}\\ &\quad+\sum_{n=0}^\infty(-1)^{8n+6}(16n+13)q^{(4n+3)(8n+7)}+\sum_{n=0}^\infty(-1)^{8n+7}(16n+15)q^{(4n+4)(8n+7)},\end{align*} $$
from which we further obtain that
 $$ \begin{align} (q;q)_\infty^3 &\equiv\sum_{n=0}^\infty q^{4n(8n+1)}-3\sum_{n=0}^\infty q^{(4n+1)(8n+1)}\notag\\ &\quad+5\sum_{n=0}^\infty q^{(4n+1)(8n+3)}-7\sum_{n=0}^\infty q^{(4n+2)(8n+3)} \nonumber \\&\quad-7\sum_{n=0}^\infty q^{(4n+2)(8n+5)}+5\sum_{n=0}^\infty q^{(4n+3)(8n+5)}\notag\\ &\quad-3\sum_{n=0}^\infty q^{(4n+3)(8n+7)}+\sum_{n=0}^\infty q^{(4n+4)(8n+7)} \pmod{16}. \end{align} $$
$$ \begin{align} (q;q)_\infty^3 &\equiv\sum_{n=0}^\infty q^{4n(8n+1)}-3\sum_{n=0}^\infty q^{(4n+1)(8n+1)}\notag\\ &\quad+5\sum_{n=0}^\infty q^{(4n+1)(8n+3)}-7\sum_{n=0}^\infty q^{(4n+2)(8n+3)} \nonumber \\&\quad-7\sum_{n=0}^\infty q^{(4n+2)(8n+5)}+5\sum_{n=0}^\infty q^{(4n+3)(8n+5)}\notag\\ &\quad-3\sum_{n=0}^\infty q^{(4n+3)(8n+7)}+\sum_{n=0}^\infty q^{(4n+4)(8n+7)} \pmod{16}. \end{align} $$
Replacing n by
![]() $-n-1$
in the last four infinite sums in (2.7),
$-n-1$
in the last four infinite sums in (2.7),
 $$ \begin{align} \sum_{n=0}^\infty q^{(4n+2)(8n+5)} &=\sum_{n=-\infty}^{-1}q^{(4n+2)(8n+3)}, \end{align} $$
$$ \begin{align} \sum_{n=0}^\infty q^{(4n+2)(8n+5)} &=\sum_{n=-\infty}^{-1}q^{(4n+2)(8n+3)}, \end{align} $$
 $$ \begin{align} \sum_{n=0}^\infty q^{(4n+3)(8n+5)} &=\sum_{n=-\infty}^{-1}q^{(4n+1)(8n+3)}, \end{align} $$
$$ \begin{align} \sum_{n=0}^\infty q^{(4n+3)(8n+5)} &=\sum_{n=-\infty}^{-1}q^{(4n+1)(8n+3)}, \end{align} $$
 $$ \begin{align} \sum_{n=0}^\infty q^{(4n+3)(8n+7)} &=\sum_{n=-\infty}^{-1}q^{(4n+1)(8n+1)}, \end{align} $$
$$ \begin{align} \sum_{n=0}^\infty q^{(4n+3)(8n+7)} &=\sum_{n=-\infty}^{-1}q^{(4n+1)(8n+1)}, \end{align} $$
 $$ \begin{align} \kern-15pt\sum_{n=0}^\infty q^{(4n+4)(8n+7)} &=\sum_{n=-\infty}^{-1}q^{4n(8n+1)}. \end{align} $$
$$ \begin{align} \kern-15pt\sum_{n=0}^\infty q^{(4n+4)(8n+7)} &=\sum_{n=-\infty}^{-1}q^{4n(8n+1)}. \end{align} $$
Substituting (2.8)–(2.11) into (2.9),
 $$ \begin{align*} (q;q)_\infty^3 &\equiv\sum_{n=-\infty}^\infty q^{4n(8n+1)}-3\sum_{n=-\infty}^\infty q^{(4n+1)(8n+1)}\notag\\ &\quad+5\sum_{n=-\infty}^\infty q^{(4n+1)(8n+3)}-7\sum_{n=-\infty}^\infty q^{(4n+2)(8n+3)} \pmod{16}. \end{align*} $$
$$ \begin{align*} (q;q)_\infty^3 &\equiv\sum_{n=-\infty}^\infty q^{4n(8n+1)}-3\sum_{n=-\infty}^\infty q^{(4n+1)(8n+1)}\notag\\ &\quad+5\sum_{n=-\infty}^\infty q^{(4n+1)(8n+3)}-7\sum_{n=-\infty}^\infty q^{(4n+2)(8n+3)} \pmod{16}. \end{align*} $$
Thanks to (2.3),
 $$ \begin{align} (q;q)_\infty^3 &\equiv(-q^{28},-q^{36},q^{64};q^{64})_\infty-3q(-q^{20},-q^{44},q^{64};q^{64})_\infty\notag\\[4pt] &\quad+5q^3(-q^{12},-q^{52},q^{64};q^{64})_\infty-7q^6(-q^4,-q^{60},q^{64};q^{64})_\infty \pmod{16}. \end{align} $$
$$ \begin{align} (q;q)_\infty^3 &\equiv(-q^{28},-q^{36},q^{64};q^{64})_\infty-3q(-q^{20},-q^{44},q^{64};q^{64})_\infty\notag\\[4pt] &\quad+5q^3(-q^{12},-q^{52},q^{64};q^{64})_\infty-7q^6(-q^4,-q^{60},q^{64};q^{64})_\infty \pmod{16}. \end{align} $$
Substituting (2.1) and (2.12) into (2.4) yields
 $$ \begin{align} \sum_{n=0}^\infty A(n)q^n &\equiv\dfrac{1}{(q^2;q^2)_\infty^2} ((-q^6,-q^{10},q^{16};q^{16})_\infty+q(-q^2,-q^{14},q^{16};q^{16})_\infty)\notag\\ &\quad-\dfrac{1}{(q^2;q^2)_\infty^2}((-q^{28},-q^{36},q^{64};q^{64})_\infty-3q(-q^{20},-q^{44},q^{64};q^{64})_\infty\notag\\[4pt] &\qquad+5q^3(-q^{12},-q^{52},q^{64};q^{64})_\infty-7q^6(-q^4,-q^{60},q^{64};q^{64})_\infty) \pmod{16}.\end{align} $$
$$ \begin{align} \sum_{n=0}^\infty A(n)q^n &\equiv\dfrac{1}{(q^2;q^2)_\infty^2} ((-q^6,-q^{10},q^{16};q^{16})_\infty+q(-q^2,-q^{14},q^{16};q^{16})_\infty)\notag\\ &\quad-\dfrac{1}{(q^2;q^2)_\infty^2}((-q^{28},-q^{36},q^{64};q^{64})_\infty-3q(-q^{20},-q^{44},q^{64};q^{64})_\infty\notag\\[4pt] &\qquad+5q^3(-q^{12},-q^{52},q^{64};q^{64})_\infty-7q^6(-q^4,-q^{60},q^{64};q^{64})_\infty) \pmod{16}.\end{align} $$
Taking all terms of the form
![]() $q^{2n}$
in (2.13), after simplification,
$q^{2n}$
in (2.13), after simplification,
 $$ \begin{align} \sum_{n=0}^\infty A(2n)q^n &\equiv\dfrac{1}{(q;q)_\infty^2} ((-q^3,-q^5,q^8;q^8)_\infty-(-q^{14},-q^{18},q^{32};q^{32})_\infty\notag\\ &\quad+7q^3(-q^2,-q^{30},q^{32};q^{32})_\infty) \pmod{16}. \end{align} $$
$$ \begin{align} \sum_{n=0}^\infty A(2n)q^n &\equiv\dfrac{1}{(q;q)_\infty^2} ((-q^3,-q^5,q^8;q^8)_\infty-(-q^{14},-q^{18},q^{32};q^{32})_\infty\notag\\ &\quad+7q^3(-q^2,-q^{30},q^{32};q^{32})_\infty) \pmod{16}. \end{align} $$
According to (2.3),
 $$ \begin{align} (-q^3,-q^5,q^8;q^8)_\infty &=\sum_{n=-\infty}^\infty q^{4n^2+n}\notag\\ &=\sum_{n=-\infty}^\infty q^{4(2n)^2+2n}+\sum_{n=-\infty}^\infty q^{4(2n-1)^2+(2n-1)}\notag\\ &=\sum_{n=-\infty}^\infty q^{16n^2+2n}+\sum_{n=-\infty}^\infty q^{16n^2-14n+3}\notag\\ &=(-q^{14},-q^{18},q^{32};q^{32})_\infty+q^3(-q^2,-q^{30},q^{32};q^{32})_\infty, \end{align} $$
$$ \begin{align} (-q^3,-q^5,q^8;q^8)_\infty &=\sum_{n=-\infty}^\infty q^{4n^2+n}\notag\\ &=\sum_{n=-\infty}^\infty q^{4(2n)^2+2n}+\sum_{n=-\infty}^\infty q^{4(2n-1)^2+(2n-1)}\notag\\ &=\sum_{n=-\infty}^\infty q^{16n^2+2n}+\sum_{n=-\infty}^\infty q^{16n^2-14n+3}\notag\\ &=(-q^{14},-q^{18},q^{32};q^{32})_\infty+q^3(-q^2,-q^{30},q^{32};q^{32})_\infty, \end{align} $$
where we have used (2.3) in the last step. Combining (2.14) and (2.15) gives
 $$ \begin{align} \sum_{n=0}^\infty A(2n)q^n\equiv 8q^3\dfrac{(-q^2,-q^{30},q^{32};q^{32})_\infty}{(q;q)_\infty^2} \pmod{16}. \end{align} $$
$$ \begin{align} \sum_{n=0}^\infty A(2n)q^n\equiv 8q^3\dfrac{(-q^2,-q^{30},q^{32};q^{32})_\infty}{(q;q)_\infty^2} \pmod{16}. \end{align} $$
The congruence (2.5) follows immediately from (2.16).
Moreover, from the congruence
![]() $(q;q)_\infty ^2\equiv (q^2;q^2)_\infty \pmod {2}$
,
$(q;q)_\infty ^2\equiv (q^2;q^2)_\infty \pmod {2}$
,
 $$ \begin{align} \sum_{n=0}^\infty A(2n)q^n\equiv8q^3\dfrac{(-q^2,-q^{30},q^{32};q^{32})_\infty}{(q^2;q^2)_\infty} \pmod{16}. \end{align} $$
$$ \begin{align} \sum_{n=0}^\infty A(2n)q^n\equiv8q^3\dfrac{(-q^2,-q^{30},q^{32};q^{32})_\infty}{(q^2;q^2)_\infty} \pmod{16}. \end{align} $$
The congruence (2.6) follows immediately from (2.17).
This completes the proof of Theorem 1.2.
3 Concluding remarks
We conclude this paper with two remarks.
First, the numerical evidence suggests the following conjecture.
Conjecture 3.1. We have
 $$ \begin{align*} \lim_{n\rightarrow\infty}\dfrac{\#\{m|\:c_o(2m+1)\equiv0 \pmod{4},\;\;1\leq m\leq n\}}{n} &=\dfrac{1}{2},\\ \lim_{n\rightarrow\infty}\dfrac{\#\{m|\:c_o(2m)\equiv0 \pmod{8},\;\;1\leq m\leq n\}}{n} &=\dfrac{1}{4},\\ \lim_{n\rightarrow\infty}\dfrac{\#\{m|\:c_o(4m+2)\equiv0 \pmod{8},\;\;1\leq m\leq n\}}{n} &=\dfrac{1}{2},\\ \lim_{n\rightarrow\infty}\dfrac{\#\{m|\:c_o(4m)\equiv0 \pmod{16},\;\;1\leq m\leq n\}}{n} &=\dfrac{1}{2}. \end{align*} $$
$$ \begin{align*} \lim_{n\rightarrow\infty}\dfrac{\#\{m|\:c_o(2m+1)\equiv0 \pmod{4},\;\;1\leq m\leq n\}}{n} &=\dfrac{1}{2},\\ \lim_{n\rightarrow\infty}\dfrac{\#\{m|\:c_o(2m)\equiv0 \pmod{8},\;\;1\leq m\leq n\}}{n} &=\dfrac{1}{4},\\ \lim_{n\rightarrow\infty}\dfrac{\#\{m|\:c_o(4m+2)\equiv0 \pmod{8},\;\;1\leq m\leq n\}}{n} &=\dfrac{1}{2},\\ \lim_{n\rightarrow\infty}\dfrac{\#\{m|\:c_o(4m)\equiv0 \pmod{16},\;\;1\leq m\leq n\}}{n} &=\dfrac{1}{2}. \end{align*} $$
Second, it would be interesting find a combinatorial proof of (1.4) and (1.5).
Acknowledgement
The author would like to acknowledge the anonymous referee for the careful reading and helpful suggestions that have improved the quality of the paper to a great extent.