Let $T$ be a finite simple group of Lie type in characteristic 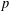$p$, and let $S$ be a Sylow subgroup of 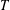$T$ with maximal order. It is well known that 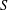$S$ is a Sylow 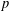$p$-subgroup except for an explicit list of exceptions and that 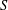$S$ is always ‘large’ in the sense that $|T|^{1/3}<|S|\leq |T|^{1/2}$. One might anticipate that, moreover, the Sylow $r$-subgroups of 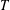$T$ with $r\neq p$ are usually significantly smaller than $S$. We verify this hypothesis by proving that, for every 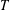$T$ and every prime divisor $r$ of 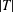$|T|$ with $r\neq p$, the order of the Sylow $r$-subgroup of $T$ is at most $|T|^{2\lfloor \log _{r}(4(\ell +1)r)\rfloor /\ell }=|T|^{O(\log _{r}(\ell )/\ell )}$, where 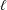$\ell$ is the Lie rank of 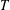$T$.