No CrossRef data available.
Article contents
Maximum average distance in complex finite dimensional normed spaces
Published online by Cambridge University Press: 17 April 2009
Abstract
A number r > 0 is called a rendezvous number for a metric space (M, d) if for any n ∈ ℕ and any x1,…xn ∈ M, there exists x ∈ M such that 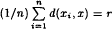 . A rendezvous number for a normed space X is a rendezvous number for its unit sphere. A surprising theorem due to O. Gross states that every finite dimensional normed space has one and only one average number, denoted by r (X). In a recent paper, A. Hinrichs solves a conjecture raised by R. Wolf. He proves that
. A rendezvous number for a normed space X is a rendezvous number for its unit sphere. A surprising theorem due to O. Gross states that every finite dimensional normed space has one and only one average number, denoted by r (X). In a recent paper, A. Hinrichs solves a conjecture raised by R. Wolf. He proves that 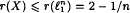 for any n-dimensional real normed space. In this paper, we prove the analogous inequality in the complex case for n ≥ 3.
for any n-dimensional real normed space. In this paper, we prove the analogous inequality in the complex case for n ≥ 3.
- Type
- Research Article
- Information
- Copyright
- Copyright © Australian Mathematical Society 2002




