2. Mathematical model

Consider a droplet of fluid in an axisymmetric well in the otherwise dry planar surface of a substrate undergoing quasi-static diffusion-limited evaporation into a quiescent atmosphere. We refer the description to polar coordinates $r$, $\phi$, $z$ with $Oz$ along the axis of the well, perpendicular to the surface of the substrate at $z = 0$, as sketched in figure 1. We denote the maximum depth of the well, which occurs at $r = 0$, by $H_0$, and the radius of its lip by $R_0$, so that the lip is located at $r = R_0$, $z = 0$. We take the droplet to be axisymmetric, and denote its free-surface profile by $z = h(r,t)$, where $t$ denotes time. The droplet is deposited into the well at $t=0$, and thereafter its volume decreases due to evaporation until it has completely evaporated, which occurs at $t=t_{lifetime}$, where $t_{lifetime}$ is the lifetime of the droplet.

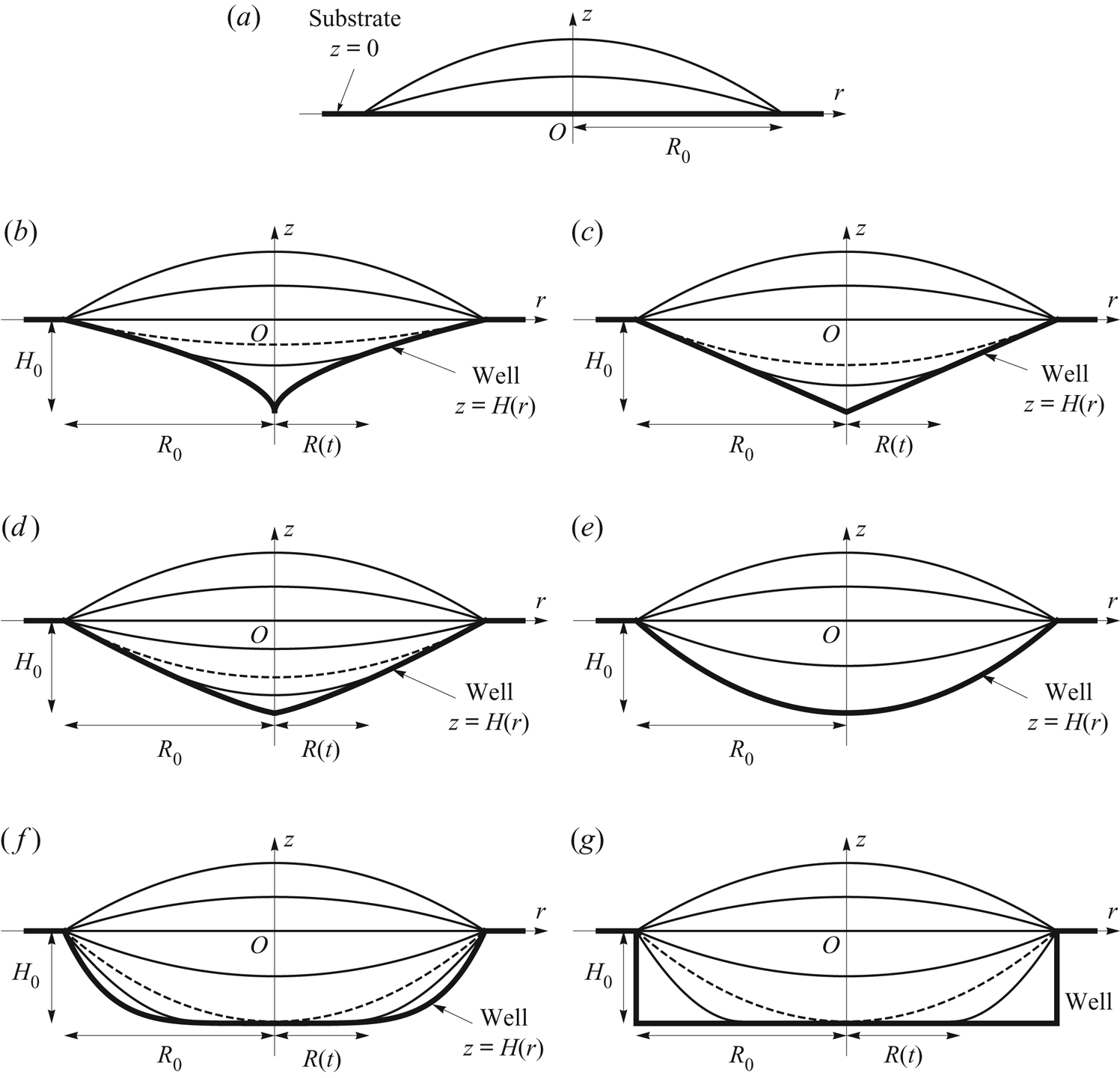
Figure 1. Sketch of snapshots of the free-surface profile $z=h(r,t)$ of a thin droplet evaporating in a shallow axisymmetric well with profile $z = H(r) = - H_0(1 - (r/R_0)^{n})$ in the otherwise dry planar surface $z = 0$ of a substrate, for (a) either $H_0 = 0$ or $n = 0$ (i.e. a planar substrate with no well), (b) $0 < n < 1$, (c) $n = 1$ (i.e. a conical well), (d) $1 < n < 2$, (e) $n = 2$ (i.e. a paraboloidal well), (f) $2 < n < \infty$ and (g) in the limit $n \to \infty$ (i.e. a cylindrical well). In (b–g) the free-surface profile at $t=t_{touchdown}$ is indicated with a dashed curve. Note that the dashed curve is not visible in (e) as touchdown occurs everywhere simultaneously within the well in the special case $n=2$.

We consider situations in which the droplet is thin and the well is shallow, the droplet is sufficiently small that the effect of gravity is negligible, and the surface tension is sufficiently strong that the free surface of the droplet evolves quasi-statically. More specifically, we consider situations in which the aspect ratio of the droplet and the well, $\epsilon = H_0/R_0 \ll 1$, is small, as are the appropriately defined Bond number ${Bo}$ and capillary number ${Ca}$, namely

(2.1a,b)\begin{equation} {Bo} = \frac{\rho g R_0^{2}}{\gamma} \ll 1 \quad \text{and} \quad {Ca} = \frac{\mu U}{\epsilon^{3}\gamma} \ll 1, \end{equation}

where $\rho$, $\gamma$ and $\mu$ are the constant density, surface tension and dynamic viscosity of the fluid, respectively, $g$ denotes the magnitude of acceleration due to gravity and $U$ is an appropriate radial velocity scale (defined in § 7).

The mean curvature of the free surface of the droplet is spatially constant, and so at leading order in the limit $\epsilon \to 0$ the free-surface profile $h$ satisfies

(2.2)\begin{equation} \frac{\partial}{\partial r}\left(\frac{1}{r}\frac{\partial}{\partial r} \left(r\frac{\partial h}{\partial r}\right)\right) = 0, \end{equation}

and hence takes the general form

(2.3)\begin{equation} h = c_1 r^{2} + c_2 \log r + c_3, \end{equation}

where the $c_i = c_i(t)$ for $i = 1$, $2$, $3$ are yet to be determined.

We consider a shaped well with profile $z = H(r)$ ($\le 0$), where

(2.4)\begin{equation} H ={-} H_0\left(1 - \left(\frac{r}{R_0}\right)^{n}\right) \quad \text{for} \ 0 \le r \le R_0, \end{equation}

in which the exponent $n$ ($\ge 0$) is a constant. The volume of the well (i.e. its volume below the plane $z = 0$) is given by

(2.5)\begin{equation} V_{well} = \frac{{\rm \pi} n H_0 R_0^{2}}{2 + n}, \end{equation}

and any three of the four quantities $V_{well}$, $R_0$, $H_0$ and $n$ may be prescribed. Cases with either $H_0 = 0$ or $n = 0$ in (2.4), sketched in figure 1(a), correspond to the familiar case of a droplet on a planar substrate with no well. The cases $n = 1$, $n = 2$ and in the limit $n \to \infty$, also included in figure 1, correspond respectively to a conical well, a paraboloidal well (which will turn out to be an important special case), and a cylindrical well with vertical side $r = R_0$ and flat bottom $z = -H_0$. The latter case, which, as we have already seen, is of particular interest from a practical point of view, is the subject of the experimental investigation reported in the present work. At its lowest point located at $r = 0$, $z = -H_0$ the profile of the well (2.4) has a cusp when $0 < n < 1$, has a corner when $n = 1$, and is flat when $n > 1$; also its curvature there is infinite when $0 < n < 2$, takes the value $4H_0/R_0^{2}$ when $n = 2$, and is zero when $n > 2$. The slope of the well at its lip is $nH_0/R_0$.

We assume that, at least in the first stage of the evolution, the contact line is pinned at the lip of the well located at $r = R_0$, $z = 0$. The initial volume of the droplet, $V_0$, could be greater than, equal to, or less than the volume of the well, $V_{well}$, in which case the initial free surface of the droplet would be respectively above, at, or below the plane $z = 0$. Although all of these cases could be analysed by the present approach, for definiteness we take $V_0$ to be greater than $V_{well}$, so that initially the well is completely filled and the free surface is above $z = 0$.

At some time $t = t_{touchdown}$ ($0 < t_{touchdown} \le t_{lifetime}$) the free surface makes contact tangentially (i.e. at zero contact angle) with the surface of the well. As shown by the dashed curves in figure 1, when $0 < n < 2$ touchdown occurs at the lip of the well, and when $n > 2$ it occurs at the centre of the well. In the special case n = 2 touchdown occurs everywhere simultaneously within the well, and so the dashed curve is not visible in figure 1(e). Before touchdown the behaviour of the droplet is the same in all three cases, which may therefore be analysed together, but after touchdown the behaviour is different, and it is then convenient to consider the three cases separately.

In the special case $n = 2$ the droplet has completely evaporated at $t = t_{touchdown}$, and so $t_{lifetime} = t_{touchdown}$. However, in the general case $n \ne 2$ the droplet has not completely evaporated at $t = t_{touchdown}$, and the nature of its subsequent evolution depends on whether $0 < n < 2$ or $n > 2$. When $0 < n < 2$ we assume that, as sketched in figure 1(b–d), the contact line de-pins from the lip of the well, and thereafter the contact line recedes (i.e. moves inwards towards the centre of the well) with decreasing radius $R = R(t)$ until $R(t_{lifetime})=0$, at which time the droplet has completely evaporated. On the other hand, when $n > 2$ we assume that, as sketched in figure 1(f,g), a new inner contact line appears at the centre of the well (i.e. at the centre of the droplet, which then becomes annular), and thereafter the inner contact line recedes (i.e. moves outwards towards the lip of the well, where the outer contact line remains pinned) with increasing radius $R = R(t)$ until $R(t_{lifetime})=R_0$, at which time the droplet has completely evaporated.

In the next two sections we analyse the evolution of the droplet before and after touchdown, respectively.

3. Evolution before touchdown, i.e. for $0 \le t \le t_{touchdown}$

Before touchdown, i.e. for $0 \le t \le t_{touchdown}$, the contact line is pinned at the lip of the well, and so the free-surface profile given by (2.3) must satisfy $h(R_0,t) = 0$; in addition, $h$ must be finite at $r = 0$, and is therefore of the familiar paraboloidal form

(3.1a,b)\begin{equation} h = h_{m} \left(1 - \frac{r^{2}}{R_0^{2}}\right), \quad h_{m} = \frac{\theta R_0}{2}, \end{equation}

where $h_{m} = h_{m}(t) = h(0,t)$ is the height of the free surface at the centre of the well, and $\theta = \theta (t) \ll 1$ is the (small) angle that the free surface at the lip of the well makes with the plane $z = 0$, i.e. $\theta = -\partial h/\partial r$ at $r = R_0$. Note that, unlike for a droplet on a planar substrate for which both $h_{m}$ and $\theta$ must be non-negative, for a droplet in a well they may be positive, zero or negative. The volume $V = V(t)$ of the droplet is related to $V_{well}$, $R_0$, $H_0$, $n$ and $\theta$ by

(3.2)\begin{equation} V = V_{well} + \frac{{\rm \pi} \theta R_0^{3}}{4} = \frac{{\rm \pi} n H_0 R_0^{2}}{2 + n} + \frac{{\rm \pi} \theta R_0^{3}}{4}. \end{equation}

According to the well-known quasi-static diffusion-limited model of the evaporation of a droplet (see, for example, Picknett & Bexon Reference Picknett and Bexon1977; Deegan et al. Reference Deegan, Bakajin, Dupont, Huber, Nagel and Witten1997; Hu & Larson Reference Hu and Larson2002; Popov Reference Popov2005; Dunn et al. Reference Dunn, Wilson, Duffy, David and Sefiane2009; Wray, Duffy & Wilson Reference Wray, Duffy and Wilson2020), the (static) concentration $c=c(r,z)$ of vapour in the atmosphere satisfies

(3.3)\begin{equation} \nabla^{2} c = 0 \quad \text{in} \ z > 0, \end{equation}

with

(3.4)\begin{gather} c \to c_{\infty} \quad \text{as} \ r^{2} + z^{2} \to \infty, \end{gather}

(3.5)\begin{gather} c = c_{sat} \quad \text{on} \ z = 0 \quad \text{for} \ 0 \le r \le R_0, \end{gather}

(3.6)\begin{gather} \frac{\partial c}{\partial z} = 0 \quad \text{on} \ z = 0 \quad \text{for} \ r > R_0, \end{gather}

where $c_{sat}$ is the constant saturation concentration and $c_{\infty } = RHc_{sat}$ is the constant ambient concentration, where $RH$ $(0 \le RH \le 1)$ is the relative humidity of the vapour in the atmosphere. The local evaporative flux $J=J(r)$ from the free surface of the droplet is given by

(3.7)\begin{equation} J ={-} D\frac{\partial c}{\partial z} \quad \text{on} \ z = 0 \quad \text{for} \ 0 \le r \le R_0, \end{equation}

where $D$ is the constant diffusion coefficient of vapour in the atmosphere, and the volume $V=V(t)$ evolves according to the global mass-conservation condition

(3.8)\begin{equation} \rho \frac{\textrm{d} V}{\textrm{d} t} ={-} 2{\rm \pi}\int_0^{R_0} J \, r \, \textrm{d} r. \end{equation}

Note that, in general, (3.5) and (3.7) apply on the free surface $z = h$, but for a thin droplet such as that considered in the present work they can be transferred to the plane $z = 0$ by Taylor expanding about $z = 0$. We denote the initial values of $\theta$, $h_{m}$ and $V$ by $\theta _0$, $h_{{m}0}$ and $V_0$, respectively, so that

(3.9)\begin{equation} \theta = \theta_0, \quad h_{m} = h_{{m}0} = \frac{\theta_0 R_0}{2}, \quad V = V_0 = \frac{{\rm \pi} n H_0 R_0^{2}}{2 + n} + \frac{{\rm \pi} \theta_0 R_0^{3}}{4} \quad \text{at} \ t = 0. \end{equation}

Note that for $0 \le t \le t_{touchdown}$ the concentration $c$ and hence the local flux $J$ are independent of $t$, whereas for $t_{touchdown} < t \le t_{lifetime}$ both of them depend on $t$ via their dependence on $R=R(t)$.

The natural time scale for the evaporation of a thin droplet is $\rho \theta _0 R_0^{2}/[D(c_{sat}-c_{\infty })]$ (see, for example, Dunn et al. Reference Dunn, Wilson, Duffy, David and Sefiane2008; Schofield et al. Reference Schofield, Wilson, Pritchard and Sefiane2018), and so we non-dimensionalise and scale the variables according to

(3.10)\begin{align} \left. \begin{gathered} r = R_0 r^{*}, \quad z = \theta_0 R_0 z^{*}, \quad h = \theta_0 R_0 h^{*}, \quad h_{m} = \theta_0 R_0 h_{m}^{*}, \quad t = \frac{\rho\theta_0 R_0^{2}}{D(c_{sat}-c_{\infty})}t^{*},\\ \theta = \theta_0 \theta^{*}, \quad R = R_0 R^{*}, \quad H = \theta_0 R_0 H^{*}, \quad H_0 = \theta_0 R_0 H_0^{*}, \quad V = \theta_0 R_0^{3} V^{*}, \\ c = c_{\infty}+(c_{sat}-c_{\infty})c^{*},\quad J = \frac{D(c_{sat}-c_{\infty})}{R_0}J^{*}\end{gathered} \right\} \end{align}

for the droplet, and similarly for the atmosphere except that $z = R_0 \hat {z}$. With the stars and the hat immediately dropped for clarity, (3.1a,b) and (3.2) give

(3.11a–c)\begin{equation} h = h_{m} \left(1 - r^{2}\right), \quad h_{m} = \frac{\theta}{2}, \quad V = \frac{{\rm \pi} n H_0}{2 + n} + \frac{{\rm \pi} \theta}{4}, \end{equation}

Laplace's equation (3.3) is unchanged, and (3.4)–(3.9) become

(3.12)\begin{gather} c \to 0 \quad \text{as} \ r^{2} + z^{2} \to \infty, \end{gather}

(3.13)\begin{gather} c = 1 \quad \text{on} \ z = 0 \quad \text{for} \ 0 \le r \le 1, \end{gather}

(3.14)\begin{gather} \frac{\partial c}{\partial z} = 0 \quad \text{on} \ z = 0 \quad \text{for} \ r > 1, \end{gather}

(3.15)\begin{gather} J ={-} \frac{\partial c}{\partial z} \quad \text{on} \ z = 0 \quad \text{for} \ 0 \le r \le 1, \end{gather}

(3.16)\begin{gather} \frac{\textrm{d} V}{\textrm{d} t} ={-} 2{\rm \pi}\int_0^{1} J \, r \, \textrm{d} r, \end{gather}

(3.17)\begin{gather} \theta = 1, \quad h_{m} = \frac{1}{2}, \quad V = \frac{{\rm \pi} n H_0}{2 + n} + \frac{\rm \pi}{4} \quad \text{at} \ t = 0, \end{gather}

respectively. The solution for the concentration $c$ may be written in the form (see, for example, Fabrikant Reference Fabrikant1995)

(3.18)\begin{equation} c = \frac{2}{\rm \pi}\sin^{{-}1} \frac{2}{ [(1 + r)^{2} + z^{2}]^{1/2} + [(1 - r)^{2} + z^{2}]^{1/2} }, \end{equation}

which, using (3.15), leads to the solution for the local flux $J$, namely

(3.19)\begin{equation} J = \frac{2}{{\rm \pi}(1 - r^{2})^{1/2}}, \end{equation}

which exhibits the familiar (integrable) square-root singularity in J at the contact line $r = 1$ even when the free surface is below the plane $z = 0$.

Substituting the expression for $V$ given by (3.11c) and the expression for $J$ given by (3.19) into (3.16) yields

(3.20)\begin{equation} \frac{\textrm{d} V}{\textrm{d} t} = \frac{\rm \pi}{4}\frac{\textrm{d} \theta}{\textrm{d} t} ={-}4, \end{equation}

and so the evolution of the droplet before touchdown is given by

(3.21a–d)\begin{equation} h = h_{m}(1 - r^{2}), \quad \theta = 1 - \frac{16t}{\rm \pi}, \quad h_{m} = \frac{1}{2} - \frac{8t}{\rm \pi}, \quad V = \frac{{\rm \pi} n H_0}{2 + n} + \frac{\rm \pi}{4} - 4t. \end{equation}

The free surface is instantaneously flat (i.e. $\theta = 0$, $h_{m} = 0$ and $V = V_{well}$) at some time $t = t_{flat}$ given by

(3.22)\begin{equation} t_{flat} = \frac{\rm \pi}{16} \simeq 0.1963. \end{equation}

As well as being of some interest in its own right, the occurrence of a flat free surface is relatively easy to observe experimentally.

When $0 < n < 2$ touchdown occurs when $\theta = -H'(1) = -n H_0$, where a dash denotes differentiation with respect to argument, showing that

(3.23a–c)\begin{equation} t = t_{touchdown} = \frac{{\rm \pi}(1 + n H_0)}{16}, \quad h_{m} ={-}\frac{n H_0}{2}, \quad V = \frac{{\rm \pi} n(2 - n) H_0}{4(2 + n)} \end{equation}

at touchdown. As we have already seen, in the special case $n = 2$ touchdown occurs everywhere simultaneously within the well, and so $t_{lifetime} = t_{touchdown} = {\rm \pi}(1 + 2 H_0)/16$. When $n > 2$ touchdown occurs when $h_{m} = -H_0$, showing that

(3.24a–c)\begin{equation} t = t_{touchdown} = \frac{{\rm \pi}(1 + 2H_0)}{16}, \quad \theta ={-}2H_0, \quad V = \frac{{\rm \pi} (n - 2) H_0}{2(n + 2)} \end{equation}

at touchdown. Setting either $H_0 = 0$ or $n=0$ in (3.23a) or $H_0=0$ in (3.24a) gives $t_{lifetime} = t_{touchdown} = t_{flat} = {\rm \pi}/16$, recovering the familiar expression for the lifetime of a pinned droplet on a planar substrate (see, for example, Stauber et al. Reference Stauber, Wilson, Duffy and Sefiane2014, Reference Stauber, Wilson, Duffy and Sefiane2015).

4. Evolution after touchdown, i.e. for $t_{touchdown} < t \le t_{lifetime}$

4.1. The case $0 < n < 2$

When $0 < n < 2$ the free surface touches down at the lip of the well located at $r = 1$, $z = 0$ at $t = t_{touchdown}$, and after touchdown, i.e. for $t_{touchdown} < t \le t_{lifetime}$, the non-annular droplet has a receding circular contact line of radius $R=R(t)$ which satisfies $R(t_{touchdown}) = 1$ and $R(t_{lifetime}) = 0$. We must specify a condition in addition to $h = H$ at the moving contact line. Since the (paraboloidal) free surface (3.21a) for $0 \le t \le t_{touchdown}$ touches down with zero contact angle at $r = 1$, we make the natural modelling assumption that the contact angle at the receding contact line remains at the value zero throughout the subsequent evolution. Thus we have the boundary conditions

(4.1)\begin{equation} h = H ={-} H_0(1 - R^{n}), \quad \frac{\partial h}{\partial r} = H' = n H_0 R^{n-1} \quad \text{at} \ r = R, \end{equation}

where again a dash denotes differentiation with respect to argument. The solution (2.3) for $h$ satisfying (4.1) with $h$ finite at $r = 0$ takes the form

(4.2)\begin{equation} h = H(R) - \frac{n H_0 R^{n-2}}{2} \left(R^{2} - r^{2}\right), \end{equation}

or, equivalently,

(4.3)\begin{equation} h = h_{m} + \frac{nH_0R^{n-2}r^{2}}{2}, \quad \text{where} \ h_{m} ={-} H_0 + \frac{(2-n)H_0R^{n}}{2}, \end{equation}

for $0 \le r \le R$, and $V$ is given by

(4.4)\begin{equation} V = \frac{{\rm \pi} n(2 - n) H_0 R^{2 + n}}{4(2 + n)}. \end{equation}

The (now quasi-static) concentration $c=c(r,z,t)$ of vapour in the atmosphere still satisfies Laplace's equation (3.3) and the boundary condition (3.12), but (3.13)–(3.17) must be replaced with

(4.5)\begin{gather} c = 1 \quad \text{on} \ z = 0 \quad \text{for} \ 0 \le r \le R, \end{gather}

(4.6)\begin{gather} \frac{\partial c}{\partial z} = 0 \quad \text{on} \ z = 0 \quad \text{for} \ r > R, \end{gather}

(4.7)\begin{gather} J ={-} \frac{\partial c}{\partial z} \quad \text{on} \ z = 0 \quad \text{for} \ 0 \le r \le R, \end{gather}

(4.8)\begin{gather} \frac{\textrm{d} V}{\textrm{d} t} ={-}2{\rm \pi}\int_0^{R} J \, r \, \textrm{d} r, \end{gather}

(4.9)\begin{gather} R = 1, \quad V = \frac{{\rm \pi} n(2 - n) H_0}{4(2 + n)} \quad \text{at} \ t = t_{touchdown}, \end{gather}

respectively, where $J=J(r,t)$ is again the local evaporative flux. In addition, we have

(4.10)\begin{equation} R = 0, \quad V = 0 \quad \text{at} \ t = t_{lifetime}. \end{equation}

As in § 3, for a thin droplet (4.5) and (4.7) are applied on $z=0$ rather than on $z=h$, and similarly for a shallow well (4.6) for $R < r \le 1$ is also applied on $z=0$ rather than on $z=H$. The solution for the concentration $c$ of the problem defined by (3.3), (3.12), (4.5) and (4.6) is analogous to (3.18) and is given by

(4.11)\begin{equation} c = \frac{2}{\rm \pi}\sin^{{-}1} \frac{2R}{ [(R + r)^{2} + z^{2}]^{1/2} + [(R - r)^{2} + z^{2}]^{1/2} }, \end{equation}

which, using (4.7), leads to the solution for the local flux $J$ analogous to (3.19), namely

(4.12)\begin{equation} J = \frac{2}{{\rm \pi}(R^{2} - r^{2})^{1/2}}. \end{equation}

Substituting (4.4) and (4.12) into (4.8) yields

(4.13)\begin{equation} R^{n} \frac{\textrm{d} R}{\textrm{d} t} ={-}\frac{16}{{\rm \pi} n(2 - n)H_0}, \end{equation}

leading to an explicit solution for $R$ after touchdown, namely

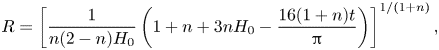(4.14)\begin{equation} R = \left[\frac{1}{n(2 - n)H_0}\left(1 + n + 3n H_0 - \frac{16(1 + n)t}{\rm \pi}\right)\right]^{1/(1 + n)}, \end{equation}

with $h$ given by (4.2) and $V$ given by (4.4), i.e. 

(4.15)\begin{equation} V = \frac{\rm \pi}{4(2 + n)\left[n(2 - n)H_0\right]^{1/(1 + n)}} \left(1 + n + 3n H_0 - \frac{16(1 + n)t}{\rm \pi}\right)^{(2 + n)/(1 + n)}. \end{equation}

Figure 2 shows the evolution of the free-surface profile $h$ for $n = 1$ and $n = 2$ with $H_0 = 1$. Figure 3 shows plots of $R$ and $V$ as functions of $t$ for a range of values of $n$, again with $H_0 = 1$. Note that $\textrm {d} R/\textrm {d} t \to \infty$ and (although not very easy to see in figure 3b) $\textrm {d} V/\textrm {d} t \to 0^{+}$ in the limit $t \to t_{lifetime}^{-}$.

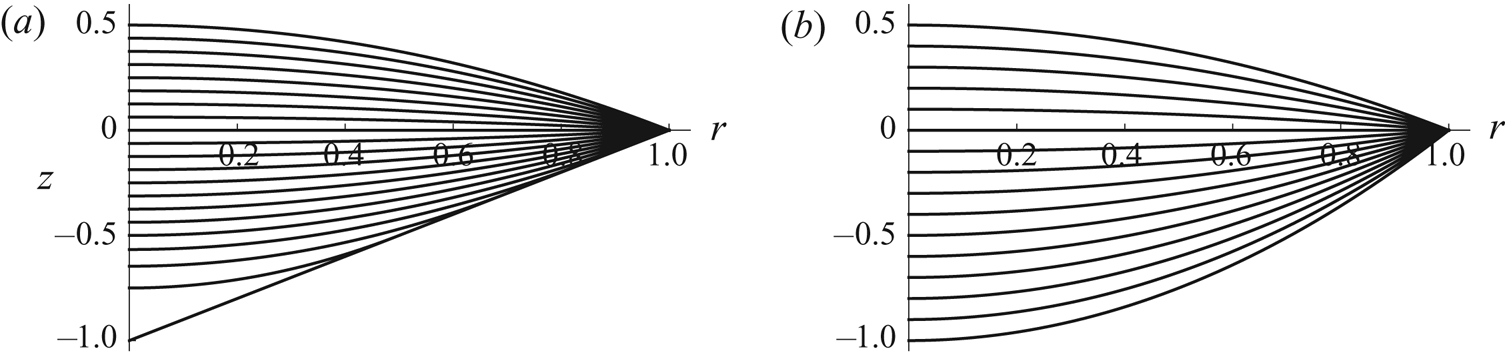
Figure 2. Evolution of the free-surface profile $h$ given by (3.21a) for $0 \le t \le t_{touchdown}$ and by (4.2) for $t_{touchdown} < t \le t_{lifetime}$ for (a) a conical well with $n = 1$ and $H_0 = 1$, and (b) a paraboloidal well with $n = 2$ and $H_0 = 1$. In (a) the curves are drawn at intervals of $t_{touchdown}/16 = {\rm \pi}/128 \simeq 0.0245$, and the lifetime is $t_{lifetime} = 5{\rm \pi} /32 \simeq 0.4909$, while in (b) the curves are drawn at intervals of $t_{touchdown}/15 = {\rm \pi}/80 \simeq 0.0393$, and the lifetime is $t_{lifetime} = t_{touchdown} = 3{\rm \pi} /16 \simeq 0.5890$.

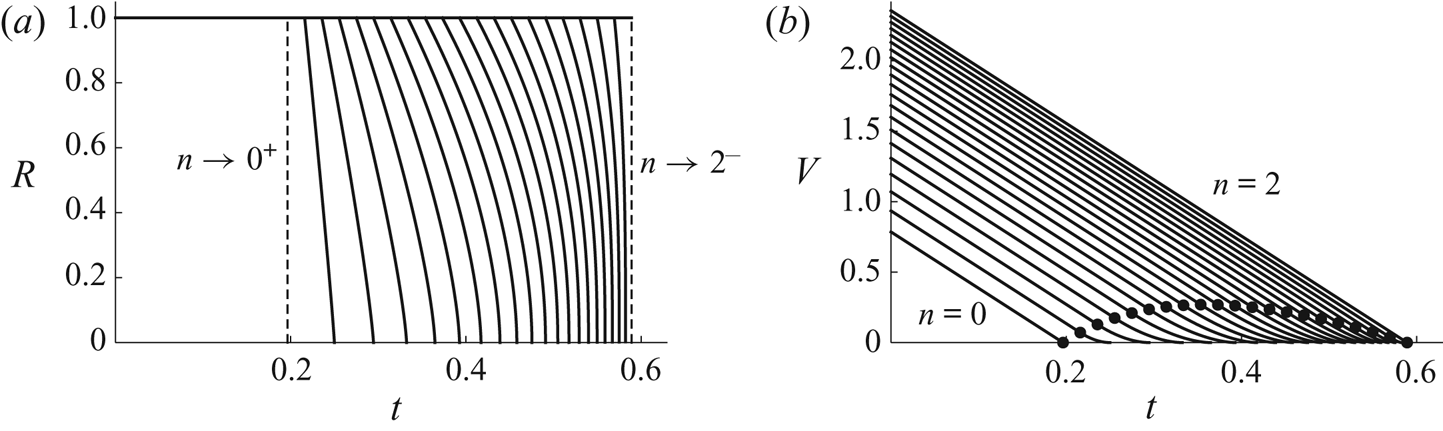
Figure 3. Plots of (a) the radius $R$ of the receding contact line given by (4.14), and (b) the volume $V$ of the droplet given by (3.21d) for $0 \le t \le t_{touchdown}$ and by (4.15) for $t_{touchdown} < t \le t_{lifetime}$ as functions of $t$ for $n = 0$, $1/10$, $1/5$, …, $2$ in the case $H_0 = 1$. The vertical dashed lines in (a) correspond to the limits $n \to 0^{+}$ and $n \to 2^{-}$, and the dots in (b) correspond to touchdown (at the lip of the well) at $t = t_{touchdown} = {\rm \pi}(1 + nH_0)/16$.

The lifetime of the droplet, $t_{lifetime}$, which corresponds to $R = 0$ and $V=0$, is given by

(4.16)\begin{equation} t_{lifetime} = \frac{{\rm \pi}(1 + n + 3n H_0)}{16(1 + n)}, \end{equation}

which is linear in $H_0$. Figure 4 includes a plot of $t_{lifetime}$ given by (4.16) as a function of $n$ for $0 \le n \le 2$ (i.e. to the left of the dots) for a range of values of $H_0$. For a given value of $H_0$, the shortest lifetime is ${\rm \pi} /16$, corresponding to $n = 0$, and the longest lifetime is ${\rm \pi} (1 + 2H_0)/16$, corresponding to $n = 2$; also the longest life after touchdown (i.e. the largest value of $t_{lifetime} - t_{touchdown}$) is $(2 - \sqrt {3}){\rm \pi} H_0/8 \simeq 0.1052 H_0$, corresponding to a well with $n = \sqrt {3} - 1 \simeq 0.7321$.

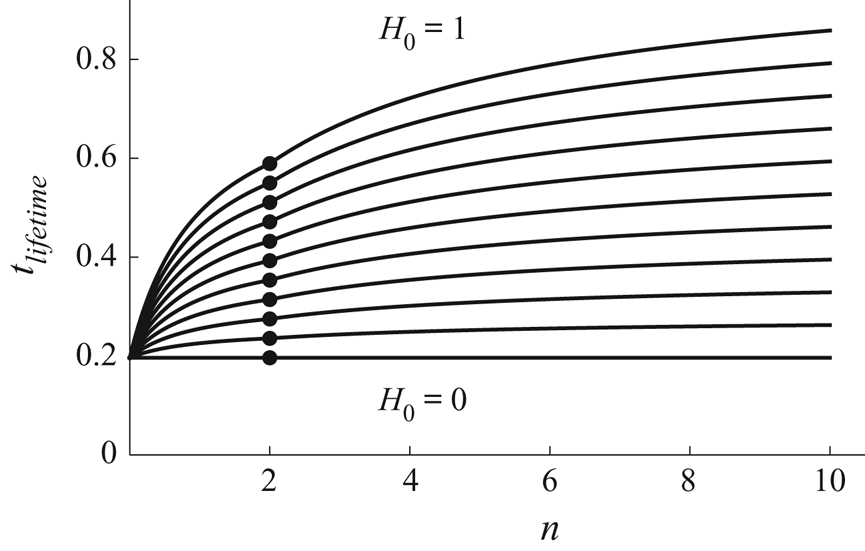
Figure 4. Plot of the lifetime of the droplet, $t_{lifetime}$, given by (4.16) for $0 \le n \le 2$ and by (4.28) for $n > 2$, as a function of $n$ for $H_0 = 0$, $1/10$, $1/5$, …, $1$. The dots denote the values $t_{lifetime} = t_{touchdown} = {\rm \pi}(1 + 2 H_0)/16$ for $n=2$. The curves approach the asymptotic values $t_{lifetime} = {\rm \pi}[1 + 2(1 + 8\alpha _{\infty })H_0]/16 \simeq 0.1963 + 0.8228H_0$ in the limit $n \to \infty$.

4.2. The case $n > 2$

When $n > 2$ the free surface touches down at the centre of the well located at $r = 0$, $z = -H_0$ at $t = t_{touchdown}$, and after touchdown, i.e. for $t_{touchdown} < t \le t_{lifetime}$, the annular droplet has a pinned circular outer contact line $r=1$ and a receding circular inner contact line of radius $R=R(t)$ which satisfies $R(t_{touchdown}) = 0$ and $R(t_{lifetime}) = 1$. We must again specify a condition in addition to $h = H$ at the moving contact line. Since the (paraboloidal) free surface (3.21a) for $0 \le t \le t_{touchdown}$ touches down with zero contact angle at $r = 0$, we again make the natural modelling assumption that the contact angle at the receding contact line remains at the value zero throughout the subsequent evolution, and so the boundary conditions (4.1) again hold. The solution (2.3) for $h$ satisfying (4.1) and $h=0$ at $r=1$ takes the form

(4.17)\begin{equation} h = \frac{H_0\left[\left({-}2R^{2} + nR^{n} - (n - 2)R^{n + 2}\right)\log r - (1 - R^{n} + nR^{n} \log R)(1 - r^{2})\right]} {1 - R^{2} + 2R^{2}\log R}\end{equation}

for $R \le r \le 1$, and $V$ is given by

(4.18)\begin{equation} V = {\rm \pi}H_0 f(R), \end{equation}

where we have defined the function $f = f(R)$ by

(4.19)\begin{equation} f = \frac{n \left[4 -(n + 2)R^{n - 2} + (n - 2)R^{n + 2}\right]}{4(n + 2)} - \frac{\left(1 - R^{2}\right)^{2} \left[(n - 2)R^{n} - n R^{n - 2} + 2\right]}{4 \left(1 - R^{2} + 2 R^{2} \log R\right)}. \end{equation}

It is useful to note that $f \to (n - 2)/[2(n + 2)]$ in the limit $R \to 0^{+}$ (in agreement with the expression for $V$ at touchdown given by (3.24c)), and that $f \sim n^{2}(n - 2)(1 - R)^{4}/36 \to 0^{+}$ in the limit $R \to 1^{-}$.

The (again quasi-static) concentration $c=c(r,z,t)$ of vapour in the atmosphere still satisfies Laplace's equation (3.3) and the boundary condition (3.12), but in this case (3.13)–(3.17) must be replaced with

(4.20)\begin{gather} c = 1 \quad \text{on} \ z = 0 \quad \text{for} \ R \le r \le 1, \end{gather}

(4.21)\begin{gather} \frac{\partial c}{\partial z} = 0 \quad \text{on} \ z = 0 \quad \text{for} \ 0 \le r < R \quad \text{and} \quad \text{for} \ r > 1, \end{gather}

(4.22)\begin{gather} J ={-} \frac{\partial c}{\partial z} \quad \text{on} \ z = 0 \quad \text{for} \ R \le r \le 1. \end{gather}

(4.23)\begin{gather} \frac{\textrm{d} V}{\textrm{d} t} ={-}F, \quad \text{where} \ F = 2{\rm \pi}\int_R^{1} J \, r \, \textrm{d} r, \end{gather}

(4.24)\begin{gather} R = 0, \quad V = \frac{{\rm \pi} (n - 2) H_0}{2(n + 2)} \quad \text{at} \ t = t_{touchdown}, \end{gather}

respectively, where $J=J(r,t)$ is again the local evaporative flux, and $F=F(R)$ (which depends on $t$ via its dependence on $R=R(t)$) is the total evaporative flux from the droplet. In addition, we have

(4.25)\begin{equation} R = 1, \quad V = 0 \quad \text{at} \ t = t_{lifetime}. \end{equation}

As in § 4.1, (4.20), (4.22) and (4.21) for $0 \le r < R$ are applied on $z = 0$.

Perhaps surprisingly, no simple closed-form solution of the problem for $c$ defined by (3.3), (3.12), (4.20) and (4.21) is available (see § 10.1.1 of Popov, Hess & Willert Reference Popov, Heß and Willert2019 for an overview of previous work on this problem in the context of contact mechanics). The problem was reformulated as equivalent integral equations by, for example, Cooke (Reference Cooke1963), Williams (Reference Williams1963) and Fabrikant (Reference Fabrikant1993), and the last-mentioned also gave an iteration-based infinite-series solution to their formulation. Since our primary concern is with the total flux $F$, we obtained this numerically in two independent ways, namely by solving the integral equation of Cooke (Reference Cooke1963) by means of Chebyshev–Gauss quadrature with typically 200 nodes, and by solving Laplace's equation for $c$ using the finite-element package COMSOL Multiphysics 5.3a (COMSOL Inc.), from which $J$ and hence $F$ were obtained; the values of $F$ obtained using these two different approaches were found to be in good agreement.

Figure 5 shows an example of contours of $c$ in the $r$–$z$ plane for an annular droplet obtained using COMSOL Multiphysics, figure 6 shows a plot of the local flux $J$ from an annular droplet as a function of $r$ ($R \le r \le 1$) for a range of values of $R$, as well as that from a non-annular droplet given by (3.19), and figure 7 shows the total flux $F$ from an annular droplet as a function of $R$ ($0 \le R \le 1$). Intriguingly, as figure 6 shows, the local flux $J$ from a non-annular droplet is smaller than that from an annular droplet with the same outer radius, though, of course, the former is effective over a larger area than the latter (i.e. over $0 \le r \le 1$ rather than $R \le r \le 1$), and so leads to a larger value of the total flux $F$. Figure 6 also shows that $J \to \infty$ as $r \to R^{+}$ and $r \to 1^{-}$, and a local analysis shows that $J$ has square-root singularities at both contact lines (i.e. the same singularity as a non-annular droplet). This is true even in the limit $R \to 0^{+}$, showing that the local flux $J$ due to an annular droplet with a vanishingly small hole at its centre is different from that due to a non-annular droplet (for which $J$ is well behaved at $r = 0$); the difference is, however, confined to a small region near to $r = R \to 0^{+}$ whose contribution to the total flux $F$ is small, leading to $F \to 4^{-}$ as $R \to 0^{+}$, in agreement with the value $F = 4$ in the case of a non-annular droplet which appears in (3.20). Note that, as figure 6 shows, $J$ is asymmetric about the midpoint $(R + 1)/2$ between the contact lines, and, as figure 7 shows, $F \to 0^{+}$ as $R \to 1^{-}$, i.e. as the width of the annulus approaches zero.

Figure 5. Plot of contours of the concentration $c$ in the $r$–$z$ plane for an annular droplet in the case $R = 1/2$. The contours are drawn at intervals of $0.04$.

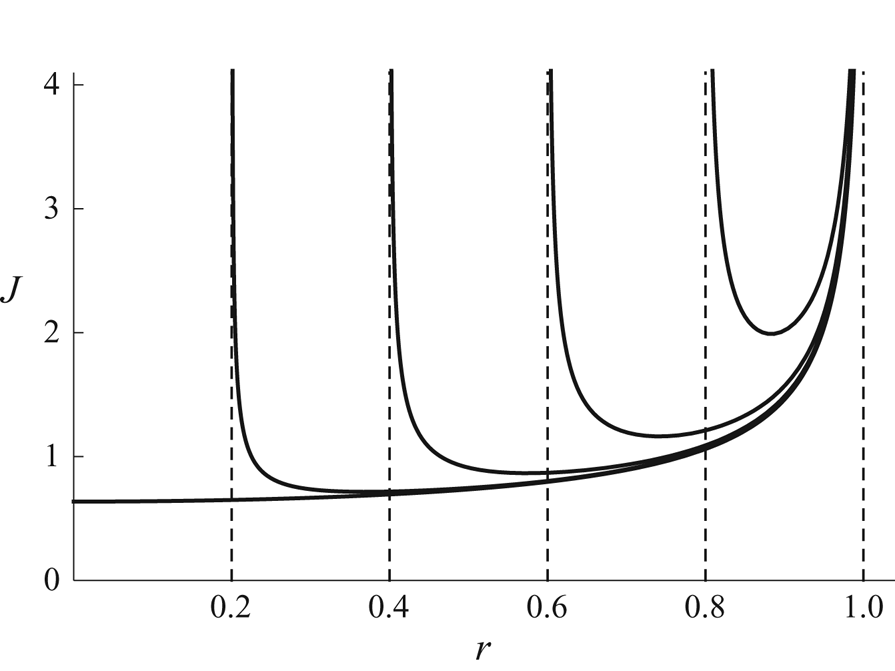
Figure 6. Plot of the local flux $J$ from an annular droplet as a function of $r$ ($R \le r \le 1$) in the cases $R = 0.2$, $0.4$, $0.6$ and $0.8$, as well as that from a non-annular droplet given by (3.19).

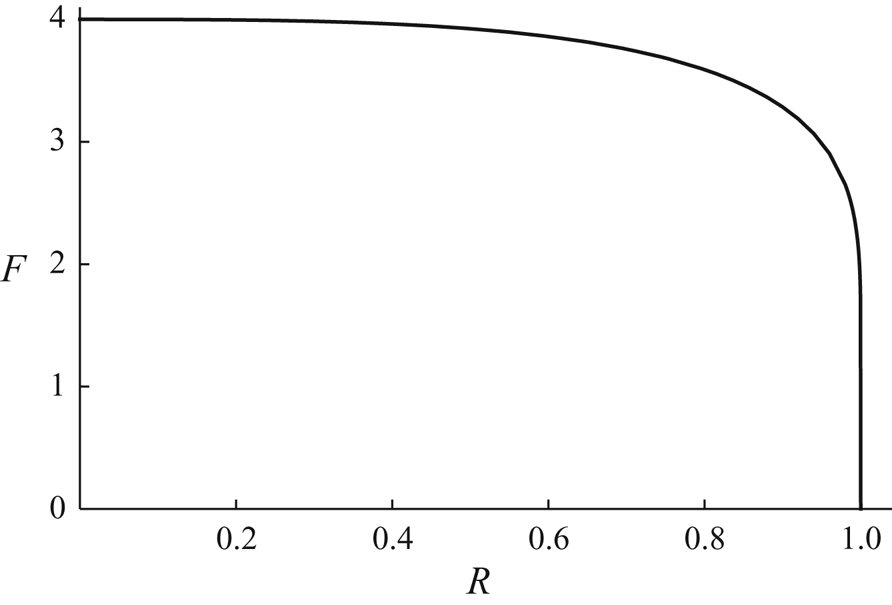
Figure 7. Plot of the total flux $F$ from an annular droplet as a function of $R$ ($0 \le R \le 1$).

As figure 7 shows, the total flux $F$ is nearly independent of $R$ until $R$ gets close to 1, showing that the increase of the perimeter $2{\rm \pi} R$ of the receding inner contact line (where $J$ is singular), which tends to increase the total flux, almost compensates for the decrease of the surface area ${\rm \pi} (1 - R^{2})$ of the annular droplet, which tends to decrease the total flux, for most of the lifetime of the droplet. It is only near to the complete evaporation of the droplet, i.e. when $R$ gets close to 1, that $F$ rapidly decreases to zero.

With the total flux $F$ now known, (4.18) and (4.23) give

(4.26)\begin{equation} {\rm \pi}H_0 f'(R) \frac{\textrm{d} R}{\textrm{d} t} ={-} F(R), \end{equation}

where again a dash denotes differentiation with respect to argument, leading to an implicit solution for $R$ after touchdown, namely

(4.27)\begin{equation} t = t_{touchdown} - {\rm \pi}H_0\int_0^{R} \frac{f'(\hat{R})}{F(\hat{R})} \, \textrm{d} \hat{R}, \end{equation}

with $h$ given by (4.17) and $V$ given by (4.18).

Figure 8(a) shows the evolution of the free-surface profile $h$ for $n=9$ with $H_0=1$. Figure 9 shows plots of $R$ and $V$ as functions of $t$ for a range of values of $n$, again with $H_0 = 1$. The nearly linear dependence of $V$ on $t$ for $t_{touchdown} < t \le t_{lifetime}$ evident in figure 9(b) is a consequence of the fact that the total flux $F$ is nearly independent of $R$ until $R$ gets close to 1 discussed above. Note that $\textrm {d} R/\textrm {d} t \to \infty$ and (although impossible to see in figure 9b) $\textrm {d} V/\textrm {d} t \to 0^{+}$ in the limit $t \to t_{lifetime}^{-}$ (i.e. the same behaviour as when $0 < n < 2$).

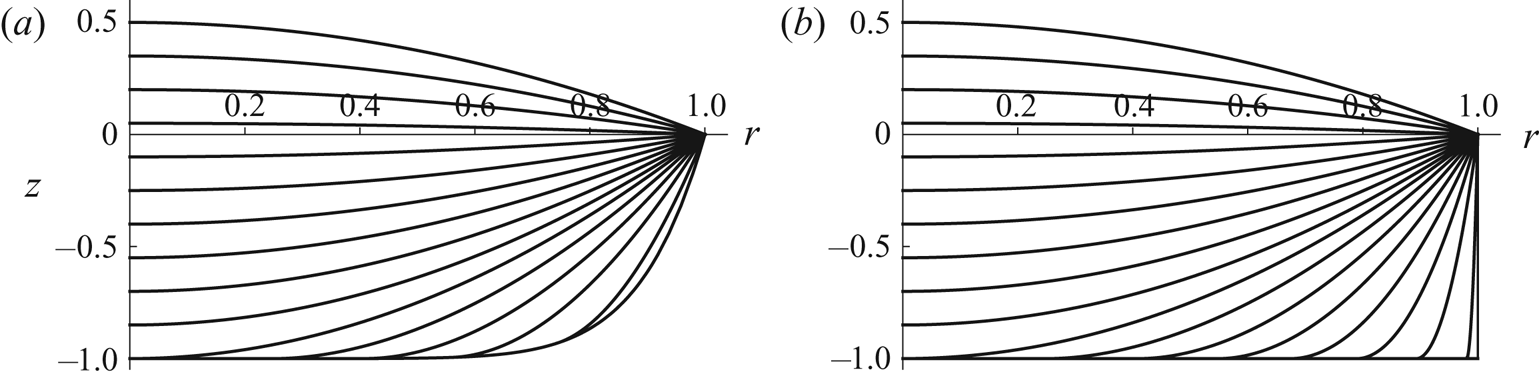
Figure 8. Evolution of the free-surface profile $h$ given by (3.21a) for $0 \le t \le t_{touchdown}$ and by (4.17) for $t_{touchdown} < t \le t_{lifetime}$ for (a) a well with $n = 9$ and $H_0 = 1$, and (b) a cylindrical well (i.e. in the limit $n \to \infty$) with $H_0 = 1$. In (a) the curves are drawn at intervals of $t_{touchdown}/10 \simeq 0.0589$, and the lifetime is $t_{lifetime} \simeq 0.8454$, while in (b) the curves are drawn at intervals of $t_{touchdown}/10 \simeq 0.0589$, and the lifetime is $t_{lifetime} \simeq 1.0193$.

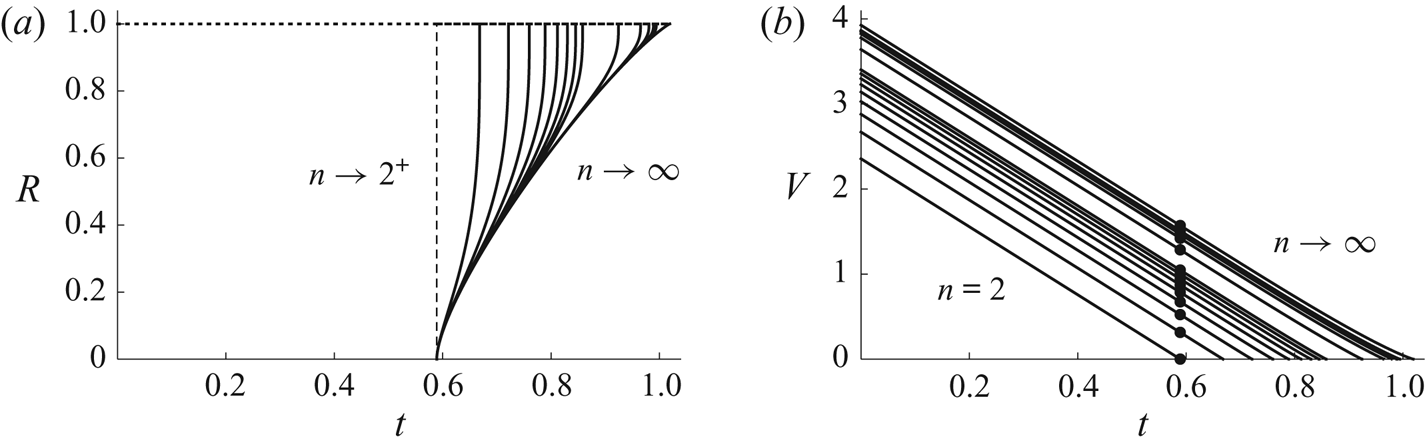
Figure 9. Plots of (a) the radius $R$ of the receding inner contact line given by (4.27), and (b) the volume $V$ of the droplet given by (3.21d) for $0 \le t \le t_{touchdown}$ and by (4.18) for $t_{touchdown} < t \le t_{lifetime}$ as functions of $t$ for $n = 2$, $3$, $4$, …, $10$, $20$, $40$, $60$, $80$ and $100$, and in the limit $n \to \infty$, in the case $H_0 = 1$. The vertical dashed line in (a) corresponds to the limit $n \to 2^{+}$, and the dots in (b) correspond to touchdown (at the centre of the well) at $t = t_{touchdown} = {\rm \pi}(1 + 2H_0)/16 \simeq 0.5890$.

The lifetime of the droplet, which corresponds to $R = 1$ and $V=0$, is given by

(4.28)\begin{equation} t_{lifetime} = t_{touchdown} + {\rm \pi}\alpha H_0 = \frac{\rm \pi}{16}\left[1 + 2\left(1 + 8\alpha\right)H_0\right], \end{equation}

where the function $\alpha = \alpha (n) \, (\ge 0)$ is given by

(4.29)\begin{equation} \alpha ={-}\int_0^{1} \frac{f'(R)}{F(R)} \, \textrm{d} R, \end{equation}

which varies monotonically from $\alpha = 0$ when $n = 2$ to $\alpha = \alpha _{\infty } \simeq 0.1369$ in the limit $n \to \infty$. Note that, like the corresponding expression for $0 < n < 2$ given by (4.16), $t_{lifetime}$ given by (4.28) is linear in $H_0$. Figure 10 shows a plot of $\alpha$ as a function of $n$ for $n \ge 2$, and figure 4 includes a plot of $t_{lifetime}$ given by (4.28) as a function of $n$ for $n > 2$ (i.e. to the right of the dots) for a range of values of $H_0$.

Figure 10. Plot of $\alpha$ given by (4.29) as a function of $n$ for $n \ge 2$. The dashed line shows the asymptotic value $\alpha = \alpha _{\infty } \simeq 0.1369$ in the limit $n \to \infty$.

4.3. The limit $n \to \infty$

In the limit $n \to \infty$, corresponding to a cylindrical well with vertical side $r=1$ and flat bottom $z=-H_0$, after touchdown the annular droplet again has a pinned circular outer contact line $r=1$ and a receding circular inner contact line $r=R$. The solution for $h$ given by (4.17) reduces to

(4.30)\begin{equation} h ={-}\frac{H_0\left(1 - r^{2} + 2R^{2}\log r\right)}{1 - R^{2} + 2R^{2}\log R}\end{equation}

for $R \le r \le 1$, and $V$ is again given by (4.18), where the function $f$ reduces to

(4.31)\begin{equation} f = \frac{1 - R^{4} + 4R^{2}\log R}{2\left(1 - R^{2} + 2R^{2}\log R\right)}. \end{equation}

The evolution of the droplet in this limit is as described in § 4.2, with, in particular, the lifetime of the droplet given by (4.28) with $\alpha = \alpha _{\infty }$, namely

(4.32)\begin{equation} t_{lifetime} = \frac{\rm \pi}{16}[1 + 2(1 + 8\alpha_{\infty})H_0] \simeq 0.1963 + 0.8228H_0. \end{equation}

Figure 8(b) shows the evolution of the free-surface profile $h$ in the limit $n \to \infty$ with $H_0=1$.

4.4. The critical times $t_{flat}$, $t_{touchdown}$ and $t_{lifetime}$

Figure 11 shows a plot of the critical times $t_{flat}$, given by (3.22), $t_{touchdown}$, given by (3.23a) and (3.24a), and $t_{lifetime}$, given by (4.16) and (4.28), as functions of $n$ in the case $H_0 = 1$. In particular, figure 11 illustrates that $t_{flat}$ is independent of $n$, $t_{touchdown}$ increases linearly with $n$ for $0 \le n \le 2$ but is independent of $n$ for $n > 2$, $t_{lifetime}$ increases nonlinearly with $n$, $t_{lifetime} = t_{touchdown} = t_{flat} = {\rm \pi}/16$ when $n=0$, $t_{lifetime} = t_{touchdown} = {\rm \pi}(1 + 2 H_0)/16$ when $n=2$, and $t_{lifetime} \to {\rm \pi}[1 + 2(1 + 8\alpha _{\infty })H_0]/16$ in the limit $n \to \infty$.

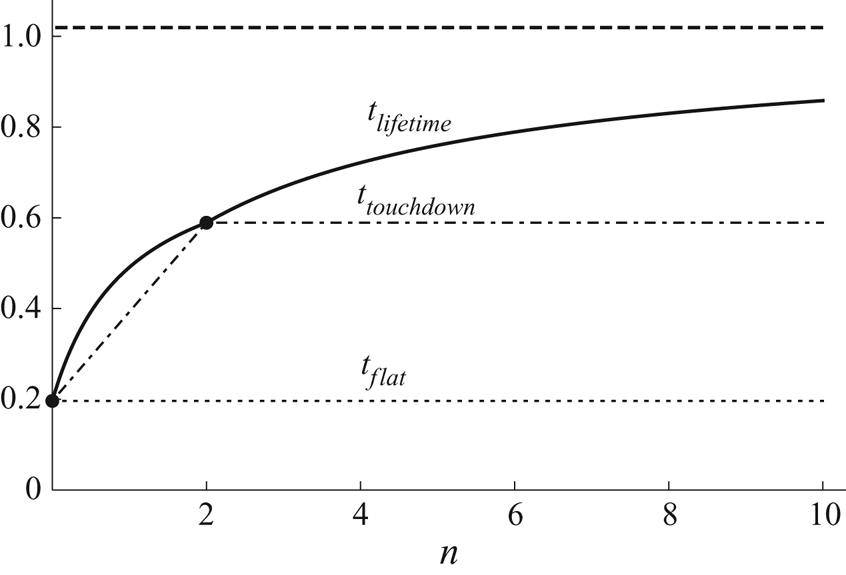
Figure 11. Plot of the critical times $t_{flat}$, given by (3.22) (dotted line), $t_{touchdown}$, given by (3.23a) for $0 \le n \le 2$ and by (3.24a) for $n > 2$ (dash-dotted line), and $t_{lifetime}$, given by (4.16) for $0 \le n \le 2$ and by (4.28) for $n > 2$ (solid line), as functions of $n$ in the case $H_0 = 1$. The dashed line shows the asymptotic value $t_{lifetime} = {\rm \pi}[1 + 2(1 + 8\alpha _{\infty })H_0]/16 \simeq 1.0191$ in the limit $n \to \infty$.

5. Experimental procedure

The experimental procedure employed involved depositing single droplets of the volatile solvent methyl benzoate into shallow axisymmetric cylindrical wells and observing their behaviour as they evaporated. Methyl benzoate is sufficiently volatile that the experiments could be conducted within a reasonable time frame, and its physical properties are consistent with the assumptions of the mathematical model. A schematic diagram of the experimental set-up used is shown in figure 12.

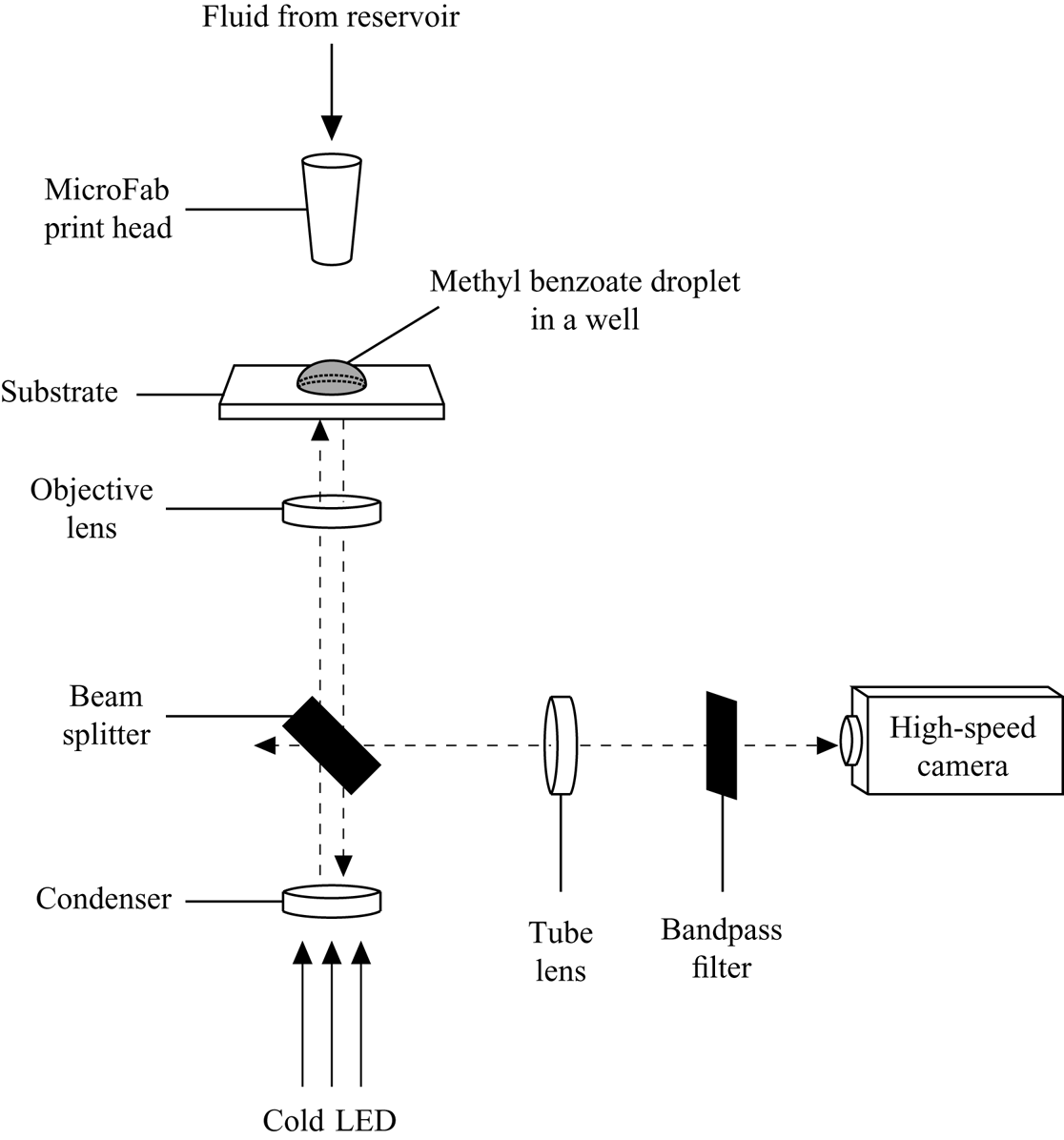
Figure 12. Schematic diagram of the experimental set-up used.

The experiments were carried out under ambient conditions with a relative humidity of methyl benzoate vapour of $RH = 0$ (and a relative humidity of water vapour of $RH = 0.34 \pm 0.10$). The atmospheric pressure was uncontrolled and the ambient temperature was controlled only to within $1\,^{\circ }$C of $22\,^{\circ }$C. The temperature of the substrate was accurately maintained at $22\,^{\circ }$C by means of a proportional-integral-derivative Peltier controller.

The experiments were performed using three shallow wells with radii $29$ $\mathrm {\mu }$m, $50$ $\mathrm {\mu }$m and $75$ $\mathrm {\mu }$m and depths $2.38$ $\mathrm {\mu }$m, $1.87$ $\mathrm {\mu }$m and $2.39$ $\mathrm {\mu }$m, respectively. The wells were fabricated in spin-cast films of photo-resist deposited onto glass substrates coated with indium tin oxide (ITO). Further details of the fabrication of the wells are given in Appendix A. Because of the nature of the manufacturing process, the sides of the wells are not perfectly vertical, but, given their small aspect ratios, it is reasonable to regard the wells as being cylindrical for the purpose of comparison with the theoretical predictions of the mathematical model described in §§ 2–4.

Picolitre droplets of methyl benzoate were ejected into the wells from a MicroFab print head (MJABP-01, Microfab Technologies Inc.) with a circular orifice of diameter $50$ $\mathrm {\mu }$m under a bipolar waveform generated by a MicroFab controller (JetDrive III CT-M3-02, Microfab Technologies Inc.). The droplet was illuminated from underneath by a cold LED at a wavelength of $470$ nm (M470L3, Thorlabs Inc.). The reflected light from the sample was collected by a $50\times$ objective lens (TU Plan ELWD, Nikon) with an image resolution of 0.4 $\mathrm {\mu }$m pixel$^{-1}$, and captured through a bandpass filter (bandwidth $10 \pm 2$ nm, Thorlabs Inc.) with a high-speed camera (FASTCAM SA4, Photron). The experiments were performed six times for each well to verify the reproducibility of the results.

6. Experimental results

Thin-film interferometry was used to measure the evolution of the free surface of the droplet during its evaporation. Figure 13 shows a typical interferometric pattern observed during the present experiments. In particular, the high degree of axisymmetry shown in figure 13 was found in all of the experiments. Further details of the image-analysis procedure used are given in Appendix B. The initial time, $t=0$, was arbitrarily chosen to be a time at which well-resolved interference fringes were observed and the contact line of the droplet coincided with the lip of the well (taken to be at the boundary of the outermost bright fringe, as indicated by the circle in figure 13), i.e. no fluid overflow.

Figure 13. A typical interferometric pattern observed during the present experiments. The circle indicates the lip of the well, the cross the centre of the well, and the line the cross-section along which the fringes are analysed.

Table 1 shows experimental values of the radius $R_0$, the depth H ₀ and the aspect ratio $\epsilon =H_0/R_0$ of the three wells investigated, together with values of the initial angle $\theta _0$ and the initial evaporation rate $\left .\textrm {d} V/\textrm {d} t\right \vert _{t=0}$, and the critical times $t_{flat}$, $t_{touchdown}$ and $t_{lifetime}$, for a representative droplet in each well. Note that since the experimental values of the critical times depend on the arbitrarily chosen initial time, $t=0$, as well as on the values of the ambient temperature and the atmospheric pressure, we give representative (rather than average) results for each well. We will discuss a parameter-free quantity involving the relative values of the critical times in § 7.

Table 1. Experimental values of the radius $R_0$, the depth $H_0$ and the aspect ratio $\epsilon =H_0/R_0$ of the three wells investigated, together with values of the initial angle $\theta _0$ and the initial evaporation rate $\left .\textrm {d} V/\textrm {d} t\right \vert _{t=0}$, and the critical times $t_{flat}$, $t_{touchdown}$ and $t_{lifetime}$, for a representative droplet in each well.

Figure 14 shows experimental results for the free-surface profile $h$ of a droplet before touchdown and paraboloidal fits to these values as functions of $r$ for all three wells at equally spaced times. All of the paraboloidal fits intersect to within $\Delta r = \pm 1$ $\mathrm {\mu }$m and $\Delta h = \pm 0.05$ $\mathrm {\mu }$m of each other, indicating that the contact line of the droplet remains pinned at the lip of the well before touchdown, and the position of the average intersection point was used to determine the radius $R_0$ and the depth $H_0$ of each well. The initial angle $\theta _0$ of the droplet was calculated from the average of the derivatives of the paraboloidal fit to the initial free-surface profile at the lip of the well.

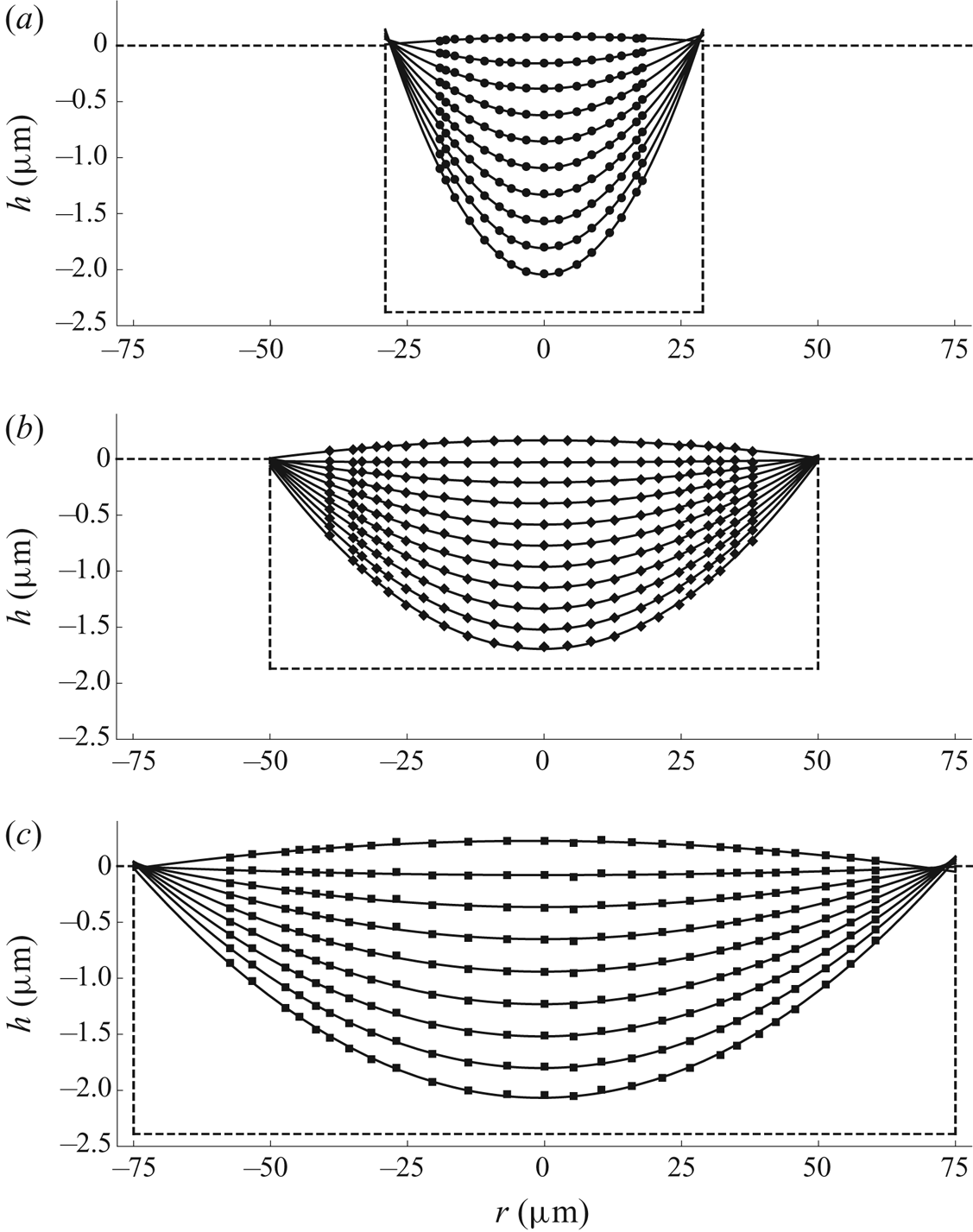
Figure 14. Experimental results for the free-surface profile $h$ of a droplet before touchdown (symbols) and paraboloidal fits to these values (solid black lines) as functions of $r$ for wells of radius $29$ $\mathrm {\mu }$m at times $t=0,0.18, \ldots , 1.62$ s, $50$ $\mathrm {\mu }$m at times $t=0,0.26, \ldots , 2.34$ s and $75$ $\mathrm {\mu }$m at times $t=0,0.56, \ldots , 4.48$ s. The experimental values are denoted by circles, diamonds and squares for the $29$ $\mathrm {\mu }$m, $50$ $\mathrm {\mu }$m and $75$ $\mathrm {\mu }$m wells, respectively. The dashed lines correspond to the radius $R_0$, the depth $H_0$, and the position of the dry substrate for each well.

Figure 15 shows experimental results for the free-surface profile $h$ of a droplet as functions of $t$ for a range of values of $r$ for the $50$ $\mathrm {\mu }$m well. The time at which the free surface is instantaneously flat, $t_{flat}$, was calculated from the average intersection point of the curves shown in figure 15. The uncertainty in the measurement of $t_{flat}$ is $\pm 0.006$ s.

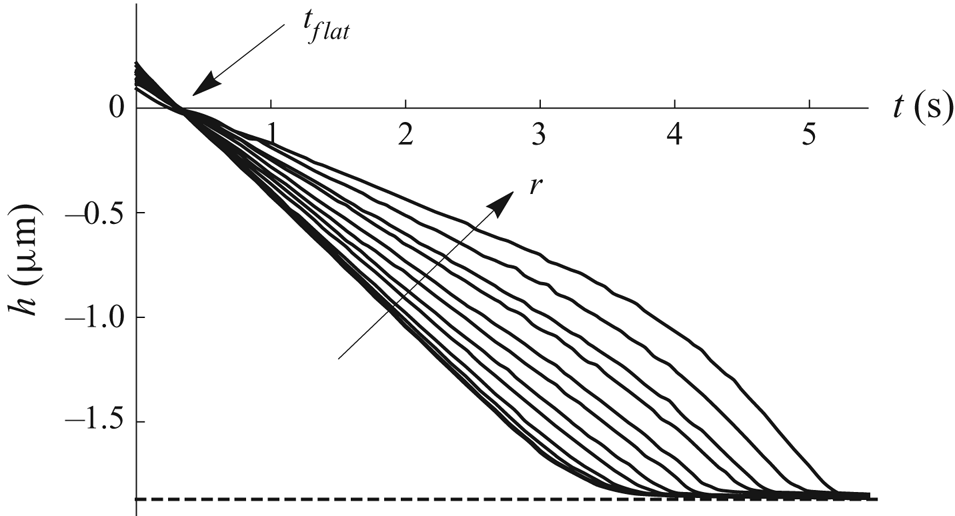
Figure 15. Experimental results for the free-surface profile $h$ of a droplet as functions of $t$ for $r=0$, 4.29, 8.57, 12.86, 17.68, 20.90, 26.79, 30.01, 32.15, 34.83 and 38.04 for the $50$ $\mathrm {\mu }$m well. The arrow indicates the direction of increasing $r$. The dashed line corresponds to the depth $H_0=1.87$ $\mathrm {\mu }$m of the well.

Figure 16 shows experimental results for the normalised height of the free surface at the centre of the well, $h_{m}/H_0$, and linear fits to these values as functions of $t$ for all three wells. For each well, the time at which the free surface touches down at the centre of the well, $t_{touchdown}$, was calculated from the intersection point of the linear fit shown in figure 16 with the bottom of the well. However, note that whereas for the $29$ $\mathrm {\mu }$m well the behaviour of $h_{m}$ is nearly linear until very close to touchdown, and hence the value of $t_{touchdown}$ calculated from the linear fit will be very close to the true value, for the $50$ $\mathrm {\mu }$m and $75$ $\mathrm {\mu }$m wells the behaviour of $h_{m}$ shows a pronounced slowing down as touchdown is approached, and so the values of $t_{touchdown}$ calculated from the linear fits will be underestimates of the true values.

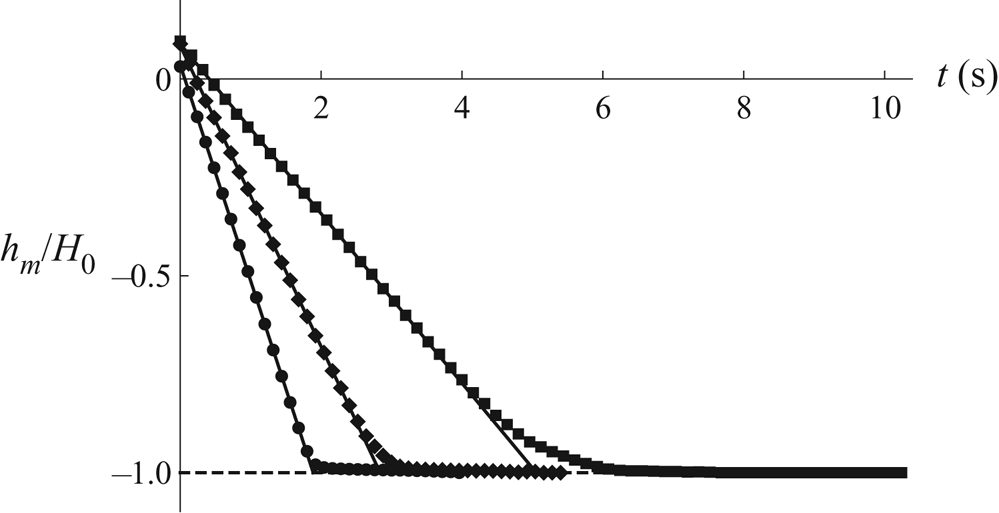
Figure 16. Experimental results for the normalised height of the free surface at the centre of the well, $h_{m}/H_0$, (symbols) and linear fits to these values (solid black lines) as functions of $t$ for wells of radius $29$ $\mathrm {\mu }$m at times $t=0,0.12, \ldots , 3.96$ s, $50$ $\mathrm {\mu }$m at times $t=0,0.12, \ldots , 5.40$ s and $75$ $\mathrm {\mu }$m at times $t=0,0.16, \ldots , 10.24$ s. The experimental values are denoted by circles, diamonds and squares for the $29$ $\mathrm {\mu }$m, $50$ $\mathrm {\mu }$m and $75$ $\mathrm {\mu }$m wells, respectively. The dashed line corresponds to the normalised depth of the wells.

After touchdown, the interference fringes become increasingly closely spaced, making it increasingly difficult to resolve the free-surface profile near to the lip of the well. The resulting lack of experimental results for the free surface means that we cannot accurately determine it for very long after touchdown, and, in particular, that we cannot be certain that the contact line remains pinned at the lip of the well after touchdown (as assumed in the mathematical model). However, given the good agreement between the experimental results and the theoretical predictions of the mathematical model which will be described in § 7, we hypothesise that the effect of any de-pinning that does occur is minimal.

Figure 17 shows experimental results for the normalised volume of a droplet, $V/V_0$, obtained by calculating the volumes of the paraboloidal fits to the free-surface profiles, and linear fits to these values as functions of $t$ for all three wells. Note that the difficulty of resolving the free-surface profile near to the lip of the well after touchdown means that the experimental values shown in figure 17 stop shortly after $t=t_{touchdown}$ for each well. However, figure 17 does show that the behaviour of $V$ is nearly linear until shortly after touchdown.

Figure 17. Experimental results for the normalised volume of a droplet, $V/V_0$, (symbols) and linear fits to these values (solid black lines) as functions of $t$ for wells of radius $29$ $\mathrm {\mu }$m at times $t=0, 0.20, \ldots , 2.00$ s, $50$ $\mathrm {\mu }$m at times $t=0, 0.16, \ldots , 3.84$ s and $75$ $\mathrm {\mu }$m at times $t=0, 0.32, \ldots , 6.40$ s. The experimental values are denoted by circles, diamonds and squares for the $29$ $\mathrm {\mu }$m, $50$ $\mathrm {\mu }$m and $75$ $\mathrm {\mu }$m wells, respectively. The symbols on the $t$-axis correspond to $t=t_{lifetime}$.

Despite the interference fringes becoming increasingly closely spaced after touchdown, we were still able to measure accurately the radius of the inner contact line. This was accomplished by applying an appropriate threshold value to the intensity of the images of the droplets captured during the experiments. However, it should be noted that when the slope of the free surface is very small, this method is sensitive to the value of the threshold used and tends to overestimate the true value of $R$. As previously noted, further details of the image-analysis procedure used are given in Appendix B. The receding inner contact line was occasionally observed to pin temporarily on a defect in the bottom of the well, but this distorted the contact line only in the immediate vicinity of the defect, and only for a time that was short compared with the time scale for the evaporation of the droplet. Figure 18 shows experimental values for the normalised radius of the inner contact line of a droplet, $R/R_0$, as functions of $t$ for all three wells.

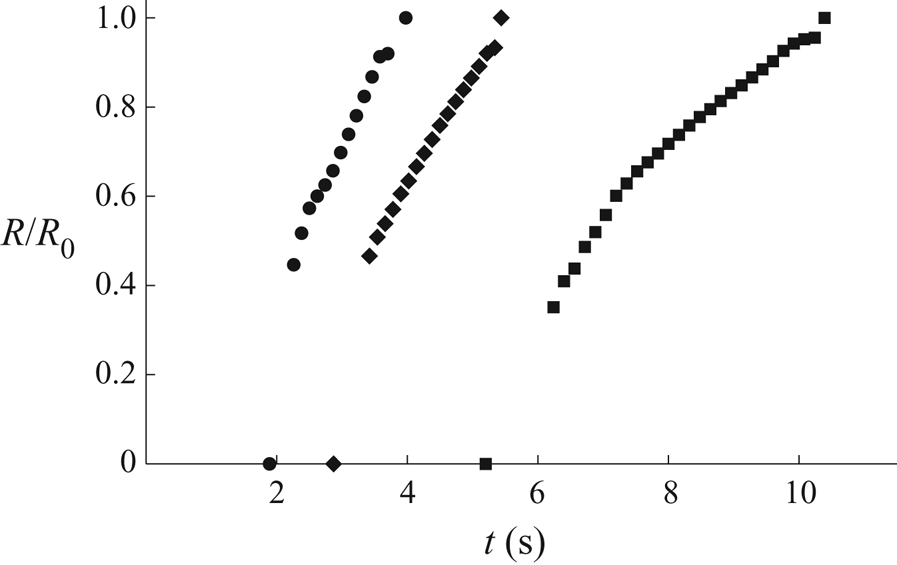
Figure 18. Experimental results for the normalised radius of the inner contact line, $R/R_0$, as functions of $t$ for wells of radius $29$ $\mathrm {\mu }$m at times $t=2.32, 2.38, \ldots , 3.76$ s, $50$ $\mathrm {\mu }$m at times $t=3.38, 3.46, \ldots , 5.30$ s and $75$ $\mathrm {\mu }$m at times $t=6.24, 6.40, \ldots , 10.24$ s. The experimental values are denoted by circles, diamonds and squares for the $29$ $\mathrm {\mu }$m, $50$ $\mathrm {\mu }$m and $75$ $\mathrm {\mu }$m wells, respectively. The symbols on the $t$-axis correspond to $t=t_{touchdown}$, and those at $R/R_0=1$ to $t=t_{lifetime}$.

The lifetime of the droplet, $t_{lifetime}$, was determined visually from the images of the droplet captured during the experiments as the time at which no further change is observed in the contrast at the contact line. The uncertainty in the measurement of $t_{lifetime}$ is $\pm 0.02$ s.

7. Comparison between theory and experiment

We now present comparisons between the theoretical predictions of the mathematical model described in §§ 2–4 in the case of a cylindrical well (i.e. in the limit $n\to \infty$) and the experimental results presented in § 6. Specifically, we compare the evolution of the free-surface profile $h$, the volume of the droplet $V$ and the radius of the inner contact line $R$, as well as the critical times $t_{flat}$, $t_{touchdown}$ and $t_{lifetime}$. The theoretical predictions were calculated using the parameter values $\rho =1.087\times 10^{3}$ kg m$^{-3}$, $c_{sat}=2.251\times 10^{-3}$ kg m$^{-3}$ and $D=6.899\times 10^{-6}$ m$^{2}$ s$^{-1}$ for methyl benzoate at the temperature $22\,^{\circ }$C. The values of $\rho$ and vapour pressure $p_{v}$, the latter of which was used to obtain $c_{sat}$, were calculated from Perry, Green & Maloney (Reference Perry, Green and Maloney1997) (tables 2-30 and 2-6, respectively), and the value of the diffusion coefficient $D$ was calculated from Fuller, Schettler & Giddings (Reference Fuller, Schettler and Giddings1966) (table 1). Note that there are no free parameters in the mathematical model, and no ‘tuning’ of the parameter values has been performed in order to improve the agreement between the experimental results and the theoretical predictions.

As described in § 2, the mathematical model is based on the assumptions that both the Bond number ${Bo}$ and the capillary number ${Ca}$ are small (so that the effect of gravity is negligible and the free surface of the droplet evolves quasi-statically, respectively). Taking the radial velocity scale to be $U=D(c_{sat}-c_{\infty })/(\rho \theta _0R_0)$, and using the values $\mu =1.851\times 10^{-3}$ Pa s and $\gamma =3.720\times 10^{-2}$ N m$^{-1}$ for methyl benzoate at $25\,^{\circ }$C given by Sheu & Tu (Reference Sheu and Tu2005), confirms that the values of ${Bo}$ and ${Ca}$ are indeed small for all of the experimental results presented in § 6. Specifically, the values of ${Bo}$ are approximately $10^{-4}$, $10^{-3}$ and $10^{-3}$ for the $29$ $\mathrm {\mu }$m, $50$ $\mathrm {\mu }$m and $75$ $\mathrm {\mu }$m wells, respectively, and the values of ${Ca}$ are approximately $10^{-2}$ for all three wells.

Figure 19 shows a comparison between the experimental results and the theoretical predictions for the free-surface profile $h$ of a droplet as functions of $r$ for all three wells, while figures 20 and 21 show comparisons between the experimental results and the theoretical predictions for the normalised volume of a droplet, $V/V_0$, and the normalised radius of the inner contact line, $R/R_0$, respectively, as functions of $t$ for all three wells. In particular, figures 19–21 show that, while the theoretical predictions are generally in good agreement with the experimental results (especially for the $50$ $\mathrm {\mu }$m well), the theoretical predictions lag slightly behind the experimental results for the $29$ $\mathrm {\mu }$m and $75$ $\mathrm {\mu }$m wells. This slight lag is due to the sensitivity of the theoretical predictions to the precise values of $c_{sat}$ and $D$ used, as well as to the calculated values of $\theta _0$. In particular, as already mentioned, the ambient temperature was controlled only to within $1\,^{\circ }$C and the value of c _sat is rather sensitive to the precise value of the temperature at which it is evaluated; specifically, $c_{sat}$ changes by $6$–$8\,\%$ for a $1\,^{\circ }$C change in temperature. Fitting the value(s) of $c_{sat}$, $D$ and/or $\theta _0$ would eliminate the lag between the theoretical predictions and the experimental results, especially given that the same values of $c_{sat}$ and $D$ are currently used across all of the experiments, but we deliberately chose not to do this in order to provide a sterner test for the mathematical model.

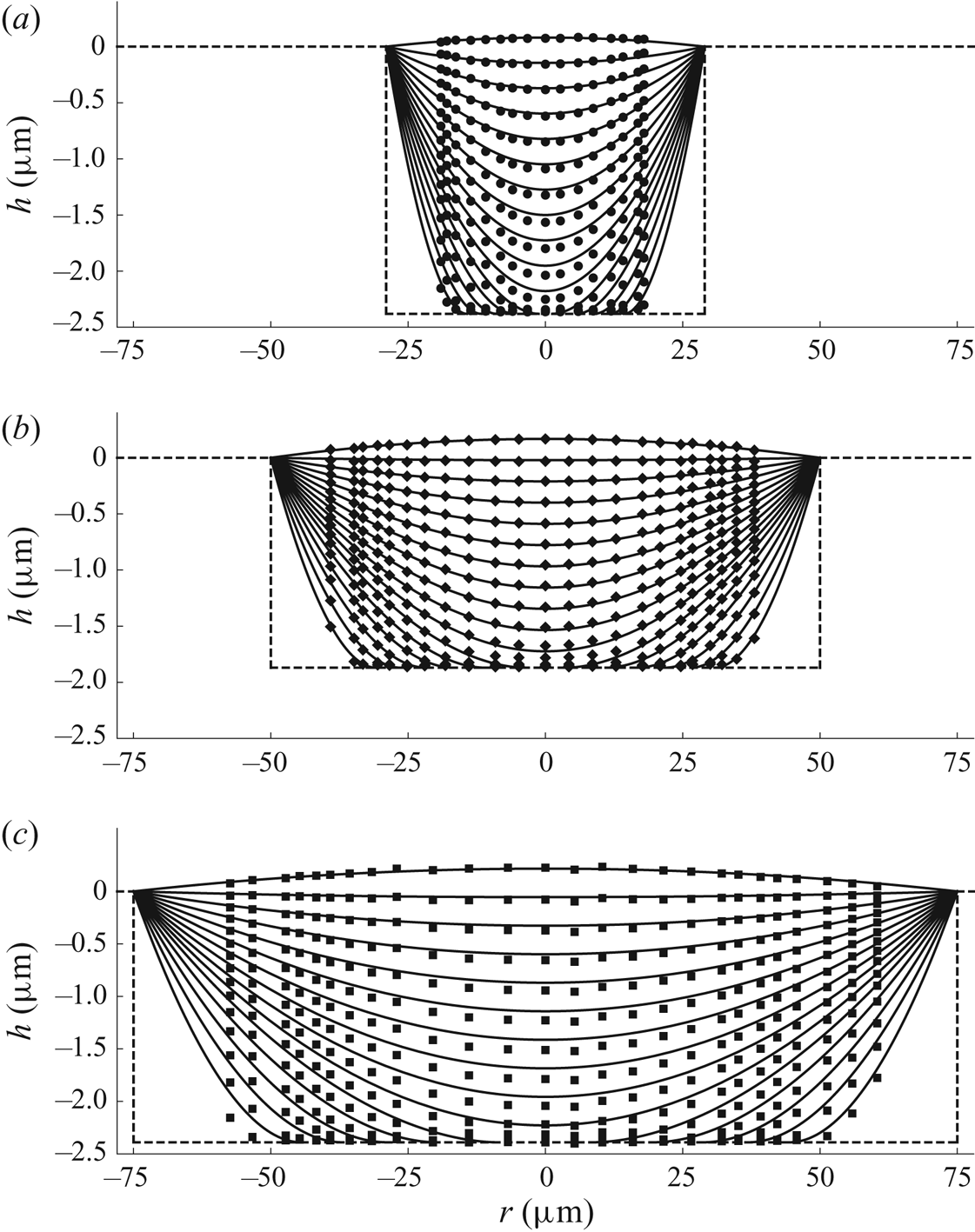
Figure 19. Comparison between the experimental results (symbols) and the theoretical predictions (solid black lines) for the free-surface profile $h$ of a droplet as functions of $r$ for wells of radius (a) $29$ $\mathrm {\mu }$m at times $t=0, 0.18, \ldots , 2.70$ s, (b) $50$ $\mathrm {\mu }$m at times $t=0, 0.26, \ldots , 4.16$ s and (c) $75$ $\mathrm {\mu }$m at times $t=0, 0.56, \ldots , 7.84$ s. The experimental values are denoted by circles, diamonds and squares for the $29$ $\mathrm {\mu }$m, $50$ $\mathrm {\mu }$m and $75$ $\mathrm {\mu }$m wells, respectively. The dashed lines correspond to the radius $R_0$, the depth $H_0$, and the position of the dry substrate for each well.

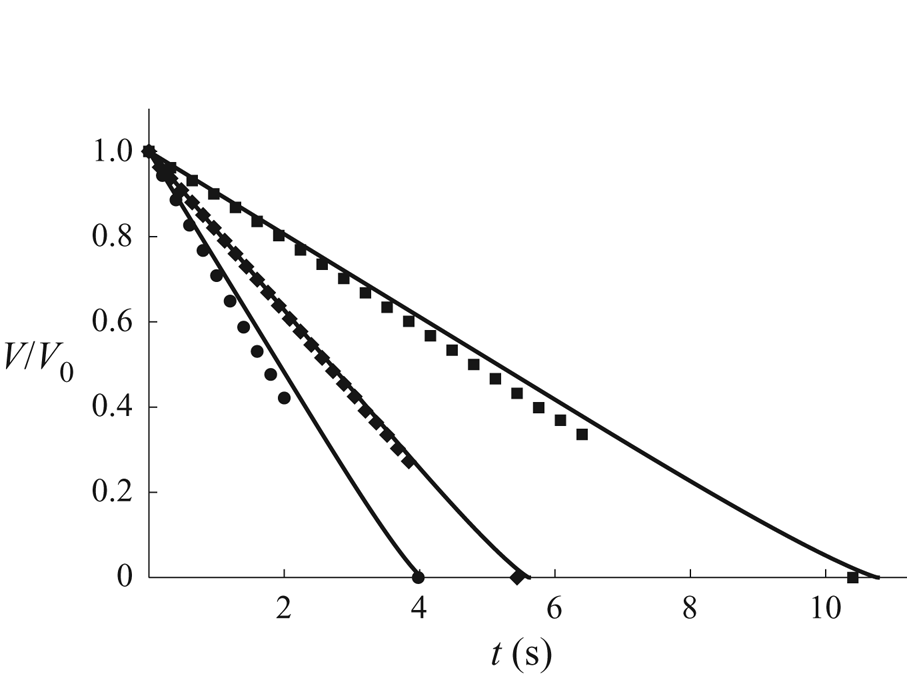
Figure 20. Comparison between the experimental results (symbols) shown in figure 17 and the theoretical predictions (solid black lines) for the normalised volume of a droplet, $V/V_0$, as functions of $t$ for all three wells. The experimental values are denoted by circles, diamonds and squares for the $29$ $\mathrm {\mu }$m, $50$ $\mathrm {\mu }$m and $75$ $\mathrm {\mu }$m wells, respectively.

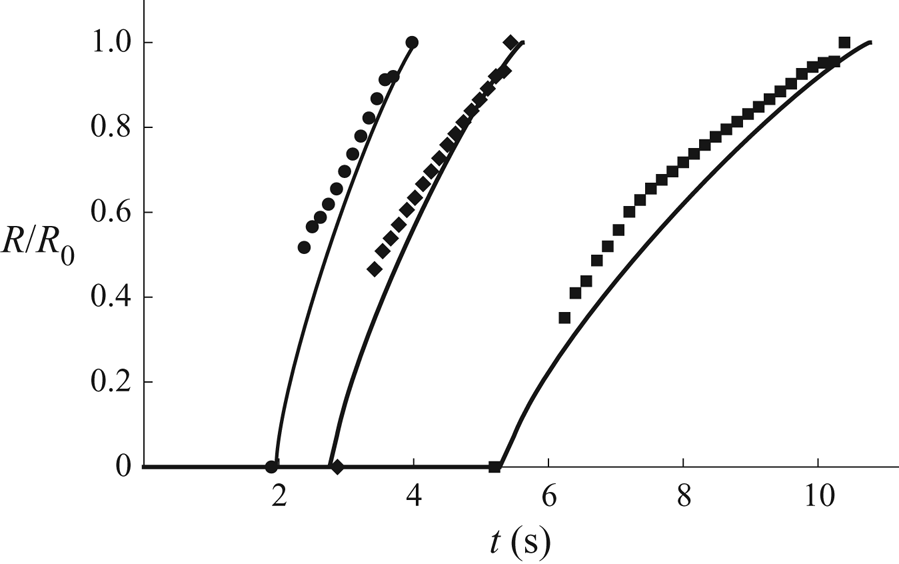
Figure 21. Comparison between the experimental results (symbols) shown in figure 18 and the theoretical predictions (solid black lines) for the normalised radius of the inner contact line, $R/R_0$, as functions of $t$ for all three wells. The experimental values are denoted by circles, diamonds and squares for the $29$ $\mathrm {\mu }$m, $50$ $\mathrm {\mu }$m and $75$ $\mathrm {\mu }$m wells, respectively.

In addition, note that the mathematical model assumes that a new inner contact line appears at the centre of the well at touchdown, and so does not capture the very thin film left briefly on the bottom of the well in the experiments, which is most visible in the experimental results for the $75$ $\mathrm {\mu }$m well shown in figure 19(c). However, the good agreement between the theoretical predictions and the experimental results shown in figures 19–21 indicates that the presence of this film has very little effect on the overall evolution of the droplet. At first sight, it might seem surprising that the most noticeable deviations from the quasi-static free-surface profiles predicted by the mathematical model occur for the shallowest well (i.e. for the well with the smallest value of $\epsilon$). However, while, as already mentioned, the values of ${Ca}$ are small for all three wells, the capillary number is inversely proportional to $\epsilon ^{3}$, and so larger values of ${Ca}$, and hence more significant deviations from quasi-static free-surface profiles, are to be expected for wells with smaller values of $\epsilon$.

As previously noted in § 6, the experimental values of the critical times depend on the arbitrarily chosen initial time, $t=0$, as well as on the values of the ambient temperature and the atmospheric pressure. Moreover, as also previously noted, the theoretical predictions for the critical times are sensitive to the precise values of $c_{sat}$, $D$ and $\theta _0$. In order to remove all of these dependencies, we consider a parameter-free quantity involving the relative values of the critical times, namely

(7.1)\begin{equation} {\mathcal{T}} = \frac{t_{lifetime}-t_{flat}}{t_{touchdown}-t_{flat}}, \end{equation}

which, using the theoretical values of the critical times given by (3.22), (3.24a) and (4.32), takes the same (purely numerical) value, namely ${\mathcal {T}} = 1+8\alpha _{\infty } \simeq 2.095$, regardless of the values of the parameters. Table 2 shows a comparison between the experimental values and the theoretical predictions for $t_{flat}$, $t_{touchdown}$, $t_{lifetime}$ and ${\mathcal {T}}$ for all three wells. Table 2 shows that the theoretical predictions are in good agreement with the experimental values, with average absolute errors of approximately $9\,\%$, $3.8\,\%$, $2.8\,\%$ and $3.3\,\%$ in ${t_{flat}}$, ${t_{touchdown}}$, ${t_{lifetime}}$ and ${{\mathcal {T}}}$, respectively.

Table 2. Comparison between the experimental values and the theoretical predictions for the critical times $t_{flat}$, $t_{touchdown}$ and $t_{lifetime}$, and the parameter-free quantity ${\mathcal {T}}$ given by (7.1) for all three wells.

The comparisons presented in this section show that, despite the inevitable experimental errors and uncertainties about the precise values of the parameters, the mathematical model captures the evolution of a thin droplet in a shallow cylindrical well rather well. The agreement is especially good given that, as already mentioned, there are no free parameters in the mathematical model, and no tuning of the parameter values has been performed in order to improve the agreement.

8. Comparison with previous experimental results

While the experimental results presented in § 6 provide the most comprehensive test for the theoretical predictions of the mathematical model, it is also of interest to consider how well the present model is able to capture experimental results reported by previous authors. Making these comparisons is, unfortunately, hampered by a lack of complete information about the experiments.

Rieger et al. (Reference Rieger, van den Doel and van Vliet2003) studied the evaporation of ethylene glycol droplets in cylindrical nanolitre wells of various radii. In particular, they reported the evolution of the free-surface profile of a droplet before touchdown in a well with radius $100$ $\mathrm {\mu }$m and depth $6.13$ $\mathrm {\mu }$m, and concluded that it was a quasi-static spherical cap that was pinned at the lip of the well. Rieger et al. (Reference Rieger, van den Doel and van Vliet2003) gave the experimental values $t_{flat}=20$ s and $t_{touchdown}=160$ s, but not the experimental value of $t_{lifetime}$, for this droplet, and so, unfortunately, we cannot calculate the experimental value of the parameter-free quantity ${\mathcal {T}}$ given by (7.1). The authors also did not give the value of the initial angle $\theta _0$, but if we calculate it in essentially the same way as we did in § 6, we obtain $\theta _0=0.0144$. The value of the ambient temperature was also not reported, but if we assume that it was $20\,^{\circ }$C, then using the parameter values $\rho =1.114\times 10^{3}$ kg m$^{-3}$, $c_{sat}=2.042\times 10^{-4}$ kg m$^{-3}$ and $D=1.098\times 10^{-5}$ m$^{2}$ s$^{-1}$ calculated from Perry et al. (Reference Perry, Green and Maloney1997) (tables 2-30 and 2-6, respectively) and Fuller et al. (Reference Fuller, Schettler and Giddings1966) (table 1) in exactly the same way as we did in § 7, the theoretical predictions are $t_{flat}=14.05$ s and $t_{touchdown}=133.7$ s, which are in error by $30\,\%$ and $16\,\%$, respectively, compared with the experimental values. It should, however, be noted that, in addition to the uncertainties about the precise values of $c_{sat}$ and $D$ already mentioned, some of this discrepancy may be due to the fact that ethylene glycol is hygroscopic, and so the droplet will absorb water vapour from the atmosphere, which will presumably lead to longer critical times than those predicted by the present mathematical model. Rieger et al. (Reference Rieger, van den Doel and van Vliet2003) did not investigate the evolution of the droplet after touchdown.

Subsequently, Chen et al. (Reference Chen, Tseng and Chieng2006) studied the evaporation of water droplets in cylindrical nanolitre wells of various radii. The experiments were carried out in an atmosphere of air with relative humidity of water vapour of $RH=0.60$ and an ambient temperature of $25\,^{\circ }$C. Chen et al. (Reference Chen, Tseng and Chieng2006) reported quantitative data for the evolution of the radius of the inner contact line $R$, but not for the evolution of the free-surface profile $h$ or the volume of the droplet $V$. Furthermore, they did not report the values of the initial angle $\theta _0$. They did, however, give the critical times for a droplet in a well with radius $250$ $\mathrm {\mu }$m and depth $65$ $\mathrm {\mu }$m to be $t_{flat} = 9 \pm 1$ s (estimated from their figure 9), $t_{touchdown} = 31$ s and $t_{lifetime} = 55$ s. In the absence of the value of $\theta _0$, the only theoretical prediction that can be compared with these experimental results is that for ${\mathcal {T}}$ for the well with radius $250$ $\mathrm {\mu }$m, for which we find that the experimental value ${\mathcal {T}}=2.09 \pm 0.05$ is in very good agreement with the theoretical value ${\mathcal {T}} \simeq 2.095$.