No CrossRef data available.
Article contents
Automaticity and Invariant Measures of Linear Cellular Automata
Published online by Cambridge University Press: 05 September 2019
Abstract
We show that spacetime diagrams of linear cellular automata  $\unicode[STIX]{x1D6F7}:\,\mathbb{F}_{p}^{\mathbb{Z}}\rightarrow \mathbb{F}_{p}^{\mathbb{Z}}$ with
$\unicode[STIX]{x1D6F7}:\,\mathbb{F}_{p}^{\mathbb{Z}}\rightarrow \mathbb{F}_{p}^{\mathbb{Z}}$ with 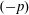 $(-p)$-automatic initial conditions are automatic. This extends existing results on initial conditions that are eventually constant. Each automatic spacetime diagram defines a
$(-p)$-automatic initial conditions are automatic. This extends existing results on initial conditions that are eventually constant. Each automatic spacetime diagram defines a 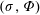 $(\unicode[STIX]{x1D70E},\unicode[STIX]{x1D6F7})$-invariant subset of
$(\unicode[STIX]{x1D70E},\unicode[STIX]{x1D6F7})$-invariant subset of 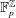 $\mathbb{F}_{p}^{\mathbb{Z}}$, where
$\mathbb{F}_{p}^{\mathbb{Z}}$, where  $\unicode[STIX]{x1D70E}$ is the left shift map, and if the initial condition is not eventually periodic, then this invariant set is nontrivial. For the Ledrappier cellular automaton we construct a family of nontrivial
$\unicode[STIX]{x1D70E}$ is the left shift map, and if the initial condition is not eventually periodic, then this invariant set is nontrivial. For the Ledrappier cellular automaton we construct a family of nontrivial  $(\unicode[STIX]{x1D70E},\unicode[STIX]{x1D6F7})$-invariant measures on
$(\unicode[STIX]{x1D70E},\unicode[STIX]{x1D6F7})$-invariant measures on  $\mathbb{F}_{3}^{\mathbb{Z}}$. Finally, given a linear cellular automaton
$\mathbb{F}_{3}^{\mathbb{Z}}$. Finally, given a linear cellular automaton 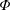 $\unicode[STIX]{x1D6F7}$, we construct a nontrivial
$\unicode[STIX]{x1D6F7}$, we construct a nontrivial  $(\unicode[STIX]{x1D70E},\unicode[STIX]{x1D6F7})$-invariant measure on
$(\unicode[STIX]{x1D70E},\unicode[STIX]{x1D6F7})$-invariant measure on  $\mathbb{F}_{p}^{\mathbb{Z}}$ for all but finitely many
$\mathbb{F}_{p}^{\mathbb{Z}}$ for all but finitely many 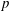 $p$.
$p$.
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2019
Footnotes
This project has received funding from the European Research Council (ERC) under the European Union’s Horizon 2020 research and innovation programme under the Grant Agreement No 648132.



