Hostname: page-component-848d4c4894-8kt4b Total loading time: 0 Render date: 2024-06-23T15:59:08.697Z Has data issue: false hasContentIssue false
  • English
  • Français

A 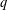$q$-ANALOGUE OF A HYPERGEOMETRIC CONGRUENCE

Part of: Basic hypergeometric functions Discontinuous groups and automorphic forms Elementary number theory

Published online by Cambridge University Press:  18 July 2019

CHENG-YANG GU Open the ORCID record for CHENG-YANG GU [Opens in a new window]  and
VICTOR J. W. GUO Open the ORCID record for VICTOR J. W. GUO [Opens in a new window]
CHENG-YANG GU
Affiliation:
School of Mathematical Sciences, Huaiyin Normal University, Huai’an 223300, Jiangsu, PR China email 525290408@qq.com
VICTOR J. W. GUO*
Affiliation:
School of Mathematical Sciences, Huaiyin Normal University, Huai’an 223300, Jiangsu, PR China email jwguo@math.ecnu.edu.cn
*
✉jwguo@math.ecnu.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

We give a 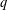$q$-analogue of the following congruence: for any odd prime 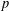$p$,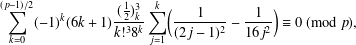

$$\begin{eqnarray}\mathop{\sum }_{k=0}^{(p-1)/2}(-1)^{k}(6k+1)\frac{(\frac{1}{2})_{k}^{3}}{k!^{3}8^{k}}\mathop{\sum }_{j=1}^{k}\biggl(\frac{1}{(2j-1)^{2}}-\frac{1}{16j^{2}}\biggr)\equiv 0\;(\text{mod}\;p),\end{eqnarray}$$
which was originally conjectured by Long and later proved by Swisher. This confirms a conjecture of the second author [‘A $q$-analogue of the (L.2) supercongruence of Van Hamme’, J. Math. Anal. Appl. 466 (2018), 749–761].

Keywords

basic hypergeometric seriesq-congruencecongruencecyclotomic polynomial

MSC classification

Primary: 33D15: Basic hypergeometric functions in one variable, $_rphi_s$
Secondary: 11A07: Congruences; primitive roots; residue systems 11F33: Congruences for modular and $p$-adic modular forms
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 101 , Issue 2 , April 2020 , pp. 294 - 298
Copyright
© 2019 Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The second author was partially supported by the National Natural Science Foundation of China (grant 11771175).

References

Gosper, W., ‘Strip mining in the abandoned orefields of nineteenth century mathematics’, in: Computers in Mathematics (eds. Chudnovsky, D. V. and Jenks, R. D.) (Dekker, New York, 1990), 261–284.Google Scholar
Guo, V. J. W., ‘A q-analogue of the (L.2) supercongruence of Van Hamme’, J. Math. Anal. Appl. 466 (2018), 749–761.Google Scholar
Guo, V. J. W., ‘A q-analogue of a curious supercongruence of Guillera and Zudilin’, J. Difference Equ. Appl. 25 (2019), 342–350.Google Scholar
Guo, V. J. W., ‘Factors of some truncated basic hypergeometric series’, J. Math. Anal. Appl. 476 (2019), 851–859.Google Scholar
Guo, V. J. W. and Schlosser, M. J., ‘Some new q-congruences for truncated basic hypergeometric series’, Symmetry 11(2) (2019), Article 268, 12 pages.Google Scholar
Guo, V. J. W. and Zudilin, W., ‘Ramanujan-type formulae for 1/𝜋: q-analogues’, Integral Transforms Spec. Funct. 29 (2018), 505–513.Google Scholar
Guo, V. J. W. and Zudilin, W., ‘A q-microscope for supercongruences’, Adv. Math. 346 (2019), 329–358.Google Scholar
Long, L., ‘Hypergeometric evaluation identities and supercongruences’, Pacific J. Math. 249 (2011), 405–418.Google Scholar
Osburn, R. and Zudilin, W., ‘On the (K.2) supercongruence of Van Hamme’, J. Math. Anal. Appl. 433 (2016), 706–711.Google Scholar
Rahman, M., ‘Some quadratic and cubic summation formulas for basic hypergeometric series’, Canad. J. Math. 45 (1993), 394–411.Google Scholar
Ramanujan, S., ‘Modular equations and approximations to 𝜋’, Quart. J. Math. Oxford Ser. (2) 45 (1914), 350–372.Google Scholar
Straub, A., ‘Supercongruences for polynomial analogs of the Apéry numbers’, Proc. Amer. Math. Soc. 147 (2019), 1023–1036.Google Scholar
Swisher, H., ‘On the supercongruence conjectures of van Hamme’, Res. Math. Sci. 2 (2015), Article 18, 21 pages.Google Scholar
Van Hamme, L., ‘Some conjectures concerning partial sums of generalized hypergeometric series’, in: p-Adic Functional Analysis (Nijmegen, 1996), Lecture Notes in Pure and Applied Mathematics, 192 (Dekker, New York, 1997), 223–236.Google Scholar