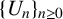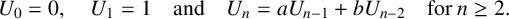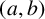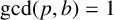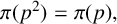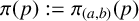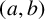1 Introduction
Throughout this note, for positive integers a and b, we let
![]() $\{U_n\}_{n\ge 0}$
be the Lucas sequence of the first kind [6] defined by
$\{U_n\}_{n\ge 0}$
be the Lucas sequence of the first kind [6] defined by
The sequence
![]() $\{U_n\}_{n\ge 0}$
is periodic modulo any prime p with
$\{U_n\}_{n\ge 0}$
is periodic modulo any prime p with
![]() $\gcd (p,b)=1$
, and we denote by
$\gcd (p,b)=1$
, and we denote by
![]() $\pi (p):=\pi _{(a,b)}(p)$
the length of the period of
$\pi (p):=\pi _{(a,b)}(p)$
the length of the period of
![]() $\{U_n\}_{n\ge 0}$
modulo p.
$\{U_n\}_{n\ge 0}$
modulo p.
We define an
![]() $(a,b)$
-Wall–Sun–Sun prime to be a prime p such that
$(a,b)$
-Wall–Sun–Sun prime to be a prime p such that
An
![]() $(a,1)$
-Wall–Sun–Sun prime is also known in the literature as an a-Wall–Sun–Sun prime [10] or an a-Fibonacci–Wieferich prime. Note that when
$(a,1)$
-Wall–Sun–Sun prime is also known in the literature as an a-Wall–Sun–Sun prime [10] or an a-Fibonacci–Wieferich prime. Note that when
![]() $(a,b)=(1,1)$
, the sequence
$(a,b)=(1,1)$
, the sequence
![]() $\{U_n\}_{n\ge 0}$
is the well-known Fibonacci sequence. In this case, such primes are referred to simply as Wall–Sun–Sun primes [Reference Crandall, Dilcher and Pomerance3, 10] or Fibonacci–Wieferich primes [11]. However, at the time this note was written, no Wall–Sun–Sun primes were known to exist. The existence of Wall–Sun–Sun primes was first investigated by Wall [Reference Wall9] in 1960, and subsequently studied by the Sun brothers [Reference Sun and Sun8], who showed that the first case of Fermat’s last theorem is false for exponent p only if p is a Wall–Sun–Sun prime.
$\{U_n\}_{n\ge 0}$
is the well-known Fibonacci sequence. In this case, such primes are referred to simply as Wall–Sun–Sun primes [Reference Crandall, Dilcher and Pomerance3, 10] or Fibonacci–Wieferich primes [11]. However, at the time this note was written, no Wall–Sun–Sun primes were known to exist. The existence of Wall–Sun–Sun primes was first investigated by Wall [Reference Wall9] in 1960, and subsequently studied by the Sun brothers [Reference Sun and Sun8], who showed that the first case of Fermat’s last theorem is false for exponent p only if p is a Wall–Sun–Sun prime.
For an a-Wall–Sun–Sun prime p, it can be shown [Reference Elsenhans and Jahnel4, Reference Jones5] that the following conditions are equivalent:
-
(1)
 $\pi (p^2)=\pi (p)$
;
$\pi (p^2)=\pi (p)$
; -
(2)
 $U_{\pi (p)}\equiv 0 \pmod {p^2}$
;
$U_{\pi (p)}\equiv 0 \pmod {p^2}$
; -
(3)
 $U_{p-\delta _p}\equiv 0 \pmod {p^2}$
, where
$U_{p-\delta _p}\equiv 0 \pmod {p^2}$
, where
 $\delta _p$
is the Legendre symbol
$\delta _p$
is the Legendre symbol
 $\big(\frac{a^2\, {+}\ 4}{p}\big)$
.
$\big(\frac{a^2\, {+}\ 4}{p}\big)$
.
Because of this equivalence, various authors have chosen to use either item (2) or item (3) for the definition of an a-Wall–Sun–Sun prime. However, for the more general
![]() $(a,b)$
-Wall–Sun–Sun prime p, it turns out that, while item (1) implies the still-equivalent items (2) and (3), the converse is false in general. For example, with
$(a,b)$
-Wall–Sun–Sun prime p, it turns out that, while item (1) implies the still-equivalent items (2) and (3), the converse is false in general. For example, with
![]() $(a,b)=(5,8)$
and
$(a,b)=(5,8)$
and
![]() $p=7$
, an easy calculation shows that items (2) and (3) are true, but item (1) is false since
$p=7$
, an easy calculation shows that items (2) and (3) are true, but item (1) is false since
![]() $\pi (49)=42$
and
$\pi (49)=42$
and
![]() $\pi (7)=6$
. Because of this phenomenon, and the fact that Wall [Reference Wall9] was originally concerned with the impossibility of item (1) in the Wall–Sun–Sun situation, we have chosen to adopt (1.2) as our definition of an
$\pi (7)=6$
. Because of this phenomenon, and the fact that Wall [Reference Wall9] was originally concerned with the impossibility of item (1) in the Wall–Sun–Sun situation, we have chosen to adopt (1.2) as our definition of an
![]() $(a,b)$
-Wall–Sun–Sun prime.
$(a,b)$
-Wall–Sun–Sun prime.
This note is motivated in part by recent results of Bouazzaoui [Reference Bouazzaoui1, Reference Bouazzaoui2] which show, under certain restrictions on a, b and p, that an odd prime p is an
![]() $(a,b)$
-Wall–Sun–Sun prime if and only if
$(a,b)$
-Wall–Sun–Sun prime if and only if
![]() ${\mathbb Q}(\sqrt {a^2+4b})$
is not p-rational. We recall that a number field K is p-rational if the Galois group of the maximal pro-p-extension of K which is unramified outside p is a free pro-p-group of rank
${\mathbb Q}(\sqrt {a^2+4b})$
is not p-rational. We recall that a number field K is p-rational if the Galois group of the maximal pro-p-extension of K which is unramified outside p is a free pro-p-group of rank
![]() $r_2 + 1$
, where
$r_2 + 1$
, where
![]() $r_2$
is the number of pairs of complex embeddings of K.
$r_2$
is the number of pairs of complex embeddings of K.
A second motivation for this note is recent work of the second author which, again under certain restrictions on a and p, establishes a connection between
![]() $(a,1)$
-Wall–Sun–Sun primes p and the monogenicity of certain power-compositional trinomials [Reference Jones5].
$(a,1)$
-Wall–Sun–Sun primes p and the monogenicity of certain power-compositional trinomials [Reference Jones5].
One restriction imposed on p in the work of these motivational articles is that
![]() $a^2+4b\not \equiv 0\pmod {p}$
. In this note, our focus is on primes p that divide
$a^2+4b\not \equiv 0\pmod {p}$
. In this note, our focus is on primes p that divide
![]() $a^2+4b$
, and in this case, we provide necessary and sufficient conditions so that p is an
$a^2+4b$
, and in this case, we provide necessary and sufficient conditions so that p is an
![]() $(a,b)$
-Wall–Sun–Sun prime. More precisely, we prove the following result.
$(a,b)$
-Wall–Sun–Sun prime. More precisely, we prove the following result.
Theorem 1.1. Let a and b be positive integers and let p be a prime divisor of
![]() $a^2+4b$
such that
$a^2+4b$
such that
![]() $\gcd (p,b)=1$
. Let
$\gcd (p,b)=1$
. Let
![]() $(a,b)_{m}:=(a \ \mathrm {mod}\ m,b \ \mathrm {mod}\ m)$
. Then
$(a,b)_{m}:=(a \ \mathrm {mod}\ m,b \ \mathrm {mod}\ m)$
. Then
-
•
 $p=2$
is an
$p=2$
is an
 $(a,b)$
-Wall–Sun–Sun prime if and only if
$(a,b)$
-Wall–Sun–Sun prime if and only if
 $(a,b)_4=(0,1)$
;
$(a,b)_4=(0,1)$
; -
•
 $p=3$
is an
$p=3$
is an
 $(a,b)$
-Wall–Sun–Sun prime if and only if
$(a,b)$
-Wall–Sun–Sun prime if and only if  $$ \begin{align*}(a,b)_9\in \{(1,8),(2,5),(4,2),(5,2),(7,5),(8,8)\};\end{align*} $$
$$ \begin{align*}(a,b)_9\in \{(1,8),(2,5),(4,2),(5,2),(7,5),(8,8)\};\end{align*} $$
-
•
 $p\ge 5$
is never an
$p\ge 5$
is never an
 $(a,b)$
-Wall–Sun–Sun prime.
$(a,b)$
-Wall–Sun–Sun prime.
2 Proof of Theorem 1.1
Note that the sequence
![]() $\{U_n\}_{n\ge 0}$
from (1.1) is explicitly
$\{U_n\}_{n\ge 0}$
from (1.1) is explicitly
We let
![]() $\{U_n\}_p$
denote the sequence (2.1) modulo the prime p.
$\{U_n\}_p$
denote the sequence (2.1) modulo the prime p.
We first address the prime
![]() $p=2$
. Since
$p=2$
. Since
![]() $a^2+4b\equiv 0 \pmod {2}$
, it follows that
$a^2+4b\equiv 0 \pmod {2}$
, it follows that
![]() ${a\equiv 0 \pmod {2}}$
. Then, since
${a\equiv 0 \pmod {2}}$
. Then, since
![]() $\gcd (p,b)=1$
, we see from (2.1) that
$\gcd (p,b)=1$
, we see from (2.1) that
Thus,
![]() $\pi (2)=2$
and
$\pi (2)=2$
and
![]() $\pi (4)=2$
if and only if
$\pi (4)=2$
if and only if
![]() $(a,b)_4=(0,1)$
, which finishes the case
$(a,b)_4=(0,1)$
, which finishes the case
![]() ${p=2}$
.
${p=2}$
.
Next, let
![]() $p=3$
. Since
$p=3$
. Since
![]() $a^2+4b\equiv 0 \pmod {3}$
, we see that
$a^2+4b\equiv 0 \pmod {3}$
, we see that
![]() $a^2\equiv -b \pmod {3}$
. Since
$a^2\equiv -b \pmod {3}$
. Since
![]() $\gcd (3,b)=1$
, we deduce that
$\gcd (3,b)=1$
, we deduce that
![]() $b\equiv 2 \pmod {3}$
and
$b\equiv 2 \pmod {3}$
and
![]() $a^2\equiv 1 \pmod {3}$
. Hence, from (2.1),
$a^2\equiv 1 \pmod {3}$
. Hence, from (2.1),
where
![]() $a\equiv 1,2 \pmod {3}$
. We conclude that
$a\equiv 1,2 \pmod {3}$
. We conclude that
 $$ \begin{align*}\pi(3)=\begin{cases} 6&\mbox{if }a\equiv 1 \pmod{3},\\ 3&\mbox{if }a\equiv 2 \pmod{3}. \end{cases}\end{align*} $$
$$ \begin{align*}\pi(3)=\begin{cases} 6&\mbox{if }a\equiv 1 \pmod{3},\\ 3&\mbox{if }a\equiv 2 \pmod{3}. \end{cases}\end{align*} $$
Observe that
![]() $\pi (9)=3$
if and only if
$\pi (9)=3$
if and only if
Since
![]() $b \ \mathrm {mod}\ 9\in \{2,5,8\}$
, it follows that
$b \ \mathrm {mod}\ 9\in \{2,5,8\}$
, it follows that
If
![]() $\pi (9)=6$
, then
$\pi (9)=6$
, then
which implies that
![]() $a^2+b\equiv 0\pmod {9}$
, since
$a^2+b\equiv 0\pmod {9}$
, since
![]() $a^2+b\equiv 0 \pmod {3}$
and
$a^2+b\equiv 0 \pmod {3}$
and
![]() $\gcd (3,b)=1$
. Hence, from (2.1), we have that
$\gcd (3,b)=1$
. Hence, from (2.1), we have that
where
![]() $a^2b^2\equiv 1 \pmod {9}$
. Thus,
$a^2b^2\equiv 1 \pmod {9}$
. Thus,
since we are assuming that
![]() $\pi (9)\ne 3$
. Consequently,
$\pi (9)\ne 3$
. Consequently,
Recall that
![]() $b\equiv 2 \pmod {3}$
. Then, for each
$b\equiv 2 \pmod {3}$
. Then, for each
![]() $b\ \mathrm {mod}\ 9\in \{2,5,8\}$
, solving (2.2) for a yields
$b\ \mathrm {mod}\ 9\in \{2,5,8\}$
, solving (2.2) for a yields
which completes the proof when
![]() $p=3$
.
$p=3$
.
Finally, suppose that
![]() $p\ge 5$
. Since
$p\ge 5$
. Since
we show that
![]() $U_{\pi (p)}\not \equiv 0 \pmod {p^2}$
to establish that p is not an
$U_{\pi (p)}\not \equiv 0 \pmod {p^2}$
to establish that p is not an
![]() $(a,b)$
-Wall–Sun–Sun prime.
$(a,b)$
-Wall–Sun–Sun prime.
We claim that, for
![]() $n\ge 0$
,
$n\ge 0$
,
 $$ \begin{align} U_n\equiv\begin{cases} \dfrac{(-1)^{n/2}ab^{(n-4)/2}n(a^2(n^2-4)+4b(n^2-10))}{48} \pmod{p^2} &\mbox{if }n\mbox{ is even},\\[2ex] \dfrac{(-1)^{(n+1)/2}b^{(n-3)/2}n(a^2(n^2-1)+4b(n^2-7))}{24} \pmod{p^2} &\mbox{if }n\mbox{ is odd.} \end{cases} \end{align} $$
$$ \begin{align} U_n\equiv\begin{cases} \dfrac{(-1)^{n/2}ab^{(n-4)/2}n(a^2(n^2-4)+4b(n^2-10))}{48} \pmod{p^2} &\mbox{if }n\mbox{ is even},\\[2ex] \dfrac{(-1)^{(n+1)/2}b^{(n-3)/2}n(a^2(n^2-1)+4b(n^2-7))}{24} \pmod{p^2} &\mbox{if }n\mbox{ is odd.} \end{cases} \end{align} $$
The proof is by induction on n. The claim is easily verified when
![]() $n\in \{0,1,2\}$
. Since p divides
$n\in \{0,1,2\}$
. Since p divides
![]() $a^2+4b$
, we see that
$a^2+4b$
, we see that
![]() $p^2$
divides
$p^2$
divides
![]() $(a^2+4b)^2=a^4+8a^2b+16b^2$
. It follows that
$(a^2+4b)^2=a^4+8a^2b+16b^2$
. It follows that
Suppose that the claim holds for all
![]() $n\leq t$
for some even integer t. Then, modulo
$n\leq t$
for some even integer t. Then, modulo
![]() $p^2$
,
$p^2$
,
 $$ \begin{align*} U_{t+1} & \equiv aU_{t}+bU_{t-1}\\ & \equiv a\dfrac{(-1)^{t/2}ab^{(t-4)/2}t(a^2(t^2-4)+4b(t^2-10))}{48}\\ & \quad +b\dfrac{(-1)^{t/2}b^{(t-4)/2}(t-1)(a^2(t^2-2t)+4b(t^2-2t-6))}{24}\\ & \equiv(-1)^{t/2}b^{(t-4)/2}\dfrac{a^4(t^3-4t)+6(t+2)(t-3)tba^2+8(t-1)(t^2-2t-6)b^2}{48}\\ & \equiv\dfrac{(-1)^{(t+2)/2}b^{(t-2)/2}(t+1)(a^2t(t+2)+4b(t^2+2t-6))}{24}\quad (\mbox{by}\ (2.4))\\ & \equiv\dfrac{(-1)^{((t+1)+1)/2}b^{((t+1)-3)/2}(t+1)(a^2((t+1)^2-1)+4b((t+1)^2-7))}{24} \end{align*} $$
$$ \begin{align*} U_{t+1} & \equiv aU_{t}+bU_{t-1}\\ & \equiv a\dfrac{(-1)^{t/2}ab^{(t-4)/2}t(a^2(t^2-4)+4b(t^2-10))}{48}\\ & \quad +b\dfrac{(-1)^{t/2}b^{(t-4)/2}(t-1)(a^2(t^2-2t)+4b(t^2-2t-6))}{24}\\ & \equiv(-1)^{t/2}b^{(t-4)/2}\dfrac{a^4(t^3-4t)+6(t+2)(t-3)tba^2+8(t-1)(t^2-2t-6)b^2}{48}\\ & \equiv\dfrac{(-1)^{(t+2)/2}b^{(t-2)/2}(t+1)(a^2t(t+2)+4b(t^2+2t-6))}{24}\quad (\mbox{by}\ (2.4))\\ & \equiv\dfrac{(-1)^{((t+1)+1)/2}b^{((t+1)-3)/2}(t+1)(a^2((t+1)^2-1)+4b((t+1)^2-7))}{24} \end{align*} $$
and
 $$ \begin{align*} U_{t+2} & \equiv aU_{t+1}+bU_{t}\\ & \equiv a\dfrac{(-1)^{((t+1)+1)/2}b^{((t+1)-3)/2}(t+1)(a^2((t+1)^2-1)+4b((t+1)^2-7))}{24}\\ & \quad +b\dfrac{(-1)^{t/2}ab^{(t-4)/2}t(a^2(t^2-4)+4b(t^2-10))}{48}\\ & \equiv (-1)^{t/2}\dfrac{-ab^{(t-2)/2}(a^2(t+2)(t^2+4t)+4b(t+2)(t^2+t-6))}{48}\\ & \equiv \dfrac{(-1)^{(t+2)/2}ab^{((t+2)-4)/2}(t+2)(a^2((t+2)^2-4)+4b((t+2)^2-10))}{48}, \end{align*} $$
$$ \begin{align*} U_{t+2} & \equiv aU_{t+1}+bU_{t}\\ & \equiv a\dfrac{(-1)^{((t+1)+1)/2}b^{((t+1)-3)/2}(t+1)(a^2((t+1)^2-1)+4b((t+1)^2-7))}{24}\\ & \quad +b\dfrac{(-1)^{t/2}ab^{(t-4)/2}t(a^2(t^2-4)+4b(t^2-10))}{48}\\ & \equiv (-1)^{t/2}\dfrac{-ab^{(t-2)/2}(a^2(t+2)(t^2+4t)+4b(t+2)(t^2+t-6))}{48}\\ & \equiv \dfrac{(-1)^{(t+2)/2}ab^{((t+2)-4)/2}(t+2)(a^2((t+2)^2-4)+4b((t+2)^2-10))}{48}, \end{align*} $$
which establishes the claim.
For brevity of notation, we let
![]() $\lambda $
denote the order of
$\lambda $
denote the order of
![]() $2^{-1}a$
modulo p. Then, since
$2^{-1}a$
modulo p. Then, since
![]() $\gcd (p,b)=1$
, it follows that
$\gcd (p,b)=1$
, it follows that
![]() $\pi (p)=p\lambda $
[Reference Renault7, Theorem 3(c)]. Since
$\pi (p)=p\lambda $
[Reference Renault7, Theorem 3(c)]. Since
![]() $\lambda $
divides
$\lambda $
divides
![]() $p-1$
, it follows that
$p-1$
, it follows that
![]() $\gcd (p,\lambda )=1$
. To finish the proof, we must show that
$\gcd (p,\lambda )=1$
. To finish the proof, we must show that
![]() $U_{\pi (p)}\not \equiv 0 \pmod {p^2}$
. We use (2.3).
$U_{\pi (p)}\not \equiv 0 \pmod {p^2}$
. We use (2.3).
If
![]() $\lambda \equiv 0 \pmod {2}$
, then modulo
$\lambda \equiv 0 \pmod {2}$
, then modulo
![]() $p^2$
,
$p^2$
,
 $$ \begin{align*} U_{p\lambda} &\equiv \dfrac{(-1)^{p\lambda/2}ab^{(p\lambda-4)/2}p\lambda(a^2((p\lambda)^2-4)+4b((p\lambda)^2-10))}{48}\\ &\equiv\dfrac{(-1)^{p\lambda/2}ab^{(p\lambda-4)/2}p\lambda(a^2(-4)+4b(-10))}{48}\\ &\equiv\dfrac{(-1)^{\lambda (p+2)/2}4ab^{(p\lambda-4)/2}p\lambda(a^2+10b)}{48}. \end{align*} $$
$$ \begin{align*} U_{p\lambda} &\equiv \dfrac{(-1)^{p\lambda/2}ab^{(p\lambda-4)/2}p\lambda(a^2((p\lambda)^2-4)+4b((p\lambda)^2-10))}{48}\\ &\equiv\dfrac{(-1)^{p\lambda/2}ab^{(p\lambda-4)/2}p\lambda(a^2(-4)+4b(-10))}{48}\\ &\equiv\dfrac{(-1)^{\lambda (p+2)/2}4ab^{(p\lambda-4)/2}p\lambda(a^2+10b)}{48}. \end{align*} $$
Since
![]() $p\not \in \{2,3\}$
and does not divide a, b or
$p\not \in \{2,3\}$
and does not divide a, b or
![]() $\lambda $
, if
$\lambda $
, if
![]() $U_{p\lambda }\equiv 0\pmod {p^2}$
, then p divides
$U_{p\lambda }\equiv 0\pmod {p^2}$
, then p divides
![]() $a^2+10b$
. However, since p divides
$a^2+10b$
. However, since p divides
![]() $a^2+4b$
, it follows that
$a^2+4b$
, it follows that
completing the proof in this case.
Suppose now that
![]() $\lambda \equiv 1 \pmod {2}$
. Then, modulo
$\lambda \equiv 1 \pmod {2}$
. Then, modulo
![]() $p^2$
,
$p^2$
,
 $$ \begin{align*} U_{p\lambda} &\equiv \dfrac{(-1)^{(p\lambda+1)/2}b^{(p\lambda-3)/2}p\lambda(a^2((p\lambda)^2-1)+4b((p\lambda)^2-7))}{24}\\ &\equiv\dfrac{(-1)^{(p\lambda+1)/2}b^{(p\lambda-3)/2}p\lambda(a^2(-1)+4b(-7))}{24}\\ &\equiv\dfrac{(-1)^{(p\lambda+3)/2}b^{(p\lambda-3)/2}p\lambda(a^2+28b)}{24}. \end{align*} $$
$$ \begin{align*} U_{p\lambda} &\equiv \dfrac{(-1)^{(p\lambda+1)/2}b^{(p\lambda-3)/2}p\lambda(a^2((p\lambda)^2-1)+4b((p\lambda)^2-7))}{24}\\ &\equiv\dfrac{(-1)^{(p\lambda+1)/2}b^{(p\lambda-3)/2}p\lambda(a^2(-1)+4b(-7))}{24}\\ &\equiv\dfrac{(-1)^{(p\lambda+3)/2}b^{(p\lambda-3)/2}p\lambda(a^2+28b)}{24}. \end{align*} $$
Reasoning as in the previous case, we see that
![]() $U_{p\lambda }\equiv 0 \pmod {p^2}$
if and only if
$U_{p\lambda }\equiv 0 \pmod {p^2}$
if and only if
![]() ${a^2+28b\equiv 0 \pmod {p}}$
. However, since
${a^2+28b\equiv 0 \pmod {p}}$
. However, since
![]() $a^2+4b\equiv 0\pmod {p}$
, it follows that
$a^2+4b\equiv 0\pmod {p}$
, it follows that
which completes the proof of the theorem.
Acknowledgement
The authors thank the anonymous referee for the suggestions that helped to improve the paper.