Hostname: page-component-848d4c4894-v5vhk Total loading time: 0 Render date: 2024-07-01T17:53:20.816Z Has data issue: false hasContentIssue false
  • English
  • Français

EXACT ANALYTICAL EXPRESSIONS FOR THE FINAL EPIDEMIC SIZE OF AN SIR MODEL ON SMALL NETWORKS

Part of: Equations and systems with randomness General theory in ordinary differential equations Markov processes

Published online by Cambridge University Press:  23 May 2016

K. MCCULLOCH Open the ORCID record for K. MCCULLOCH [Opens in a new window] ,
M. G. ROBERTS Open the ORCID record for M. G. ROBERTS [Opens in a new window]  and
C. R. LAING Open the ORCID record for C. R. LAING [Opens in a new window]
K. MCCULLOCH*
Affiliation:
Institute of Natural and Mathematical Sciences, Massey University, Albany, 0745, New Zealand email K.McCulloch@latrobe.edu.au
M. G. ROBERTS
Affiliation:
Institute of Natural and Mathematical Sciences, Massey University, Albany, 0745, New Zealand email K.McCulloch@latrobe.edu.au
C. R. LAING
Affiliation:
Institute of Natural and Mathematical Sciences, Massey University, Albany, 0745, New Zealand email K.McCulloch@latrobe.edu.au
*
✉K.McCulloch@latrobe.edu.au
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We investigate the dynamics of a susceptible infected recovered (SIR) epidemic model on small networks with different topologies, as a stepping stone to determining how the structure of a contact network impacts the transmission of infection through a population. For an SIR model on a network of 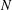$N$ nodes, there are $3^{N}$ configurations that the network can be in. To simplify the analysis, we group the states together based on the number of nodes in each infection state and the symmetries of the network. We derive analytical expressions for the final epidemic size of an SIR model on small networks composed of three or four nodes with different topological structures. Differential equations which describe the transition of the network between states are also derived and solved numerically to confirm our analysis. A stochastic SIR model is numerically simulated on each of the small networks with the same initial conditions and infection parameters to confirm our results independently. We show that the structure of the network, degree of the initial infectious node, number of initial infectious nodes and the transmission rate all significantly impact the final epidemic size of an SIR model on small networks.

Keywords

spread of infectioncontact networkprobability mass functionsnetwork topologyclustering coefficient

MSC classification

Primary: 60J28: Applications of continuous-time Markov processes on discrete state spaces
Secondary: 34A30: Linear equations and systems, general 34F05: Equations and systems with randomness
Type
Research Article
Information
The ANZIAM Journal , Volume 57 , Issue 4 , April 2016 , pp. 429 - 444
Copyright
© 2016 Australian Mathematical Society 

References

Ball, F. and Neal, P., “On methods for studying stochastic disease dynamics”, J. R. Soc. Interface 5 (2008) 171–181; doi:10.1016/j.mbs.2008.01.001.Google Scholar
Bansal, S., Grenfell, B. and Meyers, L., “When individual behaviour matters: homogeneous and network models in epidemiology”, J. R. Soc. Interface 4 (2007) 879–891; doi:10.1098/rsif.2007.1100.CrossRefGoogle ScholarPubMed
Barabási, A. and Albert, R., “Emergence of scaling in random networks”, Science 286 (1999) 509–512; doi:10.1126/science.286.5439.509.CrossRefGoogle ScholarPubMed
Danon, L., Ford, A., House, T., Jewell, C., Kelling, M., Roberts, G., Ross, J. and Vernon, M., “Networks and the epidemiology of infectious disease”, Interdiscip. Perspect. Infect. Dis. 2011 (2011) 1–28; doi:10.1155/2011/284909.CrossRefGoogle ScholarPubMed
Gleeson, J., “High-accuracy approximation of binary-state dynamics on networks”, Phys. Rev. Lett. 107 (2011) 068701; doi:10.1103/physrevlett.107.068701.CrossRefGoogle ScholarPubMed
House, T. and Keeling, M., “Epidemic prediction and control in clustered populations”, J. Theoret. Biol. 272 (2010) 1–7; doi:10.1016/j.jtbi.2010.12.009.CrossRefGoogle ScholarPubMed
Keeling, M., “The effects of local spatial structures on epidemiological invasions”, Proc. R. Soc. Lond. B 266 (1999) 859–867; doi:10.1098/rspb.1999.0716.CrossRefGoogle ScholarPubMed
Keeling, M. and Eames, K., “Networks and epidemic models”, J. R. Soc. Interface 2 (2005) 295–307; doi:10.1098/rsif.2005.0051.Google Scholar
Keeling, M. and Rohani, P., Modeling infectious diseases in humans and animals (Princeton University Press, Princeton, NJ, 2008).CrossRefGoogle Scholar
Kiss, I., Morris, C., Selley, F., Simon, P. and Wilkinson, R., “Exact deterministic representation of Markovian SIR epidemics on networks with and without loops”, J. Math. Biol. 70 (2015) 437–464; doi:10.1007/s00285-014-0772-0.CrossRefGoogle ScholarPubMed
Lindquist, J., Ma, J., van den Driessche, P. and Willeboordse, F., “Effective degree network disease models”, J. Math. Biol. 62 (2011) 143–164; doi:10.1007/s00285-010-0331-2.CrossRefGoogle ScholarPubMed
Lloyd, A. and Valeika, S., “Network models in epidemiology: an overview”, in: World Scientific Lecture Notes in Complex Systems (eds Blasius, B., Kurths, J. and Stone, L.), (World Scientific, Singapore, 2007) 189–214 ; doi:10.1142/9789812771582_0008.Google Scholar
Ma, J., van den Driessche, P. and Willeboordse, F., “The importance of contact network topology for the success of vaccination strategies”, J. Theoret. Biol. 325 (2013) 12–21; doi:10.1016/j.jtbi.2013.01.006.Google Scholar
Marceau, V., Noel, P., Hebert-Dufresne, L., Allard, A. and Dube, L., “Adaptive networks: coevolution of disease and topology”, Phys. Rev. E 82 (2010) 1–9; doi:10.1103/physreve.82.036116.CrossRefGoogle ScholarPubMed
Miller, J., “A note on a paper by Erik Volze: SIR dynamics in random networks”, J. Math. Biol. 62 (2010) 349–358; doi:10.1007/s00285-010-0337-9.Google Scholar
Miller, J. and Kiss, I., “Epidemic spread in networks: existing methods and current challenges”, Math. Model. Nat. Phenom. 9 (2014) 4–42; doi:10.1051/mmnp/20149202.CrossRefGoogle ScholarPubMed
Miller, J., Slim, A. and Volz, E., “Edge-based compartmental modelling for infectious disease spread”, J. R. Soc. Interface 9 (2012) 890–906; doi:10.1098/rsif.2011.0403.Google Scholar
Miller, J. and Volz, E., “Incorporating disease and population structure into models of SIR disease in contact networks”, PLoS ONE 8 (2013) e69162; doi:10.1371/journal.pone.0069162.Google Scholar
Newman, M., Networks: an introduction (Oxford University Press, New York, 2010).CrossRefGoogle Scholar
Pastor-Satorras, R. and Vespignani, A., “Epidemic dynamics and endemic states in complex networks”, Phys. Rev. E 63 (2001) 066117; doi:10.1103/physreve.63.066117.Google Scholar
Pellis, L., Ball, F., Bansal, S., Eames, K., House, T., Isham, V. and Trapman, P., “Eight challenges for network epidemic models”, Epidemics 10 (2015) 58–62; doi:10.1016/j.epidem.2014.07.003.Google Scholar
Ritchie, M., Berthouze, L., House, T. and Kiss, I., “Higher-order structure and epidemic dynamics in clustered networks”, J. Theoret. Biol. 348 (2014) 21–32; doi:10.1016/j.jtbi.2014.01.025.CrossRefGoogle ScholarPubMed
Simon, P., Taylor, M. and Kiss, I., “Exact epidemic models on graphs using graph-automorphism driven lumping”, J. Math. Biol. 62 (2010) 479–508; doi:10.1007/s00285-010-0344-x.CrossRefGoogle ScholarPubMed
Taylor, M., Taylor, T. and Kiss, I., “Epidemic threshold and control in a dynamic network”, Phys. Rev. E 85 (2012) 016103; doi:10.1103/physreve.85.016103.CrossRefGoogle Scholar
Volz, E., “SIR dynamics in random networks with heterogenous connectivity”, J. Math. Biol. 56 (2008) 293–310; doi:10.1007/s00285-007-0116-4.CrossRefGoogle ScholarPubMed
Watts, D. and Strogatz, S., “Collective dynamics of ‘small-world’ networks”, Nature 393 (1998) 440–442; doi:10.1038/30918.CrossRefGoogle ScholarPubMed
Zhao, Y. and Roberts, M., “Simulating epidemics on networks”, Res. Lett. Inform. Math. Sci. 6 (2007) 101–103; http://www.massey.ac.nz/massey/learning/colleges/college-of-sciences/research/natural-mathematical-sciences/mathematics-research/en/simulating-epidemics-on-networks.cfm.Google Scholar
Supplementary material: File

McCulloch supplementary material

McCulloch supplementary material

Download McCulloch supplementary material(File)
File 2.4 MB