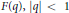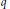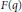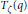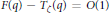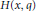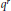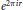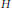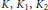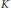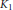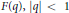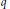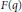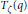In his last letter to Hardy, Ramanujan defined 17 functions $F\left( q \right),\,\left| q \right|\,<\,1$, which he called mock $\theta $-functions. He observed that as $q$ radially approaches any root of unity $\zeta $ at which $F\left( q \right)$ has an exponential singularity, there is a $\theta $-function ${{T}_{\zeta }}\left( q \right)$ with 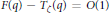$F\left( q \right)\,-\,{{T}_{\zeta }}\left( q \right)\,=\,O\left( 1 \right)$. Since then, other functions have been found that possess this property. These functions are related to a function 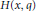$H\left( x,\,q \right)$, where $x$ is usually 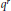${{q}^{r}}$ or 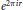${{e}^{2\pi ir}}$ for some rational number $r$. For this reason we refer to 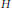$H$ as a “universal” mock $\theta $-function. Modular transformations of 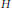$H$ give rise to the functions 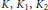$K,\,{{K}_{1}},\,{{K}_{2}}$. The functions 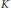$K$ and 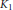${{K}_{1}}$ appear in Ramanujan's lost notebook. We prove various linear relations between these functions using Appell–Lerch sums (also called generalized Lambert series). Some relations (mock theta “conjectures”) involving mock $\theta $-functions of even order and 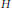$H$ are listed.

We present two infinite families of generalized Lambert series identities, and deduce several known identities from them. They include an identity due to M. Jackson, a corollary of Ramanujan's $_1\psi_1$-summation formula, and a recent identity of G. E. Andrews, R. P. Lewis and Z.-G. Liu.