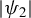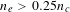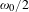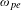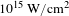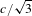4 results
Effects of second-order dispersion of ultrashort laser pulse on stimulated Raman scattering
-
- Journal:
- High Power Laser Science and Engineering / Volume 10 / 2022
- Published online by Cambridge University Press:
- 11 November 2022, e39
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Stimulated Raman scattering in a non-eigenmode regime
-
- Journal:
- High Power Laser Science and Engineering / Volume 8 / 2020
- Published online by Cambridge University Press:
- 21 May 2020, e21
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Absolute instability modes due to rescattering of stimulated Raman scattering in a large nonuniform plasma
-
- Journal:
- High Power Laser Science and Engineering / Volume 7 / 2019
- Published online by Cambridge University Press:
- 28 March 2019, e20
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Trapping of electromagnetic radiation in self-generated and preformed cavities
-
- Journal:
- Laser and Particle Beams / Volume 31 / Issue 4 / December 2013
- Published online by Cambridge University Press:
- 22 August 2013, pp. 589-595
-
- Article
- Export citation