13 results
CHARACTER LEVELS AND CHARACTER BOUNDS
- Part of
-
- Journal:
- Forum of Mathematics, Pi / Volume 8 / 2020
- Published online by Cambridge University Press:
- 24 January 2020, e2
-
- Article
-
- You have access
- Open access
- Export citation
Spinors and essential dimension
- Part of
-
- Journal:
- Compositio Mathematica / Volume 153 / Issue 3 / March 2017
- Published online by Cambridge University Press:
- 02 March 2017, pp. 535-556
- Print publication:
- March 2017
-
- Article
-
- You have access
- HTML
- Export citation
SIMPLE GROUPS STABILIZING POLYNOMIALS
- Part of
-
- Journal:
- Forum of Mathematics, Pi / Volume 3 / 2015
- Published online by Cambridge University Press:
- 15 June 2015, e3
-
- Article
-
- You have access
- Open access
- Export citation
Real class sizes and real character degrees
-
- Journal:
- Mathematical Proceedings of the Cambridge Philosophical Society / Volume 150 / Issue 1 / January 2011
- Published online by Cambridge University Press:
- 22 June 2010, pp. 47-71
- Print publication:
- January 2011
-
- Article
- Export citation
FROBENIUS GROUPS AS MONODROMY GROUPS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 85 / Issue 2 / October 2008
- Published online by Cambridge University Press:
- 01 October 2008, pp. 191-196
- Print publication:
- October 2008
-
- Article
-
- You have access
- Export citation
LOW-DIMENSIONAL REPRESENTATIONS OF SPECIAL LINEAR GROUPS IN CROSS CHARACTERISTICS
-
- Journal:
- Proceedings of the London Mathematical Society / Volume 78 / Issue 1 / January 1999
- Published online by Cambridge University Press:
- 01 January 1999, pp. 116-138
- Print publication:
- January 1999
-
- Article
- Export citation
Monodromy groups of polynomials
-
-
- Book:
- Groups of Lie Type and their Geometries
- Published online:
- 06 January 2010
- Print publication:
- 12 January 1995, pp 125-150
-
- Chapter
- Export citation
29 - The genus of a permutation group
-
-
- Book:
- Groups, Combinatorics and Geometry
- Published online:
- 07 September 2010
- Print publication:
- 10 September 1992, pp 351-363
-
- Chapter
- Export citation
30 - Primitive permutation characters
-
-
- Book:
- Groups, Combinatorics and Geometry
- Published online:
- 07 September 2010
- Print publication:
- 10 September 1992, pp 364-367
-
- Chapter
- Export citation
Generation of the lower central series II
-
- Journal:
- Glasgow Mathematical Journal / Volume 25 / Issue 2 / July 1984
- Published online by Cambridge University Press:
- 18 May 2009, pp. 193-201
- Print publication:
- July 1984
-
- Article
-
- You have access
- Export citation
Generation of the lower central series
-
- Journal:
- Glasgow Mathematical Journal / Volume 23 / Issue 1 / January 1982
- Published online by Cambridge University Press:
- 18 May 2009, pp. 15-20
- Print publication:
- January 1982
-
- Article
-
- You have access
- Export citation
Maximal subfields of algebraically closed fields
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics / Volume 29 / Issue 4 / June 1980
- Published online by Cambridge University Press:
- 09 April 2009, pp. 462-468
- Print publication:
- June 1980
-
- Article
-
- You have access
- Export citation
On groups with decomposable commutator subgroups
-
- Journal:
- Glasgow Mathematical Journal / Volume 19 / Issue 2 / July 1978
- Published online by Cambridge University Press:
- 18 May 2009, pp. 159-162
- Print publication:
- July 1978
-
- Article
-
- You have access
- Export citation



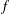
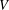


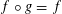
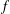
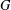
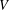
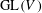
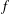
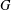
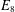
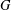
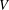
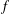
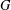
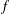
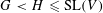
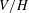
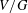
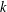
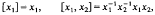
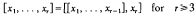
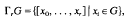
 , and for
, and for 
