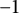1 Introduction
Irregular cusps of a modular curve are cusps where the width of translation is strictly smaller than the width for Fourier expansion. It does not arise frequently, but does exist. At such a cusp, the vanishing order of cusp forms has to be considered carefully, especially when compared with that of pluricanonical forms (cf. [Reference Diamond and Shurman3, Sections 3.2 and 3.3]). In this article, we study and classify irregular cusps for orthogonal groups of signature
![]() $(2, b)$
, and clarify the effect of such cusps on the study of Kodaira dimension of orthogonal modular varieties.
$(2, b)$
, and clarify the effect of such cusps on the study of Kodaira dimension of orthogonal modular varieties.
Let L be a lattice of signature
![]() $(2, b)$
. Let
$(2, b)$
. Let
![]() ${\mathcal {D}}={\mathcal {D}_{L}}$
be the Hermitian symmetric domain attached to L, which is defined as either of the two connected components of the space
${\mathcal {D}}={\mathcal {D}_{L}}$
be the Hermitian symmetric domain attached to L, which is defined as either of the two connected components of the space
We write
![]() ${\mathrm {O}^{+}(L)}$
for the subgroup of the orthogonal group
${\mathrm {O}^{+}(L)}$
for the subgroup of the orthogonal group
![]() $\mathrm {O}(L)$
that preserves the component
$\mathrm {O}(L)$
that preserves the component
![]() ${\mathcal {D}}$
.
${\mathcal {D}}$
.
The domain
![]() ${\mathcal {D}}$
has zero-dimensional and one-dimensional cusps. For simplicity of exposition, we speak only of zero-dimensional cusps for the moment: in fact, the case of one-dimensional cusps can be reduced to that of adjacent zero-dimensional cusps (Proposition 6.3). A zero-dimensional cusp of
${\mathcal {D}}$
has zero-dimensional and one-dimensional cusps. For simplicity of exposition, we speak only of zero-dimensional cusps for the moment: in fact, the case of one-dimensional cusps can be reduced to that of adjacent zero-dimensional cusps (Proposition 6.3). A zero-dimensional cusp of
![]() ${\mathcal {D}}$
corresponds to a rank
${\mathcal {D}}$
corresponds to a rank
![]() $1$
primitive isotropic sublattice I of L. Let
$1$
primitive isotropic sublattice I of L. Let
![]() ${U(I)_{\mathbb {Q}}}$
be the unipotent radical of the stabilizer of I in
${U(I)_{\mathbb {Q}}}$
be the unipotent radical of the stabilizer of I in
![]() $\mathrm {O}^{+}(L_{{\mathbb {Q}}})$
. Then
$\mathrm {O}^{+}(L_{{\mathbb {Q}}})$
. Then
![]() ${U(I)_{\mathbb {Q}}}$
is already abelian: it is a
${U(I)_{\mathbb {Q}}}$
is already abelian: it is a
![]() ${\mathbb {Q}}$
-vector space of dimension b (with a hyperbolic quadratic form). Let
${\mathbb {Q}}$
-vector space of dimension b (with a hyperbolic quadratic form). Let
![]() ${\Gamma }$
be a finite-index subgroup of
${\Gamma }$
be a finite-index subgroup of
![]() ${\mathrm {O}^{+}(L)}$
. The cusp I is called an irregular cusp for
${\mathrm {O}^{+}(L)}$
. The cusp I is called an irregular cusp for
![]() ${\Gamma }$
if
${\Gamma }$
if
![]() ${U(I)_{\mathbb {Q}}}\cap {\Gamma } \ne {U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
. As we will explain,
${U(I)_{\mathbb {Q}}}\cap {\Gamma } \ne {U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
. As we will explain,
![]() ${U(I)_{\mathbb {Z}}}={U(I)_{\mathbb {Q}}}\cap {\Gamma }$
is the lattice for Fourier expansion of
${U(I)_{\mathbb {Z}}}={U(I)_{\mathbb {Q}}}\cap {\Gamma }$
is the lattice for Fourier expansion of
![]() ${\Gamma }$
-modular forms around I, while
${\Gamma }$
-modular forms around I, while
![]() ${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
is the lattice of translation around I in the
${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
is the lattice of translation around I in the
![]() ${\Gamma }$
-action. We give several characterizations of irregularity (Proposition 3.1), including one suitable for explicit calculation.
${\Gamma }$
-action. We give several characterizations of irregularity (Proposition 3.1), including one suitable for explicit calculation.
Irregular cusps are rather rare: they do not exist when
![]() $-\mathrm {id}\in {\Gamma }$
or when
$-\mathrm {id}\in {\Gamma }$
or when
![]() ${\Gamma }$
is neat or when
${\Gamma }$
is neat or when
![]() ${\Gamma }\subset \mathrm {SO}^{+}(L)$
with b odd. But they do exist, in infinitely many examples in every dimension (Section 4.5). Our particular interest is in the so-called stable orthogonal groups
${\Gamma }\subset \mathrm {SO}^{+}(L)$
with b odd. But they do exist, in infinitely many examples in every dimension (Section 4.5). Our particular interest is in the so-called stable orthogonal groups
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
of even lattices L, defined as the kernel of the reduction map
${\widetilde {\mathrm {O}}^{+}(L)}$
of even lattices L, defined as the kernel of the reduction map
![]() ${\mathrm {O}^{+}(L)}\to \mathrm {O}(L^{\vee }/L)$
. This is the group that most frequently appears in the moduli problem related to orthogonal modular varieties. Our calculation concerning
${\mathrm {O}^{+}(L)}\to \mathrm {O}(L^{\vee }/L)$
. This is the group that most frequently appears in the moduli problem related to orthogonal modular varieties. Our calculation concerning
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
can be summarized as follows.
${\widetilde {\mathrm {O}}^{+}(L)}$
can be summarized as follows.
Proposition 1.1 (Sections 4.1 and 4.5)
The stable orthogonal group
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
of an even lattice L has no irregular cusp unless
${\widetilde {\mathrm {O}}^{+}(L)}$
of an even lattice L has no irregular cusp unless
![]() $L^{\vee }/L \simeq {\mathbb {Z}}/8\oplus ({\mathbb {Z}}/2)^{\oplus a}$
or
$L^{\vee }/L \simeq {\mathbb {Z}}/8\oplus ({\mathbb {Z}}/2)^{\oplus a}$
or
![]() $L^{\vee }/L \simeq ({\mathbb {Z}}/4)^{\oplus 2}\oplus ({\mathbb {Z}}/2)^{\oplus a}$
as abelian groups. Conversely, if
$L^{\vee }/L \simeq ({\mathbb {Z}}/4)^{\oplus 2}\oplus ({\mathbb {Z}}/2)^{\oplus a}$
as abelian groups. Conversely, if
![]() $L=U\oplus \langle -8 \rangle \oplus M$
or
$L=U\oplus \langle -8 \rangle \oplus M$
or
![]() $L=U\oplus \langle -4 \rangle ^{\oplus 2} \oplus M$
with
$L=U\oplus \langle -4 \rangle ^{\oplus 2} \oplus M$
with
![]() $M^{\vee }/M$
2-elementary, then
$M^{\vee }/M$
2-elementary, then
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
has an irregular zero-dimensional cusp.
${\widetilde {\mathrm {O}}^{+}(L)}$
has an irregular zero-dimensional cusp.
Consequently, we obtain classification for the following examples from moduli spaces (Section 4):
-
• The modular group for
 $K3$
surfaces of degree
$K3$
surfaces of degree
 $2d$
has an irregular cusp exactly when
$2d$
has an irregular cusp exactly when
 $d=4$
.
$d=4$
. -
• The modular group for irreducible symplectic manifolds of
 $K3^{[t+1]}$
-type with polarization of split type and degree
$K3^{[t+1]}$
-type with polarization of split type and degree
 $2d$
[Reference Gritsenko, Hulek and Sankaran10] has an irregular cusp exactly when
$2d$
[Reference Gritsenko, Hulek and Sankaran10] has an irregular cusp exactly when
 $(t, d)=(1, 4), (2, 2), (4, 1)$
.
$(t, d)=(1, 4), (2, 2), (4, 1)$
. -
• The modular group for O’Grady10 manifolds with polarization of split type and degree
 $2d$
[Reference Gritsenko, Hulek and Sankaran6], which is larger than
$2d$
[Reference Gritsenko, Hulek and Sankaran6], which is larger than
 ${\widetilde {\mathrm {O}}^{+}(L)}$
, has an irregular cusp exactly when
${\widetilde {\mathrm {O}}^{+}(L)}$
, has an irregular cusp exactly when
 $d=4$
.
$d=4$
. -
• Similarly, the modular group for deformation generalized Kummer varieties with polarization of split type and degree
 $2d$
[Reference Dawes2] has an irregular cusp exactly when
$2d$
[Reference Dawes2] has an irregular cusp exactly when
 $(t, d)=(4, 1)$
.
$(t, d)=(4, 1)$
. -
• We will also cover the groups considered in [Reference Fortuna and Mezzedimi4, Reference Petok16, Reference Tanimoto and Várilly-Alvarado18].
A subtle issue concerning irregular cusps, which is the main object of this article, is comparison of the vanishing order between cusp forms and pluricanonical forms. We take a toroidal compactification
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
of the modular variety
${\mathcal {F}(\Gamma )^{\Sigma }}$
of the modular variety
![]() ${\mathcal {F}(\Gamma )}={\Gamma }\backslash {\mathcal {D}}$
. This is defined by choosing a finite collection
${\mathcal {F}(\Gamma )}={\Gamma }\backslash {\mathcal {D}}$
. This is defined by choosing a finite collection
![]() $\Sigma =(\Sigma _{I})$
of suitable fans, one for each
$\Sigma =(\Sigma _{I})$
of suitable fans, one for each
![]() ${\Gamma }$
-equivalence class of rank
${\Gamma }$
-equivalence class of rank
![]() $1$
primitive isotropic sublattices I of L. A ray
$1$
primitive isotropic sublattices I of L. A ray
![]() $\sigma $
in
$\sigma $
in
![]() $\Sigma _{I}$
corresponds to a boundary divisor
$\Sigma _{I}$
corresponds to a boundary divisor
![]() $D(\sigma )$
of the torus embedding
$D(\sigma )$
of the torus embedding
![]() $\overline {{\mathcal {D}}/{U(I)_{\mathbb {Z}}}}$
, and thus determines a boundary divisor
$\overline {{\mathcal {D}}/{U(I)_{\mathbb {Z}}}}$
, and thus determines a boundary divisor
![]() $\Delta (\sigma )$
of
$\Delta (\sigma )$
of
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
as the image of
${\mathcal {F}(\Gamma )^{\Sigma }}$
as the image of
![]() $D(\sigma )$
. The projection
$D(\sigma )$
. The projection
![]() $\overline {{\mathcal {D}}/{U(I)_{\mathbb {Z}}}}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is ramified along
$\overline {{\mathcal {D}}/{U(I)_{\mathbb {Z}}}}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is ramified along
![]() $D(\sigma )$
(with index
$D(\sigma )$
(with index
![]() $2$
) exactly when I is irregular and the ray
$2$
) exactly when I is irregular and the ray
![]() $\sigma $
is irregular in the sense of Definition 3.2.
$\sigma $
is irregular in the sense of Definition 3.2.
The vanishing order
![]() $\nu _{\sigma }(F)$
of a
$\nu _{\sigma }(F)$
of a
![]() ${\Gamma }$
-modular form F at
${\Gamma }$
-modular form F at
![]() $D(\sigma )\subset \overline {{\mathcal {D}}/{U(I)_{\mathbb {Z}}}}$
can be measured by Fourier expansion (Section 8.2): this is done with
$D(\sigma )\subset \overline {{\mathcal {D}}/{U(I)_{\mathbb {Z}}}}$
can be measured by Fourier expansion (Section 8.2): this is done with
![]() ${U(I)_{\mathbb {Z}}}$
. On the other hand, the vanishing order of a pluricanonical form
${U(I)_{\mathbb {Z}}}$
. On the other hand, the vanishing order of a pluricanonical form
![]() $\omega $
on
$\omega $
on
![]() ${\mathcal {F}(\Gamma )}$
should be measured at the level of
${\mathcal {F}(\Gamma )}$
should be measured at the level of
![]() $\Delta (\sigma ) \subset {\mathcal {F}(\Gamma )^{\Sigma }}$
: this is essentially done with
$\Delta (\sigma ) \subset {\mathcal {F}(\Gamma )^{\Sigma }}$
: this is essentially done with
![]() ${U(I)_{\mathbb {Z}}^{\prime }}$
. When
${U(I)_{\mathbb {Z}}^{\prime }}$
. When
![]() $\omega $
is m-canonical and corresponds to F (of weight
$\omega $
is m-canonical and corresponds to F (of weight
![]() $k=mb$
and character
$k=mb$
and character
![]() $\chi =\det ^{m}$
), we have the relation (Proposition 8.7)
$\chi =\det ^{m}$
), we have the relation (Proposition 8.7)
where
![]() $a_{\sigma }=1$
if
$a_{\sigma }=1$
if
![]() $\sigma $
is regular but
$\sigma $
is regular but
![]() $a_{\sigma }=1/2$
if
$a_{\sigma }=1/2$
if
![]() $\sigma $
is irregular due to the boundary ramification. If we are involved only with modular forms of specific parity of weight k, namely k even for
$\sigma $
is irregular due to the boundary ramification. If we are involved only with modular forms of specific parity of weight k, namely k even for
![]() $\chi =1$
(e.g., [Reference Gritsenko, Hulek and Sankaran8, Reference Ma13]) or
$\chi =1$
(e.g., [Reference Gritsenko, Hulek and Sankaran8, Reference Ma13]) or
![]() $k \equiv b$
mod
$k \equiv b$
mod
![]() $2$
for
$2$
for
![]() $\chi =\det $
, we do not need to worry about irregular cusps because we can enlarge
$\chi =\det $
, we do not need to worry about irregular cusps because we can enlarge
![]() ${\Gamma }$
to
${\Gamma }$
to
![]() ${\langle \Gamma , -\mathrm {id} \rangle }$
without any loss. However, if we use a modular form of weight in the remaining parity, we cannot add
${\langle \Gamma , -\mathrm {id} \rangle }$
without any loss. However, if we use a modular form of weight in the remaining parity, we cannot add
![]() $-{\mathrm {id}}$
to
$-{\mathrm {id}}$
to
![]() ${\Gamma }$
, and have to be careful about the coefficient
${\Gamma }$
, and have to be careful about the coefficient
![]() $a_{\sigma }=1/2$
at irregular rays
$a_{\sigma }=1/2$
at irregular rays
![]() $\sigma $
.
$\sigma $
.
Gritsenko, Hulek, and Sankaran [Reference Gritsenko, Hulek and Sankaran7] gave a criterion, called the low weight cusp form trick, for
![]() ${\mathcal {F}(\Gamma )}$
to be of general type in terms of existence of a certain cusp form. It appears that irregular cusps are not covered in [Reference Gritsenko, Hulek and Sankaran7], essentially by assuming
${\mathcal {F}(\Gamma )}$
to be of general type in terms of existence of a certain cusp form. It appears that irregular cusps are not covered in [Reference Gritsenko, Hulek and Sankaran7], essentially by assuming
![]() $-\mathrm {id}\in {\Gamma }$
, explicitly for one-dimensional cusps [Reference Gritsenko, Hulek and Sankaran7, p. 539] and implicitly for zero-dimensional cusps (see a remark below). In view of the coefficient
$-\mathrm {id}\in {\Gamma }$
, explicitly for one-dimensional cusps [Reference Gritsenko, Hulek and Sankaran7, p. 539] and implicitly for zero-dimensional cusps (see a remark below). In view of the coefficient
![]() $a_{\sigma }=1/2$
at irregular
$a_{\sigma }=1/2$
at irregular
![]() $\sigma $
, it seems that this criterion needs to be modified at such boundary divisors. The result is summarized as follows (compare with [Reference Gritsenko, Hulek and Sankaran7, Theorem 1.1]).
$\sigma $
, it seems that this criterion needs to be modified at such boundary divisors. The result is summarized as follows (compare with [Reference Gritsenko, Hulek and Sankaran7, Theorem 1.1]).
Theorem 1.2 (Theorem 8.9)
Let L be a lattice of signature
![]() $(2, b)$
with
$(2, b)$
with
![]() $b\geq 9$
, and let
$b\geq 9$
, and let
![]() ${\Gamma }$
be a subgroup of
${\Gamma }$
be a subgroup of
![]() ${\mathrm {O}^{+}(L)}$
of finite index. We take a
${\mathrm {O}^{+}(L)}$
of finite index. We take a
![]() ${\Gamma }$
-admissible collection
${\Gamma }$
-admissible collection
![]() $\Sigma =(\Sigma _{I})$
of fans so that
$\Sigma =(\Sigma _{I})$
of fans so that
![]() $\Sigma _{I}$
is basic with respect to
$\Sigma _{I}$
is basic with respect to
![]() ${U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
at every zero-dimensional cusp I. Assume that there exists a
${U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
at every zero-dimensional cusp I. Assume that there exists a
![]() ${\Gamma }$
-cusp form F of weight
${\Gamma }$
-cusp form F of weight
![]() $k<b$
and some character satisfying the following:
$k<b$
and some character satisfying the following:
-
(1) F vanishes at the ramification divisor of
 ${\mathcal {D}}\to {\mathcal {F}(\Gamma )}$
.
${\mathcal {D}}\to {\mathcal {F}(\Gamma )}$
. -
(2)
 $\nu _{\sigma }(F)\geq 2$
at every irregular ray
$\nu _{\sigma }(F)\geq 2$
at every irregular ray
 $\sigma $
at every irregular I.
$\sigma $
at every irregular I.
Then
![]() ${\mathcal {F}(\Gamma )}$
is of general type.
${\mathcal {F}(\Gamma )}$
is of general type.
The condition on
![]() $\Sigma $
is imposed in order to ensure that
$\Sigma $
is imposed in order to ensure that
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
has canonical singularities [Reference Gritsenko, Hulek and Sankaran7, Reference Ma13], and this can always be satisfied. When
${\mathcal {F}(\Gamma )^{\Sigma }}$
has canonical singularities [Reference Gritsenko, Hulek and Sankaran7, Reference Ma13], and this can always be satisfied. When
![]() ${\Gamma }$
has no irregular cusp, the condition (2) is vacuous, and this is the criterion in [Reference Gritsenko, Hulek and Sankaran7]; the choice of
${\Gamma }$
has no irregular cusp, the condition (2) is vacuous, and this is the criterion in [Reference Gritsenko, Hulek and Sankaran7]; the choice of
![]() $\Sigma $
does not matter with F and can be dropped (or hidden) from the criterion. Even when
$\Sigma $
does not matter with F and can be dropped (or hidden) from the criterion. Even when
![]() ${\Gamma }$
has an irregular cusp, if the weight k is even for
${\Gamma }$
has an irregular cusp, if the weight k is even for
![]() $\chi =1$
or
$\chi =1$
or
![]() $k\equiv b$
mod
$k\equiv b$
mod
![]() $2$
for
$2$
for
![]() $\chi =\det $
, the condition (2) is still automatically satisfied by the cuspidality of F (Proposition 8.3). However, when
$\chi =\det $
, the condition (2) is still automatically satisfied by the cuspidality of F (Proposition 8.3). However, when
![]() ${\Gamma }$
has an irregular cusp and k belongs to the remaining parity, the condition (2) arises, and the choice of
${\Gamma }$
has an irregular cusp and k belongs to the remaining parity, the condition (2) arises, and the choice of
![]() $\Sigma _{I}$
is then involved with F. Practically it would not be very easy to check (or achieve)
$\Sigma _{I}$
is then involved with F. Practically it would not be very easy to check (or achieve)
![]() $\nu _{\sigma }(F) \geq 2$
for specific F and
$\nu _{\sigma }(F) \geq 2$
for specific F and
![]() $\Sigma _{I}$
. Probably the most plausible scenario would be to expect and check that the group
$\Sigma _{I}$
. Probably the most plausible scenario would be to expect and check that the group
![]() ${\Gamma }$
in question has no irregular cusp. We could say that this is a small cost for using cusp forms of arbitrary weight.
${\Gamma }$
in question has no irregular cusp. We could say that this is a small cost for using cusp forms of arbitrary weight.
By the examples discussed after Proposition 1.1, the general-type results in [Reference Dawes2, Reference Fortuna and Mezzedimi4, Reference Gritsenko, Hulek and Sankaran10–Reference Gritsenko, Hulek and Sankaran7, Reference Petok16, Reference Tanimoto and Várilly-Alvarado18] are not affected. This is our essential purpose.
As a related remark, it should be remembered that in [Reference Ash, Mumford, Rapoport and Tai1], subgroups of
![]() $\mathrm {O}^{+}(L_{{\mathbb {R}}})/\pm \mathrm {id}$
are considered, rather than of
$\mathrm {O}^{+}(L_{{\mathbb {R}}})/\pm \mathrm {id}$
are considered, rather than of
![]() $\mathrm {O}^{+}(L_{{\mathbb {R}}})$
. This means that the given group
$\mathrm {O}^{+}(L_{{\mathbb {R}}})$
. This means that the given group
![]() ${{\Gamma }<{\mathrm {O}^{+}(L)}}$
is replaced by
${{\Gamma }<{\mathrm {O}^{+}(L)}}$
is replaced by
![]() ${\langle \Gamma , -\mathrm {id} \rangle }/\pm {\mathrm {id}}$
. In this situation, it is not
${\langle \Gamma , -\mathrm {id} \rangle }/\pm {\mathrm {id}}$
. In this situation, it is not
![]() ${U(I)_{\mathbb {Z}}}={U(I)_{\mathbb {Q}}}\cap {\Gamma }$
but rather
${U(I)_{\mathbb {Z}}}={U(I)_{\mathbb {Q}}}\cap {\Gamma }$
but rather
![]() ${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
that is written as
${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
that is written as
![]() $U(F)_{{\mathbb {Z}}}$
in the notation of [Reference Ash, Mumford, Rapoport and Tai1]. This is a subtle difference that may arise when working with [Reference Ash, Mumford, Rapoport and Tai1] and that could cause overlooking of irregular cusps.
$U(F)_{{\mathbb {Z}}}$
in the notation of [Reference Ash, Mumford, Rapoport and Tai1]. This is a subtle difference that may arise when working with [Reference Ash, Mumford, Rapoport and Tai1] and that could cause overlooking of irregular cusps.
To conclude, irregular cusps are cusps where the lattice for Fourier expansion is smaller than the lattice of translation. It is the central element
![]() $-\mathrm {id}$
in the Lie group
$-\mathrm {id}$
in the Lie group
![]() $\mathrm {O}^{+}(L_{{\mathbb {R}}})$
that is eventually responsible for the presence of such cusps. We need to be careful about such cusps when we use a cusp form of odd weight with
$\mathrm {O}^{+}(L_{{\mathbb {R}}})$
that is eventually responsible for the presence of such cusps. We need to be careful about such cusps when we use a cusp form of odd weight with
![]() $\chi =1$
or weight
$\chi =1$
or weight
![]() $k\not \equiv b$
mod
$k\not \equiv b$
mod
![]() $2$
with
$2$
with
![]() $\chi =\det $
for constructing a pluricanonical form on
$\chi =\det $
for constructing a pluricanonical form on
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
.
${\mathcal {F}(\Gamma )^{\Sigma }}$
.
This article is organized as follows. In Section 2, we recall the structure of the stabilizer of a zero-dimensional cusp. In Section 3, we define and study irregular zero-dimensional cusps. In Section 4, we give examples of groups
![]() ${\Gamma }$
with/without irregular cusp. In Section 5, we recall the structure of the stabilizer of a one-dimensional cusp. In Section 6, we study irregular one-dimensional cusps. In Section 7, we study some basic properties of a toroidal compactification of
${\Gamma }$
with/without irregular cusp. In Section 5, we recall the structure of the stabilizer of a one-dimensional cusp. In Section 6, we study irregular one-dimensional cusps. In Section 7, we study some basic properties of a toroidal compactification of
![]() ${\mathcal {F}(\Gamma )}$
. In Section 8, we prove Theorem 1.2. The main contents of this article are contained in Sections 3, 4, 6, and 8. Sections 2 and 5 are expository, but we tried to be rather self-contained because of the subtle nature of irregular cusps and for calculation of explicit examples in Section 4.
${\mathcal {F}(\Gamma )}$
. In Section 8, we prove Theorem 1.2. The main contents of this article are contained in Sections 3, 4, 6, and 8. Sections 2 and 5 are expository, but we tried to be rather self-contained because of the subtle nature of irregular cusps and for calculation of explicit examples in Section 4.
Throughout the article, a lattice usually means a free
![]() ${\mathbb {Z}}$
-module of finite rank endowed with a nondegenerate integral symmetric bilinear form
${\mathbb {Z}}$
-module of finite rank endowed with a nondegenerate integral symmetric bilinear form
![]() $(\cdot , \cdot ) : L \times L \to {\mathbb {Z}}$
. In a few occasions, we use the word “lattice” just for a free
$(\cdot , \cdot ) : L \times L \to {\mathbb {Z}}$
. In a few occasions, we use the word “lattice” just for a free
![]() ${\mathbb {Z}}$
-module of finite rank, but no confusion will likely to occur. The dual lattice
${\mathbb {Z}}$
-module of finite rank, but no confusion will likely to occur. The dual lattice
![]() ${\operatorname {Hom}}(L, {\mathbb {Z}})$
of L will be denoted by
${\operatorname {Hom}}(L, {\mathbb {Z}})$
of L will be denoted by
![]() $L^{\vee }$
. A sublattice
$L^{\vee }$
. A sublattice
![]() $I\subset L$
is called primitive when
$I\subset L$
is called primitive when
![]() $L/I$
is free, and isotropic when
$L/I$
is free, and isotropic when
![]() $(I, I)\equiv 0$
. A lattice L is called even if
$(I, I)\equiv 0$
. A lattice L is called even if
![]() $(l, l)\in 2{\mathbb {Z}}$
for every
$(l, l)\in 2{\mathbb {Z}}$
for every
![]() $l\in L$
, but this is not assumed except in Section 4. We write U for the even unimodular lattice of signature
$l\in L$
, but this is not assumed except in Section 4. We write U for the even unimodular lattice of signature
![]() $(1, 1)$
given by the Gram matrix
$(1, 1)$
given by the Gram matrix
 $\begin {pmatrix} 0 & 1 \\ 1 & 0 \end {pmatrix}$
.
$\begin {pmatrix} 0 & 1 \\ 1 & 0 \end {pmatrix}$
.
2 Zero-dimensional cusps
Let L be a lattice of signature
![]() $(2, b)$
. We write
$(2, b)$
. We write
![]() $Q = Q_{L}$
for the isotropic quadric in
$Q = Q_{L}$
for the isotropic quadric in
![]() ${{\mathbb P}}L_{{\mathbb {C}}}$
defined by
${{\mathbb P}}L_{{\mathbb {C}}}$
defined by
![]() $(\omega , \omega )=0$
. The Hermitian symmetric domain attached to L is the open set of Q
$(\omega , \omega )=0$
. The Hermitian symmetric domain attached to L is the open set of Q
where
![]() $+$
means the choice of a connected component. The domain
$+$
means the choice of a connected component. The domain
![]() ${\mathcal {D}}$
has two types of rational boundary components (cusps): zero-dimensional and one-dimensional cusps. They correspond to primitive isotropic sublattices of L of ranks
${\mathcal {D}}$
has two types of rational boundary components (cusps): zero-dimensional and one-dimensional cusps. They correspond to primitive isotropic sublattices of L of ranks
![]() $1$
and
$1$
and
![]() $2$
, respectively. In this section, we recall the structure of the stabilizer of a zero-dimensional cusp and partial toroidal compactification over it. Although the contents of this section are quite standard (cf. [Reference Gritsenko, Hulek and Sankaran7, Reference Kondō11, Reference Looijenga12, Reference Scattone17]), we tried to be rather self-contained and explicit for two reasons: because of the subtle nature of irregular cusps (Section 3), and for the sake of calculation of explicit examples (Section 4).
$2$
, respectively. In this section, we recall the structure of the stabilizer of a zero-dimensional cusp and partial toroidal compactification over it. Although the contents of this section are quite standard (cf. [Reference Gritsenko, Hulek and Sankaran7, Reference Kondō11, Reference Looijenga12, Reference Scattone17]), we tried to be rather self-contained and explicit for two reasons: because of the subtle nature of irregular cusps (Section 3), and for the sake of calculation of explicit examples (Section 4).
2.1 Tube domain model
Throughout this section, we fix a rank
![]() $1$
primitive isotropic sublattice I of L. The zero-dimensional cusp corresponding to I is the point
$1$
primitive isotropic sublattice I of L. The zero-dimensional cusp corresponding to I is the point
![]() ${{\mathbb P}}I_{{\mathbb {C}}}$
of Q. We abbreviate
${{\mathbb P}}I_{{\mathbb {C}}}$
of Q. We abbreviate
![]() $I^{\perp }=I^{\perp }\cap L$
and write
$I^{\perp }=I^{\perp }\cap L$
and write
Twisting by I, not choosing its generator, will be rather essential. The quadratic form on
![]() $I^{\perp }/I$
and an isomorphism
$I^{\perp }/I$
and an isomorphism
![]() $I\simeq {\mathbb {Z}}$
define a hyperbolic quadratic form on
$I\simeq {\mathbb {Z}}$
define a hyperbolic quadratic form on
![]() $L(I)$
. This is independent of the choice of
$L(I)$
. This is independent of the choice of
![]() $I\simeq {\mathbb {Z}}$
. We denote by
$I\simeq {\mathbb {Z}}$
. We denote by
![]() ${\mathcal {C}_{I}}$
the positive cone in
${\mathcal {C}_{I}}$
the positive cone in
![]() $L(I)_{{\mathbb {R}}}$
, namely a chosen connected component of
$L(I)_{{\mathbb {R}}}$
, namely a chosen connected component of
![]() $\{ w \in L(I)_{{\mathbb {R}}} \: | \: (w, w)>0 \}$
.
$\{ w \in L(I)_{{\mathbb {R}}} \: | \: (w, w)>0 \}$
.
We write
![]() ${\mathcal {D}(I)}=Q - Q\cap {{\mathbb P}}I_{{\mathbb {C}}}^{\perp }$
. Then
${\mathcal {D}(I)}=Q - Q\cap {{\mathbb P}}I_{{\mathbb {C}}}^{\perp }$
. Then
![]() ${\mathcal {D}}$
is contained in
${\mathcal {D}}$
is contained in
![]() ${\mathcal {D}(I)}$
. Indeed, if
${\mathcal {D}(I)}$
. Indeed, if
![]() $[\omega ]\in {\mathcal {D}}\cap {{\mathbb P}}I_{{\mathbb {C}}}^{\perp }$
, the positive-definite plane
$[\omega ]\in {\mathcal {D}}\cap {{\mathbb P}}I_{{\mathbb {C}}}^{\perp }$
, the positive-definite plane
![]() $\langle \mathrm {Re}(\omega ), \mathrm {Im}(\omega ) \rangle $
would be contained in
$\langle \mathrm {Re}(\omega ), \mathrm {Im}(\omega ) \rangle $
would be contained in
![]() $I_{{\mathbb {R}}}^{\perp }/I_{{\mathbb {R}}}$
, which contradicts the hyperbolicity of
$I_{{\mathbb {R}}}^{\perp }/I_{{\mathbb {R}}}$
, which contradicts the hyperbolicity of
![]() $I_{{\mathbb {R}}}^{\perp }/I_{{\mathbb {R}}}$
. The linear projection
$I_{{\mathbb {R}}}^{\perp }/I_{{\mathbb {R}}}$
. The linear projection
![]() ${{\mathbb P}}L_{{\mathbb {C}}}\dashrightarrow {{\mathbb P}}(L/I)_{{\mathbb {C}}}$
from the point
${{\mathbb P}}L_{{\mathbb {C}}}\dashrightarrow {{\mathbb P}}(L/I)_{{\mathbb {C}}}$
from the point
![]() ${{\mathbb P}}I_{{\mathbb {C}}}\in Q$
defines an isomorphism
${{\mathbb P}}I_{{\mathbb {C}}}\in Q$
defines an isomorphism
If we choose a rank
![]() $1$
sublattice
$1$
sublattice
![]() $I'$
of L with
$I'$
of L with
![]() $(I, I')\not \equiv 0$
, this defines the base point
$(I, I')\not \equiv 0$
, this defines the base point
![]() ${{\mathbb P}}(\langle I, I' \rangle _{{\mathbb {C}}}/I_{{\mathbb {C}}})$
of the affine space
${{\mathbb P}}(\langle I, I' \rangle _{{\mathbb {C}}}/I_{{\mathbb {C}}})$
of the affine space
![]() ${{\mathbb P}}(L/I)_{{\mathbb {C}}} - {{\mathbb P}}(I^{\perp }/I)_{{\mathbb {C}}}$
, and hence an isomorphism
${{\mathbb P}}(L/I)_{{\mathbb {C}}} - {{\mathbb P}}(I^{\perp }/I)_{{\mathbb {C}}}$
, and hence an isomorphism
The image of
![]() ${\mathcal {D}}\subset {\mathcal {D}(I)}$
by this series of isomorphisms is the tube domain in
${\mathcal {D}}\subset {\mathcal {D}(I)}$
by this series of isomorphisms is the tube domain in
![]() $L(I)_{{\mathbb {C}}}$
defined by
$L(I)_{{\mathbb {C}}}$
defined by
In this way, we obtain the tube domain realization
depending on the choice of
![]() $I'$
.
$I'$
.
If we change
![]() $I'$
, the base point is changed, and the tube domain realization (2.1) is shifted by the corresponding translation of
$I'$
, the base point is changed, and the tube domain realization (2.1) is shifted by the corresponding translation of
![]() $L(I)_{{\mathbb {C}}}$
. For a given
$L(I)_{{\mathbb {C}}}$
. For a given
![]() $I'$
, we can always find a (unique) isotropic line
$I'$
, we can always find a (unique) isotropic line
![]() $\ne I_{{\mathbb {Q}}}$
from the hyperbolic plane
$\ne I_{{\mathbb {Q}}}$
from the hyperbolic plane
![]() $\langle I, I'\rangle _{{\mathbb {Q}}}$
. (Explicitly, if we take vectors
$\langle I, I'\rangle _{{\mathbb {Q}}}$
. (Explicitly, if we take vectors
![]() $l\in I_{{\mathbb {Q}}}, l'\in I^{\prime }_{{\mathbb {Q}}}$
with
$l\in I_{{\mathbb {Q}}}, l'\in I^{\prime }_{{\mathbb {Q}}}$
with
![]() $(l, l')=1$
, the vector
$(l, l')=1$
, the vector
![]() $l'-2^{-1}(l', l')l$
generates this isotropic line.) This means that we can replace the given
$l'-2^{-1}(l', l')l$
generates this isotropic line.) This means that we can replace the given
![]() $I'$
to be isotropic without changing the base point. When
$I'$
to be isotropic without changing the base point. When
![]() $I'$
is isotropic, the inverse of (2.1) is given by
$I'$
is isotropic, the inverse of (2.1) is given by
where
![]() $z\in (I^{\perp }/I)_{{\mathbb {C}}}$
,
$z\in (I^{\perp }/I)_{{\mathbb {C}}}$
,
![]() $l\in I$
, and
$l\in I$
, and
![]() $l'\in I^{\prime }_{{\mathbb {Q}}}$
with
$l'\in I^{\prime }_{{\mathbb {Q}}}$
with
![]() $(l, l')=1$
, and we identify
$(l, l')=1$
, and we identify
![]() $(I^{\perp }/I)_{{\mathbb {C}}}\simeq \langle I, I' \rangle _{{\mathbb {C}}}^{\perp }\subset L_{{\mathbb {C}}}$
in the right side.
$(I^{\perp }/I)_{{\mathbb {C}}}\simeq \langle I, I' \rangle _{{\mathbb {C}}}^{\perp }\subset L_{{\mathbb {C}}}$
in the right side.
2.2 Stabilizer over
 ${\mathbb {Q}}$
${\mathbb {Q}}$
Let
![]() ${\Gamma (I)_{\mathbb {Q}}}$
be the stabilizer of I in
${\Gamma (I)_{\mathbb {Q}}}$
be the stabilizer of I in
![]() $\mathrm {O}^{+}(L_{{\mathbb {Q}}})$
. Note that we are not considering the stabilizer of
$\mathrm {O}^{+}(L_{{\mathbb {Q}}})$
. Note that we are not considering the stabilizer of
![]() $I_{{\mathbb {Q}}}$
, but of I. This is not restrictive when restricting to subgroups of
$I_{{\mathbb {Q}}}$
, but of I. This is not restrictive when restricting to subgroups of
![]() ${\mathrm {O}^{+}(L)}$
. We put
${\mathrm {O}^{+}(L)}$
. We put
This is the unipotent radical of
![]() ${\Gamma (I)_{\mathbb {Q}}}$
and can be explicitly described as follows. For a vector
${\Gamma (I)_{\mathbb {Q}}}$
and can be explicitly described as follows. For a vector
![]() $m\otimes l$
of
$m\otimes l$
of
![]() $L(I)_{{\mathbb {Q}}}$
, the Eichler transvection
$L(I)_{{\mathbb {Q}}}$
, the Eichler transvection
![]() $E_{m\otimes l}\in {\Gamma (I)_{\mathbb {Q}}}$
is defined by (cf. [Reference Gritsenko, Hulek and Sankaran9, Reference Scattone17])
$E_{m\otimes l}\in {\Gamma (I)_{\mathbb {Q}}}$
is defined by (cf. [Reference Gritsenko, Hulek and Sankaran9, Reference Scattone17])
where
![]() $\tilde {m}\in I^{\perp }_{{\mathbb {Q}}}=I^{\perp }_{{\mathbb {Q}}}\cap L_{{\mathbb {Q}}}$
is an arbitrary lift of
$\tilde {m}\in I^{\perp }_{{\mathbb {Q}}}=I^{\perp }_{{\mathbb {Q}}}\cap L_{{\mathbb {Q}}}$
is an arbitrary lift of
![]() $m\in (I^{\perp }/I)_{{\mathbb {Q}}}$
. This does not depend on the choice of
$m\in (I^{\perp }/I)_{{\mathbb {Q}}}$
. This does not depend on the choice of
![]() $\tilde {m}$
. In particular,
$\tilde {m}$
. In particular,
![]() $E_{m\otimes l}(v) = v - (m, v)l$
when
$E_{m\otimes l}(v) = v - (m, v)l$
when
![]() $v\in I^{\perp }_{{\mathbb {Q}}}$
. We have
$v\in I^{\perp }_{{\mathbb {Q}}}$
. We have
![]() $E_{w}\circ E_{w'}=E_{w+w'}$
for
$E_{w}\circ E_{w'}=E_{w+w'}$
for
![]() $w, w'\in L(I)_{{\mathbb {Q}}}$
. Then we have the canonical isomorphism
$w, w'\in L(I)_{{\mathbb {Q}}}$
. Then we have the canonical isomorphism
We identify
![]() ${U(I)_{\mathbb {Q}}}$
with
${U(I)_{\mathbb {Q}}}$
with
![]() $L(I)_{{\mathbb {Q}}}$
in this way. We also identify
$L(I)_{{\mathbb {Q}}}$
in this way. We also identify
![]() $\textrm {O}(I_{{\mathbb {Q}}}^{\perp }/I_{{\mathbb {Q}}})\times \mathrm {GL}(I)$
with
$\textrm {O}(I_{{\mathbb {Q}}}^{\perp }/I_{{\mathbb {Q}}})\times \mathrm {GL}(I)$
with
![]() $\textrm {O}(L(I)_{{\mathbb {Q}}})\times \mathrm {GL}(I)$
by the canonical twisted isomorphism
$\textrm {O}(L(I)_{{\mathbb {Q}}})\times \mathrm {GL}(I)$
by the canonical twisted isomorphism
We thus have the canonical exact sequence
If we choose a lift
![]() $(I^{\perp }/I)_{{\mathbb {Q}}}\hookrightarrow I^{\perp }_{{\mathbb {Q}}}$
of
$(I^{\perp }/I)_{{\mathbb {Q}}}\hookrightarrow I^{\perp }_{{\mathbb {Q}}}$
of
![]() $(I^{\perp }/I)_{{\mathbb {Q}}}$
, or equivalently, a rank
$(I^{\perp }/I)_{{\mathbb {Q}}}$
, or equivalently, a rank
![]() $1$
sublattice
$1$
sublattice
![]() $I'$
of L with
$I'$
of L with
![]() $(I, I')\not \equiv 0$
, the exact sequence (2.3) splits:
$(I, I')\not \equiv 0$
, the exact sequence (2.3) splits:
Here the lifted group
![]() $\mathrm {O}^{+}(L(I)_{{\mathbb {Q}}})$
acts on the lifted component
$\mathrm {O}^{+}(L(I)_{{\mathbb {Q}}})$
acts on the lifted component
![]() $(I^{\perp }/I)_{{\mathbb {Q}}}\subset L_{{\mathbb {Q}}}$
through the natural isomorphism
$(I^{\perp }/I)_{{\mathbb {Q}}}\subset L_{{\mathbb {Q}}}$
through the natural isomorphism
![]() $\mathrm {O}(L(I)_{{\mathbb {Q}}})\simeq \mathrm { O}((I^{\perp }/I)_{{\mathbb {Q}}})$
, and
$\mathrm {O}(L(I)_{{\mathbb {Q}}})\simeq \mathrm { O}((I^{\perp }/I)_{{\mathbb {Q}}})$
, and
![]() $\mathrm {GL}(I)$
corresponds to
$\mathrm {GL}(I)$
corresponds to
![]() $\{ \pm \mathrm {id}_{L} \}$
. Since
$\{ \pm \mathrm {id}_{L} \}$
. Since
![]() $\gamma \circ E_{w} \circ \gamma ^{-1} =E_{\gamma w}$
for
$\gamma \circ E_{w} \circ \gamma ^{-1} =E_{\gamma w}$
for
![]() $\gamma \in {\Gamma (I)_{\mathbb {Q}}}$
, the adjoint action of
$\gamma \in {\Gamma (I)_{\mathbb {Q}}}$
, the adjoint action of
![]() ${\Gamma (I)_{\mathbb {Q}}}$
on
${\Gamma (I)_{\mathbb {Q}}}$
on
![]() ${U(I)_{\mathbb {Q}}}$
coincides with the natural action of
${U(I)_{\mathbb {Q}}}$
coincides with the natural action of
![]() ${\Gamma (I)_{\mathbb {Q}}}$
on
${\Gamma (I)_{\mathbb {Q}}}$
on
![]() $L(I)_{{\mathbb {Q}}}$
. Therefore, in the induced action of
$L(I)_{{\mathbb {Q}}}$
. Therefore, in the induced action of
![]() ${\mathrm {O}^{+}}(L(I)_{{\mathbb {Q}}})\times \mathrm {GL}(I)$
on
${\mathrm {O}^{+}}(L(I)_{{\mathbb {Q}}})\times \mathrm {GL}(I)$
on
![]() ${U(I)_{\mathbb {Q}}}$
,
${U(I)_{\mathbb {Q}}}$
,
![]() $\mathrm {GL}(I)=\{ \pm 1 \}$
acts trivially, and
$\mathrm {GL}(I)=\{ \pm 1 \}$
acts trivially, and
![]() ${\mathrm {O}^{+}}(L(I)_{{\mathbb {Q}}})$
acts by its natural action on
${\mathrm {O}^{+}}(L(I)_{{\mathbb {Q}}})$
acts by its natural action on
![]() $L(I)_{{\mathbb {Q}}}$
.
$L(I)_{{\mathbb {Q}}}$
.
We take the tube domain realization
![]() ${\mathcal {D}}\to {\mathcal {D}}_{I}$
associated with (the same)
${\mathcal {D}}\to {\mathcal {D}}_{I}$
associated with (the same)
![]() $I'$
. Then the action of
$I'$
. Then the action of
![]() ${\Gamma (I)_{\mathbb {Q}}}$
on
${\Gamma (I)_{\mathbb {Q}}}$
on
![]() ${\mathcal {D}}$
is translated to the action of the right side of (2.4) on
${\mathcal {D}}$
is translated to the action of the right side of (2.4) on
![]() ${\mathcal {D}}_{I}$
. This is described as follows.
${\mathcal {D}}_{I}$
. This is described as follows.
Lemma 2.1 In the action of the right side of (2.4) on
![]() ${\mathcal {D}}_{I}$
,
${\mathcal {D}}_{I}$
,
-
(1)
 $E_{w}\in {U(I)_{\mathbb {Q}}}$
acts on
$E_{w}\in {U(I)_{\mathbb {Q}}}$
acts on
 ${\mathcal {D}}_{I}$
as the translation by
${\mathcal {D}}_{I}$
as the translation by
 $w\in L(I)_{{\mathbb {Q}}}$
on
$w\in L(I)_{{\mathbb {Q}}}$
on
 $L(I)_{{\mathbb {C}}}$
;
$L(I)_{{\mathbb {C}}}$
; -
(2)
 $\mathrm {O}^{+}(L(I)_{{\mathbb {Q}}})$
acts on
$\mathrm {O}^{+}(L(I)_{{\mathbb {Q}}})$
acts on
 ${\mathcal {D}}_{I}$
by its linear action on
${\mathcal {D}}_{I}$
by its linear action on
 $L(I)_{{\mathbb {C}}}$
;
$L(I)_{{\mathbb {C}}}$
; -
(3)
 $\mathrm {GL}(I)=\{ \pm 1 \}$
acts on
$\mathrm {GL}(I)=\{ \pm 1 \}$
acts on
 ${\mathcal {D}}_{I}$
trivially.
${\mathcal {D}}_{I}$
trivially.
Proof This can be seen from direct calculation using (2.2).
2.3 Stabilizer over
 ${\mathbb {Z}}$
${\mathbb {Z}}$
Now let
![]() ${\Gamma }$
be a subgroup of
${\Gamma }$
be a subgroup of
![]() ${\mathrm {O}^{+}(L)}$
of finite index. We write
${\mathrm {O}^{+}(L)}$
of finite index. We write
Then
![]() ${U(I)_{\mathbb {Z}}}$
is a lattice on
${U(I)_{\mathbb {Z}}}$
is a lattice on
![]() ${U(I)_{\mathbb {Q}}}$
. By definition, we have the exact sequence
${U(I)_{\mathbb {Q}}}$
. By definition, we have the exact sequence
Although (2.3) splits, this does not mean that (2.5) splits. We write
![]() ${U(I)_{\mathbb {Q}/\mathbb {Z}}}={U(I)_{\mathbb {Q}}}/{U(I)_{\mathbb {Z}}}$
. This is the group of torsion points of the algebraic torus
${U(I)_{\mathbb {Q}/\mathbb {Z}}}={U(I)_{\mathbb {Q}}}/{U(I)_{\mathbb {Z}}}$
. This is the group of torsion points of the algebraic torus
![]() $T(I)=U(I)_{{\mathbb {C}}}/{U(I)_{\mathbb {Z}}}$
. We also put
$T(I)=U(I)_{{\mathbb {C}}}/{U(I)_{\mathbb {Z}}}$
. We also put
which makes sense because
![]() ${U(I)_{\mathbb {Z}}}$
is normal in
${U(I)_{\mathbb {Z}}}$
is normal in
![]() $\pi ^{-1}(\mathrm {O}^{+}({U(I)_{\mathbb {Z}}})\times \mathrm {GL}(I))$
by definition. This group has the canonical exact sequence
$\pi ^{-1}(\mathrm {O}^{+}({U(I)_{\mathbb {Z}}})\times \mathrm {GL}(I))$
by definition. This group has the canonical exact sequence
Then
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}$
is a subgroup of
${\overline {\Gamma (I)}_{\mathbb {Z}}}$
is a subgroup of
![]() ${\overline {\Gamma (I)}_{\mathbb {Q}}}$
naturally. We have
${\overline {\Gamma (I)}_{\mathbb {Q}}}$
naturally. We have
by the definition
![]() ${U(I)_{\mathbb {Z}}}={\Gamma (I)_{\mathbb {Z}}}\cap {U(I)_{\mathbb {Q}}}$
of
${U(I)_{\mathbb {Z}}}={\Gamma (I)_{\mathbb {Z}}}\cap {U(I)_{\mathbb {Q}}}$
of
![]() ${U(I)_{\mathbb {Z}}}$
.
${U(I)_{\mathbb {Z}}}$
.
We choose a rank
![]() $1$
sublattice
$1$
sublattice
![]() $I'\subset L$
with
$I'\subset L$
with
![]() $(I, I')\not \equiv 0$
and accordingly take a tube domain realization of
$(I, I')\not \equiv 0$
and accordingly take a tube domain realization of
![]() ${\mathcal {D}}$
and a splitting of
${\mathcal {D}}$
and a splitting of
![]() ${\Gamma (I)_{\mathbb {Q}}}$
. Dividing by
${\Gamma (I)_{\mathbb {Q}}}$
. Dividing by
![]() ${U(I)_{\mathbb {Z}}}$
and writing
${U(I)_{\mathbb {Z}}}$
and writing
![]() ${\mathcal {X}(I)}={\mathcal {D}}/{U(I)_{\mathbb {Z}}}$
, we obtain isomorphisms
${\mathcal {X}(I)}={\mathcal {D}}/{U(I)_{\mathbb {Z}}}$
, we obtain isomorphisms
both depending on the choice of
![]() $I'$
. By Lemma 2.1, the natural action of
$I'$
. By Lemma 2.1, the natural action of
![]() ${\overline {\Gamma (I)}_{\mathbb {Q}}}$
on
${\overline {\Gamma (I)}_{\mathbb {Q}}}$
on
![]() ${\mathcal {X}(I)}$
is translated to the standard action of
${\mathcal {X}(I)}$
is translated to the standard action of
![]() $(\mathrm {O}^{+}({U(I)_{\mathbb {Z}}})\times \mathrm {GL}(I))\ltimes {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
on
$(\mathrm {O}^{+}({U(I)_{\mathbb {Z}}})\times \mathrm {GL}(I))\ltimes {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
on
![]() $T(I)$
. Here
$T(I)$
. Here
![]() $\mathrm {O}^{+}({U(I)_{\mathbb {Z}}})$
acts by torus automorphisms fixing the identity,
$\mathrm {O}^{+}({U(I)_{\mathbb {Z}}})$
acts by torus automorphisms fixing the identity,
![]() $\mathrm {GL}(I)$
acts trivially, and
$\mathrm {GL}(I)$
acts trivially, and
![]() ${U(I)_{\mathbb {Q}/\mathbb {Z}}}$
acts by translation.
${U(I)_{\mathbb {Q}/\mathbb {Z}}}$
acts by translation.
By (2.7), the projection
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}\to \mathrm {O}^{+}({U(I)_{\mathbb {Z}}})\times \mathrm {GL}(I)$
is injective. But this does not mean that
${\overline {\Gamma (I)}_{\mathbb {Z}}}\to \mathrm {O}^{+}({U(I)_{\mathbb {Z}}})\times \mathrm {GL}(I)$
is injective. But this does not mean that
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}$
as a subgroup of
${\overline {\Gamma (I)}_{\mathbb {Z}}}$
as a subgroup of
![]() ${\overline {\Gamma (I)}_{\mathbb {Q}}}$
is contained in the lifted subgroup
${\overline {\Gamma (I)}_{\mathbb {Q}}}$
is contained in the lifted subgroup
![]() $\mathrm {O}^{+}({U(I)_{\mathbb {Z}}})\times \mathrm {GL}(I)$
in (2.8). Thus, the action of
$\mathrm {O}^{+}({U(I)_{\mathbb {Z}}})\times \mathrm {GL}(I)$
in (2.8). Thus, the action of
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}$
on
${\overline {\Gamma (I)}_{\mathbb {Z}}}$
on
![]() ${\mathcal {X}(I)}$
may have translation component.
${\mathcal {X}(I)}$
may have translation component.
Remark 2.2 Let
![]() $I={\mathbb {Z}}l$
and
$I={\mathbb {Z}}l$
and
![]() $\Gamma (l)_{{\mathbb {Z}}}<{\Gamma (I)_{\mathbb {Z}}}$
be the kernel of
$\Gamma (l)_{{\mathbb {Z}}}<{\Gamma (I)_{\mathbb {Z}}}$
be the kernel of
![]() ${\Gamma (I)_{\mathbb {Z}}}\to \mathrm {GL}(I)$
. In the case
${\Gamma (I)_{\mathbb {Z}}}\to \mathrm {GL}(I)$
. In the case
![]() $-{\mathrm {id}}\in {\Gamma }$
, we have
$-{\mathrm {id}}\in {\Gamma }$
, we have
![]() ${\Gamma (I)_{\mathbb {Z}}}=\Gamma (l)_{{\mathbb {Z}}}\times \{ \pm {\mathrm {id}} \}$
, so we may replace
${\Gamma (I)_{\mathbb {Z}}}=\Gamma (l)_{{\mathbb {Z}}}\times \{ \pm {\mathrm {id}} \}$
, so we may replace
![]() ${\Gamma (I)_{\mathbb {Z}}}$
by
${\Gamma (I)_{\mathbb {Z}}}$
by
![]() $\Gamma (l)_{{\mathbb {Z}}}$
when considering action on
$\Gamma (l)_{{\mathbb {Z}}}$
when considering action on
![]() ${\mathcal {D}}$
, as was done in [Reference Ma13, Appendix]. (The last sentence of [Reference Ma13, Remark A.8] for
${\mathcal {D}}$
, as was done in [Reference Ma13, Appendix]. (The last sentence of [Reference Ma13, Remark A.8] for
![]() ${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
should be understood under the condition
${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
should be understood under the condition
![]() $\Gamma (l)_{{\mathbb {Z}}}={\Gamma (I)_{\mathbb {Z}}}$
(e.g.,
$\Gamma (l)_{{\mathbb {Z}}}={\Gamma (I)_{\mathbb {Z}}}$
(e.g.,
![]() $\mathrm {div}(I)>2$
) or
$\mathrm {div}(I)>2$
) or
![]() $A_{L}$
2-elementary, or
$A_{L}$
2-elementary, or
![]() $\mathrm {div}(I)=1$
.)
$\mathrm {div}(I)=1$
.)
2.4 Partial toroidal compactification
We recall partial toroidal compactification of
![]() ${\mathcal {X}(I)}={\mathcal {D}}/{U(I)_{\mathbb {Z}}}$
following [Reference Ash, Mumford, Rapoport and Tai1]. We put a
${\mathcal {X}(I)}={\mathcal {D}}/{U(I)_{\mathbb {Z}}}$
following [Reference Ash, Mumford, Rapoport and Tai1]. We put a
![]() ${\mathbb {Q}}$
-structure on
${\mathbb {Q}}$
-structure on
![]() $U(I)_{{\mathbb {R}}}$
by
$U(I)_{{\mathbb {R}}}$
by
![]() ${U(I)_{\mathbb {Q}}}\simeq L(I)_{{\mathbb {Q}}}$
. We write
${U(I)_{\mathbb {Q}}}\simeq L(I)_{{\mathbb {Q}}}$
. We write
![]() ${\mathcal {C}_{I}^{+}}={\mathcal {C}_{I}}\cup \bigcup _{w}{\mathbb {R}}_{\geq 0}w$
, where w ranges over all isotropic vectors of
${\mathcal {C}_{I}^{+}}={\mathcal {C}_{I}}\cup \bigcup _{w}{\mathbb {R}}_{\geq 0}w$
, where w ranges over all isotropic vectors of
![]() $L(I)_{{\mathbb {Q}}}$
in the closure of
$L(I)_{{\mathbb {Q}}}$
in the closure of
![]() ${\mathcal {C}_{I}}$
. A rational polyhedral cone decomposition (fan)
${\mathcal {C}_{I}}$
. A rational polyhedral cone decomposition (fan)
![]() $\Sigma =(\sigma _{\alpha })_{\alpha }$
in
$\Sigma =(\sigma _{\alpha })_{\alpha }$
in
![]() $U(I)_{{\mathbb {R}}}$
is called
$U(I)_{{\mathbb {R}}}$
is called
![]() ${\Gamma (I)_{\mathbb {Z}}}$
-admissible [Reference Ash, Mumford, Rapoport and Tai1] if the support of
${\Gamma (I)_{\mathbb {Z}}}$
-admissible [Reference Ash, Mumford, Rapoport and Tai1] if the support of
![]() $\Sigma $
is
$\Sigma $
is
![]() ${\mathcal {C}_{I}^{+}}$
,
${\mathcal {C}_{I}^{+}}$
,
![]() $\Sigma $
is preserved under the adjoint (= natural) action of
$\Sigma $
is preserved under the adjoint (= natural) action of
![]() ${\Gamma (I)_{\mathbb {Z}}}$
on
${\Gamma (I)_{\mathbb {Z}}}$
on
![]() $U(I)_{{\mathbb {R}}}=L(I)_{{\mathbb {R}}}$
, and there are only finitely many cones up to the action of
$U(I)_{{\mathbb {R}}}=L(I)_{{\mathbb {R}}}$
, and there are only finitely many cones up to the action of
![]() ${\Gamma (I)_{\mathbb {Z}}}$
. Isotropic rays in
${\Gamma (I)_{\mathbb {Z}}}$
. Isotropic rays in
![]() $\Sigma $
correspond to rational isotropic lines in
$\Sigma $
correspond to rational isotropic lines in
![]() $L(I)_{{\mathbb {Q}}}$
(hence independent of
$L(I)_{{\mathbb {Q}}}$
(hence independent of
![]() $\Sigma $
), which in turn correspond to rank
$\Sigma $
), which in turn correspond to rank
![]() $2$
primitive isotropic sublattices J of L containing I.
$2$
primitive isotropic sublattices J of L containing I.
The fan
![]() $\Sigma $
defines a torus embedding
$\Sigma $
defines a torus embedding
![]() $T(I)\hookrightarrow T(I)^{\Sigma }$
of the torus
$T(I)\hookrightarrow T(I)^{\Sigma }$
of the torus
![]() $T(I)=U(I)_{{\mathbb {C}}}/{U(I)_{\mathbb {Z}}}$
. Each ray
$T(I)=U(I)_{{\mathbb {C}}}/{U(I)_{\mathbb {Z}}}$
. Each ray
![]() $\sigma $
of
$\sigma $
of
![]() $\Sigma $
defines a sub-torus embedding
$\Sigma $
defines a sub-torus embedding
![]() $T(I)\hookrightarrow T(I)^{\sigma }\subset T(I)^{\Sigma }$
, isomorphic to
$T(I)\hookrightarrow T(I)^{\sigma }\subset T(I)^{\Sigma }$
, isomorphic to
![]() $({\mathbb {C}}^{\times })^{b} \hookrightarrow {\mathbb {C}}\times ({\mathbb {C}}^{\times })^{b-1}$
, whose unique boundary divisor is the quotient torus defined by the quotient lattice
$({\mathbb {C}}^{\times })^{b} \hookrightarrow {\mathbb {C}}\times ({\mathbb {C}}^{\times })^{b-1}$
, whose unique boundary divisor is the quotient torus defined by the quotient lattice
![]() ${U(I)_{\mathbb {Z}}}/({\mathbb {R}}\sigma \cap {U(I)_{\mathbb {Z}}})$
. The character group of this boundary torus is
${U(I)_{\mathbb {Z}}}/({\mathbb {R}}\sigma \cap {U(I)_{\mathbb {Z}}})$
. The character group of this boundary torus is
![]() $\sigma ^{\perp }\cap U(I)_{{\mathbb {Z}}}^{\vee }$
. Here we regard
$\sigma ^{\perp }\cap U(I)_{{\mathbb {Z}}}^{\vee }$
. Here we regard
![]() $U(I)_{{\mathbb {Z}}}^{\vee }$
as a lattice on
$U(I)_{{\mathbb {Z}}}^{\vee }$
as a lattice on
![]() ${U(I)_{\mathbb {Q}}}$
by the quadratic form on
${U(I)_{\mathbb {Q}}}$
by the quadratic form on
![]() ${U(I)_{\mathbb {Q}}}=L(I)_{{\mathbb {Q}}}$
, which gives the pairing between
${U(I)_{\mathbb {Q}}}=L(I)_{{\mathbb {Q}}}$
, which gives the pairing between
![]() ${U(I)_{\mathbb {Z}}}$
and
${U(I)_{\mathbb {Z}}}$
and
![]() $U(I)_{{\mathbb {Z}}}^{\vee }$
.
$U(I)_{{\mathbb {Z}}}^{\vee }$
.
We take a tube domain realization of
![]() ${\mathcal {D}}$
by choosing
${\mathcal {D}}$
by choosing
![]() $I'\subset L$
with
$I'\subset L$
with
![]() $(I, I')\not \equiv 0$
. Then let
$(I, I')\not \equiv 0$
. Then let
![]() ${\mathcal {X}(I)^{\Sigma }}$
be the interior of the closure of
${\mathcal {X}(I)^{\Sigma }}$
be the interior of the closure of
![]() ${\mathcal {X}(I)}\simeq \mathcal {D}_{I}/{U(I)_{\mathbb {Z}}}$
in
${\mathcal {X}(I)}\simeq \mathcal {D}_{I}/{U(I)_{\mathbb {Z}}}$
in
![]() $T(I)^{\Sigma }$
. This embedding
$T(I)^{\Sigma }$
. This embedding
![]() ${\mathcal {X}(I)}\hookrightarrow {\mathcal {X}(I)^{\Sigma }}$
is the partial toroidal compactification over I defined by the fan
${\mathcal {X}(I)}\hookrightarrow {\mathcal {X}(I)^{\Sigma }}$
is the partial toroidal compactification over I defined by the fan
![]() $\Sigma $
. It is
$\Sigma $
. It is
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}$
-equivariant, and does not depend on the choice of
${\overline {\Gamma (I)}_{\mathbb {Z}}}$
-equivariant, and does not depend on the choice of
![]() $I'$
. We can think of
$I'$
. We can think of
![]() ${\mathcal {X}(I)^{\Sigma }}$
as giving a local chart for the boundary points of a full toroidal compactification lying over the I-cusp (see Section 7), like
${\mathcal {X}(I)^{\Sigma }}$
as giving a local chart for the boundary points of a full toroidal compactification lying over the I-cusp (see Section 7), like
![]() ${\mathcal {D}}$
gives a local chart for the interior points in
${\mathcal {D}}$
gives a local chart for the interior points in
![]() ${\Gamma }\backslash {\mathcal {D}}$
.
${\Gamma }\backslash {\mathcal {D}}$
.
3 Irregular zero-dimensional cusps
We now study irregular zero-dimensional cusps. Let
![]() ${\Gamma }$
be a finite-index subgroup of
${\Gamma }$
be a finite-index subgroup of
![]() ${\mathrm {O}^{+}(L)}$
, and let I be a rank
${\mathrm {O}^{+}(L)}$
, and let I be a rank
![]() $1$
primitive isotropic sublattice of L. We keep the notation from Section 2. We will define irregularity in two stages: irregularity of a cusp (Section 3.1), and irregularity of a toroidal boundary divisor over (or adjacent to) an irregular cusp (Section 3.2). The first stage is concerned only with
$1$
primitive isotropic sublattice of L. We keep the notation from Section 2. We will define irregularity in two stages: irregularity of a cusp (Section 3.1), and irregularity of a toroidal boundary divisor over (or adjacent to) an irregular cusp (Section 3.2). The first stage is concerned only with
![]() ${\Gamma }$
, but the second stage is also involved with a
${\Gamma }$
, but the second stage is also involved with a
![]() ${\Gamma (I)_{\mathbb {Z}}}$
-admissible fan.
${\Gamma (I)_{\mathbb {Z}}}$
-admissible fan.
3.1 Irregularity
We give several equivalent definitions of irregularity of a zero-dimensional cusp in the following form.
Proposition 3.1 The following conditions are equivalent.
-
(1)
 ${U(I)_{\mathbb {Z}}}\ne {U(I)_{\mathbb {Z}}^{\prime }}$
where
${U(I)_{\mathbb {Z}}}\ne {U(I)_{\mathbb {Z}}^{\prime }}$
where
 ${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
.
${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
. -
(2)
 $-{\mathrm {id}}\not \in {\Gamma }$
and
$-{\mathrm {id}}\not \in {\Gamma }$
and
 $-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
for some
$-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
for some
 $w\in L(I)_{{\mathbb {Q}}}$
.
$w\in L(I)_{{\mathbb {Q}}}$
. -
(3)
 $-{\mathrm {id}}\not \in {\Gamma }$
and
$-{\mathrm {id}}\not \in {\Gamma }$
and
 ${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm { O}^{+}}({U(I)_{\mathbb {Z}}})$
is not injective.
${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm { O}^{+}}({U(I)_{\mathbb {Z}}})$
is not injective. -
(4)
 ${\overline {\Gamma (I)}_{\mathbb {Z}}}$
contains an element which acts by a nonzero translation on
${\overline {\Gamma (I)}_{\mathbb {Z}}}$
contains an element which acts by a nonzero translation on
 ${{\mathcal {X}(I)}={\mathcal {D}}/{U(I)_{\mathbb {Z}}}}$
.
${{\mathcal {X}(I)}={\mathcal {D}}/{U(I)_{\mathbb {Z}}}}$
.
When these hold, we have
![]() ${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}} = \langle E_{w} \rangle \simeq {\mathbb {Z}}/2$
and
${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}} = \langle E_{w} \rangle \simeq {\mathbb {Z}}/2$
and
and the translation in
![]() $(4)$
is given by
$(4)$
is given by
![]() $[w]\in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
and is unique.
$[w]\in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
and is unique.
Definition 3.1 We say that the zero-dimensional cusp I is irregular for
![]() ${\Gamma }$
when these properties hold, and regular otherwise.
${\Gamma }$
when these properties hold, and regular otherwise.
Proof
![]() $(1) \Rightarrow (2)$
: Since
$(1) \Rightarrow (2)$
: Since
![]() ${\Gamma }\ne {\langle \Gamma , -\mathrm {id} \rangle }$
, we have
${\Gamma }\ne {\langle \Gamma , -\mathrm {id} \rangle }$
, we have
![]() $-{\mathrm {id}}\not \in {\Gamma }$
. Let
$-{\mathrm {id}}\not \in {\Gamma }$
. Let
![]() $E_{w}\in {U(I)_{\mathbb {Z}}^{\prime }}$
, but
$E_{w}\in {U(I)_{\mathbb {Z}}^{\prime }}$
, but
![]() $E_{w}\not \in {U(I)_{\mathbb {Z}}}$
. Since
$E_{w}\not \in {U(I)_{\mathbb {Z}}}$
. Since
![]() ${\langle \Gamma , -\mathrm {id} \rangle }={\Gamma }\sqcup -{\Gamma }$
, we have
${\langle \Gamma , -\mathrm {id} \rangle }={\Gamma }\sqcup -{\Gamma }$
, we have
![]() $E_{w}\in -{\Gamma }$
, and so
$E_{w}\in -{\Gamma }$
, and so
![]() $-E_{w}\in {\Gamma }$
. Note that
$-E_{w}\in {\Gamma }$
. Note that
![]() ${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}\simeq {\langle \Gamma , -\mathrm {id} \rangle }/{\Gamma }$
is of order
${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}\simeq {\langle \Gamma , -\mathrm {id} \rangle }/{\Gamma }$
is of order
![]() $2$
, and so
$2$
, and so
![]() ${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}} = \langle E_{w} \rangle $
.
${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}} = \langle E_{w} \rangle $
.
![]() $(2) \Rightarrow (1)$
: If
$(2) \Rightarrow (1)$
: If
![]() $-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
and
$-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
and
![]() $-{\mathrm {id}}\not \in {\Gamma }$
, then
$-{\mathrm {id}}\not \in {\Gamma }$
, then
![]() $E_{w}\not \in {U(I)_{\mathbb {Z}}}$
, but
$E_{w}\not \in {U(I)_{\mathbb {Z}}}$
, but
![]() $E_{w}\in {U(I)_{\mathbb {Z}}^{\prime }}$
.
$E_{w}\in {U(I)_{\mathbb {Z}}^{\prime }}$
.
![]() $(2) \Rightarrow (3)$
: Since
$(2) \Rightarrow (3)$
: Since
![]() $-E_{w}$
acts on
$-E_{w}$
acts on
![]() $L(I)_{{\mathbb {Q}}}={U(I)_{\mathbb {Q}}}$
trivially, its image in
$L(I)_{{\mathbb {Q}}}={U(I)_{\mathbb {Q}}}$
trivially, its image in
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}$
is contained in the kernel of
${\overline {\Gamma (I)}_{\mathbb {Z}}}$
is contained in the kernel of
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
.
${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
.
![]() $(3) \Rightarrow (2)$
: Recall from (2.6) that the kernel of
$(3) \Rightarrow (2)$
: Recall from (2.6) that the kernel of
![]() ${\overline {\Gamma (I)}_{\mathbb {Q}}}\to {\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
is
${\overline {\Gamma (I)}_{\mathbb {Q}}}\to {\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
is
Since
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}\cap {U(I)_{\mathbb {Q}/\mathbb {Z}}} = \{ 0 \}$
by (2.7), a nontrivial element of the kernel of
${\overline {\Gamma (I)}_{\mathbb {Z}}}\cap {U(I)_{\mathbb {Q}/\mathbb {Z}}} = \{ 0 \}$
by (2.7), a nontrivial element of the kernel of
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm { O}^{+}}({U(I)_{\mathbb {Z}}})$
must be contained in
${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm { O}^{+}}({U(I)_{\mathbb {Z}}})$
must be contained in
![]() $(-{\mathrm {id}})\cdot {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
, hence, is the image of
$(-{\mathrm {id}})\cdot {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
, hence, is the image of
![]() $-E_{w}$
for some
$-E_{w}$
for some
![]() $w\in L(I)_{{\mathbb {Q}}}$
. This also shows that the kernel is
$w\in L(I)_{{\mathbb {Q}}}$
. This also shows that the kernel is
![]() ${\mathbb {Z}}/2$
generated by
${\mathbb {Z}}/2$
generated by
![]() $-E_{w}$
.
$-E_{w}$
.
![]() $(2) \Rightarrow (4)$
: The element
$(2) \Rightarrow (4)$
: The element
![]() $-E_{w}$
of
$-E_{w}$
of
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}$
acts on
${\overline {\Gamma (I)}_{\mathbb {Z}}}$
acts on
![]() ${\mathcal {X}(I)}$
by the translation by
${\mathcal {X}(I)}$
by the translation by
![]() $[w] \in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
. Since
$[w] \in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
. Since
![]() $-{\mathrm {id}}\not \in {\Gamma }$
, we have
$-{\mathrm {id}}\not \in {\Gamma }$
, we have
![]() $E_{w}\not \in {U(I)_{\mathbb {Z}}}$
. This means that
$E_{w}\not \in {U(I)_{\mathbb {Z}}}$
. This means that
![]() $[w]\ne 0 \in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
.
$[w]\ne 0 \in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
.
![]() $(4) \Rightarrow (2), (3)$
: We choose a splitting of
$(4) \Rightarrow (2), (3)$
: We choose a splitting of
![]() ${\overline {\Gamma (I)}_{\mathbb {Q}}}$
as in (2.8) and express an element of
${\overline {\Gamma (I)}_{\mathbb {Q}}}$
as in (2.8) and express an element of
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}\subset {\overline {\Gamma (I)}_{\mathbb {Q}}}$
as
${\overline {\Gamma (I)}_{\mathbb {Z}}}\subset {\overline {\Gamma (I)}_{\mathbb {Q}}}$
as
![]() $\gamma =(\gamma _{1}, \gamma _{2}, [w])$
accordingly, where
$\gamma =(\gamma _{1}, \gamma _{2}, [w])$
accordingly, where
![]() $\gamma _{1}\in {\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
,
$\gamma _{1}\in {\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
,
![]() $\gamma _{2}\in \mathrm {GL}(I)$
and
$\gamma _{2}\in \mathrm {GL}(I)$
and
![]() $[w]\in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
. If
$[w]\in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
. If
![]() $\gamma $
acts on
$\gamma $
acts on
![]() ${\mathcal {X}(I)}$
by a nonzero translation, we must have
${\mathcal {X}(I)}$
by a nonzero translation, we must have
![]() $\gamma _{1}={\mathrm {id}}_{L(I)}$
and the translation is given by
$\gamma _{1}={\mathrm {id}}_{L(I)}$
and the translation is given by
![]() $[w]\in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
. Therefore,
$[w]\in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
. Therefore,
![]() $\gamma $
is contained in the kernel of the projection to
$\gamma $
is contained in the kernel of the projection to
![]() ${\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
. Since
${\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
. Since
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}\cap {U(I)_{\mathbb {Q}/\mathbb {Z}}} = \{ 0 \}$
by (2.7) and
${\overline {\Gamma (I)}_{\mathbb {Z}}}\cap {U(I)_{\mathbb {Q}/\mathbb {Z}}} = \{ 0 \}$
by (2.7) and
![]() $[w] \ne 0$
, we have
$[w] \ne 0$
, we have
![]() $\gamma _{2}=-{\mathrm {id}}_{I}$
. Thus,
$\gamma _{2}=-{\mathrm {id}}_{I}$
. Thus,
![]() $\gamma =-E_{w}$
. Finally, we have
$\gamma =-E_{w}$
. Finally, we have
![]() $-{\mathrm {id}}\not \in {\Gamma }$
, for otherwise
$-{\mathrm {id}}\not \in {\Gamma }$
, for otherwise
![]() $E_{w}=-\gamma $
would be contained in
$E_{w}=-\gamma $
would be contained in
![]() ${U(I)_{\mathbb {Z}}}$
and then
${U(I)_{\mathbb {Z}}}$
and then
![]() $[w]=0\in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
.
$[w]=0\in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
.
Remark 3.2 Let
![]() $\Gamma (l)_{{\mathbb {Z}}}<{\Gamma (I)_{\mathbb {Z}}}$
be as in Remark 2.2. By the condition (3), I is irregular if and only if
$\Gamma (l)_{{\mathbb {Z}}}<{\Gamma (I)_{\mathbb {Z}}}$
be as in Remark 2.2. By the condition (3), I is irregular if and only if
![]() $-{\mathrm {id}}\not \in {\Gamma }$
,
$-{\mathrm {id}}\not \in {\Gamma }$
,
![]() $\Gamma (l)_{{\mathbb {Z}}}\ne {\Gamma (I)_{\mathbb {Z}}}$
, and
$\Gamma (l)_{{\mathbb {Z}}}\ne {\Gamma (I)_{\mathbb {Z}}}$
, and
![]() $\Gamma (l)_{{\mathbb {Z}}}$
and
$\Gamma (l)_{{\mathbb {Z}}}$
and
![]() ${\Gamma (I)_{\mathbb {Z}}}$
have the same image in
${\Gamma (I)_{\mathbb {Z}}}$
have the same image in
![]() ${\mathrm { O}^{+}}({U(I)_{\mathbb {Z}}})$
. We do not use this characterization.
${\mathrm { O}^{+}}({U(I)_{\mathbb {Z}}})$
. We do not use this characterization.
The condition (2) is useful for explicit calculation (Section 4). We give some immediate consequences.
Corollary 3.3 The group
![]() ${\Gamma }$
has no irregular cusp when
${\Gamma }$
has no irregular cusp when
![]() $-{\mathrm {id}}\in {\Gamma }$
or when
$-{\mathrm {id}}\in {\Gamma }$
or when
![]() ${\Gamma }$
is neat or when
${\Gamma }$
is neat or when
![]() ${\Gamma }<\mathrm {SO}^{+}(L)$
with b odd.
${\Gamma }<\mathrm {SO}^{+}(L)$
with b odd.
Proof The case
![]() $-{\mathrm {id}}\in {\Gamma }$
is obvious. When
$-{\mathrm {id}}\in {\Gamma }$
is obvious. When
![]() ${\Gamma }$
is neat, the subquotient
${\Gamma }$
is neat, the subquotient
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}$
is torsion-free, so it does not contain an element of finite order like
${\overline {\Gamma (I)}_{\mathbb {Z}}}$
is torsion-free, so it does not contain an element of finite order like
![]() $-E_{w}$
. When b is odd,
$-E_{w}$
. When b is odd,
![]() $-E_{w}$
has determinant
$-E_{w}$
has determinant
![]() $(-1)^{b+2}=-1$
, so a subgroup of
$(-1)^{b+2}=-1$
, so a subgroup of
![]() $\mathrm {SO}^{+}(L)$
never contains such an element.
$\mathrm {SO}^{+}(L)$
never contains such an element.
Corollary 3.4 When b is even,
![]() ${\Gamma }$
has an irregular cusp if and only if
${\Gamma }$
has an irregular cusp if and only if
![]() ${\Gamma }\cap \mathrm {SO}^{+}(L)$
has an irregular cusp.
${\Gamma }\cap \mathrm {SO}^{+}(L)$
has an irregular cusp.
Proof When b is even, both
![]() $-{\mathrm {id}}$
and
$-{\mathrm {id}}$
and
![]() $-E_{w}$
are contained in
$-E_{w}$
are contained in
![]() $\mathrm {SO}^{+}(L)$
.
$\mathrm {SO}^{+}(L)$
.
Corollary 3.5 If
![]() ${\Gamma }$
has an irregular cusp, any
${\Gamma }$
has an irregular cusp, any
![]() $\Gamma ' < {\mathrm {O}^{+}(L)}$
with
$\Gamma ' < {\mathrm {O}^{+}(L)}$
with
![]() $\Gamma ' \supset {\Gamma }$
and
$\Gamma ' \supset {\Gamma }$
and
![]() $-{\mathrm {id}}\not \in \Gamma '$
has an irregular cusp. Equivalently, if
$-{\mathrm {id}}\not \in \Gamma '$
has an irregular cusp. Equivalently, if
![]() $-{\mathrm {id}}\not \in {\Gamma }$
and
$-{\mathrm {id}}\not \in {\Gamma }$
and
![]() ${\Gamma }$
has no irregular cusp, any subgroup of
${\Gamma }$
has no irregular cusp, any subgroup of
![]() ${\Gamma }$
of finite index has no irregular cusp.
${\Gamma }$
of finite index has no irregular cusp.
The lattice
![]() ${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
is the projection image of
${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
is the projection image of
in
![]() ${U(I)_{\mathbb {Q}}}$
. Thus,
${U(I)_{\mathbb {Q}}}$
. Thus,
![]() ${U(I)_{\mathbb {Z}}^{\prime }}$
is the lattice of translation in the
${U(I)_{\mathbb {Z}}^{\prime }}$
is the lattice of translation in the
![]() ${\Gamma (I)_{\mathbb {Z}}}$
-action on the tube domain model. We have
${\Gamma (I)_{\mathbb {Z}}}$
-action on the tube domain model. We have
 $$ \begin{align} U(I)_{{\mathbb{Z}}}^{\star}/{U(I)_{\mathbb{Z}}} = \begin{cases} \langle -{\mathrm{id}} \rangle \simeq {\mathbb{Z}}/2, & -{\mathrm{id}}\in {\Gamma}, \\ \{ 1 \}, & -{\mathrm{id}}\not\in {\Gamma}, I \; \textrm{regular}, \\ \langle -E_{w} \rangle \simeq {\mathbb{Z}}/2, & I \; \textrm{irregular}. \end{cases} \end{align} $$
$$ \begin{align} U(I)_{{\mathbb{Z}}}^{\star}/{U(I)_{\mathbb{Z}}} = \begin{cases} \langle -{\mathrm{id}} \rangle \simeq {\mathbb{Z}}/2, & -{\mathrm{id}}\in {\Gamma}, \\ \{ 1 \}, & -{\mathrm{id}}\not\in {\Gamma}, I \; \textrm{regular}, \\ \langle -E_{w} \rangle \simeq {\mathbb{Z}}/2, & I \; \textrm{irregular}. \end{cases} \end{align} $$
This gives yet another characterization of irregularity:
![]() $-{\mathrm {id}}\not \in {\Gamma }$
and
$-{\mathrm {id}}\not \in {\Gamma }$
and
![]() ${U(I)_{\mathbb {Z}}}\ne U(I)_{{\mathbb {Z}}}^{\star }$
.
${U(I)_{\mathbb {Z}}}\ne U(I)_{{\mathbb {Z}}}^{\star }$
.
As we will explain in Section 8.1,
![]() ${U(I)_{\mathbb {Z}}}$
is the lattice for Fourier expansion of
${U(I)_{\mathbb {Z}}}$
is the lattice for Fourier expansion of
![]() ${\Gamma }$
-modular forms around I. Thus, irregular zero-dimensional cusps are those cusps whose lattice of translation is larger than the lattice for Fourier expansion.
${\Gamma }$
-modular forms around I. Thus, irregular zero-dimensional cusps are those cusps whose lattice of translation is larger than the lattice for Fourier expansion.
Remark 3.6 In the case
![]() $b=1$
, we have an accidental isomorphism
$b=1$
, we have an accidental isomorphism
![]() $\mathrm {SO}^{+}(2, 1)\simeq \mathrm {PSL}(2, {\mathbb {R}})$
which induces an isomorphism between the type IV domain here and the upper half-plane. However,
$\mathrm {SO}^{+}(2, 1)\simeq \mathrm {PSL}(2, {\mathbb {R}})$
which induces an isomorphism between the type IV domain here and the upper half-plane. However,
![]() $\mathrm {O}^{+}(2, 1)=\mathrm {SO}^{+}(2, 1) \times \{ \pm \mathrm {id} \}$
and
$\mathrm {O}^{+}(2, 1)=\mathrm {SO}^{+}(2, 1) \times \{ \pm \mathrm {id} \}$
and
![]() $\mathrm {SL}(2, {\mathbb {R}})$
are different double covers of
$\mathrm {SL}(2, {\mathbb {R}})$
are different double covers of
![]() $\mathrm {SO}^{+}(2, 1)\simeq \mathrm {PSL}(2, {\mathbb {R}})$
. Therefore, although we have the perfect analogy
$\mathrm {SO}^{+}(2, 1)\simeq \mathrm {PSL}(2, {\mathbb {R}})$
. Therefore, although we have the perfect analogy
 $$ \begin{align*} {U(I)_{\mathbb{Z}}} \leftrightarrow \left\{ \begin{pmatrix} 1 & \ast \\ 0 & 1 \end{pmatrix} \in {\Gamma} \right\}, \end{align*} $$
$$ \begin{align*} {U(I)_{\mathbb{Z}}} \leftrightarrow \left\{ \begin{pmatrix} 1 & \ast \\ 0 & 1 \end{pmatrix} \in {\Gamma} \right\}, \end{align*} $$
 $$ \begin{align*} {U(I)_{\mathbb{Z}}^{\prime}} \leftrightarrow \left\{ \begin{pmatrix} 1 & \ast \\ 0 & 1 \end{pmatrix} \in \langle {\Gamma}, -1 \rangle \right\}, \end{align*} $$
$$ \begin{align*} {U(I)_{\mathbb{Z}}^{\prime}} \leftrightarrow \left\{ \begin{pmatrix} 1 & \ast \\ 0 & 1 \end{pmatrix} \in \langle {\Gamma}, -1 \rangle \right\}, \end{align*} $$
subgroups of
![]() $\mathrm {O}^{+}(2, 1)$
which have irregular cusps never correspond to subgroups of
$\mathrm {O}^{+}(2, 1)$
which have irregular cusps never correspond to subgroups of
![]() $\mathrm {SL}(2, {\mathbb {R}})$
which have irregular cusps (in the classical sense [Reference Diamond and Shurman3]): they live in different covers of
$\mathrm {SL}(2, {\mathbb {R}})$
which have irregular cusps (in the classical sense [Reference Diamond and Shurman3]): they live in different covers of
![]() $\mathrm {SO}^{+}(2, 1)\simeq \mathrm {PSL}(2, {\mathbb {R}})$
. Subgroups of
$\mathrm {SO}^{+}(2, 1)\simeq \mathrm {PSL}(2, {\mathbb {R}})$
. Subgroups of
![]() $\mathrm {SO}^{+}(2, 1)\simeq \mathrm {PSL}(2, {\mathbb {R}})$
have no irregular cusp anyway.
$\mathrm {SO}^{+}(2, 1)\simeq \mathrm {PSL}(2, {\mathbb {R}})$
have no irregular cusp anyway.
3.2 Irregular boundary divisors
Let
![]() $\Sigma =(\sigma _{\alpha })$
be a
$\Sigma =(\sigma _{\alpha })$
be a
![]() ${\Gamma (I)_{\mathbb {Z}}}$
-admissible fan in
${\Gamma (I)_{\mathbb {Z}}}$
-admissible fan in
![]() $U(I)_{{\mathbb {R}}}$
, and let
$U(I)_{{\mathbb {R}}}$
, and let
![]() ${\mathcal {X}(I)}\hookrightarrow {\mathcal {X}(I)^{\Sigma }}$
be the partial compactification defined in Section 2.4. For a ray
${\mathcal {X}(I)}\hookrightarrow {\mathcal {X}(I)^{\Sigma }}$
be the partial compactification defined in Section 2.4. For a ray
![]() $\sigma $
in
$\sigma $
in
![]() $\Sigma $
, we denote by
$\Sigma $
, we denote by
![]() $D(\sigma )\subset {\mathcal {X}(I)^{\Sigma }}$
the corresponding boundary divisor. When I is irregular, these boundary divisors are divided into two types as follows.
$D(\sigma )\subset {\mathcal {X}(I)^{\Sigma }}$
the corresponding boundary divisor. When I is irregular, these boundary divisors are divided into two types as follows.
Proposition 3.7 Let I be an irregular zero-dimensional cusp for
![]() ${\Gamma }$
. Let
${\Gamma }$
. Let
![]() $-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
. The following conditions for a ray
$-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
. The following conditions for a ray
![]() $\sigma $
in
$\sigma $
in
![]() $\Sigma $
are equivalent:
$\Sigma $
are equivalent:
-
(1)
 $\sigma \cap {U(I)_{\mathbb {Z}}} \ne \sigma \cap {U(I)_{\mathbb {Z}}^{\prime }}$
.
$\sigma \cap {U(I)_{\mathbb {Z}}} \ne \sigma \cap {U(I)_{\mathbb {Z}}^{\prime }}$
. -
(2)
 $-E_{w}$
acts trivially on the boundary divisor
$-E_{w}$
acts trivially on the boundary divisor
 $D(\sigma )$
.
$D(\sigma )$
. -
(3)
 $D(\sigma )$
is fixed by some nontrivial element of
$D(\sigma )$
is fixed by some nontrivial element of
 ${\overline {\Gamma (I)}_{\mathbb {Z}}}$
.
${\overline {\Gamma (I)}_{\mathbb {Z}}}$
.
When these hold, the element in
![]() $(3)$
is given by
$(3)$
is given by
![]() $-E_{w}$
. In particular, it is unique, independent of
$-E_{w}$
. In particular, it is unique, independent of
![]() $\sigma $
, and of order
$\sigma $
, and of order
![]() $2$
.
$2$
.
Definition 3.2 When these properties hold, we call
![]() $\sigma $
an irregular ray and
$\sigma $
an irregular ray and
![]() $D(\sigma )$
an irregular boundary divisor. Otherwise, we call
$D(\sigma )$
an irregular boundary divisor. Otherwise, we call
![]() $\sigma $
regular. For the sake of completeness, we call any ray
$\sigma $
regular. For the sake of completeness, we call any ray
![]() $\sigma $
regular when I is regular.
$\sigma $
regular when I is regular.
Proof
![]() $(1) \Leftrightarrow (2)$
: Recall from Lemma 2.1 that
$(1) \Leftrightarrow (2)$
: Recall from Lemma 2.1 that
![]() $-E_{w}$
acts on
$-E_{w}$
acts on
![]() ${\mathcal {X}(I)}\subset T(I)$
as the translation by
${\mathcal {X}(I)}\subset T(I)$
as the translation by
![]() $[w]\in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
. A Zariski open set of
$[w]\in {U(I)_{\mathbb {Q}/\mathbb {Z}}}$
. A Zariski open set of
![]() $D(\sigma )$
is the quotient torus (or its analytic open set) associated with the quotient lattice
$D(\sigma )$
is the quotient torus (or its analytic open set) associated with the quotient lattice
![]() ${U(I)_{\mathbb {Z}}}/\Lambda _{\sigma }$
where
${U(I)_{\mathbb {Z}}}/\Lambda _{\sigma }$
where
![]() $\Lambda _{\sigma }= {\mathbb {R}}\sigma \cap {U(I)_{\mathbb {Z}}}$
. Hence,
$\Lambda _{\sigma }= {\mathbb {R}}\sigma \cap {U(I)_{\mathbb {Z}}}$
. Hence,
![]() $-E_{w}$
acts on
$-E_{w}$
acts on
![]() $D(\sigma )$
as the translation by the image of
$D(\sigma )$
as the translation by the image of
![]() $[w]$
in
$[w]$
in
![]() ${U(I)_{\mathbb {Q}}}/({U(I)_{\mathbb {Z}}}+(\Lambda _{\sigma })_{{\mathbb {Q}}})$
. This is trivial if and only if
${U(I)_{\mathbb {Q}}}/({U(I)_{\mathbb {Z}}}+(\Lambda _{\sigma })_{{\mathbb {Q}}})$
. This is trivial if and only if
![]() $w\in {U(I)_{\mathbb {Z}}}+(\Lambda _{\sigma })_{{\mathbb {Q}}}$
, which in turn is equivalent to
$w\in {U(I)_{\mathbb {Z}}}+(\Lambda _{\sigma })_{{\mathbb {Q}}}$
, which in turn is equivalent to
![]() $\Lambda _{\sigma } \ne {\mathbb {R}}\sigma \cap {U(I)_{\mathbb {Z}}^{\prime }}$
. In this case,
$\Lambda _{\sigma } \ne {\mathbb {R}}\sigma \cap {U(I)_{\mathbb {Z}}^{\prime }}$
. In this case,
![]() $-E_{w}$
acts by
$-E_{w}$
acts by
![]() $-1$
on the normal torus
$-1$
on the normal torus
![]() $(\Lambda _{\sigma })_{{\mathbb {C}}}/\Lambda _{\sigma }\simeq {\mathbb {C}}^{\times }$
.
$(\Lambda _{\sigma })_{{\mathbb {C}}}/\Lambda _{\sigma }\simeq {\mathbb {C}}^{\times }$
.
![]() $(2) \Rightarrow (3)$
is obvious.
$(2) \Rightarrow (3)$
is obvious.
![]() $(3) \Rightarrow (2)$
: Suppose that
$(3) \Rightarrow (2)$
: Suppose that
![]() $\gamma \in {\overline {\Gamma (I)}_{\mathbb {Z}}}$
acts trivially on
$\gamma \in {\overline {\Gamma (I)}_{\mathbb {Z}}}$
acts trivially on
![]() $D(\sigma )$
. Let
$D(\sigma )$
. Let
![]() $\gamma _{1}$
be the image of
$\gamma _{1}$
be the image of
![]() $\gamma $
in
$\gamma $
in
![]() ${\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
. Then
${\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
. Then
![]() $\gamma _{1}$
must preserve
$\gamma _{1}$
must preserve
![]() $\sigma \cap {U(I)_{\mathbb {Z}}}$
and act trivially on
$\sigma \cap {U(I)_{\mathbb {Z}}}$
and act trivially on
![]() ${U(I)_{\mathbb {Z}}}/\Lambda _{\sigma }$
. Hence,
${U(I)_{\mathbb {Z}}}/\Lambda _{\sigma }$
. Hence,
![]() $\gamma _{1}$
acts trivially on
$\gamma _{1}$
acts trivially on
![]() $\Lambda _{\sigma }$
and
$\Lambda _{\sigma }$
and
![]() $\Lambda _{\sigma }^{\perp }$
, and so
$\Lambda _{\sigma }^{\perp }$
, and so
![]() $\gamma _{1}={\mathrm {id}}$
. This implies that
$\gamma _{1}={\mathrm {id}}$
. This implies that
![]() $\gamma $
is contained in the kernel of
$\gamma $
is contained in the kernel of
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm { O}^{+}}({U(I)_{\mathbb {Z}}})$
, whence
${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm { O}^{+}}({U(I)_{\mathbb {Z}}})$
, whence
![]() $\gamma =-E_{w}$
by Proposition 3.1. Therefore,
$\gamma =-E_{w}$
by Proposition 3.1. Therefore,
![]() $-E_{w}$
acts trivially on
$-E_{w}$
acts trivially on
![]() $D(\sigma )$
.
$D(\sigma )$
.
Corollary 3.8 When I is irregular, the quotient map
![]() ${\mathcal {X}(I)^{\Sigma }}\to {\mathcal {X}(I)^{\Sigma }}/{\overline {\Gamma (I)}_{\mathbb {Z}}}$
is ramified along the irregular boundary divisors with ramification index
${\mathcal {X}(I)^{\Sigma }}\to {\mathcal {X}(I)^{\Sigma }}/{\overline {\Gamma (I)}_{\mathbb {Z}}}$
is ramified along the irregular boundary divisors with ramification index
![]() $2$
, caused by the common subgroup
$2$
, caused by the common subgroup
![]() $\langle -E_{w} \rangle \simeq {\mathbb {Z}}/2$
of
$\langle -E_{w} \rangle \simeq {\mathbb {Z}}/2$
of
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}$
, and not ramified along other boundary divisors. When I is regular,
${\overline {\Gamma (I)}_{\mathbb {Z}}}$
, and not ramified along other boundary divisors. When I is regular,
![]() ${\mathcal {X}(I)^{\Sigma }}\to {\mathcal {X}(I)^{\Sigma }}/{\overline {\Gamma (I)}_{\mathbb {Z}}}$
is not ramified along any boundary divisor.
${\mathcal {X}(I)^{\Sigma }}\to {\mathcal {X}(I)^{\Sigma }}/{\overline {\Gamma (I)}_{\mathbb {Z}}}$
is not ramified along any boundary divisor.
Proof It remains to supplement the argument in the case I is regular. If
![]() $\gamma \in {\overline {\Gamma (I)}_{\mathbb {Z}}}$
fixes a boundary divisor, we see that
$\gamma \in {\overline {\Gamma (I)}_{\mathbb {Z}}}$
fixes a boundary divisor, we see that
![]() $\gamma $
acts trivially on
$\gamma $
acts trivially on
![]() ${U(I)_{\mathbb {Z}}}$
by the same argument as
${U(I)_{\mathbb {Z}}}$
by the same argument as
![]() $(3)\Rightarrow (2)$
above. When
$(3)\Rightarrow (2)$
above. When
![]() $-{\mathrm {id}}\not \in {\Gamma }$
,
$-{\mathrm {id}}\not \in {\Gamma }$
,
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
is injective by the condition (3) of Proposition 3.1, so we find that
${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm {O}^{+}}({U(I)_{\mathbb {Z}}})$
is injective by the condition (3) of Proposition 3.1, so we find that
![]() $\gamma = {\mathrm {id}}$
. When
$\gamma = {\mathrm {id}}$
. When
![]() $-{\mathrm {id}}\in {\Gamma }$
, the kernel of
$-{\mathrm {id}}\in {\Gamma }$
, the kernel of
![]() ${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm { O}^{+}}({U(I)_{\mathbb {Z}}})$
is
${\overline {\Gamma (I)}_{\mathbb {Z}}}\to {\mathrm { O}^{+}}({U(I)_{\mathbb {Z}}})$
is
![]() $\{ \pm {\mathrm {id}} \}$
, so
$\{ \pm {\mathrm {id}} \}$
, so
![]() $\gamma =\pm {\mathrm {id}}$
, which acts trivially on
$\gamma =\pm {\mathrm {id}}$
, which acts trivially on
![]() ${\mathcal {X}(I)}$
.
${\mathcal {X}(I)}$
.
4 Examples
In this section, we study some examples of groups with/without irregular cusp. Logically, this section should be read after Section 6 where we complete the discussion of irregular one-dimensional cusps. But we encourage the reader to read this section just after Section 3 for the following two reasons. First, most of Sections 5 and 6 is designed for Sections 7 and 8, while the only result from Sections 5 and 6 we need in this section is Corollary 6.4, which just says that
![]() ${\Gamma }$
has no irregular one-dimensional cusp if it has no irregular zero-dimensional cusp. Second, it is Proposition 3.1(2), which is frequently used in this section, so we do not want to put this section too far from it.
${\Gamma }$
has no irregular one-dimensional cusp if it has no irregular zero-dimensional cusp. Second, it is Proposition 3.1(2), which is frequently used in this section, so we do not want to put this section too far from it.
We assume that the lattice L is even in this section. The quotient
![]() $A_{L}=L^{\vee }/L$
is called the discriminant group of L, equipped with a canonical quadratic form
$A_{L}=L^{\vee }/L$
is called the discriminant group of L, equipped with a canonical quadratic form
![]() $A_{L}\to {\mathbb {Q}}/2{\mathbb {Z}}$
called the discriminant form. If I is a rank
$A_{L}\to {\mathbb {Q}}/2{\mathbb {Z}}$
called the discriminant form. If I is a rank
![]() $1$
primitive isotropic sublattice of L, we write
$1$
primitive isotropic sublattice of L, we write
![]() $\mathrm {div}(I)$
for the positive generator of the ideal
$\mathrm {div}(I)$
for the positive generator of the ideal
![]() $(I, L)\subset {\mathbb {Z}}$
. Then
$(I, L)\subset {\mathbb {Z}}$
. Then
![]() $I^{\ast }=\mathrm {div}(I)^{-1}I$
is primitive in
$I^{\ast }=\mathrm {div}(I)^{-1}I$
is primitive in
![]() $L^{\vee }$
, and we have a canonical isometry
$L^{\vee }$
, and we have a canonical isometry
![]() $I^{\perp }\cap L^{\vee }/I^{\ast } \simeq (I^{\perp }/I)^{\vee }$
.
$I^{\perp }\cap L^{\vee }/I^{\ast } \simeq (I^{\perp }/I)^{\vee }$
.
4.1 Stable orthogonal groups
Let L be an even lattice of signature
![]() $(2, b)$
. Let
$(2, b)$
. Let
![]() ${\widetilde {\mathrm {O}}^{+}(L)}<{\mathrm {O}^{+}(L)}$
be the kernel of the reduction map
${\widetilde {\mathrm {O}}^{+}(L)}<{\mathrm {O}^{+}(L)}$
be the kernel of the reduction map
![]() ${\mathrm { O}^{+}(L)}\to \mathrm {O}(A_{L})$
, called the stable orthogonal group or the discriminant kernel. The following was asserted in [Reference Ma13, p. 901]. We supplement the proof for the sake of completeness.
${\mathrm { O}^{+}(L)}\to \mathrm {O}(A_{L})$
, called the stable orthogonal group or the discriminant kernel. The following was asserted in [Reference Ma13, p. 901]. We supplement the proof for the sake of completeness.
Lemma 4.1 Let I be a rank
![]() $1$
primitive isotropic sublattice of L. For
$1$
primitive isotropic sublattice of L. For
![]() ${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
, we have
${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
, we have
![]() ${U(I)_{\mathbb {Z}}}=L(I)$
.
${U(I)_{\mathbb {Z}}}=L(I)$
.
Proof We take a generator l of I. The inclusion
![]() $L(I)\subset {U(I)_{\mathbb {Z}}}$
can be checked by testing the definition of
$L(I)\subset {U(I)_{\mathbb {Z}}}$
can be checked by testing the definition of
![]() $E_{m\otimes l}(v)$
for
$E_{m\otimes l}(v)$
for
![]() $v\in L^{\vee }$
and
$v\in L^{\vee }$
and
![]() $m\in I^{\perp }/I$
, taking a lift of m from
$m\in I^{\perp }/I$
, taking a lift of m from
![]() $I^{\perp }\cap L$
. Conversely, if
$I^{\perp }\cap L$
. Conversely, if
![]() $E_{m\otimes l}\in {\widetilde {\mathrm {O}}^{+}(L)}$
for a vector
$E_{m\otimes l}\in {\widetilde {\mathrm {O}}^{+}(L)}$
for a vector
![]() $m\in I^{\perp }_{{\mathbb {Q}}}/I_{{\mathbb {Q}}}$
, then
$m\in I^{\perp }_{{\mathbb {Q}}}/I_{{\mathbb {Q}}}$
, then
![]() $E_{m\otimes l}(v) = v - (m, v)l$
must be contained in
$E_{m\otimes l}(v) = v - (m, v)l$
must be contained in
![]() $v+L$
for
$v+L$
for
![]() $v\in I^{\perp }\cap L^{\vee }$
. This implies that
$v\in I^{\perp }\cap L^{\vee }$
. This implies that
![]() $(m, v)\in {\mathbb {Z}}$
for every
$(m, v)\in {\mathbb {Z}}$
for every
![]() $v\in I^{\perp }\cap L^{\vee }$
, and so
$v\in I^{\perp }\cap L^{\vee }$
, and so
![]() $m\in (I^{\perp }/I)^{\vee \vee }=I^{\perp }/I$
.
$m\in (I^{\perp }/I)^{\vee \vee }=I^{\perp }/I$
.
We obtain a first example of regular cusps.
Lemma 4.2 If
![]() ${\Gamma }\supset {\widetilde {\mathrm {O}}^{+}(L)}$
and
${\Gamma }\supset {\widetilde {\mathrm {O}}^{+}(L)}$
and
![]() $\mathrm {div}(I)=1$
, then I is a regular cusp for
$\mathrm {div}(I)=1$
, then I is a regular cusp for
![]() ${\Gamma }$
.
${\Gamma }$
.
Proof We take a generator l of I. Since
![]() $\mathrm {div}(I)=1$
, we can take an isotropic vector
$\mathrm {div}(I)=1$
, we can take an isotropic vector
![]() $l'\in L$
with
$l'\in L$
with
![]() $(l, l')=1$
. We can and do identify
$(l, l')=1$
. We can and do identify
![]() $I^{\perp }/I$
with
$I^{\perp }/I$
with
![]() $\langle l, l' \rangle ^{\perp }\cap L$
. We have the splitting
$\langle l, l' \rangle ^{\perp }\cap L$
. We have the splitting
![]() $L=\langle l, l' \rangle \oplus (\langle l, l' \rangle ^{\perp }\cap L)$
. Suppose
$L=\langle l, l' \rangle \oplus (\langle l, l' \rangle ^{\perp }\cap L)$
. Suppose
![]() $-E_{m\otimes l}\in {\Gamma }$
for a vector
$-E_{m\otimes l}\in {\Gamma }$
for a vector
![]() $m\in \langle l, l' \rangle ^{\perp }\cap L_{{\mathbb {Q}}}$
. Then
$m\in \langle l, l' \rangle ^{\perp }\cap L_{{\mathbb {Q}}}$
. Then
![]() $E_{m\otimes l}$
preserves L, so we find that the vector
$E_{m\otimes l}$
preserves L, so we find that the vector
![]() $E_{m\otimes l}(l') = l' + m - \frac {1}{2}(m, m)l$
is contained in L. This implies that
$E_{m\otimes l}(l') = l' + m - \frac {1}{2}(m, m)l$
is contained in L. This implies that
![]() $m\in \langle l, l' \rangle ^{\perp }\cap L=I^{\perp }/I$
. Since
$m\in \langle l, l' \rangle ^{\perp }\cap L=I^{\perp }/I$
. Since
![]() ${\Gamma }\supset {\widetilde {\mathrm {O}}^{+}(L)}$
, we have
${\Gamma }\supset {\widetilde {\mathrm {O}}^{+}(L)}$
, we have
![]() ${U(I)_{\mathbb {Z}}}\supset L(I)$
by Lemma 4.1, and so
${U(I)_{\mathbb {Z}}}\supset L(I)$
by Lemma 4.1, and so
![]() $m\otimes l \in {U(I)_{\mathbb {Z}}}$
. This means that
$m\otimes l \in {U(I)_{\mathbb {Z}}}$
. This means that
![]() $E_{m\otimes l}\in {\Gamma }$
, and then
$E_{m\otimes l}\in {\Gamma }$
, and then
![]() $-{\mathrm {id}}\in {\Gamma }$
.
$-{\mathrm {id}}\in {\Gamma }$
.
For
![]() ${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
, we have the following constraints for existence of irregular cusp.
${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
, we have the following constraints for existence of irregular cusp.
Lemma 4.3 If I is an irregular zero-dimensional cusp for
![]() ${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
, then
${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
, then
![]() $\mathrm {div}(I)=2$
,
$\mathrm {div}(I)=2$
,
![]() $A_{L(I)}$
is 2-elementary, and
$A_{L(I)}$
is 2-elementary, and
![]() ${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}\simeq {\mathbb {Z}}/2$
is a subgroup of
${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}\simeq {\mathbb {Z}}/2$
is a subgroup of
![]() $A_{L(I)}$
.
$A_{L(I)}$
.
Proof Let
![]() $I={\mathbb {Z}}l$
and assume that
$I={\mathbb {Z}}l$
and assume that
![]() $-E_{m\otimes l}\in {\widetilde {\mathrm {O}}^{+}(L)}$
for a vector m of
$-E_{m\otimes l}\in {\widetilde {\mathrm {O}}^{+}(L)}$
for a vector m of
![]() $I^{\perp }_{{\mathbb {Q}}}/I_{{\mathbb {Q}}}$
. Then, for any
$I^{\perp }_{{\mathbb {Q}}}/I_{{\mathbb {Q}}}$
. Then, for any
![]() $v\in I^{\perp }\cap L^{\vee }$
, the vector
$v\in I^{\perp }\cap L^{\vee }$
, the vector
![]() $-E_{m\otimes l}(v)=-v+(m, v)l$
must be contained in
$-E_{m\otimes l}(v)=-v+(m, v)l$
must be contained in
![]() $v+L$
. This implies that
$v+L$
. This implies that
If we substitute
![]() $v=l/\mathrm {div}(I)$
, we find that
$v=l/\mathrm {div}(I)$
, we find that
![]() $(2/\mathrm {div}(I))l \in L$
, and so
$(2/\mathrm {div}(I))l \in L$
, and so
![]() $\mathrm {div}(I)=1$
or
$\mathrm {div}(I)=1$
or
![]() $2$
. The case
$2$
. The case
![]() $\mathrm {div}(I)=1$
is excluded by Lemma 4.2. Thus,
$\mathrm {div}(I)=1$
is excluded by Lemma 4.2. Thus,
![]() $\mathrm {div}(I)=2$
.
$\mathrm {div}(I)=2$
.
If
![]() $[v]\in (I^{\perp }/I)^{\vee }$
denotes the image of
$[v]\in (I^{\perp }/I)^{\vee }$
denotes the image of
![]() $v\in I^{\perp }\cap L^{\vee }$
, then (4.1) means that
$v\in I^{\perp }\cap L^{\vee }$
, then (4.1) means that
![]() $2[v]\in I^{\perp }/I$
. This shows that
$2[v]\in I^{\perp }/I$
. This shows that
![]() $A_{I^{\perp }/I}$
is 2-elementary. Finally, (4.1) implies that
$A_{I^{\perp }/I}$
is 2-elementary. Finally, (4.1) implies that
![]() $(m, v)\in {\mathbb {Z}}$
for every
$(m, v)\in {\mathbb {Z}}$
for every
![]() $v\in I^{\perp }\cap L$
, and so
$v\in I^{\perp }\cap L$
, and so
![]() $m\in (I^{\perp }/I)^{\vee }$
.
$m\in (I^{\perp }/I)^{\vee }$
.
This determines the structure of
![]() $A_{L}$
when
$A_{L}$
when
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
has an irregular zero-dimensional cusp.
${\widetilde {\mathrm {O}}^{+}(L)}$
has an irregular zero-dimensional cusp.
Proposition 4.4 If
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
has an irregular cusp, then
${\widetilde {\mathrm {O}}^{+}(L)}$
has an irregular cusp, then
![]() $A_{L}\simeq {\mathbb {Z}}/8 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
or
$A_{L}\simeq {\mathbb {Z}}/8 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
or
![]() $A_{L}\simeq ({\mathbb {Z}}/4)^{\oplus 2} \oplus ({\mathbb {Z}}/2)^{\oplus a}$
as abelian groups.
$A_{L}\simeq ({\mathbb {Z}}/4)^{\oplus 2} \oplus ({\mathbb {Z}}/2)^{\oplus a}$
as abelian groups.
Proof Let
![]() $I={\mathbb {Z}}l$
be as in Lemma 4.3. Let
$I={\mathbb {Z}}l$
be as in Lemma 4.3. Let
![]() $x=[l/2]\in A_{L}$
. Since
$x=[l/2]\in A_{L}$
. Since
![]() $A_{L(I)}\simeq x^{\perp }/x$
is 2-elementary and both
$A_{L(I)}\simeq x^{\perp }/x$
is 2-elementary and both
![]() $\langle x \rangle $
and
$\langle x \rangle $
and
![]() $A_{L}/x^{\perp }$
are isomorphic to
$A_{L}/x^{\perp }$
are isomorphic to
![]() ${\mathbb {Z}}/2$
, we see that
${\mathbb {Z}}/2$
, we see that
![]() $A_{L}$
must be isomorphic to either
$A_{L}$
must be isomorphic to either
![]() $({\mathbb {Z}}/2)^{\oplus a}$
or
$({\mathbb {Z}}/2)^{\oplus a}$
or
![]() ${\mathbb {Z}}/4 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
or
${\mathbb {Z}}/4 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
or
![]() ${\mathbb {Z}}/8 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
or
${\mathbb {Z}}/8 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
or
![]() $({\mathbb {Z}}/4)^{\oplus 2} \oplus ({\mathbb {Z}}/2)^{\oplus a}$
as an abelian group. The first case
$({\mathbb {Z}}/4)^{\oplus 2} \oplus ({\mathbb {Z}}/2)^{\oplus a}$
as an abelian group. The first case
![]() $A_{L}\simeq ({\mathbb {Z}}/2)^{\oplus a}$
cannot occur because then
$A_{L}\simeq ({\mathbb {Z}}/2)^{\oplus a}$
cannot occur because then
![]() $-{\mathrm {id}}\in {\widetilde {\mathrm {O}}^{+}(L)}$
. Let us show that the second case does not occur.
$-{\mathrm {id}}\in {\widetilde {\mathrm {O}}^{+}(L)}$
. Let us show that the second case does not occur.
Suppose to the contrary that
![]() $A_{L}\simeq {\mathbb {Z}}/4 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
as an abelian group. Then we have an orthogonal decomposition
$A_{L}\simeq {\mathbb {Z}}/4 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
as an abelian group. Then we have an orthogonal decomposition
![]() $A_{L}=A_0 \oplus A_1$
where
$A_{L}=A_0 \oplus A_1$
where
![]() $A_0\simeq {\mathbb {Z}}/4$
is generated by an element
$A_0\simeq {\mathbb {Z}}/4$
is generated by an element
![]() $x_0$
of norm
$x_0$
of norm
![]() $\varepsilon /4$
for some
$\varepsilon /4$
for some
![]() $\varepsilon \in ({\mathbb {Z}}/8)^{\times }$
, and
$\varepsilon \in ({\mathbb {Z}}/8)^{\times }$
, and
![]() $A_1=A_{0}^{\perp }$
is 2-elementary. The isotropic element
$A_1=A_{0}^{\perp }$
is 2-elementary. The isotropic element
![]() $x\in A_{L}$
is either (i) contained in
$x\in A_{L}$
is either (i) contained in
![]() $A_1$
or (ii) of the from
$A_1$
or (ii) of the from
![]() $2x_{0}+x_1$
with
$2x_{0}+x_1$
with
![]() $x_1\ne 0 \in A_1$
. In the case (i),
$x_1\ne 0 \in A_1$
. In the case (i),
![]() $x^{\perp }/x \supset A_0$
is not 2-elementary. In the case (ii), we can take an element
$x^{\perp }/x \supset A_0$
is not 2-elementary. In the case (ii), we can take an element
![]() $y_1\in A_1$
with
$y_1\in A_1$
with
![]() $(x_1, y_1)=1/2$
by the nondegeneracy of
$(x_1, y_1)=1/2$
by the nondegeneracy of
![]() $A_1$
. Then the element
$A_1$
. Then the element
![]() $y=x_0+y_1$
is contained in
$y=x_0+y_1$
is contained in
![]() $x^{\perp }$
and
$x^{\perp }$
and
![]() $2y\ne x$
. Hence,
$2y\ne x$
. Hence,
![]() $x^{\perp }/x$
is not 2-elementary again.
$x^{\perp }/x$
is not 2-elementary again.
Remark 4.5 Further calculation shows that
![]() $x=[l/2]\in A_{L}$
is divisible by
$x=[l/2]\in A_{L}$
is divisible by
![]() $4$
(and hence unique) in the case
$4$
(and hence unique) in the case
![]() $A_{L}\simeq {\mathbb {Z}}/8 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
, and divisible by
$A_{L}\simeq {\mathbb {Z}}/8 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
, and divisible by
![]() $2$
in the case
$2$
in the case
![]() $A_{L}\simeq ({\mathbb {Z}}/4)^{\oplus 2} \oplus ({\mathbb {Z}}/2)^{\oplus a}$
.
$A_{L}\simeq ({\mathbb {Z}}/4)^{\oplus 2} \oplus ({\mathbb {Z}}/2)^{\oplus a}$
.
Example 4.6 Let
![]() $L=2U \oplus mE_{8} \oplus \langle -2d \rangle $
. Then
$L=2U \oplus mE_{8} \oplus \langle -2d \rangle $
. Then
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
has no irregular cusp when
${\widetilde {\mathrm {O}}^{+}(L)}$
has no irregular cusp when
![]() $d\ne 4$
. We show in Proposition 4.14 that
$d\ne 4$
. We show in Proposition 4.14 that
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
indeed has an irregular cusp when
${\widetilde {\mathrm {O}}^{+}(L)}$
indeed has an irregular cusp when
![]() $d=4$
. When
$d=4$
. When
![]() $m=2$
,
$m=2$
,
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
is the modular group for the moduli space of polarized
${\widetilde {\mathrm {O}}^{+}(L)}$
is the modular group for the moduli space of polarized
![]() $K3$
surfaces of degree
$K3$
surfaces of degree
![]() $2d$
.
$2d$
.
Example 4.7 Let
![]() $L=2U \oplus mE_{8} \oplus \langle -2t \rangle \oplus \langle -2d \rangle $
. Then
$L=2U \oplus mE_{8} \oplus \langle -2t \rangle \oplus \langle -2d \rangle $
. Then
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
has no irregular cusp when
${\widetilde {\mathrm {O}}^{+}(L)}$
has no irregular cusp when
![]() $(t, d) \ne (4, 1), (2, 2), (1, 4)$
. We show in Section 4.5 that
$(t, d) \ne (4, 1), (2, 2), (1, 4)$
. We show in Section 4.5 that
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
indeed has an irregular cusp in these exceptional cases. When
${\widetilde {\mathrm {O}}^{+}(L)}$
indeed has an irregular cusp in these exceptional cases. When
![]() $m=2$
,
$m=2$
,
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
is the modular group for the moduli space of polarized irreducible symplectic manifolds of
${\widetilde {\mathrm {O}}^{+}(L)}$
is the modular group for the moduli space of polarized irreducible symplectic manifolds of
![]() $K3^{[t-1]}$
-type with polarization of split type and degree
$K3^{[t-1]}$
-type with polarization of split type and degree
![]() $2d$
[Reference Gritsenko, Hulek and Sankaran10].
$2d$
[Reference Gritsenko, Hulek and Sankaran10].
Example 4.8 When
![]() $L=U\oplus 2E_{8} \oplus M$
, where M is a certain lattice of signature
$L=U\oplus 2E_{8} \oplus M$
, where M is a certain lattice of signature
![]() $(1, 2)$
and discriminant
$(1, 2)$
and discriminant
![]() $d\equiv 2$
mod
$d\equiv 2$
mod
![]() $6$
,
$6$
,
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
is the modular group for the moduli space of special cubic fourfolds of discriminant d [Reference Tanimoto and Várilly-Alvarado18]. Since
${\widetilde {\mathrm {O}}^{+}(L)}$
is the modular group for the moduli space of special cubic fourfolds of discriminant d [Reference Tanimoto and Várilly-Alvarado18]. Since
![]() $A_{L}$
has length
$A_{L}$
has length
![]() $\leq 3$
and order d, we find that
$\leq 3$
and order d, we find that
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
has no irregular cusp when
${\widetilde {\mathrm {O}}^{+}(L)}$
has no irregular cusp when
![]() $d \ne 8, 32$
.
$d \ne 8, 32$
.
Example 4.9 Similarly, when
![]() $L=U\oplus 2E_{8} \oplus M$
, where M is a certain lattice of signature
$L=U\oplus 2E_{8} \oplus M$
, where M is a certain lattice of signature
![]() $(1, 2)$
and discriminant
$(1, 2)$
and discriminant
![]() $d\equiv 0, 2, 4$
mod
$d\equiv 0, 2, 4$
mod
![]() $8$
,
$8$
,
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
is the modular group for the moduli space of special
${\widetilde {\mathrm {O}}^{+}(L)}$
is the modular group for the moduli space of special
![]() $K3^{[2]}$
-fourfolds of degree
$K3^{[2]}$
-fourfolds of degree
![]() $2$
and discriminant d [Reference Petok16]. This group has no irregular cusp when
$2$
and discriminant d [Reference Petok16]. This group has no irregular cusp when
![]() $d\ne 32$
.
$d\ne 32$
.
Example 4.10 When
![]() $L=U\oplus 2E_{8}\oplus \langle 2d \rangle $
,
$L=U\oplus 2E_{8}\oplus \langle 2d \rangle $
,
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
is the modular group for the moduli space of
${\widetilde {\mathrm {O}}^{+}(L)}$
is the modular group for the moduli space of
![]() $U\oplus \langle -2d \rangle $
-polarized
$U\oplus \langle -2d \rangle $
-polarized
![]() $K3$
surfaces studied in [Reference Fortuna and Mezzedimi4]. This group has no irregular cusp when
$K3$
surfaces studied in [Reference Fortuna and Mezzedimi4]. This group has no irregular cusp when
![]() $d\ne 4$
.
$d\ne 4$
.
4.2 O’Grady 10
In this subsection, we let L be an even lattice of the form
![]() $L= M \oplus \langle -2d \rangle $
with M of signature
$L= M \oplus \langle -2d \rangle $
with M of signature
![]() $(2, b-1)$
. We consider the group
$(2, b-1)$
. We consider the group
Then
![]() ${\Gamma }$
contains
${\Gamma }$
contains
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
with index
${\widetilde {\mathrm {O}}^{+}(L)}$
with index
![]() $\leq 2$
, with
$\leq 2$
, with
![]() ${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
if and only if
${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
if and only if
![]() $A_{M}$
is 2-elementary. We have
$A_{M}$
is 2-elementary. We have
![]() $-{\mathrm {id}}\in {\Gamma }$
if and only if
$-{\mathrm {id}}\in {\Gamma }$
if and only if
![]() $d=1$
. When
$d=1$
. When
![]() $M=2U\oplus 2E_{8} \oplus A_{2}$
,
$M=2U\oplus 2E_{8} \oplus A_{2}$
,
![]() ${\Gamma }$
is the modular group for the moduli space of polarized O’Grady
${\Gamma }$
is the modular group for the moduli space of polarized O’Grady
![]() $10$
manifolds with polarization of split type and degree
$10$
manifolds with polarization of split type and degree
![]() $2d$
[Reference Gritsenko, Hulek and Sankaran6].
$2d$
[Reference Gritsenko, Hulek and Sankaran6].
Proposition 4.11 The group
![]() ${\Gamma }$
has no irregular cusp when
${\Gamma }$
has no irregular cusp when
![]() $d\ne 2, 4$
.
$d\ne 2, 4$
.
Proof Assume that
![]() $I={\mathbb {Z}}l$
is an irregular cusp for
$I={\mathbb {Z}}l$
is an irregular cusp for
![]() ${\Gamma }$
and
${\Gamma }$
and
![]() $-E_{m\otimes l}\in {\Gamma }$
for
$-E_{m\otimes l}\in {\Gamma }$
for
![]() $m\in I^{\perp }_{{\mathbb {Q}}}/I_{{\mathbb {Q}}}$
. The case
$m\in I^{\perp }_{{\mathbb {Q}}}/I_{{\mathbb {Q}}}$
. The case
![]() $d=1$
is excluded by
$d=1$
is excluded by
![]() $-{\mathrm {id}}\not \in {\Gamma }$
. We shall show that
$-{\mathrm {id}}\not \in {\Gamma }$
. We shall show that
![]() $d\mid 4$
. Since
$d\mid 4$
. Since
![]() $E_{2m\otimes l}=(-E_{m\otimes l})\circ (-E_{m\otimes l}) \in {\widetilde {\mathrm {O}}^{+}(L)}$
, we see that
$E_{2m\otimes l}=(-E_{m\otimes l})\circ (-E_{m\otimes l}) \in {\widetilde {\mathrm {O}}^{+}(L)}$
, we see that
![]() $2m\otimes l \in L(I)$
by Lemma 4.1. Hence, we can take a lift
$2m\otimes l \in L(I)$
by Lemma 4.1. Hence, we can take a lift
![]() $\tilde {m}$
of m from
$\tilde {m}$
of m from
![]() $I^{\perp }\cap \frac {1}{2}L$
. Let v be a generator of
$I^{\perp }\cap \frac {1}{2}L$
. Let v be a generator of
![]() $\langle -2d \rangle ^{\vee }$
. The vector
$\langle -2d \rangle ^{\vee }$
. The vector
must be contained in
![]() $v+L$
, and hence
$v+L$
, and hence
Since
![]() $(\tilde {m}, v)\in \frac {1}{2}{\mathbb {Z}}$
,
$(\tilde {m}, v)\in \frac {1}{2}{\mathbb {Z}}$
,
![]() $(l, v)\in {\mathbb {Z}}$
and
$(l, v)\in {\mathbb {Z}}$
and
![]() $(m, m)\in \frac {1}{2}{\mathbb {Z}}$
, we find that
$(m, m)\in \frac {1}{2}{\mathbb {Z}}$
, we find that
![]() $2v\in \frac {1}{4}L$
. Hence,
$2v\in \frac {1}{4}L$
. Hence,
![]() $2d\mid 8$
.
$2d\mid 8$
.
The case
![]() $d=2$
does not occur when
$d=2$
does not occur when
![]() $|A_{M}|$
is square-free, because then
$|A_{M}|$
is square-free, because then
![]() $A_{L}$
is anisotropic and hence
$A_{L}$
is anisotropic and hence
![]() $\mathrm {div}(I)=1$
. In Proposition 4.14, we show that
$\mathrm {div}(I)=1$
. In Proposition 4.14, we show that
![]() ${\Gamma }$
indeed has an irregular cusp when
${\Gamma }$
indeed has an irregular cusp when
![]() $d=4$
and M contains U.
$d=4$
and M contains U.
4.3 Generalized Kummer
In this subsection, we let
![]() $L=M\oplus \langle -2d \rangle $
be as in Section 4.2 and consider the group
$L=M\oplus \langle -2d \rangle $
be as in Section 4.2 and consider the group
This is an index
![]() $\leq 2$
subgroup of the group considered in Section 4.2. When
$\leq 2$
subgroup of the group considered in Section 4.2. When
![]() $M=2U \oplus \langle -2t \rangle $
with
$M=2U \oplus \langle -2t \rangle $
with
![]() $t\geq 3$
,
$t\geq 3$
,
![]() ${\Gamma }$
is the modular group for the moduli space of polarized deformation generalized Kummer varieties of
${\Gamma }$
is the modular group for the moduli space of polarized deformation generalized Kummer varieties of
![]() $A^{[t]}$
-type with polarization of split type and degree
$A^{[t]}$
-type with polarization of split type and degree
![]() $2d$
[Reference Dawes2].
$2d$
[Reference Dawes2].
Proposition 4.12 The group
![]() ${\Gamma }$
has no irregular cusp when
${\Gamma }$
has no irregular cusp when
![]() $d\nmid 4$
. Moreover, when b is even,
$d\nmid 4$
. Moreover, when b is even,
![]() ${\Gamma }$
has no irregular cusp unless
${\Gamma }$
has no irregular cusp unless
![]() $A_{L}$
is isomorphic to
$A_{L}$
is isomorphic to
![]() ${\mathbb {Z}}/8 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
or
${\mathbb {Z}}/8 \oplus ({\mathbb {Z}}/2)^{\oplus a}$
or
![]() $({\mathbb {Z}}/4)^{\oplus 2} \oplus ({\mathbb {Z}}/2)^{\oplus a}$
as abelian groups.
$({\mathbb {Z}}/4)^{\oplus 2} \oplus ({\mathbb {Z}}/2)^{\oplus a}$
as abelian groups.
Proof The assertion
![]() $d\nmid 4$
follows from Corollary 3.5 and Proposition 4.11. Since
$d\nmid 4$
follows from Corollary 3.5 and Proposition 4.11. Since
![]() ${\Gamma }\cap \mathrm {SO}^{+}(L) = {\widetilde {\mathrm {O}}^{+}(L)}\cap \mathrm {SO}^{+}(L)$
, Corollary 3.4 shows that when b is even,
${\Gamma }\cap \mathrm {SO}^{+}(L) = {\widetilde {\mathrm {O}}^{+}(L)}\cap \mathrm {SO}^{+}(L)$
, Corollary 3.4 shows that when b is even,
![]() ${\Gamma }$
has an irregular cusp if and only if
${\Gamma }$
has an irregular cusp if and only if
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
has an irregular cusp. Then our assertion follows from Proposition 4.4.
${\widetilde {\mathrm {O}}^{+}(L)}$
has an irregular cusp. Then our assertion follows from Proposition 4.4.
This shows that when
![]() $M=2U \oplus \langle -2t \rangle $
,
$M=2U \oplus \langle -2t \rangle $
,
![]() ${\Gamma }$
has no irregular cusp when
${\Gamma }$
has no irregular cusp when
![]() $(t, d)\ne (4, 1), (2, 2), (1, 4)$
. In Section 4.5, we show that
$(t, d)\ne (4, 1), (2, 2), (1, 4)$
. In Section 4.5, we show that
![]() ${\Gamma }$
indeed has an irregular cusp in these exceptional cases.
${\Gamma }$
indeed has an irregular cusp in these exceptional cases.
4.4 Special cubic fourfolds
In this subsection, we let L be an even lattice of the form
![]() $L=M\oplus K$
with
$L=M\oplus K$
with
![]() $|A_{K}|>1$
odd. (K may be either negative-definite or hyperbolic or of signature
$|A_{K}|>1$
odd. (K may be either negative-definite or hyperbolic or of signature
![]() $(2, \ast )$
.) We consider the group
$(2, \ast )$
.) We consider the group
When
![]() $M=\langle 2n \rangle \oplus U \oplus 2E_{8}$
and
$M=\langle 2n \rangle \oplus U \oplus 2E_{8}$
and
![]() $K=A_{2}$
,
$K=A_{2}$
,
![]() ${\Gamma }$
is the modular group for the moduli space of special cubic fourfolds of discriminant
${\Gamma }$
is the modular group for the moduli space of special cubic fourfolds of discriminant
![]() $6n$
[Reference Tanimoto and Várilly-Alvarado18].
$6n$
[Reference Tanimoto and Várilly-Alvarado18].
Proposition 4.13 The group
![]() ${\Gamma }$
has no irregular cusp.
${\Gamma }$
has no irregular cusp.
Proof Suppose to the contrary that
![]() $I={\mathbb {Z}}l$
is an irregular cusp and
$I={\mathbb {Z}}l$
is an irregular cusp and
![]() $-E_{m\otimes l}\in {\Gamma }$
. As in the proof of Proposition 4.11, we can take a lift of m from
$-E_{m\otimes l}\in {\Gamma }$
. As in the proof of Proposition 4.11, we can take a lift of m from
![]() $I^{\perp }\cap \frac {1}{2}L$
. We take a vector
$I^{\perp }\cap \frac {1}{2}L$
. We take a vector
![]() $v\in K^{\vee }-K$
. Then
$v\in K^{\vee }-K$
. Then
![]() $-E_{m\otimes l}(v)$
must be contained in
$-E_{m\otimes l}(v)$
must be contained in
![]() $v+L$
. The same calculation as (4.2) tells us that
$v+L$
. The same calculation as (4.2) tells us that
![]() $8v\in L$
. Therefore,
$8v\in L$
. Therefore,
![]() $[v]\in A_{K}\subset A_{L}$
satisfies
$[v]\in A_{K}\subset A_{L}$
satisfies
![]() $8[v]=0$
, but this contradicts the assumption that
$8[v]=0$
, but this contradicts the assumption that
![]() $|A_{K}|$
is odd.
$|A_{K}|$
is odd.
4.5 Examples of irregular cusps
In this subsection, we present two series of examples of irregular cusps, infinitely many in every dimension. We will denote by
![]() $e, f$
the standard basis of U.
$e, f$
the standard basis of U.
As the first series of examples, we consider even lattices of the form
![]() $L=U\oplus \langle -8 \rangle \oplus M$
with M hyperbolic. We define the group
$L=U\oplus \langle -8 \rangle \oplus M$
with M hyperbolic. We define the group
![]() ${\Gamma }$
by
${\Gamma }$
by
This is the group considered in Section 4.2 with
![]() $d=4$
. The group
$d=4$
. The group
![]() ${\Gamma }$
contains
${\Gamma }$
contains
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
with index
${\widetilde {\mathrm {O}}^{+}(L)}$
with index
![]() $\leq 2$
, and we have
$\leq 2$
, and we have
![]() ${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
if and only if
${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
if and only if
![]() $A_{M}$
is 2-elementary.
$A_{M}$
is 2-elementary.
Proposition 4.14 The group
![]() ${\Gamma }$
has an irregular cusp.
${\Gamma }$
has an irregular cusp.
Proof First, note that
![]() $-{\mathrm {id}}\not \in {\Gamma }$
by the condition
$-{\mathrm {id}}\not \in {\Gamma }$
by the condition
![]() $\gamma |_{A_{\langle -8 \rangle }}={\mathrm { id}}$
. Let v be a generator of
$\gamma |_{A_{\langle -8 \rangle }}={\mathrm { id}}$
. Let v be a generator of
![]() $\langle -8 \rangle $
. We take the vectors
$\langle -8 \rangle $
. We take the vectors
and show that
![]() $-E_{m\otimes l}\in {\Gamma }$
. This amounts to checking the following:
$-E_{m\otimes l}\in {\Gamma }$
. This amounts to checking the following:
Since
![]() $M\perp \langle l, m \rangle $
,
$M\perp \langle l, m \rangle $
,
![]() $E_{m\otimes l}$
acts trivially on M. By direct calculation, we see that
$E_{m\otimes l}$
acts trivially on M. By direct calculation, we see that
This proves our assertion.
As the second series of examples, we consider even lattices of the form
![]() $L=U\oplus \langle -4 \rangle ^{\oplus 2} \oplus M$
with M hyperbolic, and the group
$L=U\oplus \langle -4 \rangle ^{\oplus 2} \oplus M$
with M hyperbolic, and the group
![]() ${\Gamma }$
defined by
${\Gamma }$
defined by
The group
![]() ${\Gamma }$
contains
${\Gamma }$
contains
![]() ${\widetilde {\mathrm {O}}^{+}(L)}$
with index
${\widetilde {\mathrm {O}}^{+}(L)}$
with index
![]() $\leq 2$
, and
$\leq 2$
, and
![]() ${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
if and only if
${\Gamma }={\widetilde {\mathrm {O}}^{+}(L)}$
if and only if
![]() $A_{M}$
is 2-elementary.
$A_{M}$
is 2-elementary.
Proposition 4.15 The group
![]() ${\Gamma }$
has an irregular cusp.
${\Gamma }$
has an irregular cusp.
Proof This is similar to the first example. We let
![]() $v_{1}, v_{2}$
be the standard basis of
$v_{1}, v_{2}$
be the standard basis of
![]() $\langle -4 \rangle ^{\oplus 2}$
and show that
$\langle -4 \rangle ^{\oplus 2}$
and show that
![]() $-E_{m\otimes l}\in {\Gamma }$
for the vectors
$-E_{m\otimes l}\in {\Gamma }$
for the vectors
The detail is left to the reader.
5 One-dimensional cusps
In this section, we recall, following [Reference Gritsenko, Hulek and Sankaran7, Reference Kondō11, Reference Looijenga12, Reference Scattone17], the structure of the stabilizer of a one-dimensional cusp of
![]() ${\mathcal {D}}={\mathcal {D}_{L}}$
with its action on the Siegel domain model, and the canonical partial toroidal compactification over the cusp. This is a long preliminary for the next Section 6. Although this section is no more than expository, we need to keep the rather self-contained style of Section 2, for the same reasons as in Section 2 and for consistency.
${\mathcal {D}}={\mathcal {D}_{L}}$
with its action on the Siegel domain model, and the canonical partial toroidal compactification over the cusp. This is a long preliminary for the next Section 6. Although this section is no more than expository, we need to keep the rather self-contained style of Section 2, for the same reasons as in Section 2 and for consistency.
Throughout this section, we fix a rank
![]() $2$
primitive isotropic sublattice J of L. The choice of the component
$2$
primitive isotropic sublattice J of L. The choice of the component
![]() ${\mathcal {D}}$
determines a connected component of
${\mathcal {D}}$
determines a connected component of
![]() ${{\mathbb P}}J_{{\mathbb {C}}} - {{\mathbb P}}J_{{\mathbb {R}}}$
, which is the cusp corresponding to J. This in turn determines an orientation of J. We abbreviate
${{\mathbb P}}J_{{\mathbb {C}}} - {{\mathbb P}}J_{{\mathbb {R}}}$
, which is the cusp corresponding to J. This in turn determines an orientation of J. We abbreviate
![]() $J^{\perp }=J^{\perp }\cap L$
and write
$J^{\perp }=J^{\perp }\cap L$
and write
which is a negative-definite lattice of rank
![]() $b-2$
. We will call an embedding
$b-2$
. We will call an embedding
![]() $2U_{{\mathbb {Q}}}\hookrightarrow L_{{\mathbb {Q}}}$
a splitting for
$2U_{{\mathbb {Q}}}\hookrightarrow L_{{\mathbb {Q}}}$
a splitting for
![]() $J_{{\mathbb {Q}}}$
if it sends the standard two-dimensional isotropic subspace of
$J_{{\mathbb {Q}}}$
if it sends the standard two-dimensional isotropic subspace of
![]() $2U_{{\mathbb {Q}}}$
to
$2U_{{\mathbb {Q}}}$
to
![]() $J_{{\mathbb {Q}}}$
. This defines a lift
$J_{{\mathbb {Q}}}$
. This defines a lift
![]() $L(J)_{{\mathbb {Q}}}\hookrightarrow J^{\perp }_{{\mathbb {Q}}}$
of
$L(J)_{{\mathbb {Q}}}\hookrightarrow J^{\perp }_{{\mathbb {Q}}}$
of
![]() $L(J)_{{\mathbb {Q}}}$
as
$L(J)_{{\mathbb {Q}}}$
as
![]() $2U_{{\mathbb {Q}}}^{\perp }$
.
$2U_{{\mathbb {Q}}}^{\perp }$
.
5.1 Siegel domain model
We consider the two-step linear projection
and restrict it to
![]() ${\mathcal {D}}\subset Q \subset {{\mathbb P}}L_{{\mathbb {C}}}$
. The center of the first projection
${\mathcal {D}}\subset Q \subset {{\mathbb P}}L_{{\mathbb {C}}}$
. The center of the first projection
![]() ${{\mathbb P}}L_{{\mathbb {C}}} \dashrightarrow {{\mathbb P}}(L/J)_{{\mathbb {C}}}$
is the line
${{\mathbb P}}L_{{\mathbb {C}}} \dashrightarrow {{\mathbb P}}(L/J)_{{\mathbb {C}}}$
is the line
![]() ${{\mathbb P}}J_{{\mathbb {C}}}$
, and its fibers are planes containing
${{\mathbb P}}J_{{\mathbb {C}}}$
, and its fibers are planes containing
![]() ${{\mathbb P}}J_{{\mathbb {C}}}$
(minus
${{\mathbb P}}J_{{\mathbb {C}}}$
(minus
![]() ${{\mathbb P}}J_{{\mathbb {C}}}$
). Since Q contains
${{\mathbb P}}J_{{\mathbb {C}}}$
). Since Q contains
![]() ${{\mathbb P}}J_{{\mathbb {C}}}$
, a plane containing
${{\mathbb P}}J_{{\mathbb {C}}}$
, a plane containing
![]() ${{\mathbb P}}J_{{\mathbb {C}}}$
either
${{\mathbb P}}J_{{\mathbb {C}}}$
either
-
• intersects with Q at two distinct lines (one is
 ${{\mathbb P}}J_{{\mathbb {C}}}$
), or
${{\mathbb P}}J_{{\mathbb {C}}}$
), or -
• intersects with Q at
 ${{\mathbb P}}J_{{\mathbb {C}}}$
with multiplicity
${{\mathbb P}}J_{{\mathbb {C}}}$
with multiplicity
 $2$
, or
$2$
, or -
• is contained in Q.
The first case occurs exactly when the plane is not contained in
![]() ${{\mathbb P}}J^{\perp }_{{\mathbb {C}}}$
. In that case, we can write the plane as
${{\mathbb P}}J^{\perp }_{{\mathbb {C}}}$
. In that case, we can write the plane as
![]() ${{\mathbb P}}\langle J, v \rangle _{{\mathbb {C}}}$
with
${{\mathbb P}}\langle J, v \rangle _{{\mathbb {C}}}$
with
![]() $(v, v)=0$
and
$(v, v)=0$
and
![]() $(v, J)\not \equiv 0$
. Then we have
$(v, J)\not \equiv 0$
. Then we have
where
![]() ${\mathbb {C}}l=v^{\perp }\cap J_{{\mathbb {C}}}$
. This shows that the restriction of the first projection
${\mathbb {C}}l=v^{\perp }\cap J_{{\mathbb {C}}}$
. This shows that the restriction of the first projection
![]() ${{\mathbb P}}L_{{\mathbb {C}}} \dashrightarrow {{\mathbb P}}(L/J)_{{\mathbb {C}}}$
to
${{\mathbb P}}L_{{\mathbb {C}}} \dashrightarrow {{\mathbb P}}(L/J)_{{\mathbb {C}}}$
to
![]() $Q-Q\cap {{\mathbb P}}J_{{\mathbb {C}}}^{\perp }$
$Q-Q\cap {{\mathbb P}}J_{{\mathbb {C}}}^{\perp }$
is an affine line bundle, with the fiber over the point
![]() ${{\mathbb P}}(\langle J, v \rangle _{{\mathbb {C}}}/J_{{\mathbb {C}}})$
being the affine line
${{\mathbb P}}(\langle J, v \rangle _{{\mathbb {C}}}/J_{{\mathbb {C}}})$
being the affine line
![]() ${{\mathbb P}}\langle l, v \rangle _{{\mathbb {C}}} - [l]$
. The inequality
${{\mathbb P}}\langle l, v \rangle _{{\mathbb {C}}} - [l]$
. The inequality
![]() $(\omega , \bar {\omega })>0$
defines a (shifted) upper half-plane in this affine line.
$(\omega , \bar {\omega })>0$
defines a (shifted) upper half-plane in this affine line.
We identify
![]() $(L/J^{\perp })_{{\mathbb {C}}}=J^{\vee }_{{\mathbb {C}}}$
by the pairing. The second projection
$(L/J^{\perp })_{{\mathbb {C}}}=J^{\vee }_{{\mathbb {C}}}$
by the pairing. The second projection
is an affine space bundle. It is (non-canonically) isomorphic to the vector bundle
![]() $L(J)_{{\mathbb {C}}} \otimes \mathcal {O}_{{{\mathbb P}}J^{\vee }_{{\mathbb {C}}}}(1)$
where, by abuse of notation,
$L(J)_{{\mathbb {C}}} \otimes \mathcal {O}_{{{\mathbb P}}J^{\vee }_{{\mathbb {C}}}}(1)$
where, by abuse of notation,
![]() $\mathcal {O}_{{{\mathbb P}}J^{\vee }_{{\mathbb {C}}}}(1)$
stands for the line bundle corresponding to this sheaf (the dual of the tautological line bundle). To be more specific, if we choose a lift
$\mathcal {O}_{{{\mathbb P}}J^{\vee }_{{\mathbb {C}}}}(1)$
stands for the line bundle corresponding to this sheaf (the dual of the tautological line bundle). To be more specific, if we choose a lift
![]() $L(J)_{{\mathbb {C}}}\hookrightarrow J_{{\mathbb {C}}}^{\perp }$
of
$L(J)_{{\mathbb {C}}}\hookrightarrow J_{{\mathbb {C}}}^{\perp }$
of
![]() $L(J)_{{\mathbb {C}}}$
, this determines a splitting
$L(J)_{{\mathbb {C}}}$
, this determines a splitting
![]() $(L/J)_{{\mathbb {C}}}\simeq L(J)_{{\mathbb {C}}}\oplus (L/J^{\perp })_{{\mathbb {C}}}$
where
$(L/J)_{{\mathbb {C}}}\simeq L(J)_{{\mathbb {C}}}\oplus (L/J^{\perp })_{{\mathbb {C}}}$
where
![]() $(L/J^{\perp })_{{\mathbb {C}}}$
is mapped to
$(L/J^{\perp })_{{\mathbb {C}}}$
is mapped to
![]() $L(J)_{{\mathbb {C}}}^{\perp }/J_{{\mathbb {C}}}$
. This splitting defines an isomorphism
$L(J)_{{\mathbb {C}}}^{\perp }/J_{{\mathbb {C}}}$
. This splitting defines an isomorphism
over
![]() ${{\mathbb P}}J_{{\mathbb {C}}}^{\vee }={{\mathbb P}}(L/J^{\perp })_{{\mathbb {C}}}$
. At the fiber over each point
${{\mathbb P}}J_{{\mathbb {C}}}^{\vee }={{\mathbb P}}(L/J^{\perp })_{{\mathbb {C}}}$
. At the fiber over each point
![]() $[v]$
of
$[v]$
of
![]() ${{\mathbb P}}(L/J^{\perp })_{{\mathbb {C}}}$
, this isomorphism is written as
${{\mathbb P}}(L/J^{\perp })_{{\mathbb {C}}}$
, this isomorphism is written as
where to a linear map
![]() ${\mathbb {C}}v\to L(J)_{{\mathbb {C}}}$
we associate its graph.
${\mathbb {C}}v\to L(J)_{{\mathbb {C}}}$
we associate its graph.
The orientation of J determines a connected component
![]() ${\mathbb {H}_{J}}$
of
${\mathbb {H}_{J}}$
of
![]() ${{\mathbb P}}J^{\vee }_{{\mathbb {C}}} - {{\mathbb P}}J^{\vee }_{{\mathbb {R}}}$
. We write
${{\mathbb P}}J^{\vee }_{{\mathbb {C}}} - {{\mathbb P}}J^{\vee }_{{\mathbb {R}}}$
. We write
![]() ${\mathcal {V}_{J}} = \pi _{2}^{-1}({\mathbb {H}_{J}})$
and
${\mathcal {V}_{J}} = \pi _{2}^{-1}({\mathbb {H}_{J}})$
and
![]() ${\mathcal {D}}(J) = \pi _{1}^{-1}({\mathcal {V}_{J}})$
. By definition,
${\mathcal {D}}(J) = \pi _{1}^{-1}({\mathcal {V}_{J}})$
. By definition,
![]() ${\mathcal {D}}(J)$
consists of points
${\mathcal {D}}(J)$
consists of points
![]() ${\mathbb {C}}\omega \in Q$
such that the map
${\mathbb {C}}\omega \in Q$
such that the map
![]() $(\cdot , \omega )\colon J_{{\mathbb {R}}}\to {\mathbb {C}}$
is an orientation-preserving
$(\cdot , \omega )\colon J_{{\mathbb {R}}}\to {\mathbb {C}}$
is an orientation-preserving
![]() ${\mathbb {R}}$
-isomorphism. We thus have the enlarged two-step fibration
${\mathbb {R}}$
-isomorphism. We thus have the enlarged two-step fibration
This is the Siegel domain realization of
![]() ${\mathcal {D}}$
with respect to J. Here
${\mathcal {D}}$
with respect to J. Here
![]() ${\mathcal {D}}(J) \to {\mathcal {V}_{J}}$
is an affine line bundle, inside which
${\mathcal {D}}(J) \to {\mathcal {V}_{J}}$
is an affine line bundle, inside which
![]() ${\mathcal {D}}\to {\mathcal {V}_{J}}$
is a fibration of upper half-planes. Over
${\mathcal {D}}\to {\mathcal {V}_{J}}$
is a fibration of upper half-planes. Over
![]() ${\mathbb {H}_{J}}\subset {{\mathbb P}}J_{{\mathbb {C}}}^{\vee }$
, we have the Hodge line bundle in
${\mathbb {H}_{J}}\subset {{\mathbb P}}J_{{\mathbb {C}}}^{\vee }$
, we have the Hodge line bundle in
![]() $\underline {J_{{\mathbb {C}}}}=J_{{\mathbb {C}}} \otimes \mathcal {O}_{{\mathbb {H}_{J}}}$
defined by
$\underline {J_{{\mathbb {C}}}}=J_{{\mathbb {C}}} \otimes \mathcal {O}_{{\mathbb {H}_{J}}}$
defined by
where we view
![]() $\mathcal {O}_{{\mathbb {H}_{J}}}(-1)$
as a sub-line bundle of
$\mathcal {O}_{{\mathbb {H}_{J}}}(-1)$
as a sub-line bundle of
![]() $J_{{\mathbb {C}}}^{\vee } \otimes \mathcal {O}_{{\mathbb {H}_{J}}}$
naturally. Then
$J_{{\mathbb {C}}}^{\vee } \otimes \mathcal {O}_{{\mathbb {H}_{J}}}$
naturally. Then
![]() $\mathcal {O}_{{\mathbb {H}_{J}}}(1)$
is naturally isomorphic to
$\mathcal {O}_{{\mathbb {H}_{J}}}(1)$
is naturally isomorphic to
![]() $\underline {J_{{\mathbb {C}}}}/F$
. To summarize, we have an isomorphism
$\underline {J_{{\mathbb {C}}}}/F$
. To summarize, we have an isomorphism
The relation with the tube domain model is as follows. We choose a rank
![]() $1$
primitive sublattice I of J. This corresponds to a zero-dimensional cusp in the closure of the one-dimensional cusp for J. The filtration
$1$
primitive sublattice I of J. This corresponds to a zero-dimensional cusp in the closure of the one-dimensional cusp for J. The filtration
![]() $I\subset J \subset J^{\perp } \subset L$
determines the projections
$I\subset J \subset J^{\perp } \subset L$
determines the projections
![]() ${{\mathbb P}}(L/I)_{{\mathbb {C}}}\dashrightarrow {{\mathbb P}}(L/J)_{{\mathbb {C}}}\dashrightarrow {{\mathbb P}}(L/J^{\perp })_{{\mathbb {C}}}$
. Then the composition of this with the tube domain realization
${{\mathbb P}}(L/I)_{{\mathbb {C}}}\dashrightarrow {{\mathbb P}}(L/J)_{{\mathbb {C}}}\dashrightarrow {{\mathbb P}}(L/J^{\perp })_{{\mathbb {C}}}$
. Then the composition of this with the tube domain realization
![]() ${\mathcal {D}}\subset {\mathcal {D}(I)} \hookrightarrow {{\mathbb P}}(L/I)_{{\mathbb {C}}}$
is the Siegel domain realization above.
${\mathcal {D}}\subset {\mathcal {D}(I)} \hookrightarrow {{\mathbb P}}(L/I)_{{\mathbb {C}}}$
is the Siegel domain realization above.
5.2 Stabilizer over
 ${\mathbb {Q}}$
${\mathbb {Q}}$
Let
![]() ${\Gamma (J)_{\mathbb {Q}}}$
be the subgroup of the stabilizer of
${\Gamma (J)_{\mathbb {Q}}}$
be the subgroup of the stabilizer of
![]() $J_{{\mathbb {Q}}}$
in
$J_{{\mathbb {Q}}}$
in
![]() $\mathrm {O}^{+}(L_{{\mathbb {Q}}})$
that acts on
$\mathrm {O}^{+}(L_{{\mathbb {Q}}})$
that acts on
![]() $J_{{\mathbb {Q}}}$
with determinant
$J_{{\mathbb {Q}}}$
with determinant
![]() $1$
. The determinant
$1$
. The determinant
![]() $1$
condition is not restrictive when restricting to subgroups of
$1$
condition is not restrictive when restricting to subgroups of
![]() ${\mathrm {O}^{+}(L)}$
. We write
${\mathrm {O}^{+}(L)}$
. We write
By definition, we have the canonical exact sequences
The group
![]() ${W(J)_{\mathbb {Q}}}$
is the unipotent radical of
${W(J)_{\mathbb {Q}}}$
is the unipotent radical of
![]() ${\Gamma (J)_{\mathbb {Q}}}$
. If we choose a splitting
${\Gamma (J)_{\mathbb {Q}}}$
. If we choose a splitting
![]() $L_{{\mathbb {Q}}}\simeq 2U_{{\mathbb {Q}}}\oplus L(J)_{{\mathbb {Q}}}$
for
$L_{{\mathbb {Q}}}\simeq 2U_{{\mathbb {Q}}}\oplus L(J)_{{\mathbb {Q}}}$
for
![]() $J_{{\mathbb {Q}}}$
, the first exact sequence (5.3) splits (non-canonically):
$J_{{\mathbb {Q}}}$
, the first exact sequence (5.3) splits (non-canonically):
Here
![]() $\mathrm {SL}(J_{{\mathbb {Q}}})$
acts on the component
$\mathrm {SL}(J_{{\mathbb {Q}}})$
acts on the component
![]() $2U_{{\mathbb {Q}}}\simeq J_{{\mathbb {Q}}}\oplus J_{{\mathbb {Q}}}^{\vee }$
, and
$2U_{{\mathbb {Q}}}\simeq J_{{\mathbb {Q}}}\oplus J_{{\mathbb {Q}}}^{\vee }$
, and
![]() $\mathrm {O}(L(J)_{{\mathbb {Q}}})$
acts on the component
$\mathrm {O}(L(J)_{{\mathbb {Q}}})$
acts on the component
![]() $L(J)_{{\mathbb {Q}}}$
.
$L(J)_{{\mathbb {Q}}}$
.
On the other hand, the second exact sequence (5.4) never splits. Indeed,
![]() ${W(J)_{\mathbb {Q}}}$
is a Heisenberg group as follows. We have a canonical
${W(J)_{\mathbb {Q}}}$
is a Heisenberg group as follows. We have a canonical
![]() $\wedge ^{2}J$
-valued symplectic form on
$\wedge ^{2}J$
-valued symplectic form on
![]() $L(J)\otimes J$
as the tensor product of the quadratic form on
$L(J)\otimes J$
as the tensor product of the quadratic form on
![]() $L(J)$
and the canonical symplectic form
$L(J)$
and the canonical symplectic form
![]() $J\times J \to \wedge ^{2}J$
on J. This gives a Heisenberg group structure on
$J\times J \to \wedge ^{2}J$
on J. This gives a Heisenberg group structure on
![]() $\wedge ^{2}J_{{\mathbb {Q}}}\times (L(J)_{{\mathbb {Q}}}\otimes J_{{\mathbb {Q}}})$
. Explicitly, we take a bijection
$\wedge ^{2}J_{{\mathbb {Q}}}\times (L(J)_{{\mathbb {Q}}}\otimes J_{{\mathbb {Q}}})$
. Explicitly, we take a bijection
![]() $L(J)_{{\mathbb {Q}}}\otimes J_{{\mathbb {Q}}} \simeq L(J)_{{\mathbb {Q}}} \times L(J)_{{\mathbb {Q}}}$
by choosing a positive basis of J, and define a product on
$L(J)_{{\mathbb {Q}}}\otimes J_{{\mathbb {Q}}} \simeq L(J)_{{\mathbb {Q}}} \times L(J)_{{\mathbb {Q}}}$
by choosing a positive basis of J, and define a product on
![]() $\wedge ^{2}J_{{\mathbb {Q}}}\times L(J)_{{\mathbb {Q}}} \times L(J)_{{\mathbb {Q}}}$
by
$\wedge ^{2}J_{{\mathbb {Q}}}\times L(J)_{{\mathbb {Q}}} \times L(J)_{{\mathbb {Q}}}$
by
The center is
![]() $\wedge ^{2}J_{{\mathbb {Q}}}\times \{ 0 \} \times \{ 0 \}$
.
$\wedge ^{2}J_{{\mathbb {Q}}}\times \{ 0 \} \times \{ 0 \}$
.
Lemma 5.1
![]() ${W(J)_{\mathbb {Q}}}$
is isomorphic to the Heisenberg group for
${W(J)_{\mathbb {Q}}}$
is isomorphic to the Heisenberg group for
![]() $L(J)_{{\mathbb {Q}}}\otimes J_{{\mathbb {Q}}}$
with center
$L(J)_{{\mathbb {Q}}}\otimes J_{{\mathbb {Q}}}$
with center
![]() ${U(J)_{\mathbb {Q}}}$
, and we have the canonical isomorphisms
${U(J)_{\mathbb {Q}}}$
, and we have the canonical isomorphisms
Proof This should be well-known (see, e.g., [Reference Looijenga12]), but we provide a proof in the present context for the convenience of the readers. We choose a rank
![]() $1$
primitive sublattice I of J and put
$1$
primitive sublattice I of J and put
![]() $\bar {J}=(J/I)\otimes I \subset L(I)$
. Note that
$\bar {J}=(J/I)\otimes I \subset L(I)$
. Note that
![]() $\bar {J}\simeq \wedge ^{2}J$
naturally. We restrict the sequence (2.3) for
$\bar {J}\simeq \wedge ^{2}J$
naturally. We restrict the sequence (2.3) for
![]() ${\Gamma (I)_{\mathbb {Q}}}$
to
${\Gamma (I)_{\mathbb {Q}}}$
to
![]() ${W(J)_{\mathbb {Q}}}\subset {\Gamma (I)_{\mathbb {Q}}}$
. It is clear that
${W(J)_{\mathbb {Q}}}\subset {\Gamma (I)_{\mathbb {Q}}}$
. It is clear that
![]() ${W(J)_{\mathbb {Q}}} \cap {U(I)_{\mathbb {Q}}} = \bar {J}_{{\mathbb {Q}}}^{\perp }\cap L(I)_{{\mathbb {Q}}}$
, which contains
${W(J)_{\mathbb {Q}}} \cap {U(I)_{\mathbb {Q}}} = \bar {J}_{{\mathbb {Q}}}^{\perp }\cap L(I)_{{\mathbb {Q}}}$
, which contains
![]() ${U(J)_{\mathbb {Q}}}$
with
${U(J)_{\mathbb {Q}}}$
with
The image of
![]() ${W(J)_{\mathbb {Q}}}\to {\mathrm {O}^{+}}(L(I)_{{\mathbb {Q}}})$
is the subgroup of the stabilizer of
${W(J)_{\mathbb {Q}}}\to {\mathrm {O}^{+}}(L(I)_{{\mathbb {Q}}})$
is the subgroup of the stabilizer of
![]() $\bar {J}_{{\mathbb {Q}}}$
that acts trivially on
$\bar {J}_{{\mathbb {Q}}}$
that acts trivially on
![]() $\bar {J}_{{\mathbb {Q}}}$
and
$\bar {J}_{{\mathbb {Q}}}$
and
![]() $\bar {J}_{{\mathbb {Q}}}^{\perp }/\bar {J}_{{\mathbb {Q}}}$
. This consists of Eichler transvections of
$\bar {J}_{{\mathbb {Q}}}^{\perp }/\bar {J}_{{\mathbb {Q}}}$
. This consists of Eichler transvections of
![]() $L(I)_{{\mathbb {Q}}}$
with respect to
$L(I)_{{\mathbb {Q}}}$
with respect to
![]() $\bar {J}_{{\mathbb {Q}}}$
, hence isomorphic to
$\bar {J}_{{\mathbb {Q}}}$
, hence isomorphic to
![]() $(\bar {J}_{{\mathbb {Q}}}^{\perp }/\bar {J}_{{\mathbb {Q}}})\otimes \bar {J}_{{\mathbb {Q}}} \simeq L(J)_{{\mathbb {Q}}}\otimes (J/I)_{{\mathbb {Q}}}$
. In this way, we obtain the exact sequence
$(\bar {J}_{{\mathbb {Q}}}^{\perp }/\bar {J}_{{\mathbb {Q}}})\otimes \bar {J}_{{\mathbb {Q}}} \simeq L(J)_{{\mathbb {Q}}}\otimes (J/I)_{{\mathbb {Q}}}$
. In this way, we obtain the exact sequence
We choose lifts
![]() $L(J)_{{\mathbb {Q}}}\hookrightarrow J_{{\mathbb {Q}}}^{\perp }$
and
$L(J)_{{\mathbb {Q}}}\hookrightarrow J_{{\mathbb {Q}}}^{\perp }$
and
![]() $(J/I)_{{\mathbb {Q}}}\hookrightarrow J_{{\mathbb {Q}}}$
. This induces a section of (5.7) which consists of the Eichler transvections
$(J/I)_{{\mathbb {Q}}}\hookrightarrow J_{{\mathbb {Q}}}$
. This induces a section of (5.7) which consists of the Eichler transvections
![]() $E_{w}$
of
$E_{w}$
of
![]() $L_{{\mathbb {Q}}}$
with
$L_{{\mathbb {Q}}}$
with
![]() $w\in L(J)_{{\mathbb {Q}}}\otimes (J/I)_{{\mathbb {Q}}}$
. Together with the splitting
$w\in L(J)_{{\mathbb {Q}}}\otimes (J/I)_{{\mathbb {Q}}}$
. Together with the splitting
![]() $\bar {J}_{{\mathbb {Q}}}^{\perp }\cap L(I)_{{\mathbb {Q}}} \simeq \bar {J}_{{\mathbb {Q}}} \oplus (L(J)_{{\mathbb {Q}}}\otimes I_{{\mathbb {Q}}})$
, we obtain a bijection
$\bar {J}_{{\mathbb {Q}}}^{\perp }\cap L(I)_{{\mathbb {Q}}} \simeq \bar {J}_{{\mathbb {Q}}} \oplus (L(J)_{{\mathbb {Q}}}\otimes I_{{\mathbb {Q}}})$
, we obtain a bijection
This gives an isomorphism with the Heisenberg group.
Note that
![]() ${U(J)_{\mathbb {Q}}}$
is not just the center of
${U(J)_{\mathbb {Q}}}$
is not just the center of
![]() ${W(J)_{\mathbb {Q}}}$
, but also the center of
${W(J)_{\mathbb {Q}}}$
, but also the center of
![]() ${\Gamma (J)_{\mathbb {Q}}}$
. This is the reason we put the determinant
${\Gamma (J)_{\mathbb {Q}}}$
. This is the reason we put the determinant
![]() $1$
condition in the definition of
$1$
condition in the definition of
![]() ${\Gamma (J)_{\mathbb {Q}}}$
.
${\Gamma (J)_{\mathbb {Q}}}$
.
The action of
![]() ${\Gamma (J)_{\mathbb {Q}}}$
on the Siegel domain model can be described through the filtration
${\Gamma (J)_{\mathbb {Q}}}$
on the Siegel domain model can be described through the filtration
![]() ${U(J)_{\mathbb {Q}}}\subset {W(J)_{\mathbb {Q}}}\subset {\Gamma (J)_{\mathbb {Q}}}$
. By definition
${U(J)_{\mathbb {Q}}}\subset {W(J)_{\mathbb {Q}}}\subset {\Gamma (J)_{\mathbb {Q}}}$
. By definition
![]() ${U(J)_{\mathbb {Q}}}$
acts on
${U(J)_{\mathbb {Q}}}$
acts on
![]() ${\mathcal {V}_{J}}\subset {{\mathbb P}}(J_{{\mathbb {C}}}^{\perp })^{\vee }$
trivially and
${\mathcal {V}_{J}}\subset {{\mathbb P}}(J_{{\mathbb {C}}}^{\perp })^{\vee }$
trivially and
![]() ${W(J)_{\mathbb {Q}}}$
acts on
${W(J)_{\mathbb {Q}}}$
acts on
![]() ${\mathbb {H}_{J}}\subset {{\mathbb P}}J_{{\mathbb {C}}}^{\vee }$
trivially. We let
${\mathbb {H}_{J}}\subset {{\mathbb P}}J_{{\mathbb {C}}}^{\vee }$
trivially. We let
![]() $U(J)_{{\mathbb {C}}}\subset \mathrm {O}(L_{{\mathbb {C}}})$
be the group of Eichler transvections
$U(J)_{{\mathbb {C}}}\subset \mathrm {O}(L_{{\mathbb {C}}})$
be the group of Eichler transvections
![]() $E_{l\otimes l'}$
with
$E_{l\otimes l'}$
with
![]() $l, l'\in J_{{\mathbb {C}}}$
. Then
$l, l'\in J_{{\mathbb {C}}}$
. Then
![]() $U(J)_{{\mathbb {C}}}\simeq \wedge ^{2}J_{{\mathbb {C}}}$
preserves
$U(J)_{{\mathbb {C}}}\simeq \wedge ^{2}J_{{\mathbb {C}}}$
preserves
![]() ${\mathcal {D}}(J)$
and acts on
${\mathcal {D}}(J)$
and acts on
![]() ${\mathcal {V}_{J}}$
trivially. The following descriptions should be well-known, but we provide a proof in the present setting for the convenience of the readers.
${\mathcal {V}_{J}}$
trivially. The following descriptions should be well-known, but we provide a proof in the present setting for the convenience of the readers.
Lemma 5.2 The following holds.
(1)
![]() ${\mathcal {D}}(J)\to {\mathcal {V}_{J}}$
is a principal
${\mathcal {D}}(J)\to {\mathcal {V}_{J}}$
is a principal
![]() $U(J)_{{\mathbb {C}}}$
-bundle.
$U(J)_{{\mathbb {C}}}$
-bundle.
(2) The group
![]() ${V(J)_{\mathbb {Q}}}\simeq L(J)_{{\mathbb {Q}}}\otimes J_{{\mathbb {Q}}}$
acts on
${V(J)_{\mathbb {Q}}}\simeq L(J)_{{\mathbb {Q}}}\otimes J_{{\mathbb {Q}}}$
acts on
![]() ${\mathcal {V}_{J}}\to {\mathbb {H}_{J}}$
as the relative translation on the vector bundle
${\mathcal {V}_{J}}\to {\mathbb {H}_{J}}$
as the relative translation on the vector bundle
![]() $L(J)_{{\mathbb {C}}} \otimes (\underline {J_{{\mathbb {C}}}}/F)$
via an isomorphism (5.2).
$L(J)_{{\mathbb {C}}} \otimes (\underline {J_{{\mathbb {C}}}}/F)$
via an isomorphism (5.2).
(3) We choose a splitting for
![]() $J_{{\mathbb {Q}}}$
, which induces a splitting (5.5) of
$J_{{\mathbb {Q}}}$
, which induces a splitting (5.5) of
![]() ${\Gamma (J)_{\mathbb {Q}}}$
and an isomorphism (5.2) for
${\Gamma (J)_{\mathbb {Q}}}$
and an isomorphism (5.2) for
![]() ${\mathcal {V}_{J}}$
. Then the lifted subgroup
${\mathcal {V}_{J}}$
. Then the lifted subgroup
![]() $\mathrm {O}(L(J)_{{\mathbb {Q}}})\times \mathrm {SL}(J_{{\mathbb {Q}}})$
of
$\mathrm {O}(L(J)_{{\mathbb {Q}}})\times \mathrm {SL}(J_{{\mathbb {Q}}})$
of
![]() ${\Gamma (J)_{\mathbb {Q}}}$
acts on
${\Gamma (J)_{\mathbb {Q}}}$
acts on
![]() ${\mathcal {V}_{J}}\to {\mathbb {H}_{J}}$
by the equivariant action of
${\mathcal {V}_{J}}\to {\mathbb {H}_{J}}$
by the equivariant action of
![]() $\mathrm {SL}(J_{{\mathbb {Q}}})$
on
$\mathrm {SL}(J_{{\mathbb {Q}}})$
on
![]() $\underline {J_{{\mathbb {C}}}}/F$
and the linear action of
$\underline {J_{{\mathbb {C}}}}/F$
and the linear action of
![]() $\mathrm {O}(L(J)_{{\mathbb {Q}}})$
on
$\mathrm {O}(L(J)_{{\mathbb {Q}}})$
on
![]() $L(J)_{{\mathbb {C}}}$
.
$L(J)_{{\mathbb {C}}}$
.
Proof (1) Recall from Section 5.1 that a fiber of
![]() ${\mathcal {D}}(J)\to {\mathcal {V}_{J}}$
is an affine line
${\mathcal {D}}(J)\to {\mathcal {V}_{J}}$
is an affine line
![]() ${{\mathbb P}}\langle l, v \rangle _{{\mathbb {C}}}-[l]$
where
${{\mathbb P}}\langle l, v \rangle _{{\mathbb {C}}}-[l]$
where
![]() $v\in L_{{\mathbb {C}}}$
is an isotropic vector with
$v\in L_{{\mathbb {C}}}$
is an isotropic vector with
![]() $(v, J)\not \equiv 0$
and
$(v, J)\not \equiv 0$
and
![]() ${\mathbb {C}}l=v^{\perp }\cap J_{{\mathbb {C}}}$
. We take
${\mathbb {C}}l=v^{\perp }\cap J_{{\mathbb {C}}}$
. We take
![]() $l'\in J_{{\mathbb {C}}}$
with
$l'\in J_{{\mathbb {C}}}$
with
![]() $(l', v)=1$
. Then
$(l', v)=1$
. Then
![]() $E_{\alpha l\wedge l'}\in U(J)_{{\mathbb {C}}}$
,
$E_{\alpha l\wedge l'}\in U(J)_{{\mathbb {C}}}$
,
![]() $\alpha \in {\mathbb {C}}$
, sends a point
$\alpha \in {\mathbb {C}}$
, sends a point
![]() ${\mathbb {C}}(v+\beta l)$
of
${\mathbb {C}}(v+\beta l)$
of
![]() ${{\mathbb P}}\langle l, v \rangle _{{\mathbb {C}}}-[l]$
to
${{\mathbb P}}\langle l, v \rangle _{{\mathbb {C}}}-[l]$
to
This shows that
![]() $U(J)_{{\mathbb {C}}}$
acts on each fiber of
$U(J)_{{\mathbb {C}}}$
acts on each fiber of
![]() ${\mathcal {D}}(J)\to {\mathcal {V}_{J}}$
freely and transitively.
${\mathcal {D}}(J)\to {\mathcal {V}_{J}}$
freely and transitively.
(2) We choose a splitting
![]() $L_{{\mathbb {Q}}}\simeq J_{{\mathbb {Q}}}\oplus L(J)_{{\mathbb {Q}}} \oplus (L/J^{\perp })_{{\mathbb {Q}}}$
for
$L_{{\mathbb {Q}}}\simeq J_{{\mathbb {Q}}}\oplus L(J)_{{\mathbb {Q}}} \oplus (L/J^{\perp })_{{\mathbb {Q}}}$
for
![]() $J_{{\mathbb {Q}}}$
where the lift of
$J_{{\mathbb {Q}}}$
where the lift of
![]() $(L/J^{\perp })_{{\mathbb {Q}}}$
is perpendicular to the lift of
$(L/J^{\perp })_{{\mathbb {Q}}}$
is perpendicular to the lift of
![]() $L(J)_{{\mathbb {Q}}}$
. Let
$L(J)_{{\mathbb {Q}}}$
. Let
![]() $[v]$
be a point of
$[v]$
be a point of
![]() ${\mathbb {H}_{J}}\subset {{\mathbb P}}(L/J^{\perp })_{{\mathbb {C}}}$
. By (5.1), the fiber of
${\mathbb {H}_{J}}\subset {{\mathbb P}}(L/J^{\perp })_{{\mathbb {C}}}$
. By (5.1), the fiber of
![]() ${\mathcal {V}_{J}}\to {\mathbb {H}_{J}}$
over
${\mathcal {V}_{J}}\to {\mathbb {H}_{J}}$
over
![]() $[v]$
is the affine line
$[v]$
is the affine line
in
![]() ${{\mathbb P}}(L/J)_{{\mathbb {C}}}$
. Here the point corresponding to
${{\mathbb P}}(L/J)_{{\mathbb {C}}}$
. Here the point corresponding to
![]() $f\in {\operatorname {Hom}}({\mathbb {C}}v, L(J)_{{\mathbb {C}}})$
is its graph
$f\in {\operatorname {Hom}}({\mathbb {C}}v, L(J)_{{\mathbb {C}}})$
is its graph
![]() ${\mathbb {C}}(v+f(v))$
. We take
${\mathbb {C}}(v+f(v))$
. We take
![]() $E_{m\otimes l}\in {W(J)_{\mathbb {Q}}}/{U(J)_{\mathbb {Q}}}$
where
$E_{m\otimes l}\in {W(J)_{\mathbb {Q}}}/{U(J)_{\mathbb {Q}}}$
where
![]() $m\in L(J)_{{\mathbb {Q}}}$
and
$m\in L(J)_{{\mathbb {Q}}}$
and
![]() $l\in J_{{\mathbb {Q}}}$
. Then
$l\in J_{{\mathbb {Q}}}$
. Then
![]() $E_{m\otimes l}$
sends
$E_{m\otimes l}$
sends
![]() ${\mathbb {C}}(v+f(v))$
to
${\mathbb {C}}(v+f(v))$
to
 $$ \begin{align*} {\mathbb{C}}(v+f(v)) & \mapsto {\mathbb{C}}(v + (l, v)m - 2^{-1}(m, m)(l, v)l + f(v) - (m, f(v))l) \\ & = {\mathbb{C}}(v + f(v) + (l, v)m) \: \: \in {{\mathbb P}}(L/J)_{{\mathbb{C}}}. \end{align*} $$
$$ \begin{align*} {\mathbb{C}}(v+f(v)) & \mapsto {\mathbb{C}}(v + (l, v)m - 2^{-1}(m, m)(l, v)l + f(v) - (m, f(v))l) \\ & = {\mathbb{C}}(v + f(v) + (l, v)m) \: \: \in {{\mathbb P}}(L/J)_{{\mathbb{C}}}. \end{align*} $$
This means that
![]() $E_{m\otimes l}$
acts on
$E_{m\otimes l}$
acts on
![]() ${\operatorname {Hom}}({\mathbb {C}}v, L(J)_{{\mathbb {C}}})$
by the translation
${\operatorname {Hom}}({\mathbb {C}}v, L(J)_{{\mathbb {C}}})$
by the translation
![]() $f\mapsto f + (l, \cdot )m$
. Finally, we notice that the
$f\mapsto f + (l, \cdot )m$
. Finally, we notice that the
![]() ${\mathbb {R}}$
-isomorphism
${\mathbb {R}}$
-isomorphism
sends
![]() $m\otimes l$
to
$m\otimes l$
to
![]() $m\otimes (l, \cdot )$
. This proves the assertion (2).
$m\otimes (l, \cdot )$
. This proves the assertion (2).
The proof of (3) is straightforward and is left to the readers.
5.3 Stabilizer over
 ${\mathbb {Z}}$
${\mathbb {Z}}$
Now let
![]() ${\Gamma }$
be a finite-index subgroup of
${\Gamma }$
be a finite-index subgroup of
![]() ${\mathrm {O}^{+}(L)}$
. We write
${\mathrm {O}^{+}(L)}$
. We write
and consider the quotients
By definition, we have the canonical exact sequences
Then (5.8) is canonically embedded in (5.9). We have
as subgroups of
![]() ${W(J)_{\mathbb {Q}/\mathbb {Z}}}$
because
${W(J)_{\mathbb {Q}/\mathbb {Z}}}$
because
![]() ${W(J)_{\mathbb {Z}}} \cap {U(J)_{\mathbb {Q}}}={U(J)_{\mathbb {Z}}}$
by definition. Note that
${W(J)_{\mathbb {Z}}} \cap {U(J)_{\mathbb {Q}}}={U(J)_{\mathbb {Z}}}$
by definition. Note that
![]() ${U(J)_{\mathbb {Q}/\mathbb {Z}}}$
is the group of torsion points of the one-dimensional torus
${U(J)_{\mathbb {Q}/\mathbb {Z}}}$
is the group of torsion points of the one-dimensional torus
![]() $T(J)=U(J)_{{\mathbb {C}}}/{U(J)_{\mathbb {Z}}}$
. If we choose a splitting for
$T(J)=U(J)_{{\mathbb {C}}}/{U(J)_{\mathbb {Z}}}$
. If we choose a splitting for
![]() $J_{{\mathbb {Q}}}$
, the induced splitting (5.5) of
$J_{{\mathbb {Q}}}$
, the induced splitting (5.5) of
![]() ${\Gamma (J)_{\mathbb {Q}}}$
defines a splitting of (5.9):
${\Gamma (J)_{\mathbb {Q}}}$
defines a splitting of (5.9):
But this does not mean that (5.8) splits.
5.4 Partial toroidal compactification
We denote
![]() ${\mathcal {T}(J)}={\mathcal {D}}(J)/{U(J)_{\mathbb {Z}}}$
and
${\mathcal {T}(J)}={\mathcal {D}}(J)/{U(J)_{\mathbb {Z}}}$
and
![]() ${\mathcal {X}(J)}={\mathcal {D}}/{U(J)_{\mathbb {Z}}}$
. By Lemma 5.2,
${\mathcal {X}(J)}={\mathcal {D}}/{U(J)_{\mathbb {Z}}}$
. By Lemma 5.2,
![]() ${\mathcal {T}(J)}\to {\mathcal {V}_{J}}$
is a principal
${\mathcal {T}(J)}\to {\mathcal {V}_{J}}$
is a principal
![]() $T(J)$
-bundle acted on equivariantly by
$T(J)$
-bundle acted on equivariantly by
![]() ${\overline {\Gamma (J)}_{\mathbb {Q}}}$
. The projection
${\overline {\Gamma (J)}_{\mathbb {Q}}}$
. The projection
![]() ${\mathcal {X}(J)\to {\mathcal {V}_{J}}}$
is a punctured disk bundle in
${\mathcal {X}(J)\to {\mathcal {V}_{J}}}$
is a punctured disk bundle in
![]() ${\mathcal {T}(J)}\to {\mathcal {V}_{J}}$
. By Lemma 5.2, the action of
${\mathcal {T}(J)}\to {\mathcal {V}_{J}}$
. By Lemma 5.2, the action of
![]() ${\overline {\Gamma (J)}_{\mathbb {Q}}}$
on
${\overline {\Gamma (J)}_{\mathbb {Q}}}$
on
![]() ${\mathcal {V}_{J}}\to {\mathbb {H}_{J}}$
is described as follows.
${\mathcal {V}_{J}}\to {\mathbb {H}_{J}}$
is described as follows.
Lemma 5.3 We choose a splitting for
![]() $J_{{\mathbb {Q}}}$
to give an isomorphism (5.2) for
$J_{{\mathbb {Q}}}$
to give an isomorphism (5.2) for
![]() ${\mathcal {V}_{J}}$
and a splitting (5.11) of
${\mathcal {V}_{J}}$
and a splitting (5.11) of
![]() ${\overline {\Gamma (J)}_{\mathbb {Q}}}$
. We express an element
${\overline {\Gamma (J)}_{\mathbb {Q}}}$
. We express an element
![]() $\gamma $
of
$\gamma $
of
![]() ${\overline {\Gamma (J)}_{\mathbb {Q}}}$
as
${\overline {\Gamma (J)}_{\mathbb {Q}}}$
as
![]() $\gamma =(\gamma _{1}, \gamma _{2}, \alpha )$
accordingly, where
$\gamma =(\gamma _{1}, \gamma _{2}, \alpha )$
accordingly, where
![]() $\gamma _{1}\in \mathrm {O}(L(J))$
,
$\gamma _{1}\in \mathrm {O}(L(J))$
,
![]() $\gamma _{2}\in \mathrm {SL}(J)$
, and
$\gamma _{2}\in \mathrm {SL}(J)$
, and
![]() $\alpha \in {W(J)_{\mathbb {Q}/\mathbb {Z}}}$
. Then
$\alpha \in {W(J)_{\mathbb {Q}/\mathbb {Z}}}$
. Then
![]() $\gamma $
acts on
$\gamma $
acts on
![]() ${\mathcal {V}_{J}}\simeq L(J)_{{\mathbb {C}}}\otimes (\underline {J_{{\mathbb {C}}}}/F)$
as the equivariant action by
${\mathcal {V}_{J}}\simeq L(J)_{{\mathbb {C}}}\otimes (\underline {J_{{\mathbb {C}}}}/F)$
as the equivariant action by
![]() $(\gamma _{1}, \gamma _{2})$
and the translation by
$(\gamma _{1}, \gamma _{2})$
and the translation by
![]() $[\alpha ]\in {V(J)_{\mathbb {Q}}}\simeq L(J)_{{\mathbb {Q}}}\otimes J_{{\mathbb {Q}}}$
.
$[\alpha ]\in {V(J)_{\mathbb {Q}}}\simeq L(J)_{{\mathbb {Q}}}\otimes J_{{\mathbb {Q}}}$
.
Thus,
![]() ${\mathcal {V}_{J}}/{V(J)_{\mathbb {Z}}}$
is a fibration of abelian varieties over
${\mathcal {V}_{J}}/{V(J)_{\mathbb {Z}}}$
is a fibration of abelian varieties over
![]() ${\mathbb {H}_{J}}$
isogenous to the self-fiber product of the universal elliptic curve. The group
${\mathbb {H}_{J}}$
isogenous to the self-fiber product of the universal elliptic curve. The group
![]() $\Gamma _{J}$
acts on
$\Gamma _{J}$
acts on
![]() ${\mathcal {V}_{J}}/{V(J)_{\mathbb {Z}}}$
by the equivariant action plus some possible translation.
${\mathcal {V}_{J}}/{V(J)_{\mathbb {Z}}}$
by the equivariant action plus some possible translation.
Now let
![]() $\overline {T(J)}\simeq {\mathbb {C}}$
be the canonical partial compactification of the torus
$\overline {T(J)}\simeq {\mathbb {C}}$
be the canonical partial compactification of the torus
![]() $T(J)$
. We take the relative torus embedding
$T(J)$
. We take the relative torus embedding
This is the line bundle associated with the principal
![]() $T(J)$
-bundle
$T(J)$
-bundle
![]() ${\mathcal {T}(J)}\to {\mathcal {V}_{J}}$
and the standard character of
${\mathcal {T}(J)}\to {\mathcal {V}_{J}}$
and the standard character of
![]() $T(J)$
. Let
$T(J)$
. Let
![]() ${\overline {\mathcal {X}(J)}}$
be the interior of the closure of
${\overline {\mathcal {X}(J)}}$
be the interior of the closure of
![]() ${\mathcal {X}(J)}$
in
${\mathcal {X}(J)}$
in
![]() ${\overline {\mathcal {T}(J)}}$
. This is the partial toroidal compactification of
${\overline {\mathcal {T}(J)}}$
. This is the partial toroidal compactification of
![]() ${\mathcal {X}(J)}$
over the one-dimensional cusp J. Note that no choice of fan is required: this is canonical. The boundary divisor of
${\mathcal {X}(J)}$
over the one-dimensional cusp J. Note that no choice of fan is required: this is canonical. The boundary divisor of
![]() ${\overline {\mathcal {X}(J)}}$
is canonically isomorphic to
${\overline {\mathcal {X}(J)}}$
is canonically isomorphic to
![]() ${\mathcal {V}_{J}}$
.
${\mathcal {V}_{J}}$
.
The relation with a partial toroidal compactification over an adjacent zero-dimensional cusp
![]() $I \subset J$
is as follows. Recall that
$I \subset J$
is as follows. Recall that
![]() $\bar {J}=(J/I)\otimes I \simeq \wedge ^{2}J$
is an isotropic sublattice of
$\bar {J}=(J/I)\otimes I \simeq \wedge ^{2}J$
is an isotropic sublattice of
![]() $L(I)$
, oriented by the orientation of J. The ray
$L(I)$
, oriented by the orientation of J. The ray
![]() $\sigma _{J}=(\bar {J}_{{\mathbb {R}}})_{\geq 0}$
is in the closure of the positive cone, and it is contained in any
$\sigma _{J}=(\bar {J}_{{\mathbb {R}}})_{\geq 0}$
is in the closure of the positive cone, and it is contained in any
![]() ${\Gamma (I)_{\mathbb {Z}}}$
-admissible fan
${\Gamma (I)_{\mathbb {Z}}}$
-admissible fan
![]() $\Sigma $
. The torus embedding
$\Sigma $
. The torus embedding
![]() $T(I)\hookrightarrow T(I)^{\sigma _{J}}$
defined by
$T(I)\hookrightarrow T(I)^{\sigma _{J}}$
defined by
![]() $\sigma _{J}$
is a Zariski open set of
$\sigma _{J}$
is a Zariski open set of
![]() $T(I)^{\Sigma }$
. By (5.6), we have
$T(I)^{\Sigma }$
. By (5.6), we have
![]() $U(J)_{{\mathbb {R}}}=\bar {J}_{{\mathbb {R}}}\subset U(I)_{{\mathbb {R}}}$
and
$U(J)_{{\mathbb {R}}}=\bar {J}_{{\mathbb {R}}}\subset U(I)_{{\mathbb {R}}}$
and
Therefore, the inclusion
![]() ${\mathcal {D}}(J)\subset {\mathcal {D}(I)}$
induces the etale map
${\mathcal {D}}(J)\subset {\mathcal {D}(I)}$
induces the etale map
which maps the boundary divisor of
![]() ${\overline {\mathcal {T}(J)}}$
to the unique boundary divisor of
${\overline {\mathcal {T}(J)}}$
to the unique boundary divisor of
![]() $T(I)^{\sigma _{J}}$
. We note that
$T(I)^{\sigma _{J}}$
. We note that
![]() ${U(I)_{\mathbb {Z}}}\subset {\Gamma (J)_{\mathbb {Z}}}$
.
${U(I)_{\mathbb {Z}}}\subset {\Gamma (J)_{\mathbb {Z}}}$
.
6 Irregular one-dimensional cusps
In this section, we define and study irregular one-dimensional cusps. For simplicity, we assume
![]() $b\geq 3$
so that
$b\geq 3$
so that
![]() $L(J)\ne \{ 0 \}$
. Let
$L(J)\ne \{ 0 \}$
. Let
![]() ${\Gamma }$
be a finite-index subgroup of
${\Gamma }$
be a finite-index subgroup of
![]() ${\mathrm {O}^{+}(L)}$
, and let J be a rank
${\mathrm {O}^{+}(L)}$
, and let J be a rank
![]() $2$
primitive isotropic sublattice of L. We keep the notation from Section 5. Irregularity of the one-dimensional cusp J can be characterized as follows.
$2$
primitive isotropic sublattice of L. We keep the notation from Section 5. Irregularity of the one-dimensional cusp J can be characterized as follows.
Proposition 6.1 The following conditions are equivalent.
-
(1)
 ${U(J)_{\mathbb {Z}}}\ne {U(J)_{\mathbb {Z}}^{\prime }}$
where
${U(J)_{\mathbb {Z}}}\ne {U(J)_{\mathbb {Z}}^{\prime }}$
where
 ${U(J)_{\mathbb {Z}}^{\prime }}={U(J)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
.
${U(J)_{\mathbb {Z}}^{\prime }}={U(J)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
. -
(2)
 $-{\mathrm {id}}\not \in {\Gamma }$
and
$-{\mathrm {id}}\not \in {\Gamma }$
and
 $-E_{w}\in {\Gamma (J)_{\mathbb {Z}}}$
for some
$-E_{w}\in {\Gamma (J)_{\mathbb {Z}}}$
for some
 $w\in \wedge ^{2}J_{{\mathbb {Q}}}$
.
$w\in \wedge ^{2}J_{{\mathbb {Q}}}$
. -
(3)
 $-{\mathrm {id}}\not \in {\Gamma }$
and
$-{\mathrm {id}}\not \in {\Gamma }$
and
 ${\overline {\Gamma (J)}_{\mathbb {Z}}}$
contains an element
${\overline {\Gamma (J)}_{\mathbb {Z}}}$
contains an element
 $\gamma $
of finite order whose image in
$\gamma $
of finite order whose image in
 $\mathrm {O}(L(J))\times \mathrm {SL}(J)$
is
$\mathrm {O}(L(J))\times \mathrm {SL}(J)$
is
 $(-{\mathrm {id}}_{L(J)}, -{\mathrm {id}}_{J})$
.
$(-{\mathrm {id}}_{L(J)}, -{\mathrm {id}}_{J})$
. -
(4)
 ${\overline {\Gamma (J)}_{\mathbb {Z}}}$
contains an element
${\overline {\Gamma (J)}_{\mathbb {Z}}}$
contains an element
 $\gamma $
which acts trivially on
$\gamma $
which acts trivially on
 ${\mathcal {V}_{J}}$
but nontrivially on
${\mathcal {V}_{J}}$
but nontrivially on
 ${\mathcal {X}(J)}$
.
${\mathcal {X}(J)}$
.
When these hold, the element
![]() $\gamma $
of
$\gamma $
of
![]() ${\overline {\Gamma (J)}_{\mathbb {Z}}}$
in (3), (4) is given by
${\overline {\Gamma (J)}_{\mathbb {Z}}}$
in (3), (4) is given by
![]() $-E_{w}$
in (2), has order
$-E_{w}$
in (2), has order
![]() $2$
, and is unique.
$2$
, and is unique.
Definition 6.1 We say that the one-dimensional cusp J is irregular when these properties hold, and regular otherwise.
Proof The equivalence
![]() $(1) \Leftrightarrow (2)$
is similar to
$(1) \Leftrightarrow (2)$
is similar to
![]() $(1) \Leftrightarrow (2)$
in Proposition 3.1. The quotient
$(1) \Leftrightarrow (2)$
in Proposition 3.1. The quotient
![]() ${U(J)_{\mathbb {Z}}^{\prime }}/{U(J)_{\mathbb {Z}}}\simeq {\mathbb {Z}}/2$
is generated by
${U(J)_{\mathbb {Z}}^{\prime }}/{U(J)_{\mathbb {Z}}}\simeq {\mathbb {Z}}/2$
is generated by
![]() $E_{w}$
in (2).
$E_{w}$
in (2).
![]() $(2) \Rightarrow (4)$
: Since
$(2) \Rightarrow (4)$
: Since
![]() $E_{w}$
for
$E_{w}$
for
![]() $w\in \wedge ^{2}J_{{\mathbb {Q}}}$
acts trivially on
$w\in \wedge ^{2}J_{{\mathbb {Q}}}$
acts trivially on
![]() ${\mathcal {V}_{J}}$
by Lemma 5.2, so does
${\mathcal {V}_{J}}$
by Lemma 5.2, so does
![]() $-E_{w}$
.
$-E_{w}$
.
![]() $(2) \Rightarrow (3)$
: The element
$(2) \Rightarrow (3)$
: The element
![]() $\gamma =[-E_{w}]$
of
$\gamma =[-E_{w}]$
of
![]() ${\overline {\Gamma (J)}_{\mathbb {Z}}}$
is of order
${\overline {\Gamma (J)}_{\mathbb {Z}}}$
is of order
![]() $2$
and acts on J,
$2$
and acts on J,
![]() $L(J)$
by
$L(J)$
by
![]() $-1$
.
$-1$
.
![]() $(3) \Rightarrow (4)$
: By the description of the
$(3) \Rightarrow (4)$
: By the description of the
![]() ${\overline {\Gamma (J)}_{\mathbb {Z}}}$
-action on
${\overline {\Gamma (J)}_{\mathbb {Z}}}$
-action on
![]() ${\mathcal {V}_{J}}$
in Lemma 5.3, we find that the element
${\mathcal {V}_{J}}$
in Lemma 5.3, we find that the element
![]() $\gamma $
of (3) acts on
$\gamma $
of (3) acts on
![]() ${\mathcal {V}_{J}}$
by some translation. Since
${\mathcal {V}_{J}}$
by some translation. Since
![]() $\gamma $
is of finite order by assumption, this translation must be trivial.
$\gamma $
is of finite order by assumption, this translation must be trivial.
![]() $(4) \Rightarrow (2), (3)$
: Suppose that
$(4) \Rightarrow (2), (3)$
: Suppose that
![]() $\gamma \in {\overline {\Gamma (J)}_{\mathbb {Z}}}$
acts trivially on
$\gamma \in {\overline {\Gamma (J)}_{\mathbb {Z}}}$
acts trivially on
![]() ${\mathcal {V}_{J}}$
but nontrivially on
${\mathcal {V}_{J}}$
but nontrivially on
![]() ${\mathcal {X}(J)}$
. We take a splitting (5.11) of
${\mathcal {X}(J)}$
. We take a splitting (5.11) of
![]() ${\overline {\Gamma (J)}_{\mathbb {Q}}}$
and express
${\overline {\Gamma (J)}_{\mathbb {Q}}}$
and express
![]() $\gamma = (\gamma _{1}, \gamma _{2}, \alpha )$
accordingly. Since
$\gamma = (\gamma _{1}, \gamma _{2}, \alpha )$
accordingly. Since
![]() $\gamma $
acts on
$\gamma $
acts on
![]() $\mathbb {H}_{J}$
trivially, we must have
$\mathbb {H}_{J}$
trivially, we must have
![]() $\gamma _{2}={\mathrm {id}}_{J}$
or
$\gamma _{2}={\mathrm {id}}_{J}$
or
![]() $-{\mathrm {id}}_{J}$
. Then, since
$-{\mathrm {id}}_{J}$
. Then, since
![]() $\gamma $
acts on
$\gamma $
acts on
![]() ${\mathcal {V}_{J}}\simeq L(J)_{{\mathbb {C}}}\otimes (\underline {J_{{\mathbb {C}}}}/F)$
trivially, we see from Lemma 5.3 that
${\mathcal {V}_{J}}\simeq L(J)_{{\mathbb {C}}}\otimes (\underline {J_{{\mathbb {C}}}}/F)$
trivially, we see from Lemma 5.3 that
![]() $(\gamma _{1}, \gamma _{2}) = ({\mathrm {id}}_{L(J)}, {\mathrm {id}}_{J})$
or
$(\gamma _{1}, \gamma _{2}) = ({\mathrm {id}}_{L(J)}, {\mathrm {id}}_{J})$
or
![]() $(-{\mathrm {id}}_{L(J)}, -{\mathrm { id}}_{J})$
, and the image of
$(-{\mathrm {id}}_{L(J)}, -{\mathrm { id}}_{J})$
, and the image of
![]() $\alpha \in {W(J)_{\mathbb {Q}/\mathbb {Z}}}$
in
$\alpha \in {W(J)_{\mathbb {Q}/\mathbb {Z}}}$
in
![]() ${V(J)_{\mathbb {Q}}}$
must be
${V(J)_{\mathbb {Q}}}$
must be
![]() $0$
, namely
$0$
, namely
![]() $\alpha \in {U(J)_{\mathbb {Q}/\mathbb {Z}}}$
. The case
$\alpha \in {U(J)_{\mathbb {Q}/\mathbb {Z}}}$
. The case
![]() $(\gamma _{1}, \gamma _{2}) = ({\mathrm {id}}_{L(J)}, {\mathrm {id}}_{J})$
cannot occur, because then
$(\gamma _{1}, \gamma _{2}) = ({\mathrm {id}}_{L(J)}, {\mathrm {id}}_{J})$
cannot occur, because then
![]() $\gamma \in {U(J)_{\mathbb {Q}/\mathbb {Z}}}\cap {V(J)_{\mathbb {Z}}}$
and so
$\gamma \in {U(J)_{\mathbb {Q}/\mathbb {Z}}}\cap {V(J)_{\mathbb {Z}}}$
and so
![]() $\gamma =\mathrm {id}$
by (5.10). Therefore,
$\gamma =\mathrm {id}$
by (5.10). Therefore,
![]() $\gamma =(-{\mathrm {id}}_{L(J)}, -{\mathrm {id}}_{J}, E_{w})$
for some
$\gamma =(-{\mathrm {id}}_{L(J)}, -{\mathrm {id}}_{J}, E_{w})$
for some
![]() $w\in \wedge ^{2}J_{{\mathbb {Q}}}$
. Since
$w\in \wedge ^{2}J_{{\mathbb {Q}}}$
. Since
![]() $-{\mathrm {id}}_{L}=(-{\mathrm {id}}_{L(J)}, -{\mathrm {id}}_{J}, 0)$
with respect to this (and any) splitting, we find that
$-{\mathrm {id}}_{L}=(-{\mathrm {id}}_{L(J)}, -{\mathrm {id}}_{J}, 0)$
with respect to this (and any) splitting, we find that
![]() $\gamma =-E_{w}$
. Thus,
$\gamma =-E_{w}$
. Thus,
![]() $-E_{w}\in {\Gamma }$
. Finally, we have
$-E_{w}\in {\Gamma }$
. Finally, we have
![]() $-{\mathrm {id}}\not \in {\Gamma }$
, for otherwise
$-{\mathrm {id}}\not \in {\Gamma }$
, for otherwise
![]() $E_{w}=-\gamma $
would be contained in
$E_{w}=-\gamma $
would be contained in
![]() ${U(J)_{\mathbb {Z}}}$
, which in turn implies that
${U(J)_{\mathbb {Z}}}$
, which in turn implies that
![]() $\gamma $
acts trivially on
$\gamma $
acts trivially on
![]() ${\mathcal {X}(J)}$
.
${\mathcal {X}(J)}$
.
As in the case of zero-dimensional cusps,
![]() $U(J)_{{\mathbb {Z}}}'$
is the projection image of
$U(J)_{{\mathbb {Z}}}'$
is the projection image of
![]() $U(J)_{{\mathbb {Z}}}^{\star }=(\{ \pm {\mathrm {id}} \} \cdot {U(J)_{\mathbb {Q}}})\cap {\Gamma }$
in
$U(J)_{{\mathbb {Z}}}^{\star }=(\{ \pm {\mathrm {id}} \} \cdot {U(J)_{\mathbb {Q}}})\cap {\Gamma }$
in
![]() ${U(J)_{\mathbb {Q}}}$
, and we have
${U(J)_{\mathbb {Q}}}$
, and we have
![]() $U(J)_{{\mathbb {Z}}}^{\star }/{U(J)_{\mathbb {Z}}}=\langle -E_{w} \rangle $
when J is irregular.
$U(J)_{{\mathbb {Z}}}^{\star }/{U(J)_{\mathbb {Z}}}=\langle -E_{w} \rangle $
when J is irregular.
Since the boundary divisor of
![]() ${\overline {\mathcal {X}(J)}}$
is naturally isomorphic to
${\overline {\mathcal {X}(J)}}$
is naturally isomorphic to
![]() ${\mathcal {V}_{J}}$
, the condition
${\mathcal {V}_{J}}$
, the condition
![]() $(4)$
can be restated as follows.
$(4)$
can be restated as follows.
Corollary 6.2 A one-dimensional cusp J is irregular if and only if
![]() ${\overline {\mathcal {X}(J)}}\to {\overline {\mathcal {X}(J)}}/{\overline {\Gamma (J)}_{\mathbb {Z}}}$
is ramified along the boundary divisor of
${\overline {\mathcal {X}(J)}}\to {\overline {\mathcal {X}(J)}}/{\overline {\Gamma (J)}_{\mathbb {Z}}}$
is ramified along the boundary divisor of
![]() ${\overline {\mathcal {X}(J)}}$
. In that case, the ramification index is
${\overline {\mathcal {X}(J)}}$
. In that case, the ramification index is
![]() $2$
, and the unique nontrivial element of
$2$
, and the unique nontrivial element of
![]() ${\overline {\Gamma (J)}_{\mathbb {Z}}}$
fixing the boundary divisor is given by
${\overline {\Gamma (J)}_{\mathbb {Z}}}$
fixing the boundary divisor is given by
![]() $-E_{w}$
.
$-E_{w}$
.
By the condition (1), irregularity of a one-dimensional cusp reduces to that of an adjacent zero-dimensional cusp as follows.
Proposition 6.3 Let
![]() $I\subset J$
be a rank
$I\subset J$
be a rank
![]() $1$
primitive sublattice, and let
$1$
primitive sublattice, and let
![]() $\sigma _{J}\subset U(I)_{{\mathbb {R}}}$
be the isotropic ray corresponding to J. Then J is irregular if and only if I is irregular and
$\sigma _{J}\subset U(I)_{{\mathbb {R}}}$
be the isotropic ray corresponding to J. Then J is irregular if and only if I is irregular and
![]() $\sigma _{J}$
is an irregular ray.
$\sigma _{J}$
is an irregular ray.
Proof Recall from Definition 3.2 that the ray
![]() $\sigma _{J}$
is called irregular when
$\sigma _{J}$
is called irregular when
![]() ${\mathbb {R}}\sigma _{J}\cap {U(I)_{\mathbb {Z}}} \ne {\mathbb {R}}\sigma _{J} \cap {U(I)_{\mathbb {Z}}^{\prime }}$
. By (5.12), we have
${\mathbb {R}}\sigma _{J}\cap {U(I)_{\mathbb {Z}}} \ne {\mathbb {R}}\sigma _{J} \cap {U(I)_{\mathbb {Z}}^{\prime }}$
. By (5.12), we have
![]() ${\mathbb {R}}\sigma _{J}\cap {U(I)_{\mathbb {Z}}} = {U(J)_{\mathbb {Z}}}$
, and similarly
${\mathbb {R}}\sigma _{J}\cap {U(I)_{\mathbb {Z}}} = {U(J)_{\mathbb {Z}}}$
, and similarly
![]() ${\mathbb {R}}\sigma _{J}\cap {U(I)_{\mathbb {Z}}^{\prime }} = {U(J)_{\mathbb {Z}}^{\prime }}$
. This proves our assertion.
${\mathbb {R}}\sigma _{J}\cap {U(I)_{\mathbb {Z}}^{\prime }} = {U(J)_{\mathbb {Z}}^{\prime }}$
. This proves our assertion.
Corollary 6.4 If
![]() ${\Gamma }$
has no irregular zero-dimensional cusp, it has no irregular one-dimensional cusp.
${\Gamma }$
has no irregular zero-dimensional cusp, it has no irregular one-dimensional cusp.
7 Toroidal compactification
In this section, we study singularities and ramification divisors in the boundary of a toroidal compactification of the modular variety. These are studied in [Reference Gritsenko, Hulek and Sankaran7, Reference Ma13] under the condition
![]() $-{\mathrm {id}}\in {\Gamma }$
, and we explain what modification is necessary in the general case, especially at the irregular cusps.
$-{\mathrm {id}}\in {\Gamma }$
, and we explain what modification is necessary in the general case, especially at the irregular cusps.
Let L be a lattice of signature
![]() $(2, b)$
, and let
$(2, b)$
, and let
![]() ${\Gamma }$
be a subgroup of
${\Gamma }$
be a subgroup of
![]() ${\mathrm {O}^{+}(L)}$
of finite index. The input data for constructing a toroidal compactification of
${\mathrm {O}^{+}(L)}$
of finite index. The input data for constructing a toroidal compactification of
![]() ${\mathcal {F}(\Gamma )}={\Gamma }\backslash {\mathcal {D}}$
are a collection
${\mathcal {F}(\Gamma )}={\Gamma }\backslash {\mathcal {D}}$
are a collection
![]() $\Sigma =(\Sigma _{I})_{I}$
of
$\Sigma =(\Sigma _{I})_{I}$
of
![]() ${\Gamma (I)_{\mathbb {Z}}}$
-admissible fans (Section 2.4), one for each
${\Gamma (I)_{\mathbb {Z}}}$
-admissible fans (Section 2.4), one for each
![]() ${\Gamma }$
-equivalence class of rank
${\Gamma }$
-equivalence class of rank
![]() $1$
primitive isotropic sublattices I of L. No choice is required for one-dimensional cusps. Thus,
$1$
primitive isotropic sublattices I of L. No choice is required for one-dimensional cusps. Thus,
![]() $\Sigma $
is a finite collection of independent fans.
$\Sigma $
is a finite collection of independent fans.
The toroidal compactification associated with
![]() $\Sigma $
is defined as ([Reference Ash, Mumford, Rapoport and Tai1, p. 163])
$\Sigma $
is defined as ([Reference Ash, Mumford, Rapoport and Tai1, p. 163])
 $$ \begin{align*} {\mathcal{F}(\Gamma)^{\Sigma}} = \left( {\mathcal{D}} \sqcup \bigsqcup_{I} \mathcal{X}(I)^{\Sigma_{I}} \sqcup \bigsqcup_{J}{\overline{\mathcal{X}(J)}} \right) / \sim, \end{align*} $$
$$ \begin{align*} {\mathcal{F}(\Gamma)^{\Sigma}} = \left( {\mathcal{D}} \sqcup \bigsqcup_{I} \mathcal{X}(I)^{\Sigma_{I}} \sqcup \bigsqcup_{J}{\overline{\mathcal{X}(J)}} \right) / \sim, \end{align*} $$
where I (resp. J) ranges over all primitive isotropic sublattices of L of rank
![]() $1$
(resp.
$1$
(resp.
![]() $2$
), and
$2$
), and
![]() $\sim $
is the equivalence relation generated by the following:
$\sim $
is the equivalence relation generated by the following:
-
• action of
 $\gamma \in {\Gamma }$
giving
$\gamma \in {\Gamma }$
giving
 ${\mathcal {D}}\to {\mathcal {D}}$
,
${\mathcal {D}}\to {\mathcal {D}}$
,
 $\mathcal {X}(I)^{\Sigma _{I}}\to \mathcal {X}(\gamma I)^{\Sigma _{\gamma I}}$
, and
$\mathcal {X}(I)^{\Sigma _{I}}\to \mathcal {X}(\gamma I)^{\Sigma _{\gamma I}}$
, and
 ${\overline {\mathcal {X}(J)}} \to \overline {\mathcal {X}(\gamma J)}$
;
${\overline {\mathcal {X}(J)}} \to \overline {\mathcal {X}(\gamma J)}$
; -
• the natural maps
 ${\mathcal {D}}\to \mathcal {X}(I)^{\Sigma _{I}}$
and
${\mathcal {D}}\to \mathcal {X}(I)^{\Sigma _{I}}$
and
 ${\mathcal {D}}\to {\overline {\mathcal {X}(J)}}$
;
${\mathcal {D}}\to {\overline {\mathcal {X}(J)}}$
; -
• the etale gluing maps
 ${\overline {\mathcal {X}(J)}}\to \mathcal {X}(I)^{\Sigma _{I}}$
for
${\overline {\mathcal {X}(J)}}\to \mathcal {X}(I)^{\Sigma _{I}}$
for
 $I\subset J$
given by (5.13).
$I\subset J$
given by (5.13).
Theorem 7.1 [Reference Ash, Mumford, Rapoport and Tai1]
The space
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
is a compact Moishezon space containing
${\mathcal {F}(\Gamma )^{\Sigma }}$
is a compact Moishezon space containing
![]() ${\mathcal {F}(\Gamma )}$
as a Zariski open set, and we have a morphism from
${\mathcal {F}(\Gamma )}$
as a Zariski open set, and we have a morphism from
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
to the Baily–Borel compactification of
${\mathcal {F}(\Gamma )^{\Sigma }}$
to the Baily–Borel compactification of
![]() ${\mathcal {F}(\Gamma )}$
. For each cusp I, J, the natural map
${\mathcal {F}(\Gamma )}$
. For each cusp I, J, the natural map
is locally isomorphic in an open neighborhood of boundary points lying over that cusp.
Perhaps a word might be in order because, strictly speaking, the theory of [Reference Ash, Mumford, Rapoport and Tai1] is applied to the image of
![]() ${\Gamma }$
in
${\Gamma }$
in
![]() $\mathrm { O}^{+}(L_{{\mathbb {R}}})/\pm {\mathrm {id}}$
, which is
$\mathrm { O}^{+}(L_{{\mathbb {R}}})/\pm {\mathrm {id}}$
, which is
![]() ${\langle \Gamma , -\mathrm {id} \rangle }/\pm {\mathrm {id}}$
, rather than
${\langle \Gamma , -\mathrm {id} \rangle }/\pm {\mathrm {id}}$
, rather than
![]() ${\Gamma }$
itself. Then
${\Gamma }$
itself. Then
![]() ${U(I)_{\mathbb {Z}}}$
should be replaced by
${U(I)_{\mathbb {Z}}}$
should be replaced by
![]() ${U(I)_{\mathbb {Z}}^{\prime }}$
,
${U(I)_{\mathbb {Z}}^{\prime }}$
,
![]() ${\mathcal {X}(I)}={\mathcal {D}}/{U(I)_{\mathbb {Z}}}$
by
${\mathcal {X}(I)}={\mathcal {D}}/{U(I)_{\mathbb {Z}}}$
by
![]() ${\mathcal {X}(I)'}={\mathcal {D}}/{U(I)_{\mathbb {Z}}^{\prime }}$
,
${\mathcal {X}(I)'}={\mathcal {D}}/{U(I)_{\mathbb {Z}}^{\prime }}$
,
![]() ${\Gamma (I)_{\mathbb {Z}}}$
by
${\Gamma (I)_{\mathbb {Z}}}$
by
![]() $\Gamma '(I)_{{\mathbb {Z}}}=\langle {\Gamma (I)_{\mathbb {Z}}}, -{\mathrm {id}} \rangle / \pm {\mathrm {id}}$
, and similarly for one-dimensional cusps J. But since
$\Gamma '(I)_{{\mathbb {Z}}}=\langle {\Gamma (I)_{\mathbb {Z}}}, -{\mathrm {id}} \rangle / \pm {\mathrm {id}}$
, and similarly for one-dimensional cusps J. But since
![]() ${\mathcal {X}(I)'}={\mathcal {X}(I)}$
or
${\mathcal {X}(I)'}={\mathcal {X}(I)}$
or
![]() ${\mathcal {X}(I)'}={\mathcal {X}(I)}/\langle -E_{w} \rangle $
with
${\mathcal {X}(I)'}={\mathcal {X}(I)}/\langle -E_{w} \rangle $
with
![]() $-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
(and similarly for J), we have naturally
$-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
(and similarly for J), we have naturally
 $$ \begin{align*} \left( {\mathcal{D}} \sqcup \bigsqcup_{I} \mathcal{X}(I)^{\Sigma_{I}} \sqcup \bigsqcup_{J}{\overline{\mathcal{X}(J)}} \right) / \sim \; \; = \; \; \left( {\mathcal{D}} \sqcup \bigsqcup_{I} ({\mathcal{X}(I)'})^{\Sigma_{I}} \sqcup \bigsqcup_{J}\overline{\mathcal{X}(J)'} \right) / \sim', \end{align*} $$
$$ \begin{align*} \left( {\mathcal{D}} \sqcup \bigsqcup_{I} \mathcal{X}(I)^{\Sigma_{I}} \sqcup \bigsqcup_{J}{\overline{\mathcal{X}(J)}} \right) / \sim \; \; = \; \; \left( {\mathcal{D}} \sqcup \bigsqcup_{I} ({\mathcal{X}(I)'})^{\Sigma_{I}} \sqcup \bigsqcup_{J}\overline{\mathcal{X}(J)'} \right) / \sim', \end{align*} $$
where
![]() $\sim '$
is the equivalence relation similar to
$\sim '$
is the equivalence relation similar to
![]() $\sim $
. The last statement of Theorem 7.1 [Reference Ash, Mumford, Rapoport and Tai1, p. 175] is justified because we have
$\sim $
. The last statement of Theorem 7.1 [Reference Ash, Mumford, Rapoport and Tai1, p. 175] is justified because we have
(see also (7.1)), and similarly for J.
The reason we prefer to work with
![]() ${U(I)_{\mathbb {Z}}}$
rather than
${U(I)_{\mathbb {Z}}}$
rather than
![]() ${U(I)_{\mathbb {Z}}^{\prime }}$
is that Fourier expansion of
${U(I)_{\mathbb {Z}}^{\prime }}$
is that Fourier expansion of
![]() ${\Gamma }$
-modular forms of arbitrary weight can be done with
${\Gamma }$
-modular forms of arbitrary weight can be done with
![]() ${U(I)_{\mathbb {Z}}}$
(see Section 8).
${U(I)_{\mathbb {Z}}}$
(see Section 8).
If
![]() $D(\sigma )\subset \mathcal {X}(I)^{\Sigma _{I}}$
is the boundary divisor corresponding to a ray
$D(\sigma )\subset \mathcal {X}(I)^{\Sigma _{I}}$
is the boundary divisor corresponding to a ray
![]() $\sigma \in \Sigma _{I}$
, general points of
$\sigma \in \Sigma _{I}$
, general points of
![]() $D(\sigma )$
lie over the I-cusp if and only if
$D(\sigma )$
lie over the I-cusp if and only if
![]() $\sigma $
is positive-definite. When
$\sigma $
is positive-definite. When
![]() $\sigma =\sigma _{J}$
is isotropic corresponding to a one-dimensional cusp
$\sigma =\sigma _{J}$
is isotropic corresponding to a one-dimensional cusp
![]() $J\supset I$
,
$J\supset I$
,
![]() $D(\sigma _{J})$
is glued with the boundary divisor of
$D(\sigma _{J})$
is glued with the boundary divisor of
![]() ${\overline {\mathcal {X}(J)}}$
, and its general points lie over the J-cusp. By combining the last statement of Theorem 7.1 with Corollaries 3.8 and 6.2, we obtain the following.
${\overline {\mathcal {X}(J)}}$
, and its general points lie over the J-cusp. By combining the last statement of Theorem 7.1 with Corollaries 3.8 and 6.2, we obtain the following.
Proposition 7.2 (1) The projection
![]() $\mathcal {X}(I)^{\Sigma _{I}}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is ramified along irregular boundary divisors of
$\mathcal {X}(I)^{\Sigma _{I}}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is ramified along irregular boundary divisors of
![]() $\mathcal {X}(I)^{\Sigma _{I}}$
with ramification index
$\mathcal {X}(I)^{\Sigma _{I}}$
with ramification index
![]() $2$
, and not ramified along other boundary divisors. If we take quotient by
$2$
, and not ramified along other boundary divisors. If we take quotient by
![]() $U(I)_{{\mathbb {Z}}}^{\star }/{U(I)_{\mathbb {Z}}}$
, then
$U(I)_{{\mathbb {Z}}}^{\star }/{U(I)_{\mathbb {Z}}}$
, then
![]() $({\mathcal {D}}/U(I)_{{\mathbb {Z}}}^{\star })^{\Sigma _{I}}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is not ramified along the boundary divisors.
$({\mathcal {D}}/U(I)_{{\mathbb {Z}}}^{\star })^{\Sigma _{I}}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is not ramified along the boundary divisors.
(2) The projection
![]() ${\overline {\mathcal {X}(J)}}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is ramified along the unique boundary divisor (with index
${\overline {\mathcal {X}(J)}}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is ramified along the unique boundary divisor (with index
![]() $2$
) if and only if J is irregular. If we take quotient by
$2$
) if and only if J is irregular. If we take quotient by
![]() $U(J)_{{\mathbb {Z}}}^{\star }/{U(J)_{\mathbb {Z}}}$
, then
$U(J)_{{\mathbb {Z}}}^{\star }/{U(J)_{\mathbb {Z}}}$
, then
![]() $\overline {{\mathcal {D}}/U(J)_{{\mathbb {Z}}}^{\star }}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is not ramified along the boundary divisor.
$\overline {{\mathcal {D}}/U(J)_{{\mathbb {Z}}}^{\star }}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is not ramified along the boundary divisor.
Proof What remains is to show that (1) is still true even when a ray
![]() $\sigma =\sigma _{J}$
is isotropic. Since the map
$\sigma =\sigma _{J}$
is isotropic. Since the map
![]() ${\overline {\mathcal {X}(J)}}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
in (2) factorizes as
${\overline {\mathcal {X}(J)}}\to {\mathcal {F}(\Gamma )^{\Sigma }}$
in (2) factorizes as
![]() ${\overline {\mathcal {X}(J)}}\to \mathcal {X}(I)^{\Sigma _{I}} \to {\mathcal {F}(\Gamma )^{\Sigma }}$
and the gluing map
${\overline {\mathcal {X}(J)}}\to \mathcal {X}(I)^{\Sigma _{I}} \to {\mathcal {F}(\Gamma )^{\Sigma }}$
and the gluing map
![]() ${\overline {\mathcal {X}(J)}}\to \mathcal {X}(I)^{\Sigma _{I}}$
is etale, our assertion for
${\overline {\mathcal {X}(J)}}\to \mathcal {X}(I)^{\Sigma _{I}}$
is etale, our assertion for
![]() $\mathcal {X}(I)^{\Sigma _{I}} \to {\mathcal {F}(\Gamma )^{\Sigma }}$
follows from (2) and Proposition 6.3.
$\mathcal {X}(I)^{\Sigma _{I}} \to {\mathcal {F}(\Gamma )^{\Sigma }}$
follows from (2) and Proposition 6.3.
When
![]() ${\Gamma }$
contains
${\Gamma }$
contains
![]() $-{\mathrm {id}}$
, Proposition 7.2 is proved in [Reference Gritsenko, Hulek and Sankaran7, Reference Ma13]. In that case, we have no irregular cusp, so no ramification divisor in the boundary.
$-{\mathrm {id}}$
, Proposition 7.2 is proved in [Reference Gritsenko, Hulek and Sankaran7, Reference Ma13]. In that case, we have no irregular cusp, so no ramification divisor in the boundary.
Remark 7.3 It appears that in some literatures, the “no ramification boundary divisor” property is used to claim that
![]() $\mathcal {F}(\Gamma ')^{\Sigma }\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is not ramified along the boundary divisors for neat subgroups
$\mathcal {F}(\Gamma ')^{\Sigma }\to {\mathcal {F}(\Gamma )^{\Sigma }}$
is not ramified along the boundary divisors for neat subgroups
![]() $\Gamma ' < {\Gamma }$
. This seems not true already in the case of modular curves: for example,
$\Gamma ' < {\Gamma }$
. This seems not true already in the case of modular curves: for example,
![]() $\Gamma (N) < \mathrm {SL}_{2}({\mathbb {Z}})$
. The point is that
$\Gamma (N) < \mathrm {SL}_{2}({\mathbb {Z}})$
. The point is that
![]() $U(I)_{{\mathbb {Z}},{\Gamma }}={U(I)_{\mathbb {Q}}}\cap {\Gamma }$
depends on
$U(I)_{{\mathbb {Z}},{\Gamma }}={U(I)_{\mathbb {Q}}}\cap {\Gamma }$
depends on
![]() ${\Gamma }$
, so
${\Gamma }$
, so
![]() $U(I)_{{\mathbb {Z}},{\Gamma }'}={U(I)_{\mathbb {Q}}}\cap {\Gamma }'$
is in general smaller than
$U(I)_{{\mathbb {Z}},{\Gamma }'}={U(I)_{\mathbb {Q}}}\cap {\Gamma }'$
is in general smaller than
![]() $U(I)_{{\mathbb {Z}},{\Gamma }}$
. If
$U(I)_{{\mathbb {Z}},{\Gamma }}$
. If
![]() $\sigma $
is a ray in
$\sigma $
is a ray in
![]() $\Sigma _{I}$
, assumed regular for simplicity, we have ramification index
$\Sigma _{I}$
, assumed regular for simplicity, we have ramification index
at the corresponding boundary divisor. It seems that so far, all argument using the above claim can be avoided: see the proof of Theorem 8.9.
Next, we study singularities. A fan
![]() $\Sigma _{I}=(\sigma _{\alpha })$
is called basic with respect to a lattice
$\Sigma _{I}=(\sigma _{\alpha })$
is called basic with respect to a lattice
![]() $\Lambda \subset {U(I)_{\mathbb {Q}}}$
if each cone
$\Lambda \subset {U(I)_{\mathbb {Q}}}$
if each cone
![]() $\sigma _{\alpha }$
is generated by a part of a basis of
$\sigma _{\alpha }$
is generated by a part of a basis of
![]() $\Lambda $
. The singularity theorem [Reference Gritsenko, Hulek and Sankaran7, Reference Ma13] is still true, if we require the fan
$\Lambda $
. The singularity theorem [Reference Gritsenko, Hulek and Sankaran7, Reference Ma13] is still true, if we require the fan
![]() $\Sigma _{I}$
to be basic with respect to
$\Sigma _{I}$
to be basic with respect to
![]() ${U(I)_{\mathbb {Z}}^{\prime }}$
, rather than
${U(I)_{\mathbb {Z}}^{\prime }}$
, rather than
![]() ${U(I)_{\mathbb {Z}}}$
.
${U(I)_{\mathbb {Z}}}$
.
Proposition 7.4 (cf. [Reference Gritsenko, Hulek and Sankaran7, Reference Ma13])
(1) We choose the fans
![]() $\Sigma =(\Sigma _{I})$
so that each
$\Sigma =(\Sigma _{I})$
so that each
![]() $\Sigma _{I}$
is basic with respect to
$\Sigma _{I}$
is basic with respect to
![]() ${U(I)_{\mathbb {Z}}^{\prime }}$
. Then
${U(I)_{\mathbb {Z}}^{\prime }}$
. Then
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
has canonical singularities at the boundary points lying over the zero-dimensional cusps.
${\mathcal {F}(\Gamma )^{\Sigma }}$
has canonical singularities at the boundary points lying over the zero-dimensional cusps.
(2) When
![]() $b\geq 9$
,
$b\geq 9$
,
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
has canonical singularities at the boundary points lying over the one-dimensional cusps.
${\mathcal {F}(\Gamma )^{\Sigma }}$
has canonical singularities at the boundary points lying over the one-dimensional cusps.
Proof When
![]() ${\Gamma }$
contains
${\Gamma }$
contains
![]() $-{\mathrm {id}}$
, this is proved in [Reference Gritsenko, Hulek and Sankaran7, Reference Ma13] for zero-dimensional cusps, and in [Reference Gritsenko, Hulek and Sankaran7] for one-dimensional cusps. We show that the general case is reduced to this case. We consider zero-dimensional cusps. The case of one-dimensional cusps is similar. It suffices to show that
$-{\mathrm {id}}$
, this is proved in [Reference Gritsenko, Hulek and Sankaran7, Reference Ma13] for zero-dimensional cusps, and in [Reference Gritsenko, Hulek and Sankaran7] for one-dimensional cusps. We show that the general case is reduced to this case. We consider zero-dimensional cusps. The case of one-dimensional cusps is similar. It suffices to show that
![]() ${\mathcal {X}(I)}^{\Sigma _{I}}/{\overline {\Gamma (I)}_{\mathbb {Z}}}$
has canonical singularities.
${\mathcal {X}(I)}^{\Sigma _{I}}/{\overline {\Gamma (I)}_{\mathbb {Z}}}$
has canonical singularities.
Let
![]() $\Gamma ' = {\langle \Gamma , -\mathrm {id} \rangle }$
and
$\Gamma ' = {\langle \Gamma , -\mathrm {id} \rangle }$
and
![]() $\Gamma '(I)_{{\mathbb {Z}}}=\Gamma ' \cap {\Gamma (I)_{\mathbb {Q}}}$
. Then
$\Gamma '(I)_{{\mathbb {Z}}}=\Gamma ' \cap {\Gamma (I)_{\mathbb {Q}}}$
. Then
![]() ${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap \Gamma '$
and
${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap \Gamma '$
and
![]() $\Gamma '(I)_{{\mathbb {Z}}} = \langle {\Gamma (I)_{\mathbb {Z}}}, -{\mathrm {id}} \rangle $
. Since the fan
$\Gamma '(I)_{{\mathbb {Z}}} = \langle {\Gamma (I)_{\mathbb {Z}}}, -{\mathrm {id}} \rangle $
. Since the fan
![]() $\Sigma _{I}$
is also rational with respect to
$\Sigma _{I}$
is also rational with respect to
![]() ${U(I)_{\mathbb {Z}}^{\prime }}$
, it defines a toroidal embedding
${U(I)_{\mathbb {Z}}^{\prime }}$
, it defines a toroidal embedding
![]() $({\mathcal {D}}/{U(I)_{\mathbb {Z}}^{\prime }})^{\Sigma _{I}}$
of
$({\mathcal {D}}/{U(I)_{\mathbb {Z}}^{\prime }})^{\Sigma _{I}}$
of
![]() ${\mathcal {D}}/{U(I)_{\mathbb {Z}}^{\prime }}$
. This is the quotient of
${\mathcal {D}}/{U(I)_{\mathbb {Z}}^{\prime }}$
. This is the quotient of
![]() $({\mathcal {D}}/{U(I)_{\mathbb {Z}}})^{\Sigma _{I}}$
by the translation by
$({\mathcal {D}}/{U(I)_{\mathbb {Z}}})^{\Sigma _{I}}$
by the translation by
![]() ${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}$
(which is nontrivial exactly when I is irregular). Since
${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}$
(which is nontrivial exactly when I is irregular). Since
![]() ${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}\subset \Gamma '(I)_{{\mathbb {Z}}}/{U(I)_{\mathbb {Z}}}$
, we have
${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}\subset \Gamma '(I)_{{\mathbb {Z}}}/{U(I)_{\mathbb {Z}}}$
, we have
 $$ \begin{align} ({\mathcal{D}}/{U(I)_{\mathbb{Z}}})^{\Sigma_{I}}/{\overline{\Gamma(I)}_{\mathbb{Z}}} & = ({\mathcal{D}}/{U(I)_{\mathbb{Z}}})^{\Sigma_{I}}/(\Gamma'(I)_{{\mathbb{Z}}}/{U(I)_{\mathbb{Z}}}) \\ \nonumber & \simeq ({\mathcal{D}}/{U(I)_{\mathbb{Z}}^{\prime}})^{\Sigma_{I}}/(\Gamma'(I)_{{\mathbb{Z}}}/{U(I)_{\mathbb{Z}}^{\prime}}). \end{align} $$
$$ \begin{align} ({\mathcal{D}}/{U(I)_{\mathbb{Z}}})^{\Sigma_{I}}/{\overline{\Gamma(I)}_{\mathbb{Z}}} & = ({\mathcal{D}}/{U(I)_{\mathbb{Z}}})^{\Sigma_{I}}/(\Gamma'(I)_{{\mathbb{Z}}}/{U(I)_{\mathbb{Z}}}) \\ \nonumber & \simeq ({\mathcal{D}}/{U(I)_{\mathbb{Z}}^{\prime}})^{\Sigma_{I}}/(\Gamma'(I)_{{\mathbb{Z}}}/{U(I)_{\mathbb{Z}}^{\prime}}). \end{align} $$
Since
![]() $\Sigma _{I}$
is basic with respect to
$\Sigma _{I}$
is basic with respect to
![]() ${U(I)_{\mathbb {Z}}^{\prime }}$
and
${U(I)_{\mathbb {Z}}^{\prime }}$
and
![]() $-{\mathrm {id}}\in \Gamma '$
, we can apply the result of [Reference Ma13] to the last quotient to see that this has canonical singularities.
$-{\mathrm {id}}\in \Gamma '$
, we can apply the result of [Reference Ma13] to the last quotient to see that this has canonical singularities.
8 Modular forms and pluricanonical forms
Let L be a lattice of signature
![]() $(2, b)$
, and let
$(2, b)$
, and let
![]() ${\Gamma }$
be a subgroup of
${\Gamma }$
be a subgroup of
![]() ${\mathrm {O}^{+}(L)}$
of finite index. For simplicity, we assume
${\mathrm {O}^{+}(L)}$
of finite index. For simplicity, we assume
![]() $b\geq 3$
. In this section, we compare the vanishing order of cusp forms and pluricanonical forms, and explain how the low weight cusp form trick of Gritsenko, Hulek, and Sankaran [Reference Gritsenko, Hulek and Sankaran7] is modified at irregular boundary divisors. We take this occasion to generalize “low weight” to “low slope,” for possible future use.
$b\geq 3$
. In this section, we compare the vanishing order of cusp forms and pluricanonical forms, and explain how the low weight cusp form trick of Gritsenko, Hulek, and Sankaran [Reference Gritsenko, Hulek and Sankaran7] is modified at irregular boundary divisors. We take this occasion to generalize “low weight” to “low slope,” for possible future use.
8.1 Modular forms
Let
![]() $\mathcal {L}=\mathcal {O}_{{{\mathbb P}}L_{{\mathbb {C}}}}(-1)|_{{\mathcal {D}}}$
be the restriction of the tautological line bundle to
$\mathcal {L}=\mathcal {O}_{{{\mathbb P}}L_{{\mathbb {C}}}}(-1)|_{{\mathcal {D}}}$
be the restriction of the tautological line bundle to
![]() ${\mathcal {D}}\subset {{\mathbb P}}L_{{\mathbb {C}}}$
. Let
${\mathcal {D}}\subset {{\mathbb P}}L_{{\mathbb {C}}}$
. Let
![]() $\chi $
be a character of
$\chi $
be a character of
![]() ${\Gamma }$
. By our assumption
${\Gamma }$
. By our assumption
![]() $b\geq 3$
,
$b\geq 3$
,
![]() $\chi ({\Gamma })\subset {\mathbb {C}}^{\times }$
is finite [Reference Margulis14]. We assume that
$\chi ({\Gamma })\subset {\mathbb {C}}^{\times }$
is finite [Reference Margulis14]. We assume that
![]() $\chi |_{{U(I)_{\mathbb {Z}}}}\equiv 1$
for every zero-dimensional cusp I. This holds, e.g., for
$\chi |_{{U(I)_{\mathbb {Z}}}}\equiv 1$
for every zero-dimensional cusp I. This holds, e.g., for
![]() $\chi =1, \det $
. A
$\chi =1, \det $
. A
![]() ${\Gamma }$
-invariant section of the
${\Gamma }$
-invariant section of the
![]() ${\Gamma }$
-linearized line bundle
${\Gamma }$
-linearized line bundle
![]() $\mathcal {L}^{\otimes k}\otimes \chi $
over
$\mathcal {L}^{\otimes k}\otimes \chi $
over
![]() ${\mathcal {D}}$
is called a modular form of weight k and character
${\mathcal {D}}$
is called a modular form of weight k and character
![]() $\chi $
with respect to
$\chi $
with respect to
![]() ${\Gamma }$
.
${\Gamma }$
.
Let I be a rank
![]() $1$
primitive isotropic sublattice of L. We choose a generator
$1$
primitive isotropic sublattice of L. We choose a generator
![]() $l_{I}$
of I. This defines a frame
$l_{I}$
of I. This defines a frame
![]() $s_{I}$
of
$s_{I}$
of
![]() $\mathcal {L}$
determined by the condition
$\mathcal {L}$
determined by the condition
![]() $(s_{I}([\omega ]), l_{I})=1$
, where we view
$(s_{I}([\omega ]), l_{I})=1$
, where we view
![]() $s_{I}([\omega ])\in \mathcal {L}_{[\omega ]}={\mathbb {C}}\omega \subset L_{{\mathbb {C}}}$
. The factor of automorphy with respect to
$s_{I}([\omega ])\in \mathcal {L}_{[\omega ]}={\mathbb {C}}\omega \subset L_{{\mathbb {C}}}$
. The factor of automorphy with respect to
![]() $s_{I}$
is given by
$s_{I}$
is given by
 $$ \begin{align} j(\gamma, [\omega]) = \frac{(\gamma\omega, l_{I})}{(\omega, l_{I})} = \frac{(\omega, \gamma^{-1}l_{I})}{(\omega, l_{I})} , \qquad \gamma\in {\Gamma}, \; [\omega]\in {\mathcal{D}}. \end{align} $$
$$ \begin{align} j(\gamma, [\omega]) = \frac{(\gamma\omega, l_{I})}{(\omega, l_{I})} = \frac{(\omega, \gamma^{-1}l_{I})}{(\omega, l_{I})} , \qquad \gamma\in {\Gamma}, \; [\omega]\in {\mathcal{D}}. \end{align} $$
Let
![]() $1_{\chi }$
be a nonzero vector in the representation line of
$1_{\chi }$
be a nonzero vector in the representation line of
![]() $\chi $
. Then
$\chi $
. Then
![]() $s_{I}^{\otimes k}\otimes 1_{\chi }$
is a frame of the line bundle
$s_{I}^{\otimes k}\otimes 1_{\chi }$
is a frame of the line bundle
![]() $\mathcal {L}^{\otimes k}\otimes \chi $
, via which modular forms
$\mathcal {L}^{\otimes k}\otimes \chi $
, via which modular forms
![]() $F=f s_{I}^{\otimes k}\otimes 1_{\chi }$
of weight k and character
$F=f s_{I}^{\otimes k}\otimes 1_{\chi }$
of weight k and character
![]() $\chi $
are identified with holomorphic functions f on
$\chi $
are identified with holomorphic functions f on
![]() ${\mathcal {D}}$
satisfying
${\mathcal {D}}$
satisfying
Since
![]() $s_{I}^{\otimes k}\otimes 1_{\chi }$
is invariant under
$s_{I}^{\otimes k}\otimes 1_{\chi }$
is invariant under
![]() ${U(I)_{\mathbb {Z}}}$
by our assumption, f is
${U(I)_{\mathbb {Z}}}$
by our assumption, f is
![]() ${U(I)_{\mathbb {Z}}}$
-invariant, hence descends to a function on
${U(I)_{\mathbb {Z}}}$
-invariant, hence descends to a function on
![]() ${\mathcal {D}}/{U(I)_{\mathbb {Z}}}$
. By the tube domain realization
${\mathcal {D}}/{U(I)_{\mathbb {Z}}}$
. By the tube domain realization
![]() ${\mathcal {D}}\to {\mathcal {D}}_{I}\subset U(I)_{{\mathbb {C}}}$
(after a choice of
${\mathcal {D}}\to {\mathcal {D}}_{I}\subset U(I)_{{\mathbb {C}}}$
(after a choice of
![]() $I'\subset L$
with
$I'\subset L$
with
![]() $(I, I')\not \equiv 0$
), f is identified with a function on
$(I, I')\not \equiv 0$
), f is identified with a function on
![]() ${\mathcal {D}}_{I}$
invariant under translation by the lattice
${\mathcal {D}}_{I}$
invariant under translation by the lattice
![]() ${U(I)_{\mathbb {Z}}}$
. Then it admits a Fourier expansion
${U(I)_{\mathbb {Z}}}$
. Then it admits a Fourier expansion
By the Koecher principle, we have
![]() $a(l)\ne 0$
only when
$a(l)\ne 0$
only when
![]() $l\in \overline {{\mathcal {C}_{I}}}$
. The modular form F is called a cusp form if
$l\in \overline {{\mathcal {C}_{I}}}$
. The modular form F is called a cusp form if
![]() $a(l)=0$
for every
$a(l)=0$
for every
![]() $l\in U(I)_{{\mathbb {Z}}}^{\vee }$
with
$l\in U(I)_{{\mathbb {Z}}}^{\vee }$
with
![]() $(l, l)=0$
at every rank
$(l, l)=0$
at every rank
![]() $1$
primitive isotropic sublattice I of L. (
$1$
primitive isotropic sublattice I of L. (
![]() $a(0)$
is the value of f at the zero-dimensional cusp for I, and
$a(0)$
is the value of f at the zero-dimensional cusp for I, and
![]() $\sum _{\sigma \cap U(I)_{{\mathbb {Z}}}^{\vee }}a(l)q^{l}$
for an isotropic ray
$\sum _{\sigma \cap U(I)_{{\mathbb {Z}}}^{\vee }}a(l)q^{l}$
for an isotropic ray
![]() $\sigma =\sigma _{J}$
gives the restriction of f to the one-dimensional cusp for
$\sigma =\sigma _{J}$
gives the restriction of f to the one-dimensional cusp for
![]() $J\supset I$
.)
$J\supset I$
.)
Fourier expansion at an irregular cusp satisfies the following.
Lemma 8.1 Suppose that I is irregular and
![]() $-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
. When the weight k satisfies
$-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
. When the weight k satisfies
![]() $\chi (-E_{w})=(-1)^{k+1}$
, e.g., k odd for
$\chi (-E_{w})=(-1)^{k+1}$
, e.g., k odd for
![]() $\chi =1$
or
$\chi =1$
or
![]() $k\not \equiv b$
mod
$k\not \equiv b$
mod
![]() $2$
for
$2$
for
![]() $\chi =\det $
, then we have
$\chi =\det $
, then we have
![]() $a(l)=0$
for
$a(l)=0$
for
![]() $l\in (U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
. In particular,
$l\in (U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
. In particular,
![]() $a(0)=0$
in this case. When
$a(0)=0$
in this case. When
![]() $\chi (-E_{w})=(-1)^{k}$
, we have
$\chi (-E_{w})=(-1)^{k}$
, we have
![]() $a(l)=0$
for
$a(l)=0$
for
![]() $l\not \in (U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
.
$l\not \in (U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
.
Proof Since
![]() $-E_{w}$
acts on I by
$-E_{w}$
acts on I by
![]() $-1$
, the factor of automorphy of
$-1$
, the factor of automorphy of
![]() $-E_{w}$
on
$-E_{w}$
on
![]() $\mathcal {L}$
is
$\mathcal {L}$
is
![]() $-1$
by (8.1). Therefore, we find that
$-1$
by (8.1). Therefore, we find that
![]() $f(Z+w)=\chi (-E_{w})(-1)^{k}f(Z)$
. Thus, we have
$f(Z+w)=\chi (-E_{w})(-1)^{k}f(Z)$
. Thus, we have
![]() ${f(Z+w)=-f(Z)}$
when
${f(Z+w)=-f(Z)}$
when
![]() $\chi (-E_{w})=(-1)^{k+1}$
, while
$\chi (-E_{w})=(-1)^{k+1}$
, while
![]() $f(Z+w)=f(Z)$
when
$f(Z+w)=f(Z)$
when
![]() ${\chi (-E_{w})=(-1)^{k}}$
.
${\chi (-E_{w})=(-1)^{k}}$
.
On the other hand, since w generates
![]() ${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}\simeq {\mathbb {Z}}/2$
by Proposition 3.1, pairing with w defines an isomorphism
${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}\simeq {\mathbb {Z}}/2$
by Proposition 3.1, pairing with w defines an isomorphism
![]() $U(I)_{{\mathbb {Z}}}^{\vee }/({U(I)_{\mathbb {Z}}^{\prime }})^{\vee } \to \frac {1}{2}{\mathbb {Z}}/{\mathbb {Z}}$
. Thus, we have
$U(I)_{{\mathbb {Z}}}^{\vee }/({U(I)_{\mathbb {Z}}^{\prime }})^{\vee } \to \frac {1}{2}{\mathbb {Z}}/{\mathbb {Z}}$
. Thus, we have
![]() $(l, w)\in {\mathbb {Z}}$
for
$(l, w)\in {\mathbb {Z}}$
for
![]() $l\in (U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
, while
$l\in (U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
, while
![]() $(l, w)\in 1/2+{\mathbb {Z}}$
for
$(l, w)\in 1/2+{\mathbb {Z}}$
for
![]() $l\in U(I)_{{\mathbb {Z}}}^{\vee }-(U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
. Therefore, if we substitute
$l\in U(I)_{{\mathbb {Z}}}^{\vee }-(U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
. Therefore, if we substitute
![]() $Z\to Z+w$
into
$Z\to Z+w$
into
![]() $q^{l}=\mathrm {exp}(2\pi i(l, Z))$
, then
$q^{l}=\mathrm {exp}(2\pi i(l, Z))$
, then
![]() $q^{l}\to q^{l}$
if
$q^{l}\to q^{l}$
if
![]() $l\in (U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
and
$l\in (U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
and
![]() $q^{l}\to -q^{l}$
if
$q^{l}\to -q^{l}$
if
![]() $l\in U(I)_{{\mathbb {Z}}}^{\vee }-(U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
. This implies our assertion.
$l\in U(I)_{{\mathbb {Z}}}^{\vee }-(U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
. This implies our assertion.
8.2 Vanishing order
In this subsection, we study the vanishing order of modular forms along boundary divisors. We will define two types of vanishing order:
![]() $\nu _{\sigma }(F)$
and
$\nu _{\sigma }(F)$
and
![]() $\nu _{\sigma , geom}(F)$
.
$\nu _{\sigma , geom}(F)$
.
![]() $\nu _{\sigma }(F)$
is defined by Fourier expansion and is always an integer. On the other hand,
$\nu _{\sigma }(F)$
is defined by Fourier expansion and is always an integer. On the other hand,
![]() $\nu _{\sigma , geom}(F)$
can be strictly half-integral, and measures the vanishing order at the level of
$\nu _{\sigma , geom}(F)$
can be strictly half-integral, and measures the vanishing order at the level of
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
.
${\mathcal {F}(\Gamma )^{\Sigma }}$
.
Let I be a rank
![]() $1$
primitive isotropic sublattice of L. Let
$1$
primitive isotropic sublattice of L. Let
![]() $\Sigma =\Sigma _{I}=(\sigma _{\alpha })$
be a
$\Sigma =\Sigma _{I}=(\sigma _{\alpha })$
be a
![]() ${\Gamma (I)_{\mathbb {Z}}}$
-admissible fan in
${\Gamma (I)_{\mathbb {Z}}}$
-admissible fan in
![]() $U(I)_{{\mathbb {R}}}$
, and let
$U(I)_{{\mathbb {R}}}$
, and let
![]() $\sigma $
be a ray in
$\sigma $
be a ray in
![]() $\Sigma $
. Let
$\Sigma $
. Let
![]() $w_{\sigma }$
be the generator of
$w_{\sigma }$
be the generator of
![]() $\sigma \cap {U(I)_{\mathbb {Z}}}$
. Let
$\sigma \cap {U(I)_{\mathbb {Z}}}$
. Let
![]() $f(Z)=\sum _{l\in U(I)_{{\mathbb {Z}}}^{\vee }}a(l)q^{l}$
be the Fourier expansion of a
$f(Z)=\sum _{l\in U(I)_{{\mathbb {Z}}}^{\vee }}a(l)q^{l}$
be the Fourier expansion of a
![]() ${\Gamma }$
-modular form
${\Gamma }$
-modular form
![]() ${F=f s_{I}^{\otimes k}\otimes 1_{\chi }}$
around I. We define the vanishing order of F along
${F=f s_{I}^{\otimes k}\otimes 1_{\chi }}$
around I. We define the vanishing order of F along
![]() $\sigma $
as
$\sigma $
as
This is a nonnegative integer because
![]() $w_{\sigma }\in {\mathcal {C}_{I}}$
has nonnegative pairing with
$w_{\sigma }\in {\mathcal {C}_{I}}$
has nonnegative pairing with
![]() $\overline {{\mathcal {C}_{I}}}$
. Clearly,
$\overline {{\mathcal {C}_{I}}}$
. Clearly,
![]() $\nu _{\sigma }(F)$
depends on
$\nu _{\sigma }(F)$
depends on
![]() ${U(I)_{\mathbb {Z}}}$
and hence on
${U(I)_{\mathbb {Z}}}$
and hence on
![]() ${\Gamma }$
. If we shrink
${\Gamma }$
. If we shrink
![]() ${\Gamma }$
without changing F and
${\Gamma }$
without changing F and
![]() $\sigma $
, then
$\sigma $
, then
![]() $\nu _{\sigma }(F)$
will be multiplied in general.
$\nu _{\sigma }(F)$
will be multiplied in general.
When
![]() $\sigma $
is positive-definite, we have
$\sigma $
is positive-definite, we have
![]() $\sigma ^{\perp }\cap \overline {{\mathcal {C}_{I}}} = \{ 0 \}$
, and so
$\sigma ^{\perp }\cap \overline {{\mathcal {C}_{I}}} = \{ 0 \}$
, and so
![]() $l=0$
is the only vector in
$l=0$
is the only vector in
![]() $\overline {{\mathcal {C}_{I}}}$
with
$\overline {{\mathcal {C}_{I}}}$
with
![]() $(l, w_{\sigma })=0$
. Therefore, for such
$(l, w_{\sigma })=0$
. Therefore, for such
![]() $\sigma $
, we have
$\sigma $
, we have
![]() $\nu _{\sigma }(F)>0$
if and only if
$\nu _{\sigma }(F)>0$
if and only if
![]() $a(0)=0$
. Similarly, when
$a(0)=0$
. Similarly, when
![]() $\sigma $
is isotropic, we have
$\sigma $
is isotropic, we have
![]() $\sigma ^{\perp }\cap \overline {{\mathcal {C}_{I}}} = \sigma $
. Therefore, in this case, we have
$\sigma ^{\perp }\cap \overline {{\mathcal {C}_{I}}} = \sigma $
. Therefore, in this case, we have
![]() $\nu _{\sigma }(F)>0$
if and only if
$\nu _{\sigma }(F)>0$
if and only if
![]() $a(l)=0$
for all
$a(l)=0$
for all
![]() $l\in \sigma \cap U(I)_{{\mathbb {Z}}}^{\vee }$
. Thus, F is a cusp form if and only if
$l\in \sigma \cap U(I)_{{\mathbb {Z}}}^{\vee }$
. Thus, F is a cusp form if and only if
![]() $\nu _{\sigma }(F)>0$
at every ray
$\nu _{\sigma }(F)>0$
at every ray
![]() $\sigma $
at every zero-dimensional cusp I.
$\sigma $
at every zero-dimensional cusp I.
The following criterion is trivial but perhaps might be sometimes useful in view of Theorem 8.9. Compare with [Reference Ash, Mumford, Rapoport and Tai1, Reference Freitag5] in related cases.
Corollary 8.2 Assume that the following holds: if
![]() $a(l)\ne 0$
, then
$a(l)\ne 0$
, then
![]() $(l, w)\geq r$
for every
$(l, w)\geq r$
for every
![]() $w\in {U(I)_{\mathbb {Z}}}\cap \overline {{\mathcal {C}_{I}}}$
. Then we have
$w\in {U(I)_{\mathbb {Z}}}\cap \overline {{\mathcal {C}_{I}}}$
. Then we have
![]() $\nu _{\sigma }(F)\geq r$
for every ray
$\nu _{\sigma }(F)\geq r$
for every ray
![]() $\sigma \in \Sigma $
.
$\sigma \in \Sigma $
.
Proof Take w to be the generator of
![]() $\sigma \cap {U(I)_{\mathbb {Z}}}$
.
$\sigma \cap {U(I)_{\mathbb {Z}}}$
.
When
![]() $\sigma $
is irregular,
$\sigma $
is irregular,
![]() $\nu _{\sigma }(F)$
belongs to the following parity.
$\nu _{\sigma }(F)$
belongs to the following parity.
Proposition 8.3 Suppose that the ray
![]() $\sigma $
is irregular and
$\sigma $
is irregular and
![]() $-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
. Then
$-E_{w}\in {\Gamma (I)_{\mathbb {Z}}}$
. Then
![]() $\nu _{\sigma }(F)$
is odd when
$\nu _{\sigma }(F)$
is odd when
![]() $\chi (-E_{w})=(-1)^{k+1}$
, and even when
$\chi (-E_{w})=(-1)^{k+1}$
, and even when
![]() $\chi (-E_{w})=(-1)^{k}$
.
$\chi (-E_{w})=(-1)^{k}$
.
Proof Let
![]() $w_{\sigma }$
be the generator of
$w_{\sigma }$
be the generator of
![]() $\sigma \cap {U(I)_{\mathbb {Z}}}$
. Since
$\sigma \cap {U(I)_{\mathbb {Z}}}$
. Since
![]() ${U(I)_{\mathbb {Z}}^{\prime }}=\langle {U(I)_{\mathbb {Z}}}, w_{\sigma }/2 \rangle $
, a vector l of
${U(I)_{\mathbb {Z}}^{\prime }}=\langle {U(I)_{\mathbb {Z}}}, w_{\sigma }/2 \rangle $
, a vector l of
![]() $U(I)_{{\mathbb {Z}}}^{\vee }$
belongs to
$U(I)_{{\mathbb {Z}}}^{\vee }$
belongs to
![]() $(U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
if and only if
$(U(I)_{{\mathbb {Z}}}^{\prime })^{\vee }$
if and only if
![]() $(l, w_{\sigma })$
is even. Then our assertion follows from Lemma 8.1.
$(l, w_{\sigma })$
is even. Then our assertion follows from Lemma 8.1.
We also define the geometric vanishing order of F along
![]() $\sigma $
as
$\sigma $
as
 $$ \begin{align*} \nu_{\sigma, geom}(F) = \begin{cases} \nu_{\sigma}(F), & \sigma : \textrm{regular}, \\ \frac{1}{2}\nu_{\sigma}(F), & \sigma : \textrm{irregular}. \end{cases} \end{align*} $$
$$ \begin{align*} \nu_{\sigma, geom}(F) = \begin{cases} \nu_{\sigma}(F), & \sigma : \textrm{regular}, \\ \frac{1}{2}\nu_{\sigma}(F), & \sigma : \textrm{irregular}. \end{cases} \end{align*} $$
If
![]() $w_{\sigma }^{\prime }$
is the generator of
$w_{\sigma }^{\prime }$
is the generator of
![]() $\sigma \cap {U(I)_{\mathbb {Z}}^{\prime }}$
, we can write uniformly as
$\sigma \cap {U(I)_{\mathbb {Z}}^{\prime }}$
, we can write uniformly as
Note that
![]() $\nu _{\sigma , geom}(F)$
is in
$\nu _{\sigma , geom}(F)$
is in
![]() $1/2+{\mathbb {Z}}$
when
$1/2+{\mathbb {Z}}$
when
![]() $\sigma $
is irregular and the weight k satisfies
$\sigma $
is irregular and the weight k satisfies
![]() $\chi (-E_{w})=(-1)^{k+1}$
so that
$\chi (-E_{w})=(-1)^{k+1}$
so that
![]() $\nu _{\sigma }(F)$
is odd.
$\nu _{\sigma }(F)$
is odd.
Geometric interpretation of
![]() $\nu _{\sigma }(F)$
is as follows. Recall that the ray
$\nu _{\sigma }(F)$
is as follows. Recall that the ray
![]() $\sigma $
corresponds to a boundary divisor
$\sigma $
corresponds to a boundary divisor
![]() $D(\sigma )$
of the partial compactification
$D(\sigma )$
of the partial compactification
![]() ${\mathcal {X}(I)^{\Sigma }}$
of
${\mathcal {X}(I)^{\Sigma }}$
of
![]() ${\mathcal {X}(I)}={\mathcal {D}}/{U(I)_{\mathbb {Z}}}$
. The line bundle
${\mathcal {X}(I)}={\mathcal {D}}/{U(I)_{\mathbb {Z}}}$
. The line bundle
![]() $\mathcal {L}^{\otimes k} \otimes \chi $
descends to a line bundle over
$\mathcal {L}^{\otimes k} \otimes \chi $
descends to a line bundle over
![]() ${\mathcal {X}(I)}$
, again denoted by
${\mathcal {X}(I)}$
, again denoted by
![]() $\mathcal {L}^{\otimes k} \otimes \chi $
. The point is that, since
$\mathcal {L}^{\otimes k} \otimes \chi $
. The point is that, since
![]() $s_{I}^{\otimes k}\otimes 1_{\chi }$
is
$s_{I}^{\otimes k}\otimes 1_{\chi }$
is
![]() ${U(I)_{\mathbb {Z}}}$
-invariant, it descends to a frame of
${U(I)_{\mathbb {Z}}}$
-invariant, it descends to a frame of
![]() $\mathcal {L}^{\otimes k} \otimes \chi $
over
$\mathcal {L}^{\otimes k} \otimes \chi $
over
![]() ${\mathcal {X}(I)}$
, and we use this frame to extend
${\mathcal {X}(I)}$
, and we use this frame to extend
![]() $\mathcal {L}^{\otimes k} \otimes \chi $
to a line bundle over
$\mathcal {L}^{\otimes k} \otimes \chi $
to a line bundle over
![]() ${\mathcal {X}(I)^{\Sigma }}$
, still denoted by the same notation. Namely,
${\mathcal {X}(I)^{\Sigma }}$
, still denoted by the same notation. Namely,
![]() $s_{I}^{\otimes k}\otimes 1_{\chi }$
extends to a frame of the extended line bundle by definition. The property
$s_{I}^{\otimes k}\otimes 1_{\chi }$
extends to a frame of the extended line bundle by definition. The property
![]() $l \in \overline {{\mathcal {C}_{I}}}=\overline {{\mathcal {C}_{I}}}^{\vee }$
in the Fourier expansion implies that a modular form F extends holomorphically over
$l \in \overline {{\mathcal {C}_{I}}}=\overline {{\mathcal {C}_{I}}}^{\vee }$
in the Fourier expansion implies that a modular form F extends holomorphically over
![]() ${\mathcal {X}(I)^{\Sigma }}$
as a section of
${\mathcal {X}(I)^{\Sigma }}$
as a section of
![]() $\mathcal {L}^{\otimes k}\otimes \chi $
.
$\mathcal {L}^{\otimes k}\otimes \chi $
.
Proposition 8.4
![]() $\nu _{\sigma }(F)$
is equal to the vanishing order of F as a section of
$\nu _{\sigma }(F)$
is equal to the vanishing order of F as a section of
![]() $\mathcal {L}^{\otimes k} \otimes \chi $
over
$\mathcal {L}^{\otimes k} \otimes \chi $
over
![]() ${\mathcal {X}(I)^{\Sigma }}$
along the boundary divisor
${\mathcal {X}(I)^{\Sigma }}$
along the boundary divisor
![]() $D(\sigma )$
.
$D(\sigma )$
.
Proof Recall that
![]() $\sigma $
defines a sub-toroidal embedding
$\sigma $
defines a sub-toroidal embedding
![]() $\mathcal {X}(I)^{\sigma }\subset {\mathcal {X}(I)^{\Sigma }}$
, the unique boundary divisor of which is a Zariski open set of
$\mathcal {X}(I)^{\sigma }\subset {\mathcal {X}(I)^{\Sigma }}$
, the unique boundary divisor of which is a Zariski open set of
![]() $D(\sigma )$
and is the quotient torus (or its analytic open set) defined by the quotient lattice
$D(\sigma )$
and is the quotient torus (or its analytic open set) defined by the quotient lattice
![]() ${U(I)_{\mathbb {Z}}}/{\mathbb {Z}}w_{\sigma }$
. The character group of this boundary torus is
${U(I)_{\mathbb {Z}}}/{\mathbb {Z}}w_{\sigma }$
. The character group of this boundary torus is
![]() $\sigma ^{\perp }\cap U(I)_{{\mathbb {Z}}}^{\vee }$
. We choose a vector
$\sigma ^{\perp }\cap U(I)_{{\mathbb {Z}}}^{\vee }$
. We choose a vector
![]() $l_{\sigma } \in U(I)_{{\mathbb {Z}}}^{\vee }$
such that
$l_{\sigma } \in U(I)_{{\mathbb {Z}}}^{\vee }$
such that
![]() $(l_{\sigma }, w_{\sigma })=1$
and put
$(l_{\sigma }, w_{\sigma })=1$
and put
![]() $q=q^{l_{\sigma }}$
, which is a character of
$q=q^{l_{\sigma }}$
, which is a character of
![]() $T(I)$
. Then q extends holomorphically over
$T(I)$
. Then q extends holomorphically over
![]() $\mathcal {X}(I)^{\sigma }$
with
$\mathcal {X}(I)^{\sigma }$
with
![]() $D(\sigma )=(q=0)$
. The Fourier expansion (8.2) can be arranged as
$D(\sigma )=(q=0)$
. The Fourier expansion (8.2) can be arranged as
![]() $f=\sum _{m\geq 0}\varphi _{m}q^{m}$
where
$f=\sum _{m\geq 0}\varphi _{m}q^{m}$
where
This is a Taylor expansion of f along the divisor
![]() $D(\sigma )$
. Since
$D(\sigma )$
. Since
![]() $(l+ml_{\sigma }, w_{\sigma })=m$
for
$(l+ml_{\sigma }, w_{\sigma })=m$
for
![]() $l\in \sigma ^{\perp }\cap U(I)_{{\mathbb {Z}}}^{\vee }$
, we find that
$l\in \sigma ^{\perp }\cap U(I)_{{\mathbb {Z}}}^{\vee }$
, we find that
This proves our assertion.
We can also give a geometric interpretation of
![]() $\nu _{\sigma , geom}(F)$
when
$\nu _{\sigma , geom}(F)$
when
This holds, e.g., when k is even with
![]() $\chi =1$
and when
$\chi =1$
and when
![]() $k\equiv b$
mod
$k\equiv b$
mod
![]() $2$
with
$2$
with
![]() $\chi =\det $
. Recall that
$\chi =\det $
. Recall that
![]() ${U(I)_{\mathbb {Z}}^{\prime }}$
is the image of
${U(I)_{\mathbb {Z}}^{\prime }}$
is the image of
![]() $U(I)_{{\mathbb {Z}}}^{\star }$
in
$U(I)_{{\mathbb {Z}}}^{\star }$
in
![]() ${U(I)_{\mathbb {Q}}}$
. Under the condition (8.4), the function
${U(I)_{\mathbb {Q}}}$
. Under the condition (8.4), the function
![]() $f(Z)$
on the tube domain
$f(Z)$
on the tube domain
![]() $\mathcal {D}_{I}$
is invariant under translation by
$\mathcal {D}_{I}$
is invariant under translation by
![]() ${U(I)_{\mathbb {Z}}^{\prime }}$
, so the index lattice in the Fourier expansion reduces to
${U(I)_{\mathbb {Z}}^{\prime }}$
, so the index lattice in the Fourier expansion reduces to
![]() $({U(I)_{\mathbb {Z}}^{\prime }})^{\vee }\subset U(I)_{{\mathbb {Z}}}^{\vee }$
. In other words,
$({U(I)_{\mathbb {Z}}^{\prime }})^{\vee }\subset U(I)_{{\mathbb {Z}}}^{\vee }$
. In other words,
![]() $a(l)=0$
if
$a(l)=0$
if
![]() $l\not \in ({U(I)_{\mathbb {Z}}^{\prime }})^{\vee }$
, so
$l\not \in ({U(I)_{\mathbb {Z}}^{\prime }})^{\vee }$
, so
![]() $\nu _{\sigma , geom}(F)$
is an integer. The frame
$\nu _{\sigma , geom}(F)$
is an integer. The frame
![]() $s_{I}^{\otimes k}\otimes 1_{\chi }$
descends to a frame of
$s_{I}^{\otimes k}\otimes 1_{\chi }$
descends to a frame of
![]() $\mathcal {L}^{\otimes k}\otimes \chi $
over
$\mathcal {L}^{\otimes k}\otimes \chi $
over
using which we can extend
![]() $\mathcal {L}^{\otimes k}\otimes \chi $
to a line bundle over
$\mathcal {L}^{\otimes k}\otimes \chi $
to a line bundle over
![]() ${(\mathcal {X}(I)')^{\Sigma }}$
. The ray
${(\mathcal {X}(I)')^{\Sigma }}$
. The ray
![]() $\sigma $
corresponds to a boundary divisor
$\sigma $
corresponds to a boundary divisor
![]() $D(\sigma )'$
of
$D(\sigma )'$
of
![]() ${(\mathcal {X}(I)')^{\Sigma }}$
. We have
${(\mathcal {X}(I)')^{\Sigma }}$
. We have
-
•
 $D(\sigma )'=D(\sigma )$
in
$D(\sigma )'=D(\sigma )$
in
 ${\mathcal {X}(I)^{\Sigma }}={(\mathcal {X}(I)')^{\Sigma }}$
when I is regular.
${\mathcal {X}(I)^{\Sigma }}={(\mathcal {X}(I)')^{\Sigma }}$
when I is regular. -
•
 $D(\sigma )'\simeq D(\sigma )$
with
$D(\sigma )'\simeq D(\sigma )$
with
 ${\mathcal {X}(I)^{\Sigma }}\to {(\mathcal {X}(I)')^{\Sigma }}$
doubly ramified along
${\mathcal {X}(I)^{\Sigma }}\to {(\mathcal {X}(I)')^{\Sigma }}$
doubly ramified along
 $D(\sigma )'$
when
$D(\sigma )'$
when
 $\sigma $
is irregular.
$\sigma $
is irregular. -
•
 $D(\sigma )'$
is the quotient of
$D(\sigma )'$
is the quotient of
 $D(\sigma )$
by
$D(\sigma )$
by
 ${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}\simeq {\mathbb {Z}}/2$
with
${U(I)_{\mathbb {Z}}^{\prime }}/{U(I)_{\mathbb {Z}}}\simeq {\mathbb {Z}}/2$
with
 ${\mathcal {X}(I)^{\Sigma }}\to {(\mathcal {X}(I)')^{\Sigma }}$
unramified along
${\mathcal {X}(I)^{\Sigma }}\to {(\mathcal {X}(I)')^{\Sigma }}$
unramified along
 $D(\sigma )'$
when I is irregular but
$D(\sigma )'$
when I is irregular but
 $\sigma $
is regular.
$\sigma $
is regular.
Then we see, either from Proposition 8.4 or by a similar argument, the following.
Proposition 8.5 When (8.4) holds,
![]() $\nu _{\sigma , geom}(F)$
is equal to the vanishing order of F as a section of
$\nu _{\sigma , geom}(F)$
is equal to the vanishing order of F as a section of
![]() $\mathcal {L}^{\otimes k}\otimes \chi $
over
$\mathcal {L}^{\otimes k}\otimes \chi $
over
![]() ${(\mathcal {X}(I)')^{\Sigma }}$
along the boundary divisor
${(\mathcal {X}(I)')^{\Sigma }}$
along the boundary divisor
![]() $D(\sigma )'$
.
$D(\sigma )'$
.
The vanishing order at a one-dimensional cusp J is reduced to the case considered above. We choose a rank
![]() $1$
primitive sublattice
$1$
primitive sublattice
![]() $I\subset J$
and let
$I\subset J$
and let
![]() $\sigma _{J}$
be the isotropic ray in
$\sigma _{J}$
be the isotropic ray in
![]() $U(I)_{{\mathbb {R}}}$
corresponding to J. Then we define
$U(I)_{{\mathbb {R}}}$
corresponding to J. Then we define
The Taylor expansion
![]() $f=\sum _{m}\varphi _{m}q^{m}$
in this case is nothing but the Fourier–Jacobi expansion, and
$f=\sum _{m}\varphi _{m}q^{m}$
in this case is nothing but the Fourier–Jacobi expansion, and
![]() $\varphi _{m}$
is essentially the mth Fourier–Jacobi coefficient. Thus,
$\varphi _{m}$
is essentially the mth Fourier–Jacobi coefficient. Thus,
![]() $\nu _{J}(F)$
is the minimal degree of nonzero Fourier–Jacobi coefficients.
$\nu _{J}(F)$
is the minimal degree of nonzero Fourier–Jacobi coefficients.
We also have the following geometric interpretation of
![]() $\nu _{J}(F)$
. We use the
$\nu _{J}(F)$
. We use the
![]() ${U(J)_{\mathbb {Z}}}$
-invariant frame
${U(J)_{\mathbb {Z}}}$
-invariant frame
![]() $s_{I}^{\otimes k}\otimes 1_{\chi }$
to extend
$s_{I}^{\otimes k}\otimes 1_{\chi }$
to extend
![]() $\mathcal {L}^{\otimes k}\otimes \chi $
to a line bundle over
$\mathcal {L}^{\otimes k}\otimes \chi $
to a line bundle over
![]() ${\overline {\mathcal {X}(J)}}$
. This is the pullback of the extended line bundle
${\overline {\mathcal {X}(J)}}$
. This is the pullback of the extended line bundle
![]() $\mathcal {L}^{\otimes k}\otimes \chi $
over
$\mathcal {L}^{\otimes k}\otimes \chi $
over
![]() ${\mathcal {X}(I)^{\Sigma }}$
by the etale gluing map
${\mathcal {X}(I)^{\Sigma }}$
by the etale gluing map
![]() ${\overline {\mathcal {X}(J)}}\to {\mathcal {X}(I)^{\Sigma }}$
. This extension does not depend on the choice of I up to isomorphism. Then
${\overline {\mathcal {X}(J)}}\to {\mathcal {X}(I)^{\Sigma }}$
. This extension does not depend on the choice of I up to isomorphism. Then
![]() $\nu _{J}(F)$
is the vanishing order of F as a section of the extended line bundle
$\nu _{J}(F)$
is the vanishing order of F as a section of the extended line bundle
![]() $\mathcal {L}^{\otimes k}\otimes \chi $
over
$\mathcal {L}^{\otimes k}\otimes \chi $
over
![]() ${\overline {\mathcal {X}(J)}}$
along the boundary divisor. Similarly, when
${\overline {\mathcal {X}(J)}}$
along the boundary divisor. Similarly, when
![]() $s_{I}^{\otimes k}\otimes 1_{\chi }$
is invariant under
$s_{I}^{\otimes k}\otimes 1_{\chi }$
is invariant under
![]() $U(J)_{{\mathbb {Z}}}^{\star }$
,
$U(J)_{{\mathbb {Z}}}^{\star }$
,
![]() $\nu _{J,geom}(F)$
equals to the vanishing order of F along the boundary divisor of
$\nu _{J,geom}(F)$
equals to the vanishing order of F along the boundary divisor of
![]() $\overline {{\mathcal {D}}/U(J)_{{\mathbb {Z}}}^{\star }}=\overline {{\mathcal {D}}/{U(J)_{\mathbb {Z}}^{\prime }}}$
.
$\overline {{\mathcal {D}}/U(J)_{{\mathbb {Z}}}^{\star }}=\overline {{\mathcal {D}}/{U(J)_{\mathbb {Z}}^{\prime }}}$
.
8.3 Pluricanonical forms
In this subsection, we compare the vanishing order of modular forms and pluricanonical forms along the boundary divisors. Recall that we have a canonical isomorphism
over
![]() ${\mathcal {D}}$
, as a consequence of the isomorphism
${\mathcal {D}}$
, as a consequence of the isomorphism
![]() $K_{{{\mathbb P}}L_{{\mathbb {C}}}}\simeq \mathcal {O}_{{{\mathbb P}}L_{{\mathbb {C}}}}(-b-2)\otimes \det $
and the adjunction formula. Let I be a rank
$K_{{{\mathbb P}}L_{{\mathbb {C}}}}\simeq \mathcal {O}_{{{\mathbb P}}L_{{\mathbb {C}}}}(-b-2)\otimes \det $
and the adjunction formula. Let I be a rank
![]() $1$
primitive isotropic sublattice of L. The above isomorphism descends to
$1$
primitive isotropic sublattice of L. The above isomorphism descends to
![]() $\mathcal {L}^{\otimes b}\otimes \det \simeq K_{{\mathcal {X}(I)'}}$
over
$\mathcal {L}^{\otimes b}\otimes \det \simeq K_{{\mathcal {X}(I)'}}$
over
![]() ${\mathcal {X}(I)'}={\mathcal {D}}/U(I)_{{\mathbb {Z}}}^{\star }$
. Both line bundles are extended over the partial compactification
${\mathcal {X}(I)'}={\mathcal {D}}/U(I)_{{\mathbb {Z}}}^{\star }$
. Both line bundles are extended over the partial compactification
![]() ${(\mathcal {X}(I)')^{\Sigma }}$
in the respective manner:
${(\mathcal {X}(I)')^{\Sigma }}$
in the respective manner:
![]() $\mathcal {L}^{\otimes b}\otimes \det $
is extended by the frame
$\mathcal {L}^{\otimes b}\otimes \det $
is extended by the frame
![]() $s_{I}^{\otimes b}\otimes 1_{\det }$
, while
$s_{I}^{\otimes b}\otimes 1_{\det }$
, while
![]() $K_{{\mathcal {X}(I)'}}$
is extended to
$K_{{\mathcal {X}(I)'}}$
is extended to
![]() $K_{{(\mathcal {X}(I)')^{\Sigma }}}$
.
$K_{{(\mathcal {X}(I)')^{\Sigma }}}$
.
Proposition 8.6 (cf. [Reference Mumford15])
Over
![]() ${(\mathcal {X}(I)')^{\Sigma }}$
, the above isomorphism extends to
${(\mathcal {X}(I)')^{\Sigma }}$
, the above isomorphism extends to
 $$ \begin{align*} \mathcal{L}^{\otimes b}\otimes \det \: \simeq \: K_{{(\mathcal{X}(I)')^{\Sigma}}} \left( \sum_{\sigma}D(\sigma)'\right), \end{align*} $$
$$ \begin{align*} \mathcal{L}^{\otimes b}\otimes \det \: \simeq \: K_{{(\mathcal{X}(I)')^{\Sigma}}} \left( \sum_{\sigma}D(\sigma)'\right), \end{align*} $$
where
![]() $\sigma $
ranges over all rays in
$\sigma $
ranges over all rays in
![]() $\Sigma $
and
$\Sigma $
and
![]() $D(\sigma )'$
is the boundary divisor of
$D(\sigma )'$
is the boundary divisor of
![]() ${(\mathcal {X}(I)')^{\Sigma }}$
corresponding to
${(\mathcal {X}(I)')^{\Sigma }}$
corresponding to
![]() $\sigma $
.
$\sigma $
.
Proof By the isomorphism
![]() $\mathcal {L}^{\otimes b}\otimes \det \simeq K_{{\mathcal {D}}}$
, the frame
$\mathcal {L}^{\otimes b}\otimes \det \simeq K_{{\mathcal {D}}}$
, the frame
![]() $s_{I}^{\otimes b}\otimes 1_{\det }$
of
$s_{I}^{\otimes b}\otimes 1_{\det }$
of
![]() $\mathcal {L}^{\otimes b}\otimes \det $
corresponds to a flat canonical form
$\mathcal {L}^{\otimes b}\otimes \det $
corresponds to a flat canonical form
![]() $\omega _{I}$
on the tube domain
$\omega _{I}$
on the tube domain
![]() ${\mathcal {D}}_{I}\subset U(I)_{{\mathbb {C}}}$
, because both extend over
${\mathcal {D}}_{I}\subset U(I)_{{\mathbb {C}}}$
, because both extend over
![]() ${\mathcal {D}}(I)\simeq U(I)_{{\mathbb {C}}}$
and are
${\mathcal {D}}(I)\simeq U(I)_{{\mathbb {C}}}$
and are
![]() $U(I)_{{\mathbb {C}}}$
-invariant. Let
$U(I)_{{\mathbb {C}}}$
-invariant. Let
![]() $\sigma $
be a ray in
$\sigma $
be a ray in
![]() $\Sigma $
and
$\Sigma $
and
![]() $w_{\sigma }^{\prime }$
be the generator of
$w_{\sigma }^{\prime }$
be the generator of
![]() $\sigma \cap {U(I)_{\mathbb {Z}}^{\prime }}$
. We take a vector
$\sigma \cap {U(I)_{\mathbb {Z}}^{\prime }}$
. We take a vector
![]() $l_{\sigma }\in ({U(I)_{\mathbb {Z}}^{\prime }})^{\vee }$
with
$l_{\sigma }\in ({U(I)_{\mathbb {Z}}^{\prime }})^{\vee }$
with
![]() $(l_{\sigma }, w_{\sigma }^{\prime })=1$
and extend it to a basis of
$(l_{\sigma }, w_{\sigma }^{\prime })=1$
and extend it to a basis of
![]() $({U(I)_{\mathbb {Z}}^{\prime }})^{\vee }$
. This defines a coordinate
$({U(I)_{\mathbb {Z}}^{\prime }})^{\vee }$
. This defines a coordinate
![]() $Z_{1}=(l_{\sigma }, \cdot ), Z_{2}, \ldots , Z_{b}$
on
$Z_{1}=(l_{\sigma }, \cdot ), Z_{2}, \ldots , Z_{b}$
on
![]() $U(I)_{{\mathbb {C}}}$
. We have
$U(I)_{{\mathbb {C}}}$
. We have
![]() $\omega _{I}=dZ_{1}\wedge \cdots \wedge dZ_{b}$
up to constant. Then
$\omega _{I}=dZ_{1}\wedge \cdots \wedge dZ_{b}$
up to constant. Then
![]() $q=q^{l_{\sigma }}, Z_{2}, \ldots , Z_{b}$
define a local coordinate around a point of
$q=q^{l_{\sigma }}, Z_{2}, \ldots , Z_{b}$
define a local coordinate around a point of
![]() $D(\sigma )'\subset {(\mathcal {X}(I)')^{\Sigma }}$
with
$D(\sigma )'\subset {(\mathcal {X}(I)')^{\Sigma }}$
with
![]() $D(\sigma )'=(q=0)$
. Since we have
$D(\sigma )'=(q=0)$
. Since we have
around a point of
![]() $D(\sigma )'$
, this proves our assertion.
$D(\sigma )'$
, this proves our assertion.
This is the situation at a local chart for the boundary. We pass to the global situation.
Proposition 8.7 Let F be a modular form of weight
![]() $mb$
and character
$mb$
and character
![]() $\det ^{m}$
with respect to
$\det ^{m}$
with respect to
![]() ${\Gamma }$
and
${\Gamma }$
and
![]() $\omega _{F}$
be the corresponding rational m-canonical form on
$\omega _{F}$
be the corresponding rational m-canonical form on
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
. Let I be a zero-dimensional cusp,
${\mathcal {F}(\Gamma )^{\Sigma }}$
. Let I be a zero-dimensional cusp,
![]() $\sigma $
be a ray in
$\sigma $
be a ray in
![]() $\Sigma _{I}$
, and
$\Sigma _{I}$
, and
![]() $\Delta (\sigma )$
be the corresponding boundary divisor of
$\Delta (\sigma )$
be the corresponding boundary divisor of
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
. Then the vanishing order
${\mathcal {F}(\Gamma )^{\Sigma }}$
. Then the vanishing order
![]() $\nu _{\Delta (\sigma )}(\omega _{F})$
of
$\nu _{\Delta (\sigma )}(\omega _{F})$
of
![]() $\omega _{F}$
along
$\omega _{F}$
along
![]() $\Delta (\sigma )$
is given by
$\Delta (\sigma )$
is given by
 $$ \begin{align*} \nu_{\Delta(\sigma)}(\omega_{F}) = \nu_{\sigma, geom}(F) - m = \begin{cases} \nu_{\sigma}(F)-m, & \sigma : \textrm{regular}, \\ \frac{1}{2}\nu_{\sigma}(F)-m, & \sigma : \textrm{irregular}. \end{cases} \end{align*} $$
$$ \begin{align*} \nu_{\Delta(\sigma)}(\omega_{F}) = \nu_{\sigma, geom}(F) - m = \begin{cases} \nu_{\sigma}(F)-m, & \sigma : \textrm{regular}, \\ \frac{1}{2}\nu_{\sigma}(F)-m, & \sigma : \textrm{irregular}. \end{cases} \end{align*} $$
Proof Let
![]() $\pi \colon (\mathcal {X}(I)')^{\Sigma _{I}} \to {\mathcal {F}(\Gamma )^{\Sigma }}$
be the projection. By Propositions 8.5 and 8.6, we have
$\pi \colon (\mathcal {X}(I)')^{\Sigma _{I}} \to {\mathcal {F}(\Gamma )^{\Sigma }}$
be the projection. By Propositions 8.5 and 8.6, we have
By Proposition 7.2(1),
![]() $\pi $
is not ramified along
$\pi $
is not ramified along
![]() $D(\sigma )'$
, regardless of whether
$D(\sigma )'$
, regardless of whether
![]() $\sigma $
is positive-definite or isotropic. This implies that
$\sigma $
is positive-definite or isotropic. This implies that
![]() $\nu _{D(\sigma )'}(\pi ^{\ast }\omega _{F})=\nu _{\Delta (\sigma )}(\omega _{F})$
.
$\nu _{D(\sigma )'}(\pi ^{\ast }\omega _{F})=\nu _{\Delta (\sigma )}(\omega _{F})$
.
When
![]() $\sigma =\sigma _{J}$
is isotropic, the above equality can be written as
$\sigma =\sigma _{J}$
is isotropic, the above equality can be written as
where
![]() $\Delta (\sigma _{J})$
is the boundary divisor of
$\Delta (\sigma _{J})$
is the boundary divisor of
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
over J.
${\mathcal {F}(\Gamma )^{\Sigma }}$
over J.
By Gritsenko, Hulek, and Sankaran [Reference Gritsenko, Hulek and Sankaran7], every irreducible component of the ramification divisor of
![]() ${\mathcal {D}}\to {\mathcal {F}(\Gamma )}$
has ramification index
${\mathcal {D}}\to {\mathcal {F}(\Gamma )}$
has ramification index
![]() $2$
(and is defined by a reflection). Since every boundary divisor of
$2$
(and is defined by a reflection). Since every boundary divisor of
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
is of the form
${\mathcal {F}(\Gamma )^{\Sigma }}$
is of the form
![]() $\Delta (\sigma )$
for some ray
$\Delta (\sigma )$
for some ray
![]() $\sigma $
at some zero-dimensional cusp I, Proposition 8.7 implies the following.
$\sigma $
at some zero-dimensional cusp I, Proposition 8.7 implies the following.
Corollary 8.8 The m-canonical form
![]() $\omega _{F}$
extends holomorphically over the regular locus of
$\omega _{F}$
extends holomorphically over the regular locus of
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
if and only if the following hold:
${\mathcal {F}(\Gamma )^{\Sigma }}$
if and only if the following hold:
-
(1)
 $\nu _{R}(F)\geq m$
at every irreducible component R of the ramification divisor of
$\nu _{R}(F)\geq m$
at every irreducible component R of the ramification divisor of
 ${\mathcal {D}}\to {\mathcal {F}(\Gamma )}$
.
${\mathcal {D}}\to {\mathcal {F}(\Gamma )}$
. -
(2)
 $\nu _{\sigma }(F)\geq m$
at every regular ray
$\nu _{\sigma }(F)\geq m$
at every regular ray
 $\sigma $
for every zero-dimensional cusp.
$\sigma $
for every zero-dimensional cusp. -
(3)
 $\nu _{\sigma }(F)\geq 2m$
at every irregular ray
$\nu _{\sigma }(F)\geq 2m$
at every irregular ray
 $\sigma $
for every irregular zero-dimensional cusp.
$\sigma $
for every irregular zero-dimensional cusp.
Note that extendability at the boundary divisors over the one-dimensional cusps is encoded in the conditions (2) and (3) at isotropic rays
![]() $\sigma $
for adjacent zero-dimensional cusps.
$\sigma $
for adjacent zero-dimensional cusps.
8.4 Low slope cusp form criterion
We now arrive at our principal purpose. Theorem 1.2 follows from the case
![]() $k<b$
in the following.
$k<b$
in the following.
Theorem 8.9 Let L be a lattice of signature
![]() $(2, b)$
with
$(2, b)$
with
![]() $b\geq 9$
. Let
$b\geq 9$
. Let
![]() ${\Gamma }$
be a subgroup of
${\Gamma }$
be a subgroup of
![]() ${\mathrm {O}^{+}(L)}$
of finite index. We take a
${\mathrm {O}^{+}(L)}$
of finite index. We take a
![]() ${\Gamma }$
-admissible collection of fans
${\Gamma }$
-admissible collection of fans
![]() $\Sigma =(\Sigma _{I})$
such that
$\Sigma =(\Sigma _{I})$
such that
![]() $\Sigma _{I}$
is basic with respect to
$\Sigma _{I}$
is basic with respect to
![]() ${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
at each zero-dimensional cusp I. Assume that we have a cusp form F of some weight k and character with respect to
${U(I)_{\mathbb {Z}}^{\prime }}={U(I)_{\mathbb {Q}}}\cap {\langle \Gamma , -\mathrm {id} \rangle }$
at each zero-dimensional cusp I. Assume that we have a cusp form F of some weight k and character with respect to
![]() ${\Gamma }$
satisfying the following:
${\Gamma }$
satisfying the following:
-
(1) At every irreducible component R of the ramification divisor of
 ${\mathcal {D}}\to {\mathcal {F}(\Gamma )}$
, we have
${\mathcal {D}}\to {\mathcal {F}(\Gamma )}$
, we have
 $\nu _{R}(F)/k> 1/b$
.
$\nu _{R}(F)/k> 1/b$
. -
(2) At every regular ray
 $\sigma $
of
$\sigma $
of
 $\Sigma _{I}$
at every zero-dimensional cusp I, we have
$\Sigma _{I}$
at every zero-dimensional cusp I, we have
 $\nu _{\sigma }(F)/k> 1/b$
.
$\nu _{\sigma }(F)/k> 1/b$
. -
(3) At every irregular ray
 $\sigma $
of
$\sigma $
of
 $\Sigma _{I}$
at every irregular zero-dimensional cusp I, we have
$\Sigma _{I}$
at every irregular zero-dimensional cusp I, we have
 $\nu _{\sigma }(F)/k> 2/b$
.
$\nu _{\sigma }(F)/k> 2/b$
.
Then
![]() ${\mathcal {F}(\Gamma )}$
is of general type.
${\mathcal {F}(\Gamma )}$
is of general type.
Proof The following argument is a slight modification of the proof of [Reference Gritsenko, Hulek and Sankaran7, Theorem 1.1], avoiding the use of a neat cover.
Replacing F with its power, which does not change the slopes
![]() $\nu _{\ast }(F)/k$
, we may assume that the character
$\nu _{\ast }(F)/k$
, we may assume that the character
![]() $\chi $
is trivial. We first consider the case
$\chi $
is trivial. We first consider the case
![]() $b\nmid k$
. By further replacing F with its power
$b\nmid k$
. By further replacing F with its power
![]() $F^{2^{N}}$
, where N is determined by
$F^{2^{N}}$
, where N is determined by
![]() $[k/b]+2^{-N-1} \leq k/b < [k/b]+2^{-N}$
, we may assume that
$[k/b]+2^{-N-1} \leq k/b < [k/b]+2^{-N}$
, we may assume that
![]() $k/b\geq [k/b]+1/2$
so that
$k/b\geq [k/b]+1/2$
so that
![]() $[2k/b]=2[k/b]+1$
. We write
$[2k/b]=2[k/b]+1$
. We write
![]() $N_{0}=[k/b]+1$
. Then F has vanishing order
$N_{0}=[k/b]+1$
. Then F has vanishing order
![]() $\geq N_{0}$
at the ramification divisors of
$\geq N_{0}$
at the ramification divisors of
![]() ${\mathcal {D}}\to {\mathcal {F}(\Gamma )}$
and at the regular boundary divisors, and vanishing order
${\mathcal {D}}\to {\mathcal {F}(\Gamma )}$
and at the regular boundary divisors, and vanishing order
![]() $\geq 2N_{0}$
at the irregular boundary divisors. We denote by
$\geq 2N_{0}$
at the irregular boundary divisors. We denote by
![]() $M_{l}({\Gamma })$
the space of
$M_{l}({\Gamma })$
the space of
![]() ${\Gamma }$
-modular forms of weight l with trivial character. For an even number m, we consider the subspace
${\Gamma }$
-modular forms of weight l with trivial character. For an even number m, we consider the subspace
![]() $V_{m}=F^{m}\cdot M_{(bN_{0}-k)m}({\Gamma })$
of
$V_{m}=F^{m}\cdot M_{(bN_{0}-k)m}({\Gamma })$
of
![]() $M_{bN_{0}m}({\Gamma })$
. Modular forms in
$M_{bN_{0}m}({\Gamma })$
. Modular forms in
![]() $V_{m}$
have vanishing order
$V_{m}$
have vanishing order
![]() $\geq mN_{0}$
at the interior ramification divisors and at the regular boundary divisors, and vanishing order
$\geq mN_{0}$
at the interior ramification divisors and at the regular boundary divisors, and vanishing order
![]() $\geq 2mN_{0}$
at the irregular boundary divisors. Thus, the corresponding
$\geq 2mN_{0}$
at the irregular boundary divisors. Thus, the corresponding
![]() $mN_{0}$
-canonical forms extend holomorphically over the regular locus of
$mN_{0}$
-canonical forms extend holomorphically over the regular locus of
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
by Corollary 8.8. By our choice of
${\mathcal {F}(\Gamma )^{\Sigma }}$
by Corollary 8.8. By our choice of
![]() $\Sigma $
,
$\Sigma $
,
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
has canonical singularities at the boundary points by Proposition 7.4, and the interior
${\mathcal {F}(\Gamma )^{\Sigma }}$
has canonical singularities at the boundary points by Proposition 7.4, and the interior
![]() ${\mathcal {F}(\Gamma )}$
has canonical singularities by Gritsenko, Hulek, and Sankaran [Reference Gritsenko, Hulek and Sankaran7]. Therefore, these
${\mathcal {F}(\Gamma )}$
has canonical singularities by Gritsenko, Hulek, and Sankaran [Reference Gritsenko, Hulek and Sankaran7]. Therefore, these
![]() $mN_{0}$
-canonical forms extend holomorphically over a desingularization X of
$mN_{0}$
-canonical forms extend holomorphically over a desingularization X of
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
. Since
${\mathcal {F}(\Gamma )^{\Sigma }}$
. Since
![]() $bN_{0}>k$
, we have
$bN_{0}>k$
, we have
for some
![]() $c>0$
, so we find that
$c>0$
, so we find that
![]() $K_{X}$
is big.
$K_{X}$
is big.
When
![]() $b\mid k$
, we replace F with the product of a sufficiently large power of F and a modular form of weight indivisible by b. This perturbs the slopes
$b\mid k$
, we replace F with the product of a sufficiently large power of F and a modular form of weight indivisible by b. This perturbs the slopes
![]() $\nu _{\ast }(F)/k$
only by
$\nu _{\ast }(F)/k$
only by
![]() $\varepsilon $
, so the inequalities in (1)–(3) still hold. Then the same argument works.
$\varepsilon $
, so the inequalities in (1)–(3) still hold. Then the same argument works.
Remark 8.10 If we replace “
![]() $>$
” in the conditions (1)–(3) by “
$>$
” in the conditions (1)–(3) by “
![]() $\geq $
,” then the conclusion will be weakened to “
$\geq $
,” then the conclusion will be weakened to “
![]() ${\mathcal {F}(\Gamma )}$
has nonnegative Kodaira dimension.” A power of F gives a nonzero pluricanonical form.
${\mathcal {F}(\Gamma )}$
has nonnegative Kodaira dimension.” A power of F gives a nonzero pluricanonical form.
Geometric explanation of Theorem 8.9 is as follows. We have the
![]() ${\mathbb {Q}}$
-linear equivalence
${\mathbb {Q}}$
-linear equivalence
over
![]() ${\mathcal {F}(\Gamma )^{\Sigma }}$
, where B is the interior branch divisor and
${\mathcal {F}(\Gamma )^{\Sigma }}$
, where B is the interior branch divisor and
![]() $\Delta _{reg}, \Delta _{irr}$
are the regular and irregular boundary divisors, respectively. The coefficients of B and
$\Delta _{reg}, \Delta _{irr}$
are the regular and irregular boundary divisors, respectively. The coefficients of B and
![]() $\Delta _{irr}$
will be multiplied by
$\Delta _{irr}$
will be multiplied by
![]() $2$
when pulled back to local charts. The existence of the cusp form F means that
$2$
when pulled back to local charts. The existence of the cusp form F means that
![]() $b'\mathcal {L} - B/2 - \Delta _{reg} - \Delta _{irr}$
is
$b'\mathcal {L} - B/2 - \Delta _{reg} - \Delta _{irr}$
is
![]() ${\mathbb {Q}}$
-effective for some
${\mathbb {Q}}$
-effective for some
![]() $b'<b$
,
$b'<b$
,
![]() $b'\in {\mathbb {Q}}$
. (To be explicit,
$b'\in {\mathbb {Q}}$
. (To be explicit,
![]() $b'=k/N_{0}$
in the case
$b'=k/N_{0}$
in the case
![]() $b\nmid k$
in the proof.) Thus, we have
$b\nmid k$
in the proof.) Thus, we have
and the singularities do not impose obstruction.
Acknowledgments
I would like to thank Valery Gritsenko, Klaus Hulek, Shigeyuki Kondo, and Gregory Sankaran for their valuable comments.