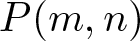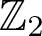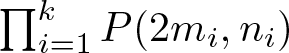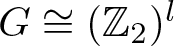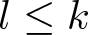1. Introduction
The famous topological spherical space form problem posed by Hopf [Reference Hopf18] asks for a classification of manifolds with universal cover homeomorphic to the n-dimensional sphere S n, where n > 1. This is equivalent to determining all finite groups that can act freely on S n. Smith [Reference Smith29] showed that such a group G must have periodic cohomology, that is, G does not contain a subgroup of the form ![]() $ \mathbb{Z}_p \oplus \mathbb{Z}_p$ for any prime p.
$ \mathbb{Z}_p \oplus \mathbb{Z}_p$ for any prime p.
A natural generalization of the above problem is to classify all finite groups that can act freely on products of finitely many spheres. More generally, one can ask a similar question for arbitrary topological spaces. This led to the concept of free rank of symmetry of a topological space, defined as follows:
Definition 1.1. Let X be a finite CW-complex and p be a prime number. The free p-rank of symmetry of X, denoted by ![]() ${\operatorname{frk}}_p(X)$, is the largest r such that
${\operatorname{frk}}_p(X)$, is the largest r such that ![]() $(\mathbb{Z}_p)^r$ acts freely on X. The smallest value of
$(\mathbb{Z}_p)^r$ acts freely on X. The smallest value of ![]() ${\operatorname{frk}}_p(X)$ over all primes p is called the free rank of symmetry. We use the terminology cohomological free 2-rank,
${\operatorname{frk}}_p(X)$ over all primes p is called the free rank of symmetry. We use the terminology cohomological free 2-rank, ![]() $ c-\operatorname{frk}_2(X) $, to refer the largest value of r such that
$ c-\operatorname{frk}_2(X) $, to refer the largest value of r such that ![]() $ (\mathbb{Z}_2)^r $ acts freely on X with a trivial action on
$ (\mathbb{Z}_2)^r $ acts freely on X with a trivial action on ![]() $ \mathbb{Z}_2 $ cohomology. They satisfy the obvious relation
$ \mathbb{Z}_2 $ cohomology. They satisfy the obvious relation ![]() $ c-\operatorname{frk}_2(X) \le \operatorname{frk}_2(X) $.
$ c-\operatorname{frk}_2(X) \le \operatorname{frk}_2(X) $.
By Smith’s result, if n is odd then ![]() ${\operatorname{frk}}_p \,(S^ n)= 1 $ for all primes p, and when n is even, then
${\operatorname{frk}}_p \,(S^ n)= 1 $ for all primes p, and when n is even, then ![]() $ {\operatorname{frk}}_p \,(S^ n)= 1$ if p = 2 and 0 if p > 2. For products of finitely many spheres, the following well-known problem can be found in various places, for example, see [Reference Adem and Browder1, Question 7.2] and [Reference Adem, Davis, Daverman and Sher2, p. 28].
$ {\operatorname{frk}}_p \,(S^ n)= 1$ if p = 2 and 0 if p > 2. For products of finitely many spheres, the following well-known problem can be found in various places, for example, see [Reference Adem and Browder1, Question 7.2] and [Reference Adem, Davis, Daverman and Sher2, p. 28].
Conjecture 1.2.
If ![]() $ (\mathbb{Z}_p)^r $ acts freely on
$ (\mathbb{Z}_p)^r $ acts freely on ![]() $S^{n_1}\times S^{n_2} \times \cdots \times S^{n_k} $, then
$S^{n_1}\times S^{n_2} \times \cdots \times S^{n_k} $, then ![]() $ r\leq k$.
$ r\leq k$.
Carlsson [Reference Carlsson6] proved the above conjecture for homologically trivial actions on products of equidimensional spheres. Adem and Browder [Reference Adem and Browder1] resolved the homological non-trivial case except in the cases p = 2 and ![]() $ n=1, 3, 7 $. Later, Yalçin [Reference Yalçin32] established that
$ n=1, 3, 7 $. Later, Yalçin [Reference Yalçin32] established that ![]() ${\operatorname{frk}}_2((S^1)^k)=k $. The most general result which settles a stable form of the above conjecture is due to Hanke [Reference Hanke16, Theorem 1.3], which states that
${\operatorname{frk}}_2((S^1)^k)=k $. The most general result which settles a stable form of the above conjecture is due to Hanke [Reference Hanke16, Theorem 1.3], which states that
where k 0 is the number of odd-dimensional spheres in the product ![]() $ S^{n_1} \times \cdots \times S ^{n_k} $. In [Reference Okutan and Yalçin25, Theorem 1.2], Okutan and Yalçin settle the conjecture in the case when the dimensions of the spheres are high compared to all the differences between the dimensions. To our knowledge, the general case remains open.
$ S^{n_1} \times \cdots \times S ^{n_k} $. In [Reference Okutan and Yalçin25, Theorem 1.2], Okutan and Yalçin settle the conjecture in the case when the dimensions of the spheres are high compared to all the differences between the dimensions. To our knowledge, the general case remains open.
For products of even-dimensional spheres, Cusick [Reference Cusick10] proved that if a finite group G acts freely and ![]() $ \mathbb{Z}_2 $-cohomologically trivially on
$ \mathbb{Z}_2 $-cohomologically trivially on ![]() $S^{2n_1} \times \cdots \times S ^{2n_k}$, then
$S^{2n_1} \times \cdots \times S ^{2n_k}$, then ![]() $G\cong (\mathbb{Z}_2)^r$ for some
$G\cong (\mathbb{Z}_2)^r$ for some ![]() $r\le k$.
$r\le k$.
For products of real projective spaces, Cusick [Reference Cusick9] conjectured that if ![]() $( \mathbb{Z}_2)^r $ acts freely on
$( \mathbb{Z}_2)^r $ acts freely on ![]() $ \mathbb{R}P^{n_1}\times\cdots\times\mathbb{R}P^{n_k} $ with a trivial action on
$ \mathbb{R}P^{n_1}\times\cdots\times\mathbb{R}P^{n_k} $ with a trivial action on ![]() $\mathbb{Z}_2$ cohomology, then
$\mathbb{Z}_2$ cohomology, then ![]() $ r\leq \eta(n_1)+\cdots+\eta(n_k),$ where the function η on
$ r\leq \eta(n_1)+\cdots+\eta(n_k),$ where the function η on ![]() $ \mathbb{N} $ is defined by
$ \mathbb{N} $ is defined by
 \begin{equation*}
\eta(n)= \left\{\begin{array}{ll}
0 & \text{if}\ n\ \text{is even},\\
1 & \text{if}\ n\ \equiv1\!\!\!\!\pmod 4,\\
2 & \text{if}\ n\ \equiv3\!\!\!\!\pmod 4.\\
\end{array} \right.
\end{equation*}
\begin{equation*}
\eta(n)= \left\{\begin{array}{ll}
0 & \text{if}\ n\ \text{is even},\\
1 & \text{if}\ n\ \equiv1\!\!\!\!\pmod 4,\\
2 & \text{if}\ n\ \equiv3\!\!\!\!\pmod 4.\\
\end{array} \right.
\end{equation*} The same paper established the conjecture when ![]() $ n_i\not\equiv3\!\pmod4 $ for all i. Later, the case when n i is odd for all i was settled in [Reference Yalçin32, Theorem 8.3]. The general case remains open.
$ n_i\not\equiv3\!\pmod4 $ for all i. Later, the case when n i is odd for all i was settled in [Reference Yalçin32, Theorem 8.3]. The general case remains open.
For products of complex projective spaces, Cusick [Reference Cusick11, Theorem 4.13] proved that if a finite group G acts freely on ![]() $ (\mathbb{C} P^m)^k $, then G is a 2-group of order at most 2k and the exponent of G cannot exceed 2 k.
$ (\mathbb{C} P^m)^k $, then G is a 2-group of order at most 2k and the exponent of G cannot exceed 2 k.
Viewing the product of two projective spaces as a trivial bundle, it is interesting to determine the free rank of symmetry of twisted projective space bundles over projective spaces. Milnor and Dold manifolds are fundamental examples of such spaces introduced by Milnor [Reference Milnor22] and Dold [Reference Dold13], respectively. It is well known that Milnor and Dold manifolds give generators for the unoriented cobordism algebra of smooth manifolds. Therefore, determining various invariants of these manifolds is an interesting problem. The free 2-rank of symmetry of products of Milnor manifolds has been investigated in [Reference Singh28], wherein some bounds have been obtained for the same.
In this paper, we investigate the free rank of symmetry of products of Dold manifolds. A Dold manifold ![]() $ P(m, n) $ is the quotient of
$ P(m, n) $ is the quotient of ![]() $ S^m \times \mathbb{C}P^n $ by the free involution that acts antipodally on
$ S^m \times \mathbb{C}P^n $ by the free involution that acts antipodally on ![]() $\mathbb{S}^m$ and by complex conjugation on
$\mathbb{S}^m$ and by complex conjugation on ![]() $\mathbb{C}P^n$ (see Definition 2.1). The free rank of symmetry of
$\mathbb{C}P^n$ (see Definition 2.1). The free rank of symmetry of  ${\prod_{i=1}^{k} P(2m_i,n_i)}$ is computed by determining the structure of finite groups that act freely on these manifolds. To state our results, we define a function
${\prod_{i=1}^{k} P(2m_i,n_i)}$ is computed by determining the structure of finite groups that act freely on these manifolds. To state our results, we define a function ![]() $ \mu:\mathbb{N} \to \{0,1\} $ by
$ \mu:\mathbb{N} \to \{0,1\} $ by
 \begin{equation}
\mu(n)= \left\{ \begin{array}{ll}
1 & \text{if}\ n\ \text{is odd},\\
0 & \text{if}\ n\ \text{is even.}\\
\end{array} \right.
\end{equation}
\begin{equation}
\mu(n)= \left\{ \begin{array}{ll}
1 & \text{if}\ n\ \text{is odd},\\
0 & \text{if}\ n\ \text{is even.}\\
\end{array} \right.
\end{equation}We establish the following result for products of Dold manifolds (see Theorem 4.11).
Theorem A
Let G be a finite group acting freely on  $X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)}$ with a trivial action on
$X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)}$ with a trivial action on ![]() $ \mathbb{Z}_2 $ cohomology. Then
$ \mathbb{Z}_2 $ cohomology. Then ![]() $G\cong (\mathbb{Z}_2)^l$ for some l satisfying
$G\cong (\mathbb{Z}_2)^l$ for some l satisfying
There is a more general conjecture due to Carlsson [Reference Carlsson, Jackowski and Pawałowski7, Conjecture I.3] for finite free G-CW-complexes.
Conjecture 1.4.
If ![]() $G=(\mathbb{Z}_p)^k$ and X is a finite free G-complex, then
$G=(\mathbb{Z}_p)^k$ and X is a finite free G-complex, then
 \begin{equation*}2^k\leq \sum_{i=0}^{\dim X} \dim_{\mathbb{F}_p} H_i(X;\mathbb{Z}_p).\end{equation*}
\begin{equation*}2^k\leq \sum_{i=0}^{\dim X} \dim_{\mathbb{F}_p} H_i(X;\mathbb{Z}_p).\end{equation*} For ![]() $\mathbb{Z}_2$-cohomologically trivial actions, the above conjecture has a positive answer for
$\mathbb{Z}_2$-cohomologically trivial actions, the above conjecture has a positive answer for  $ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $ by Theorem A. Also, the upper bound of Theorem A is sharp because of examples in
$ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $ by Theorem A. Also, the upper bound of Theorem A is sharp because of examples in ![]() $\S$ 3.
$\S$ 3.
When n i’s are even, that is, for  $ X\simeq \prod_{i=1}^k P(m_i,2n_i) $, following the Carlsson’s approach [Reference Carlsson5], we obtain the next result (see Theorem 4.20).
$ X\simeq \prod_{i=1}^k P(m_i,2n_i) $, following the Carlsson’s approach [Reference Carlsson5], we obtain the next result (see Theorem 4.20).
Theorem B
Let ![]() $ G=(\mathbb{Z}_2)^l$ act freely on
$ G=(\mathbb{Z}_2)^l$ act freely on  $ X\simeq \prod_{i=1}^k P(m_i,2n_i) $ with a trivial action on
$ X\simeq \prod_{i=1}^k P(m_i,2n_i) $ with a trivial action on ![]() $ \mathbb{Z}_2 $ cohomology.
$ \mathbb{Z}_2 $ cohomology.
(a) Then
 $ l\leq 2(\mu(m_1)+\mu(m_2)+ \cdots + \mu(m_k)) $.
$ l\leq 2(\mu(m_1)+\mu(m_2)+ \cdots + \mu(m_k)) $.(b) Moreover, if all of the odd m i’s are of the form
 $4k_i +1 $, then
$4k_i +1 $, then
 \begin{equation*}
l\leq \mu(m_1)+\mu(m_2)+ \cdots +\mu(m_k).
\end{equation*}
\begin{equation*}
l\leq \mu(m_1)+\mu(m_2)+ \cdots +\mu(m_k).
\end{equation*}
The paper is organized as follows. In ![]() $ \S$ 2, we recall basic facts about Dold manifolds. In
$ \S$ 2, we recall basic facts about Dold manifolds. In ![]() $ \S $ 3, we construct free involutions on these manifolds. Finally, in
$ \S $ 3, we construct free involutions on these manifolds. Finally, in ![]() $ \S $ 4, we investigate the free rank of symmetry of products of Dold manifolds.
$ \S $ 4, we investigate the free rank of symmetry of products of Dold manifolds.
Throughout the paper, the cyclic group of order p is denoted by ![]() $\mathbb{Z}_p$. The notation
$\mathbb{Z}_p$. The notation  $ X\simeq {\prod_{i=1}^{k} P(m_i,n_i)} $ means that X is a finite CW-complex homotopy equivalent to
$ X\simeq {\prod_{i=1}^{k} P(m_i,n_i)} $ means that X is a finite CW-complex homotopy equivalent to  $ {\prod_{i=1}^{k} P(m_i,n_i)} $. A group G acting
$ {\prod_{i=1}^{k} P(m_i,n_i)} $. A group G acting ![]() $ \mathbb{Z}_2 $-cohomologically trivially on X means the induced action on
$ \mathbb{Z}_2 $-cohomologically trivially on X means the induced action on ![]() $ H^*(X;\mathbb{Z}_2)$ is trivial.
$ H^*(X;\mathbb{Z}_2)$ is trivial.
2. Definition and cohomology of Dold manifolds
Definition 2.1. Let m and n be non-negative integers such that ![]() $m+n\gt0$. The Dold manifold
$m+n\gt0$. The Dold manifold ![]() $P(m,n)$ is a closed connected smooth manifold of dimension
$P(m,n)$ is a closed connected smooth manifold of dimension ![]() $m+2n$, obtained as the quotient of
$m+2n$, obtained as the quotient of ![]() $S^m \times \mathbb{C}P^n$ under the free involution
$S^m \times \mathbb{C}P^n$ under the free involution
The projection ![]() $S^m \times \mathbb{C}P^n \rightarrow S^m$ induces the map
$S^m \times \mathbb{C}P^n \rightarrow S^m$ induces the map
which is a locally trivial fiber bundle with fiber ![]() $\mathbb{C}P^n$. It was shown by Dold [Reference Dold13] that, for suitable values of m and n, the cobordism classes of
$\mathbb{C}P^n$. It was shown by Dold [Reference Dold13] that, for suitable values of m and n, the cobordism classes of ![]() $P(m,n)$ serve as generators in odd degrees for the unoriented cobordism algebra of smooth manifolds. In that same paper, the cohomology ring structure was also determined,
$P(m,n)$ serve as generators in odd degrees for the unoriented cobordism algebra of smooth manifolds. In that same paper, the cohomology ring structure was also determined,
 \begin{equation*}H^*(P(m,n); \mathbb{Z}_2) \cong \mathbb{Z}_2[a,b]/\left\langle a^{m+1}, b^{n+1} \right\rangle,\end{equation*}
\begin{equation*}H^*(P(m,n); \mathbb{Z}_2) \cong \mathbb{Z}_2[a,b]/\left\langle a^{m+1}, b^{n+1} \right\rangle,\end{equation*}where ![]() $\deg (a)=1$ and
$\deg (a)=1$ and ![]() $\deg (b) =2$. The action of the Steenrod square Sq 1 on the generators is given by
$\deg (b) =2$. The action of the Steenrod square Sq 1 on the generators is given by
Dold manifolds have been well-studied in the past. In [Reference Fujii14], Fujii computed the K U-groups of these manifolds. Ucci [Reference Ucci30] characterized Dold manifolds which admit codimension-one embeddings in the Euclidean space. In [Reference Peltier and Beem27], Peltier and Beem obtained some results concerning possible ![]() $ \mathbb{Z}_2 $ cohomologies of components of fixed-point sets of smooth involutions on Dold manifolds. Korbaš [Reference Korbaš20] studied parallelizability of Dold manifolds. In a recent work [Reference Nath and Sankaran24], Nath and Sankaran defined generalized Dold manifolds and investigated their stable parallelizability. Orbit spaces of free involutions on Dold manifolds of type
$ \mathbb{Z}_2 $ cohomologies of components of fixed-point sets of smooth involutions on Dold manifolds. Korbaš [Reference Korbaš20] studied parallelizability of Dold manifolds. In a recent work [Reference Nath and Sankaran24], Nath and Sankaran defined generalized Dold manifolds and investigated their stable parallelizability. Orbit spaces of free involutions on Dold manifolds of type ![]() $P(1,n)$ have been investigated recently in [Reference Morita, de Mattos and Pergher23]. Generalizing this, the possible
$P(1,n)$ have been investigated recently in [Reference Morita, de Mattos and Pergher23]. Generalizing this, the possible ![]() $ \mathbb{Z}_2 $-cohomology algebra of orbit spaces of arbitrary free involutions on a Dold manifold was determined in [Reference Dey12].
$ \mathbb{Z}_2 $-cohomology algebra of orbit spaces of arbitrary free involutions on a Dold manifold was determined in [Reference Dey12].
3. Free involutions on Dold manifolds
In this section, we give some examples of free involutions on Dold manifolds. If both m and n are even, then by Euler characteristic argument, we see that ![]() $ \mathbb{Z}_2 $ cannot act freely on
$ \mathbb{Z}_2 $ cannot act freely on ![]() $P(m, n)$.
$P(m, n)$.
(i) When n is odd: Define
 $\tau_1:\mathbb{C}P^n \rightarrow \mathbb{C}P^n $ as the free involution given by
$\tau_1:\mathbb{C}P^n \rightarrow \mathbb{C}P^n $ as the free involution given by
 \begin{equation*} \tau_1 ([z_0,\dots,z_n ]) = [-\overline{z}_1, \overline{z}_0, \dots, -\overline{z}_n, \overline{z}_{n-1}].\end{equation*}
\begin{equation*} \tau_1 ([z_0,\dots,z_n ]) = [-\overline{z}_1, \overline{z}_0, \dots, -\overline{z}_n, \overline{z}_{n-1}].\end{equation*}Then
 $id \times \tau_1$ gives a free involution on
$id \times \tau_1$ gives a free involution on  $S^m \times \mathbb{C}P^n$. Since τ 1 commutes with the action defining the Dold manifold, we obtain a free involution on
$S^m \times \mathbb{C}P^n$. Since τ 1 commutes with the action defining the Dold manifold, we obtain a free involution on  $P(m,n)$.
$P(m,n)$.(ii) When both m and n are odd: Note that the preceding free involution is defined in this case as well. Further, for
 $m \equiv 1 \pmod 4$, we define the action τ 2 on
$m \equiv 1 \pmod 4$, we define the action τ 2 on  $S^m \times \mathbb{C}P^n$ by
$S^m \times \mathbb{C}P^n$ by
 \begin{equation*}\tau_2((x_0, \ldots, x_{m}), [z_0, \ldots, z_{n}]) =((-x_1, x_0, \ldots, -x_{m}, x_{m-1}), [-\overline{z}_1, {z}_0, \ldots, -\overline{z}_n, {z}_{n-1}]).\end{equation*}
\begin{equation*}\tau_2((x_0, \ldots, x_{m}), [z_0, \ldots, z_{n}]) =((-x_1, x_0, \ldots, -x_{m}, x_{m-1}), [-\overline{z}_1, {z}_0, \ldots, -\overline{z}_n, {z}_{n-1}]).\end{equation*}This gives a free involution on
 $P(m,n)$ by passing to the quotient. Note that the composition
$P(m,n)$ by passing to the quotient. Note that the composition  $ \tau_1\circ \tau_2 $ does not define a free involution on
$ \tau_1\circ \tau_2 $ does not define a free involution on  $ P(m,n) $.
$ P(m,n) $.(iii) Suppose both
 $ m, n\equiv 3 \pmod 4 $: We know that the quaternion group Q 8 acts freely on
$ m, n\equiv 3 \pmod 4 $: We know that the quaternion group Q 8 acts freely on  $ S^{4k+3} $. This lets us define the free involutions
$ S^{4k+3} $. This lets us define the free involutions  $\varphi_\mathbf{i}$,
$\varphi_\mathbf{i}$,  $\varphi_\mathbf{j}$ and
$\varphi_\mathbf{j}$ and  $ \varphi_\mathbf{k} $ on
$ \varphi_\mathbf{k} $ on  $ P(m,n) $ as follows:
$ P(m,n) $ as follows:
 \begin{align*}
\varphi_\mathbf{j}((x_0, \ldots, x_{m}), [z_0, \ldots, z_{n}]) &=\left((-x_2, x_3, x_0,-x_{1},\ldots, -x_{m-2}),\right.\\ &\quad\left.[\overline{z}_2, {z}_3, z_0, \overline{z}_1,\ldots, \overline{z}_{n-2}]\right)\!,\end{align*}
\begin{align*}
\varphi_\mathbf{j}((x_0, \ldots, x_{m}), [z_0, \ldots, z_{n}]) &=\left((-x_2, x_3, x_0,-x_{1},\ldots, -x_{m-2}),\right.\\ &\quad\left.[\overline{z}_2, {z}_3, z_0, \overline{z}_1,\ldots, \overline{z}_{n-2}]\right)\!,\end{align*} \begin{align*}
\varphi_\mathbf{k}((x_0, \ldots, x_{m}), [z_0, \ldots, z_{n}]) &=\left((-x_3, -x_2, x_1, x_0, \ldots, x_{m-3}),\right.\\ &\quad\left.[\overline{z}_3, \overline{z}_2, {z}_1, z_0, \ldots, z_{m-3}]\right)\!.\end{align*}
\begin{align*}
\varphi_\mathbf{k}((x_0, \ldots, x_{m}), [z_0, \ldots, z_{n}]) &=\left((-x_3, -x_2, x_1, x_0, \ldots, x_{m-3}),\right.\\ &\quad\left.[\overline{z}_3, \overline{z}_2, {z}_1, z_0, \ldots, z_{m-3}]\right)\!.\end{align*}These two actions commute, and the composition
 $ \varphi_\mathbf{j}\cdot \varphi_\mathbf{k}$ gives another free involution
$ \varphi_\mathbf{j}\cdot \varphi_\mathbf{k}$ gives another free involution  $\varphi_\mathbf{i}$. Hence,
$\varphi_\mathbf{i}$. Hence,  $ \mathbb{Z}_2\oplus \mathbb{Z}_2$ acts freely in this case.
$ \mathbb{Z}_2\oplus \mathbb{Z}_2$ acts freely in this case.(iv) When m is odd and n is even: By [Reference Conner8, p-79], we know that if a closed smooth manifold does not bound, then it cannot admit free involutions. If m is odd and n is even with
 $m\lt n+1$, then
$m\lt n+1$, then  $P(m,n)$ does not bound (see [Reference Khare19, Proposition 2.4]). Hence,
$P(m,n)$ does not bound (see [Reference Khare19, Proposition 2.4]). Hence,  $P(m,n)$ does not admit any free involution in this situation.
$P(m,n)$ does not admit any free involution in this situation.
4. Free rank of symmetry
In this section, we prove our main results. Our main technique is the Serre spectral sequence associated to the Borel fibration. Let G be a finite group and EG be the universal free G-space. Suppose X is a G-space. The bundle ![]() $ X\to EG\times_G X\to BG $ associated to the universal principal G-bundle
$ X\to EG\times_G X\to BG $ associated to the universal principal G-bundle ![]() $ G\to EG\to BG $ is called the Borel fibration (see [Reference Borel, Bredon, Floyd, Montgomery and Palais4, Chapter IV, § 3], [Reference Allday and Puppe3, p. 10]). Moreover, for free G-spaces,
$ G\to EG\to BG $ is called the Borel fibration (see [Reference Borel, Bredon, Floyd, Montgomery and Palais4, Chapter IV, § 3], [Reference Allday and Puppe3, p. 10]). Moreover, for free G-spaces, ![]() $ EG\times_G X $ and
$ EG\times_G X $ and ![]() $ X/G $ are homotopy equivalent [Reference Allday and Puppe3, p. 20].
$ X/G $ are homotopy equivalent [Reference Allday and Puppe3, p. 20].
Let X be a finite CW-complex homotopy equivalent to  $ {\prod_{i=1}^{k} P(m_i,n_i)} $. The cohomology ring of X with
$ {\prod_{i=1}^{k} P(m_i,n_i)} $. The cohomology ring of X with ![]() $ \mathbb{Z}_2 $-coefficient is given by
$ \mathbb{Z}_2 $-coefficient is given by
 \begin{equation}
H^*({X};\mathbb{Z}_2)\cong \mathbb{Z}_2[a_1,\cdots,a_k, b_1,\cdots,b_k]/( a_1^{m_1+1},\cdots, a_k^{m_k+1},b_1^{n_1+1},\cdots,b_k^{n_k+1}),
\end{equation}
\begin{equation}
H^*({X};\mathbb{Z}_2)\cong \mathbb{Z}_2[a_1,\cdots,a_k, b_1,\cdots,b_k]/( a_1^{m_1+1},\cdots, a_k^{m_k+1},b_1^{n_1+1},\cdots,b_k^{n_k+1}),
\end{equation}where ![]() $ \deg(a_i)=1$ and
$ \deg(a_i)=1$ and ![]() $ \deg(b_i)=2 $ for each
$ \deg(b_i)=2 $ for each ![]() $ 1\le i\le k $.
$ 1\le i\le k $.
4.1. Free rank in the case  $ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $
$ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $
We start with the following lemma
Lemma 4.2. Let  $ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $. If
$ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $. If ![]() $ \mathbb{Z}_4 $ acts freely on X with a trivial action on
$ \mathbb{Z}_4 $ acts freely on X with a trivial action on ![]() $ \mathbb{Z}_2 $ cohomology, then
$ \mathbb{Z}_2 $ cohomology, then
Proof. Consider the Serre spectral sequence associated to the fibration ![]() $ X\hookrightarrow X/\mathbb{Z}_4\to B\mathbb{Z}_4$ whose E 2 term is
$ X\hookrightarrow X/\mathbb{Z}_4\to B\mathbb{Z}_4$ whose E 2 term is
Recall that,
Using this spectral sequence, we first show that ![]() $ H^1(X/\mathbb{Z}_4;\mathbb{Z}_2)\cong (\mathbb{Z}_2) ^{k+1}$. For this, it suffices to prove that the generators
$ H^1(X/\mathbb{Z}_4;\mathbb{Z}_2)\cong (\mathbb{Z}_2) ^{k+1}$. For this, it suffices to prove that the generators  $ a_i\in E_2^{0,1} $ are permanent cocycles. Suppose
$ a_i\in E_2^{0,1} $ are permanent cocycles. Suppose ![]() $ d_2(a_i) \ne 0$. Then
$ d_2(a_i) \ne 0$. Then  $ a_i^{2m_i+1} =0$ implies
$ a_i^{2m_i+1} =0$ implies
 \begin{equation*} 0= d_2(a_i^{2m_i+1}) =a_i^{2m_i}\otimes d_2(a_i)=a_i^{2m_i}\otimes v\ne 0,\end{equation*}
\begin{equation*} 0= d_2(a_i^{2m_i+1}) =a_i^{2m_i}\otimes d_2(a_i)=a_i^{2m_i}\otimes v\ne 0,\end{equation*}a contradiction. Hence, all the generators  $ a_i\in E_2^{0,1} $ survives to
$ a_i\in E_2^{0,1} $ survives to ![]() $ E_\infty $ page. So,
$ E_\infty $ page. So, ![]() $ H_1(X/\mathbb{Z}_4;\mathbb{Z}_2) \cong (\mathbb{Z}_2) ^{k+1}$. Note that the
$ H_1(X/\mathbb{Z}_4;\mathbb{Z}_2) \cong (\mathbb{Z}_2) ^{k+1}$. Note that the ![]() $ \mathbb{Z}_4 $ action on X induces a
$ \mathbb{Z}_4 $ action on X induces a ![]() $ \mathbb{Z}_4 $ action on
$ \mathbb{Z}_4 $ action on ![]() $ \pi_1(X,x_0) $ as
$ \pi_1(X,x_0) $ as ![]() $ \pi_1(X,x_0) $ is abelian. Moreover, by assumption, this induced
$ \pi_1(X,x_0) $ is abelian. Moreover, by assumption, this induced ![]() $ \mathbb{Z}_4 $ action on
$ \mathbb{Z}_4 $ action on ![]() $ \pi_1(X,x_0) $ is trivial. We claim that the following short exact sequence of groups is a central extension
$ \pi_1(X,x_0) $ is trivial. We claim that the following short exact sequence of groups is a central extension
 \begin{equation}
0\to \pi_1(X,x_0) \xrightarrow{i_*} \pi_1(X/\mathbb{Z}_4,\overline{x}_0)\xrightarrow{\pi_*} \mathbb{Z}_4\to 0,
\end{equation}
\begin{equation}
0\to \pi_1(X,x_0) \xrightarrow{i_*} \pi_1(X/\mathbb{Z}_4,\overline{x}_0)\xrightarrow{\pi_*} \mathbb{Z}_4\to 0,
\end{equation}where ![]() $ i:X\to X/\mathbb{Z}_4 $. For this, we observe that the induced
$ i:X\to X/\mathbb{Z}_4 $. For this, we observe that the induced ![]() $ \mathbb{Z}_4 $ action mentioned above is the same as the action of
$ \mathbb{Z}_4 $ action mentioned above is the same as the action of ![]() $ \mathbb{Z}_4 $ on
$ \mathbb{Z}_4 $ on ![]() $ \pi_1(X,x_0)$ induced by the conjugation action in
$ \pi_1(X,x_0)$ induced by the conjugation action in ![]() $ \pi_1(X/\mathbb{Z}_4,\overline{x}_0) $. To see this, let
$ \pi_1(X/\mathbb{Z}_4,\overline{x}_0) $. To see this, let ![]() $ g\in \mathbb{Z}_4 $. The action of g on
$ g\in \mathbb{Z}_4 $. The action of g on ![]() $\pi_1(X,x_0) $ is given by
$\pi_1(X,x_0) $ is given by
where θ is a path in X from x 0 to gx 0. Then applying ![]() $ i_*: \pi_1(X,x_0)\to \pi_1(X/\mathbb{Z}_4,\overline{x}_0) $, we obtain
$ i_*: \pi_1(X,x_0)\to \pi_1(X/\mathbb{Z}_4,\overline{x}_0) $, we obtain
where ![]() $ [i(\theta)]\in \pi_1(X/\mathbb{Z}_4,\overline{x}_0)$ satisfies the property that
$ [i(\theta)]\in \pi_1(X/\mathbb{Z}_4,\overline{x}_0)$ satisfies the property that ![]() $ \pi_*([i(\theta)])=g $. This is because
$ \pi_*([i(\theta)])=g $. This is because ![]() $ i(\theta) $ considered as a path in
$ i(\theta) $ considered as a path in ![]() $ E\mathbb{Z}_4\times_{\mathbb{Z}_4} X $ is a lift of a loop in
$ E\mathbb{Z}_4\times_{\mathbb{Z}_4} X $ is a lift of a loop in ![]() $ B\mathbb{Z}_4 $ representing g. Hence, Equation (4.3) is a central extension. Moreover, the quotient
$ B\mathbb{Z}_4 $ representing g. Hence, Equation (4.3) is a central extension. Moreover, the quotient ![]() $ \pi_1(X/\mathbb{Z}_4, \overline{x}_0) /\pi_1(X, x_0)\cong \mathbb{Z}_4$ is a cyclic group, so
$ \pi_1(X/\mathbb{Z}_4, \overline{x}_0) /\pi_1(X, x_0)\cong \mathbb{Z}_4$ is a cyclic group, so ![]() $ \pi_1(X/\mathbb{Z}_4,\overline{x}_0) $ is abelian. This implies
$ \pi_1(X/\mathbb{Z}_4,\overline{x}_0) $ is abelian. This implies ![]() $ \pi_1(X/\mathbb{Z}_4,\overline{x}_0)\cong H_1(X/\mathbb{Z}_4;\mathbb{Z}) \cong (\mathbb{Z}_2)^k\oplus \mathbb{Z}_4$ as all other possibilities can be ruled out by Equation (4.3).
$ \pi_1(X/\mathbb{Z}_4,\overline{x}_0)\cong H_1(X/\mathbb{Z}_4;\mathbb{Z}) \cong (\mathbb{Z}_2)^k\oplus \mathbb{Z}_4$ as all other possibilities can be ruled out by Equation (4.3).
In case of ![]() $ G =(\mathbb{Z}_2)^{r}$, we have the following:
$ G =(\mathbb{Z}_2)^{r}$, we have the following:
Lemma 4.4. Let ![]() $ G=(\mathbb{Z}_2)^{r} $ and
$ G=(\mathbb{Z}_2)^{r} $ and  $ X\simeq {\displaystyle\prod_{i=1}^{k} P(2m_i,n_i)} $. If G acts freely on X with a trivial action on
$ X\simeq {\displaystyle\prod_{i=1}^{k} P(2m_i,n_i)} $. If G acts freely on X with a trivial action on ![]() $ \mathbb{Z}_2 $ cohomology, then
$ \mathbb{Z}_2 $ cohomology, then
Proof. Since G acts freely on X, we get the following extension of groups
where ![]() $ \pi_1(X) \cong (\mathbb{Z}_2)^k$. The E 2 term of the Serre spectral sequence associated to the fibration
$ \pi_1(X) \cong (\mathbb{Z}_2)^k$. The E 2 term of the Serre spectral sequence associated to the fibration ![]() $ X\hookrightarrow X/G\to BG$ is given by
$ X\hookrightarrow X/G\to BG$ is given by
where
Using similar argument to that of Lemma 4.2, we obtain that the generators  $ a_i \in E_{2}^{0,1}$ are permanent cocycles. Thus,
$ a_i \in E_{2}^{0,1}$ are permanent cocycles. Thus, ![]() $ H_1(X/G;\mathbb{Z}_2) \cong (\mathbb{Z}_2) ^{k+r}$. As abelianization reduces the order of a non-abelian group,
$ H_1(X/G;\mathbb{Z}_2) \cong (\mathbb{Z}_2) ^{k+r}$. As abelianization reduces the order of a non-abelian group, ![]() $ \pi_1(X/ G) $ must be abelian. Hence, isomorphic to
$ \pi_1(X/ G) $ must be abelian. Hence, isomorphic to ![]() $ (\mathbb{Z}_2 )^{k+r} $.
$ (\mathbb{Z}_2 )^{k+r} $.
In the case ![]() $ G=\mathbb{Z}_p $, we have the following:
$ G=\mathbb{Z}_p $, we have the following:
Lemma 4.6. Let  $ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $. If
$ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $. If ![]() $ \mathbb{Z}_p $ (p an odd prime) acts freely on X with a trivial action on
$ \mathbb{Z}_p $ (p an odd prime) acts freely on X with a trivial action on ![]() $ \mathbb{Z}_2 $ cohomology, then
$ \mathbb{Z}_2 $ cohomology, then
Proof. As in the case of Lemma 4.2, corresponding to the ![]() $ \mathbb{Z}_p $ action, we have the following extension of groups
$ \mathbb{Z}_p $ action, we have the following extension of groups
The extension class of the extension (4.7) gives an element in ![]() $ H^2(\mathbb{Z}_p;(\mathbb{Z}_2)^k) $, which is equal to 0 as the order of
$ H^2(\mathbb{Z}_p;(\mathbb{Z}_2)^k) $, which is equal to 0 as the order of ![]() $ \mathbb{Z}_p $ and
$ \mathbb{Z}_p $ and ![]() $ (\mathbb{Z}_2)^k $ are coprime. Moreover, by assumption,
$ (\mathbb{Z}_2)^k $ are coprime. Moreover, by assumption, ![]() $ \mathbb{Z}_p $ acts trivially on
$ \mathbb{Z}_p $ acts trivially on ![]() $ \pi_1(X,x_0) $. Hence, we have the claim.
$ \pi_1(X,x_0) $. Hence, we have the claim.
Suppose that G is one of the groups ![]() $ \mathbb{Z}_4 $,
$ \mathbb{Z}_4 $, ![]() $ (\mathbb{Z}_2)^k $ or
$ (\mathbb{Z}_2)^k $ or ![]() $ \mathbb{Z}_p $. Further, let G act freely on
$ \mathbb{Z}_p $. Further, let G act freely on  $ X\simeq {\displaystyle\prod_{i=1}^{k} P(2m_i,n_i)} $. From Equations (4.3), (4.5) and (4.7), we see that the extensions
$ X\simeq {\displaystyle\prod_{i=1}^{k} P(2m_i,n_i)} $. From Equations (4.3), (4.5) and (4.7), we see that the extensions
are split extensions. As a result, we obtain that G also acts freely on the universal cover  $ \tilde{X}\simeq{\prod_{i=1}^{k} S^{2m_i}\times\mathbb{C}P^{n_i}} $. Let
$ \tilde{X}\simeq{\prod_{i=1}^{k} S^{2m_i}\times\mathbb{C}P^{n_i}} $. Let ![]() $ g\in G $. The induced action of g on
$ g\in G $. The induced action of g on ![]() $H^*(\tilde{X}; \mathbb{Z}_2)$ is denoted by
$H^*(\tilde{X}; \mathbb{Z}_2)$ is denoted by  $ \tilde{g}^*_{\mathbb{Z}_2}$ and similarly
$ \tilde{g}^*_{\mathbb{Z}_2}$ and similarly ![]() $ \tilde{g}^*_{\mathbb{Z}}$. Recall that
$ \tilde{g}^*_{\mathbb{Z}}$. Recall that
 \begin{equation}
H^*(\tilde{X};\mathbb{Z}_2)\cong \mathbb{Z}_2[\alpha_1,\ldots,\alpha_k, \beta_1,\ldots,\beta_k]/( \alpha_1^2,\ldots, \alpha_k^2,\beta_1^{n_1+1},\ldots,\beta_k^{n_k+1}),
\end{equation}
\begin{equation}
H^*(\tilde{X};\mathbb{Z}_2)\cong \mathbb{Z}_2[\alpha_1,\ldots,\alpha_k, \beta_1,\ldots,\beta_k]/( \alpha_1^2,\ldots, \alpha_k^2,\beta_1^{n_1+1},\ldots,\beta_k^{n_k+1}),
\end{equation}where ![]() $ \deg(\alpha_i)=2m_i$ and
$ \deg(\alpha_i)=2m_i$ and ![]() $ \deg(\beta_i)=2 $ for each
$ \deg(\beta_i)=2 $ for each ![]() $ 1\le i\le k $. With this notation, we have the following description of the induced action on
$ 1\le i\le k $. With this notation, we have the following description of the induced action on ![]() $H^*(\tilde{X}; \mathbb{Z}_2)$:
$H^*(\tilde{X}; \mathbb{Z}_2)$:
Lemma 4.9. Let  $ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $. The action of
$ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $. The action of  $\tilde{g}^*_{\mathbb{Z}_2}$ on the generators of
$\tilde{g}^*_{\mathbb{Z}_2}$ on the generators of ![]() $H^*(\tilde{X}; \mathbb{Z}_2)$ is given by
$H^*(\tilde{X}; \mathbb{Z}_2)$ is given by  $\tilde{g}^*_{\mathbb{Z}_2}(\beta_i)=\beta_j$ where
$\tilde{g}^*_{\mathbb{Z}_2}(\beta_i)=\beta_j$ where ![]() $n_i=n_j$ and
$n_i=n_j$ and  $\tilde{g}^*_{\mathbb{Z}_2}(\alpha_r)= \alpha_s$ where
$\tilde{g}^*_{\mathbb{Z}_2}(\alpha_r)= \alpha_s$ where ![]() $\deg \alpha _r=\deg \alpha_s$. Here
$\deg \alpha _r=\deg \alpha_s$. Here ![]() $ 1\le i, j\le k $ and
$ 1\le i, j\le k $ and ![]() $ 1\le r, s\le k $.
$ 1\le r, s\le k $.
Proof. Note that for each i, n i is the maximum value of t such that ![]() $ \beta_i^{t}\ne 0 $. In order to determine the action of
$ \beta_i^{t}\ne 0 $. In order to determine the action of  $ \tilde{g}^*_{\mathbb{Z}_2} $ on
$ \tilde{g}^*_{\mathbb{Z}_2} $ on ![]() $ H^*(\tilde{X};\mathbb{Z}_2) $, we first analyse the action of
$ H^*(\tilde{X};\mathbb{Z}_2) $, we first analyse the action of ![]() $ \tilde{g}^*_{\mathbb{Z}} $ on
$ \tilde{g}^*_{\mathbb{Z}} $ on ![]() $ H^*(\tilde{X};\mathbb{Z}) $. Then by the naturality of the change of coefficient homomorphism induced by
$ H^*(\tilde{X};\mathbb{Z}) $. Then by the naturality of the change of coefficient homomorphism induced by ![]() $ \mathbb{Z}\to \mathbb{Z}_2 $, we will have the lemma. We claim that
$ \mathbb{Z}\to \mathbb{Z}_2 $, we will have the lemma. We claim that ![]() $\tilde{g}^*_{\mathbb{Z}}(\beta_i)=\pm\beta_j$, where
$\tilde{g}^*_{\mathbb{Z}}(\beta_i)=\pm\beta_j$, where ![]() $n_i=n_j$. For this, we proceed by induction on the values of n i’s. Let β u be a generator for which n u is minimum. The relation
$n_i=n_j$. For this, we proceed by induction on the values of n i’s. Let β u be a generator for which n u is minimum. The relation ![]() $\beta_u^{n_u+1}=0$ yields
$\beta_u^{n_u+1}=0$ yields ![]() $(\tilde{g}^*_{\mathbb{Z}}(\beta_u))^{n_u+1}=0$. Suppose
$(\tilde{g}^*_{\mathbb{Z}}(\beta_u))^{n_u+1}=0$. Suppose
 \begin{equation*}
\tilde{g}^*_{\mathbb{Z}}(\beta_u)=\sum_v \lambda_v\beta_v,
\end{equation*}
\begin{equation*}
\tilde{g}^*_{\mathbb{Z}}(\beta_u)=\sum_v \lambda_v\beta_v,
\end{equation*}where ![]() $ \lambda_v\in \mathbb{Z} $. As n u is minimum, any non-zero integer multiples of
$ \lambda_v\in \mathbb{Z} $. As n u is minimum, any non-zero integer multiples of ![]() $\beta_v^{n_u}\cdot\beta_w$ are non-zero if
$\beta_v^{n_u}\cdot\beta_w$ are non-zero if ![]() $ v\ne w $ in the right hand side of the above equation. Hence, in order to get
$ v\ne w $ in the right hand side of the above equation. Hence, in order to get ![]() $(\tilde{g}^*_{\mathbb{Z}}(\beta_u))^{n_u+1}=0$, we must have all λ v’s are zero except one, say
$(\tilde{g}^*_{\mathbb{Z}}(\beta_u))^{n_u+1}=0$, we must have all λ v’s are zero except one, say ![]() $\lambda_\ell$ and
$\lambda_\ell$ and ![]() $(\beta_\ell)^{n_u+1}=0$. Moreover,
$(\beta_\ell)^{n_u+1}=0$. Moreover, ![]() $\lambda_\ell$ must be equal to ±1. Thus, we have the claim when n u is minimum. Now suppose the claim holds for any generator β q with
$\lambda_\ell$ must be equal to ±1. Thus, we have the claim when n u is minimum. Now suppose the claim holds for any generator β q with ![]() $n_q\lt n_x$. Then we show this holds for β x. Let
$n_q\lt n_x$. Then we show this holds for β x. Let
 \begin{equation}
\tilde{g}^*_{\mathbb{Z}}(\beta_x)=\sum \lambda_y\beta_y.
\end{equation}
\begin{equation}
\tilde{g}^*_{\mathbb{Z}}(\beta_x)=\sum \lambda_y\beta_y.
\end{equation} Case (a): Suppose for some β y the value of n y is equal to n x and ![]() $\lambda_y \ne 0$. Then, as before, all other
$\lambda_y \ne 0$. Then, as before, all other ![]() $\lambda_{y^\prime}$ should be zero for
$\lambda_{y^\prime}$ should be zero for ![]() $y\ne y^\prime$; otherwise,
$y\ne y^\prime$; otherwise, ![]() $(\tilde{g}^*_{\mathbb{Z}} (\beta_x))^{n_x+1}\ne 0$, a contradiction.
$(\tilde{g}^*_{\mathbb{Z}} (\beta_x))^{n_x+1}\ne 0$, a contradiction.
Case (b): Suppose for all β y in Equation (4.10) ![]() $n_y \lt n_x$. Then by induction hypothesis, we know that
$n_y \lt n_x$. Then by induction hypothesis, we know that ![]() $(\tilde{g}^*_{\mathbb{Z}})^{-1}(\beta_y)=\pm \beta_z$ for some z such that
$(\tilde{g}^*_{\mathbb{Z}})^{-1}(\beta_y)=\pm \beta_z$ for some z such that ![]() $n_y=n_z$. As a result,
$n_y=n_z$. As a result,
 \begin{equation*}(\tilde{g}^*_{\mathbb{Z}})^{-1}\left(\sum\lambda_y\beta_y\right)=\sum\pm\lambda_y\beta_z\ne\beta_x.\end{equation*}
\begin{equation*}(\tilde{g}^*_{\mathbb{Z}})^{-1}\left(\sum\lambda_y\beta_y\right)=\sum\pm\lambda_y\beta_z\ne\beta_x.\end{equation*}Hence, this case is not possible. Thus, the claim holds for the generators β i’s.
To determine the action of ![]() $\tilde{g}^*_{\mathbb{Z}}$ on α r’s, choose an α u such that
$\tilde{g}^*_{\mathbb{Z}}$ on α r’s, choose an α u such that ![]() $\deg \alpha_u=2m_u$ is minimum. Using similar argument as in Case (b), we find that
$\deg \alpha_u=2m_u$ is minimum. Using similar argument as in Case (b), we find that ![]() $\tilde{g}^*_{\mathbb{Z}}(\alpha_u)$ cannot be a monomial of the form
$\tilde{g}^*_{\mathbb{Z}}(\alpha_u)$ cannot be a monomial of the form  $\beta_{j_1}^{t_1}\cdots\beta_{j_r}^{t_r}$ of
$\beta_{j_1}^{t_1}\cdots\beta_{j_r}^{t_r}$ of ![]() $\deg$
$\deg$ ![]() $2m_u$. Then arguing as in Case (a), we get
$2m_u$. Then arguing as in Case (a), we get ![]() $ \tilde{g}^*_{\mathbb{Z}}(\alpha_u)=\pm\alpha_v $, where
$ \tilde{g}^*_{\mathbb{Z}}(\alpha_u)=\pm\alpha_v $, where ![]() $ \deg \alpha_u=\deg \alpha_v $. Finally, by induction on degrees of α r’s, we get
$ \deg \alpha_u=\deg \alpha_v $. Finally, by induction on degrees of α r’s, we get ![]() $ \tilde{g}^*_{\mathbb{Z}}(\alpha_r)=\pm\alpha_s $. Now using the naturality of the change of coefficient homomorphism,
$ \tilde{g}^*_{\mathbb{Z}}(\alpha_r)=\pm\alpha_s $. Now using the naturality of the change of coefficient homomorphism, ![]() $ \mathbb{Z} \to \mathbb{Z}_2 $, we get the result.
$ \mathbb{Z} \to \mathbb{Z}_2 $, we get the result.
We are now in a position to prove our main result for products of Dold manifolds.
Theorem 4.11 Let G be a finite group acting freely and ![]() $\mathbb{Z}_2$-cohomologically trivially on a finite CW-complex X homotopy equivalent to
$\mathbb{Z}_2$-cohomologically trivially on a finite CW-complex X homotopy equivalent to  ${\prod_{i=1}^{k} P(2m_i,n_i)}$. Then
${\prod_{i=1}^{k} P(2m_i,n_i)}$. Then ![]() $G\cong (\mathbb{Z}_2)^l$ for some l satisfying
$G\cong (\mathbb{Z}_2)^l$ for some l satisfying
Proof. We prove the case ![]() $ m_i\gt0 $. The case when some m i’s are zero is analogous. The proof of the theorem is divided into three steps. In step 1, we observe that G cannot contain an element of order 4. In step 2, we show that G cannot have odd torsion. These two together imply that G must be an elementary abelian 2-group. Then finally in step 3, we derive the bound on l. The following proposition is essential for step 1 and step 3:
$ m_i\gt0 $. The case when some m i’s are zero is analogous. The proof of the theorem is divided into three steps. In step 1, we observe that G cannot contain an element of order 4. In step 2, we show that G cannot have odd torsion. These two together imply that G must be an elementary abelian 2-group. Then finally in step 3, we derive the bound on l. The following proposition is essential for step 1 and step 3:
Proposition 4.12. Let ![]() $G= \mathbb{Z}_4 $ or
$G= \mathbb{Z}_4 $ or ![]() $ (\mathbb{Z}_2)^l $. If G acts freely on
$ (\mathbb{Z}_2)^l $. If G acts freely on  $ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $ with a trivial action on
$ X\simeq {\prod_{i=1}^{k} P(2m_i,n_i)} $ with a trivial action on ![]() $ \mathbb{Z}_2 $ cohomology, then the induced action of G on the
$ \mathbb{Z}_2 $ cohomology, then the induced action of G on the ![]() $ \mathbb{Z}_2 $ cohomology of the universal cover
$ \mathbb{Z}_2 $ cohomology of the universal cover  $ \tilde{X} \simeq {\displaystyle \prod_{i=1}^{k} S^{2m_i}\times\mathbb{C}P^{n_i}}$ is trivial.
$ \tilde{X} \simeq {\displaystyle \prod_{i=1}^{k} S^{2m_i}\times\mathbb{C}P^{n_i}}$ is trivial.
Proof. If G acts freely and ![]() $ \mathbb{Z}_2 $-cohomologically trivially on X, then the action of G on
$ \mathbb{Z}_2 $-cohomologically trivially on X, then the action of G on ![]() $ \pi_1(X) \simeq (\mathbb{Z}_2)^k$ is trivial. In particular, it acts trivially on
$ \pi_1(X) \simeq (\mathbb{Z}_2)^k$ is trivial. In particular, it acts trivially on ![]() $ B(\mathbb{Z}_2)^k $. For an element
$ B(\mathbb{Z}_2)^k $. For an element ![]() $ g\in G $, this gives the following diagram of fibrations
$ g\in G $, this gives the following diagram of fibrations

Consider the Serre spectral sequence associated with the fibration
whose E 2 term is given by
The action of ![]() $ \mathbb{Z}_2 $ on
$ \mathbb{Z}_2 $ on ![]() $ S^m\times \mathbb{C} P^n $ that defines the Dold manifold induces trivial action on
$ S^m\times \mathbb{C} P^n $ that defines the Dold manifold induces trivial action on ![]() $ H^*(S^m\times \mathbb{C} P^n;\mathbb{Z}_2) $. So the product action of
$ H^*(S^m\times \mathbb{C} P^n;\mathbb{Z}_2) $. So the product action of ![]() $ (\mathbb{Z}_2)^k $ on
$ (\mathbb{Z}_2)^k $ on  $ \tilde{X}\simeq {\prod_{i=1}^{k} S^{2m_i}\times\mathbb{C}P^{n_i}} $ also induces trivial action on
$ \tilde{X}\simeq {\prod_{i=1}^{k} S^{2m_i}\times\mathbb{C}P^{n_i}} $ also induces trivial action on ![]() $ H^*(\tilde{X};\mathbb{Z}_2) $. Therefore,
$ H^*(\tilde{X};\mathbb{Z}_2) $. Therefore,
Recall that
 \begin{equation*} H^s(\tilde{X};\mathbb{Z}_2)\cong \mathbb{Z}_2[\alpha_1,\ldots,\alpha_k, \beta_1,\ldots,\beta_k]/( \alpha_1^2,\ldots, \alpha_k^2,\beta_1^{n_1+1},\ldots,\beta_k^{n_k+1}),\end{equation*}
\begin{equation*} H^s(\tilde{X};\mathbb{Z}_2)\cong \mathbb{Z}_2[\alpha_1,\ldots,\alpha_k, \beta_1,\ldots,\beta_k]/( \alpha_1^2,\ldots, \alpha_k^2,\beta_1^{n_1+1},\ldots,\beta_k^{n_k+1}),\end{equation*}where ![]() $ \deg(\alpha_i)=2m_i$ and
$ \deg(\alpha_i)=2m_i$ and ![]() $ \deg(\beta_i)=2 $ for each
$ \deg(\beta_i)=2 $ for each ![]() $ 1\le i\le k $. The cohomology ring structure of X, Equation (4.1) tells us that the generators β i’s in
$ 1\le i\le k $. The cohomology ring structure of X, Equation (4.1) tells us that the generators β i’s in  $E_2^{0,2} $ must be permanent cocycles, and all the generators α i’s in
$E_2^{0,2} $ must be permanent cocycles, and all the generators α i’s in  $E_2^{0,2m_i}$ must be transgressive with
$E_2^{0,2m_i}$ must be transgressive with ![]() $ d_{2m_i+1}(\alpha_i)\ne0 $. Let x be an element of
$ d_{2m_i+1}(\alpha_i)\ne0 $. Let x be an element of ![]() $ H^*(\tilde{X},\mathbb{Z}_2) $. Then the naturality of the spectral sequence associated with Equation (4.13) implies
$ H^*(\tilde{X},\mathbb{Z}_2) $. Then the naturality of the spectral sequence associated with Equation (4.13) implies
 \begin{equation}
\tilde{g}^*_{\mathbb{Z}_2}( d_r(x))
=d_r(\tilde{g}^*_{\mathbb{Z}_2}(x)) . \end{equation}
\begin{equation}
\tilde{g}^*_{\mathbb{Z}_2}( d_r(x))
=d_r(\tilde{g}^*_{\mathbb{Z}_2}(x)) . \end{equation} By Lemma 4.9,  $\tilde{g}^*_{\mathbb{Z}_2}(\beta_i)=\beta_j$, where
$\tilde{g}^*_{\mathbb{Z}_2}(\beta_i)=\beta_j$, where ![]() $n_i=n_j$, and
$n_i=n_j$, and  $\tilde{g}^*_{\mathbb{Z}_2}(\alpha_r)= \alpha_s$, where
$\tilde{g}^*_{\mathbb{Z}_2}(\alpha_r)= \alpha_s$, where ![]() $\deg \alpha _r=\deg \alpha_s$.
$\deg \alpha _r=\deg \alpha_s$.
Claim: We claim that ![]() $ \alpha_r=\alpha_s $. Suppose
$ \alpha_r=\alpha_s $. Suppose ![]() $ \alpha_r \ne \alpha_s $, and let
$ \alpha_r \ne \alpha_s $, and let ![]() $ d_{2m_r+1}(\alpha_r)=\gamma_r $. Then by Equation (4.14), we obtain
$ d_{2m_r+1}(\alpha_r)=\gamma_r $. Then by Equation (4.14), we obtain
 \begin{equation*}
\tilde{g}^*_{\mathbb{Z}_2}(\gamma_r)=\tilde{g}^*_{\mathbb{Z}_2}(d_{2m_{r+1}}(\alpha_r))=d_{2m_{r+1}}(\tilde{g}^*_{\mathbb{Z}_2}(\alpha_r))=d_{2m_{r+1}}(\alpha_s)=\gamma_s.
\end{equation*}
\begin{equation*}
\tilde{g}^*_{\mathbb{Z}_2}(\gamma_r)=\tilde{g}^*_{\mathbb{Z}_2}(d_{2m_{r+1}}(\alpha_r))=d_{2m_{r+1}}(\tilde{g}^*_{\mathbb{Z}_2}(\alpha_r))=d_{2m_{r+1}}(\alpha_s)=\gamma_s.
\end{equation*} The fact that g acts trivially on ![]() $B({\mathbb{Z}_2})^k $ implies that
$B({\mathbb{Z}_2})^k $ implies that  $\tilde{g}^*_{\mathbb{Z}_2}(\gamma_t)=\gamma_t$. So
$\tilde{g}^*_{\mathbb{Z}_2}(\gamma_t)=\gamma_t$. So ![]() $ \gamma_r=\gamma_s $. For the cohomology ring structure of X, Equation (4.1) ensures that if
$ \gamma_r=\gamma_s $. For the cohomology ring structure of X, Equation (4.1) ensures that if ![]() $ \alpha_r\ne \alpha_s $, then
$ \alpha_r\ne \alpha_s $, then ![]() $ \gamma_r\ne \gamma_s$, hence a contradiction. Therefore,
$ \gamma_r\ne \gamma_s$, hence a contradiction. Therefore, ![]() $ \alpha_r=\alpha_s $.
$ \alpha_r=\alpha_s $.
Next observe that  $ \tilde{g}^*_{\mathbb{Z}_2}(\beta_i) $ must be equal to β i as the generator β i gives a generator of
$ \tilde{g}^*_{\mathbb{Z}_2}(\beta_i) $ must be equal to β i as the generator β i gives a generator of ![]() $ H^2(X;{\mathbb{Z}_2}) $ on which the action is trivial by assumption. This completes the proof of the proposition.
$ H^2(X;{\mathbb{Z}_2}) $ on which the action is trivial by assumption. This completes the proof of the proposition.
Proof of step 1
On the contrary, suppose G contains an element of order 4, that is, ![]() $ {\mathbb{Z}_4} $ acts freely and
$ {\mathbb{Z}_4} $ acts freely and ![]() $ {\mathbb{Z}_2} $-cohomologically trivially on X. Then Proposition 4.12 guarantees that
$ {\mathbb{Z}_2} $-cohomologically trivially on X. Then Proposition 4.12 guarantees that ![]() $ {\mathbb{Z}_4} $ acts freely on
$ {\mathbb{Z}_4} $ acts freely on ![]() $ \tilde{X} $ with a trivial action on
$ \tilde{X} $ with a trivial action on ![]() $ {\mathbb{Z}_2} $ cohomology. However, this is impossible because of [Reference Cusick10, Theorem A].
$ {\mathbb{Z}_2} $ cohomology. However, this is impossible because of [Reference Cusick10, Theorem A].
Proof of step 2
In case of ![]() $ G={\mathbb{Z}_p} $, we use transfer long exact sequence to give a more direct proof of the fact that if
$ G={\mathbb{Z}_p} $, we use transfer long exact sequence to give a more direct proof of the fact that if ![]() $ {\mathbb{Z}_p} $ acts freely and
$ {\mathbb{Z}_p} $ acts freely and ![]() $ \mathbb{Z}_2 $-cohomologically trivially on X, then the induced action of
$ \mathbb{Z}_2 $-cohomologically trivially on X, then the induced action of ![]() $ \mathbb{Z}_p $ on the
$ \mathbb{Z}_p $ on the ![]() $ \mathbb{Z}_2 $ cohomology of the universal cover
$ \mathbb{Z}_2 $ cohomology of the universal cover ![]() $ \tilde{X}$ is trivial (this also follows from a similar argument to that of Proposition 4.12). Consider a two-sheeted covering
$ \tilde{X}$ is trivial (this also follows from a similar argument to that of Proposition 4.12). Consider a two-sheeted covering ![]() $ \tilde{X}_1 $ of X. Let
$ \tilde{X}_1 $ of X. Let ![]() $ \pi:\tilde{X}_1\to X $ be the covering projection. Then we have the following long exact sequence
$ \pi:\tilde{X}_1\to X $ be the covering projection. Then we have the following long exact sequence
 \begin{equation*}\cdots\to H^n(X;{\mathbb{Z}_2})\xrightarrow{\pi^*} H^n(\tilde{X}_1;{\mathbb{Z}_2})\xrightarrow{\tau^*} H^n(X;{\mathbb{Z}_2})\to \cdots,\end{equation*}
\begin{equation*}\cdots\to H^n(X;{\mathbb{Z}_2})\xrightarrow{\pi^*} H^n(\tilde{X}_1;{\mathbb{Z}_2})\xrightarrow{\tau^*} H^n(X;{\mathbb{Z}_2})\to \cdots,\end{equation*}where ![]() $ \tau^* $ is the transfer homomorphism [Reference Hatcher17, p. 321]. Let
$ \tau^* $ is the transfer homomorphism [Reference Hatcher17, p. 321]. Let ![]() $ g\in {\mathbb{Z}_p} $ and
$ g\in {\mathbb{Z}_p} $ and ![]() $ y\in H^n(\tilde{X};{\mathbb{Z}_2}) $. Note that the action of G commutes with τ. Hence,
$ y\in H^n(\tilde{X};{\mathbb{Z}_2}) $. Note that the action of G commutes with τ. Hence,
where the last equality holds because g acts ![]() $ {\mathbb{Z}_2} $-cohomologically trivially on X. Hence,
$ {\mathbb{Z}_2} $-cohomologically trivially on X. Hence, ![]() $ g^*(y)+y\in \ker(\tau^*)={\rm Im}(\pi^*) $. Suppose
$ g^*(y)+y\in \ker(\tau^*)={\rm Im}(\pi^*) $. Suppose ![]() $ \pi^*(z)=g^*(y)+y $, where
$ \pi^*(z)=g^*(y)+y $, where ![]() $ z\in H^n({X};{\mathbb{Z}_2}) $. Then
$ z\in H^n({X};{\mathbb{Z}_2}) $. Then
Hence,  $ g^{*^2}(y)=y $. Since
$ g^{*^2}(y)=y $. Since ![]() $ (g^*)^p=1 $, this implies
$ (g^*)^p=1 $, this implies ![]() $ g^* $ acts trivially on
$ g^* $ acts trivially on ![]() $ H^*(\tilde{X}_1;{\mathbb{Z}_2}) $. We can repeat this process with a two-sheeted covering of
$ H^*(\tilde{X}_1;{\mathbb{Z}_2}) $. We can repeat this process with a two-sheeted covering of ![]() $ \tilde{X}_1$ until we reach to
$ \tilde{X}_1$ until we reach to ![]() $ \tilde{X} $.
$ \tilde{X} $.
Following the proof of Lemma 4.9, we obtain ![]() $ \tilde{g}^*_{\mathbb{Z}}(\beta_i)=\pm \beta_j$, where
$ \tilde{g}^*_{\mathbb{Z}}(\beta_i)=\pm \beta_j$, where ![]() $ n_i=n_j $ and
$ n_i=n_j $ and ![]() $ \tilde{g}^*_{\mathbb{Z}}(\alpha_r)=\pm \alpha_s$, where
$ \tilde{g}^*_{\mathbb{Z}}(\alpha_r)=\pm \alpha_s$, where ![]() $ \deg \alpha_r=\deg \alpha_s $. This together with the fact that
$ \deg \alpha_r=\deg \alpha_s $. This together with the fact that  $ \tilde{g}^*_{\mathbb{Z}_2}$ acts trivially on
$ \tilde{g}^*_{\mathbb{Z}_2}$ acts trivially on ![]() $ H^*(\tilde{X};{\mathbb{Z}_2}) $ implies
$ H^*(\tilde{X};{\mathbb{Z}_2}) $ implies ![]() $ \tilde{g}^*_{\mathbb{Z}}(\beta_i)=\pm \beta_i $ and
$ \tilde{g}^*_{\mathbb{Z}}(\beta_i)=\pm \beta_i $ and ![]() $ \tilde{g}^*_{\mathbb{Z}}(\alpha_i)=\pm \alpha_i$. But then
$ \tilde{g}^*_{\mathbb{Z}}(\alpha_i)=\pm \alpha_i$. But then ![]() $ (\tilde{g}^*_{\mathbb{Z}})^2 $ is identity. This contradicts the fact that if G acts freely, then the Lefschetz number must be zero for all elements of G. Hence, G cannot have odd torsion.
$ (\tilde{g}^*_{\mathbb{Z}})^2 $ is identity. This contradicts the fact that if G acts freely, then the Lefschetz number must be zero for all elements of G. Hence, G cannot have odd torsion.
Proof of step 3
Step 1 and step 2 together imply that if G acts freely on X with a trivial action on ![]() $ {\mathbb{Z}_2} $ cohomology, then G should be an elementary abelian two-group. So assume
$ {\mathbb{Z}_2} $ cohomology, then G should be an elementary abelian two-group. So assume ![]() $ G\cong({\mathbb{Z}_2})^l $. So G acts freely on
$ G\cong({\mathbb{Z}_2})^l $. So G acts freely on  $ \tilde{X}=\prod_{i=1}^kS^{2{m_i}} \times \mathbb{C}P^{n_i}$. We may apply similar reasoning as in Proposition 4.12 to show that the induced action of G on
$ \tilde{X}=\prod_{i=1}^kS^{2{m_i}} \times \mathbb{C}P^{n_i}$. We may apply similar reasoning as in Proposition 4.12 to show that the induced action of G on ![]() $ H^*(\tilde{X};{\mathbb{Z}_2}) $ is trivial. Moreover, the action of
$ H^*(\tilde{X};{\mathbb{Z}_2}) $ is trivial. Moreover, the action of ![]() $ (\mathbb{Z}_2)^k\cong \pi_1(X) $ on
$ (\mathbb{Z}_2)^k\cong \pi_1(X) $ on ![]() $ \tilde{X} $ that defines X also induces trivial action on
$ \tilde{X} $ that defines X also induces trivial action on ![]() $ H^*(\tilde{X};{\mathbb{Z}_2}) $. Hence, by Lemma 4.4,
$ H^*(\tilde{X};{\mathbb{Z}_2}) $. Hence, by Lemma 4.4, ![]() $ \pi_1(X/G) $ acts trivially on
$ \pi_1(X/G) $ acts trivially on ![]() $ H^*(\tilde{X};{\mathbb{Z}_2}) $. Let
$ H^*(\tilde{X};{\mathbb{Z}_2}) $. Let ![]() $ g\in \pi_1(X/G) $. Following the proof of Lemma 4.9, we obtain
$ g\in \pi_1(X/G) $. Following the proof of Lemma 4.9, we obtain ![]() $ \tilde{g}^*_{\mathbb{Z}}(\beta_i)=\pm \beta_j$, where
$ \tilde{g}^*_{\mathbb{Z}}(\beta_i)=\pm \beta_j$, where ![]() $ n_i=n_j $, and
$ n_i=n_j $, and ![]() $ \tilde{g}^*_{\mathbb{Z}}(\alpha_r)=\pm \alpha_s$, where
$ \tilde{g}^*_{\mathbb{Z}}(\alpha_r)=\pm \alpha_s$, where ![]() $ \deg \alpha_r=\deg \alpha_s $. Then using the fact that
$ \deg \alpha_r=\deg \alpha_s $. Then using the fact that  $ \tilde{g}^*_{\mathbb{Z}_2}$ acts trivially on
$ \tilde{g}^*_{\mathbb{Z}_2}$ acts trivially on ![]() $ H^*(\tilde{X};{\mathbb{Z}_2}) $, we get
$ H^*(\tilde{X};{\mathbb{Z}_2}) $, we get ![]() $ \tilde{g}^*_{\mathbb{Z}}(\beta_i)=\pm \beta_i $ and
$ \tilde{g}^*_{\mathbb{Z}}(\beta_i)=\pm \beta_i $ and ![]() $ \tilde{g}^*_{\mathbb{Z}}(\alpha_i)=\pm \alpha_i$. Since
$ \tilde{g}^*_{\mathbb{Z}}(\alpha_i)=\pm \alpha_i$. Since ![]() $ \pi_1(X/G)\cong (\mathbb{Z}_2)^{k+l} $ acts freely, for each
$ \pi_1(X/G)\cong (\mathbb{Z}_2)^{k+l} $ acts freely, for each ![]() $ g\ne 1 $, the Lefschetz number of
$ g\ne 1 $, the Lefschetz number of ![]() $ \tilde{g}^*_{\mathbb{Z}} $,
$ \tilde{g}^*_{\mathbb{Z}} $, ![]() $ \tau(\tilde{g}^*_{\mathbb{Z}}) $ must be zero. Suppose
$ \tau(\tilde{g}^*_{\mathbb{Z}}) $ must be zero. Suppose ![]() $ \tilde{g}^*_{\mathbb{Z}}(\beta_i) =\lambda_i\beta_i$ and
$ \tilde{g}^*_{\mathbb{Z}}(\beta_i) =\lambda_i\beta_i$ and ![]() $ \tilde{g}^*_{\mathbb{Z}}(\alpha_i) =\zeta_i\alpha_i$, for
$ \tilde{g}^*_{\mathbb{Z}}(\alpha_i) =\zeta_i\alpha_i$, for ![]() $ \lambda_i,\zeta_i \in \{\pm1\} $. Then
$ \lambda_i,\zeta_i \in \{\pm1\} $. Then
 \begin{equation*} \tau(\tilde{g}^*_{\mathbb{Z}}) = \prod_{i=1}^k (1 + \zeta_i) (1 + \lambda_i + \cdots + \lambda_i^{n_i}),
\end{equation*}
\begin{equation*} \tau(\tilde{g}^*_{\mathbb{Z}}) = \prod_{i=1}^k (1 + \zeta_i) (1 + \lambda_i + \cdots + \lambda_i^{n_i}),
\end{equation*}which is zero if either ![]() $ \zeta_i=-1 $ or in the case when n i is odd and
$ \zeta_i=-1 $ or in the case when n i is odd and ![]() $ \lambda_i=-1 $. This implies
$ \lambda_i=-1 $. This implies
Therefore, we obtain the required bound. This completes the proof of the theorem.
Remark 4.15. Examples from ![]() $\S$ 3 show that the bound given in Theorem 4.11 is sharp. As a consequence, we obtain the following bound of the cohomological free 2-rank of products of Dold manifolds:
$\S$ 3 show that the bound given in Theorem 4.11 is sharp. As a consequence, we obtain the following bound of the cohomological free 2-rank of products of Dold manifolds:
 \begin{equation*}
{c-\operatorname{frk}}_2\,\left({\displaystyle \prod_{i=1}^{k} P(2m_i, n_i)} \right)=
\mu(n_1)+\mu(n_2)+\cdots+\mu(n_k).
\end{equation*}
\begin{equation*}
{c-\operatorname{frk}}_2\,\left({\displaystyle \prod_{i=1}^{k} P(2m_i, n_i)} \right)=
\mu(n_1)+\mu(n_2)+\cdots+\mu(n_k).
\end{equation*}4.2. Free rank in the case  $ X\simeq {\displaystyle\prod_{i=1}^{k} P(m_i,2n_i)} $
$ X\simeq {\displaystyle\prod_{i=1}^{k} P(m_i,2n_i)} $
In this subsection, we prove Theorem B. For this, we closely follow the Carlsson’s approach [Reference Carlsson5]. Also, see [Reference Cusick9] and [Reference Singh28]. First, we recall the following results:
Proposition 4.16. see [Reference Greenberg15, p. 18]
Suppose ![]() $ f_1,\ldots, f_s $ are homogenous polynomials of
$ f_1,\ldots, f_s $ are homogenous polynomials of ![]() $ \deg m $ in l variables. Then they have a non-trivial common zero in
$ \deg m $ in l variables. Then they have a non-trivial common zero in ![]() $ (\mathbb{Z}_2)^l$ if l > ms.
$ (\mathbb{Z}_2)^l$ if l > ms.
Proposition 4.17. see [Reference Carlsson5, Corollary 4, Proposition 5]
Suppose ![]() $ f_1,\ldots, f_s $ are elements of
$ f_1,\ldots, f_s $ are elements of ![]() $ H^m(B(\mathbb{Z}_2)^l;\mathbb{Z}_2) $, regarded as homogenous polynomials of
$ H^m(B(\mathbb{Z}_2)^l;\mathbb{Z}_2) $, regarded as homogenous polynomials of ![]() $ \deg m $ in l variables. Then they have a non-trivial common zero in
$ \deg m $ in l variables. Then they have a non-trivial common zero in ![]() $ (\mathbb{Z}_2)^l$ if and only if there is a subgroup inclusion
$ (\mathbb{Z}_2)^l$ if and only if there is a subgroup inclusion ![]() $ i: \mathbb{Z}_2\hookrightarrow (\mathbb{Z}_2)^l $ such that
$ i: \mathbb{Z}_2\hookrightarrow (\mathbb{Z}_2)^l $ such that ![]() $ i^*(f_j)=0 $ for each j, where
$ i^*(f_j)=0 $ for each j, where ![]() $i^*: H^*(B(\mathbb{Z}_2)^l;\mathbb{Z}_2)\to H^*(B\mathbb{Z}_2;\mathbb{Z}_2) $.
$i^*: H^*(B(\mathbb{Z}_2)^l;\mathbb{Z}_2)\to H^*(B\mathbb{Z}_2;\mathbb{Z}_2) $.
With the help of Steenrod algebra, one obtains the following stronger restriction.
Proposition 4.18. see [Reference Carlsson5, Proposition 4]
Let ![]() $ \langle f_1, \ldots, f_s \rangle $ be an ideal generated by the homogenous polynomials f j’s in
$ \langle f_1, \ldots, f_s \rangle $ be an ideal generated by the homogenous polynomials f j’s in ![]() $ \mathbb{Z}_2[t_1,\ldots, t_l] $. Further, suppose that this ideal is invariant under the action of Steenrod algebra. Then the polynomials f j’s have a non-trivial common zero in
$ \mathbb{Z}_2[t_1,\ldots, t_l] $. Further, suppose that this ideal is invariant under the action of Steenrod algebra. Then the polynomials f j’s have a non-trivial common zero in ![]() $ (\mathbb{Z}_2)^l $ if l > s.
$ (\mathbb{Z}_2)^l $ if l > s.
Returning back to our situation, suppose  $ X \simeq \displaystyle\prod_{i=1}^k P(m_i,2n_i)$, and let
$ X \simeq \displaystyle\prod_{i=1}^k P(m_i,2n_i)$, and let ![]() $ G=(\mathbb{Z}_2)^l $ act freely and
$ G=(\mathbb{Z}_2)^l $ act freely and ![]() $ \mathbb{Z}_2 $-cohomologically trivially on X. Consider the Serre spectral sequence associated to the Borel fibration
$ \mathbb{Z}_2 $-cohomologically trivially on X. Consider the Serre spectral sequence associated to the Borel fibration ![]() $ X \hookrightarrow X/G \to BG $, whose E 2 term is given by
$ X \hookrightarrow X/G \to BG $, whose E 2 term is given by
Let us recall the cohomology ring structure of ![]() $ H^*(X;\mathbb{Z}_2) $ from Equation (4.1). For
$ H^*(X;\mathbb{Z}_2) $ from Equation (4.1). For  $ b_i\in E_2^{0,2} $, we have
$ b_i\in E_2^{0,2} $, we have
 \begin{equation*}
0=d_2(b_i^{2n_i+1})=b_i^{2n_i} \otimes d_2(b_i).
\end{equation*}
\begin{equation*}
0=d_2(b_i^{2n_i+1})=b_i^{2n_i} \otimes d_2(b_i).
\end{equation*} This implies ![]() $ d_2(b_i)=0 $. Similarly if for some i, m i is even, then we get
$ d_2(b_i)=0 $. Similarly if for some i, m i is even, then we get ![]() $ d_2(a_i)=0 $, where
$ d_2(a_i)=0 $, where  $ a_i\in E_2^{0,1} $. Moreover, the generators
$ a_i\in E_2^{0,1} $. Moreover, the generators  $ b_i\in E_2^{0,2} $ are permanent cocycles. For this it is enough to show
$ b_i\in E_2^{0,2} $ are permanent cocycles. For this it is enough to show ![]() $ d_3(b_i)=0 $. Suppose
$ d_3(b_i)=0 $. Suppose  $ d_3(b_i)=u_i \in E_3^{3,0} $. Then
$ d_3(b_i)=u_i \in E_3^{3,0} $. Then
 \begin{equation*}
0=d_3(b_i^{2n_i+1}) = b_i^{2n_i}\otimes d_3(b_i)=b_i^{2n_i} \otimes u_i.
\end{equation*}
\begin{equation*}
0=d_3(b_i^{2n_i+1}) = b_i^{2n_i}\otimes d_3(b_i)=b_i^{2n_i} \otimes u_i.
\end{equation*} This implies the element  $ b_i^{2n_i} \otimes u_i \in E_2^{3,4{n_i}} $ is in the image of d 2. Suppose
$ b_i^{2n_i} \otimes u_i \in E_2^{3,4{n_i}} $ is in the image of d 2. Suppose  $ b_i^{2n_i}\otimes u_i =d_2(w) $ for
$ b_i^{2n_i}\otimes u_i =d_2(w) $ for  $ w\in E_2^{1,{4n_i+1}} $. Since the d 2-differential is completely determined by its value on the generators a i’s in
$ w\in E_2^{1,{4n_i+1}} $. Since the d 2-differential is completely determined by its value on the generators a i’s in  $ E_2^{0,1}$, we get
$ E_2^{0,1}$, we get  $ b_i^{2n_i} \otimes u_i =b_i^{2n_i}\otimes d_2(z)$ for some element
$ b_i^{2n_i} \otimes u_i =b_i^{2n_i}\otimes d_2(z)$ for some element  $ z\in E_2^{1,1} $. As a result,
$ z\in E_2^{1,1} $. As a result, ![]() $ u_i=d_2(z) $. Hence,
$ u_i=d_2(z) $. Hence, ![]() $ d_3(b_i)=0 $. Let
$ d_3(b_i)=0 $. Let ![]() $ d_2(a_i)=x_i $, and let
$ d_2(a_i)=x_i $, and let ![]() $ \mathcal{I} $ be the ideal
$ \mathcal{I} $ be the ideal ![]() $ \langle x_1, \ldots , x_k \rangle $ in
$ \langle x_1, \ldots , x_k \rangle $ in ![]() $ H^*(BG;\mathbb{Z}_2) $. With this notation, we have the following
$ H^*(BG;\mathbb{Z}_2) $. With this notation, we have the following
Proposition 4.19. Let ![]() $ G=(\mathbb{Z}_2)^l $ act freely on
$ G=(\mathbb{Z}_2)^l $ act freely on  $ X \simeq \prod_{i=1}^k P(m_i,2n_i)$ with a trivial action on
$ X \simeq \prod_{i=1}^k P(m_i,2n_i)$ with a trivial action on ![]() $ \mathbb{Z}_2$ cohomology. Further, suppose all of the odd m i’s are of the form
$ \mathbb{Z}_2$ cohomology. Further, suppose all of the odd m i’s are of the form ![]() $ m_i\equiv 1 \pmod 4$ for
$ m_i\equiv 1 \pmod 4$ for ![]() $1\le i\le k $. Then the ideal
$1\le i\le k $. Then the ideal ![]() $\mathcal{I}$ is invariant under the action of Steenrod algebra.
$\mathcal{I}$ is invariant under the action of Steenrod algebra.
Proof. Note from [Reference McCleary21, Theorem 6.14] that the action of the Steenrod algebra commutes with transgressive differentials of the Serre spectral sequence. Therefore, we get
Since odd m i’s are of the form ![]() $ 4k_i+1 $, the following shows that the elements
$ 4k_i+1 $, the following shows that the elements  $ a_i^2 \in E_2^{0,2} $ are permanent cocycles:
$ a_i^2 \in E_2^{0,2} $ are permanent cocycles:
 \begin{equation*}
0=d_3(a_i^{4k_i+2})=a_i^{4k_i}\otimes d_3(a_i^2).
\end{equation*}
\begin{equation*}
0=d_3(a_i^{4k_i+2})=a_i^{4k_i}\otimes d_3(a_i^2).
\end{equation*} This implies  $ a_i^{4k_i}\otimes Sq^1(x_i)$ is in the image of d 2. Since the d 2-differential is completely determined by its value on the generators a i’s in
$ a_i^{4k_i}\otimes Sq^1(x_i)$ is in the image of d 2. Since the d 2-differential is completely determined by its value on the generators a i’s in  $ E_2^{0,1}$, we get
$ E_2^{0,1}$, we get
 \begin{equation*}
a_i^{4k_i}\otimes Sq^1(x_i)=a_i^{4k_i}\otimes d_2(\xi)
\end{equation*}
\begin{equation*}
a_i^{4k_i}\otimes Sq^1(x_i)=a_i^{4k_i}\otimes d_2(\xi)
\end{equation*}for some element  $ \xi \in E_2^{1,1} $. Hence,
$ \xi \in E_2^{1,1} $. Hence, ![]() $ d_2(\xi) =Sq^1(x_i)$. Let
$ d_2(\xi) =Sq^1(x_i)$. Let
 \begin{equation*} \xi =\sum c_{ij}t_i\otimes a_j,\end{equation*}
\begin{equation*} \xi =\sum c_{ij}t_i\otimes a_j,\end{equation*}where ![]() $ c_{ij} \in \mathbb{Z}_2 $, and for
$ c_{ij} \in \mathbb{Z}_2 $, and for ![]() $ 1\le i\le l $, t i denotes a generator of
$ 1\le i\le l $, t i denotes a generator of ![]() $ H^1(BG;\mathbb{Z}_2) $. Hence,
$ H^1(BG;\mathbb{Z}_2) $. Hence, ![]() $ Sq^1(x_i)=\sum c_{ij} t_ix_j \in \mathcal{I} $.
$ Sq^1(x_i)=\sum c_{ij} t_ix_j \in \mathcal{I} $.
Theorem 4.20 Let ![]() $ G=(\mathbb{Z}_2)^l$ act freely on
$ G=(\mathbb{Z}_2)^l$ act freely on  $ X\simeq \prod_{i=1}^k P(m_i,2n_i) $ with a trivial action on
$ X\simeq \prod_{i=1}^k P(m_i,2n_i) $ with a trivial action on ![]() $ \mathbb{Z}_2 $ cohomology.
$ \mathbb{Z}_2 $ cohomology.
(a) Then
 $ l\leq 2(\mu(m_1)+\mu(m_2)+ \cdots + \mu(m_k)) $.
$ l\leq 2(\mu(m_1)+\mu(m_2)+ \cdots + \mu(m_k)) $.(b) Moreover, if all of the odd m i’s are of the form
 $4k_i +1 $, then
$4k_i +1 $, then
 \begin{equation*}
l\leq \mu(m_1)+\mu(m_2)+ \cdots +\mu(m_k).
\end{equation*}
\begin{equation*}
l\leq \mu(m_1)+\mu(m_2)+ \cdots +\mu(m_k).
\end{equation*}
Proof. We first claim that the degree 2 polynomials x i cannot have a non-trivial common zero. Suppose they have a non-trivial common zero. Then, as given in Proposition 4.17, there exists a subgroup inclusion ![]() $ i:\mathbb{Z}_2\hookrightarrow (\mathbb{Z}_2)^l $ such that
$ i:\mathbb{Z}_2\hookrightarrow (\mathbb{Z}_2)^l $ such that ![]() $ i^*(x_i)=0 $ for each i. Let
$ i^*(x_i)=0 $ for each i. Let ![]() $ (E^{r,s}_n,d_n) $ and
$ (E^{r,s}_n,d_n) $ and ![]() $ (\tilde{E}^{r,s}_n,\tilde{d}_n) $ be the Serre spectral sequences associated with the Borel fibrations
$ (\tilde{E}^{r,s}_n,\tilde{d}_n) $ be the Serre spectral sequences associated with the Borel fibrations ![]() $ X\hookrightarrow X/G\to BG $ and
$ X\hookrightarrow X/G\to BG $ and ![]() $X\to X/\mathbb{Z}_2\to B\mathbb{Z}_2 $, respectively. Note that the system of local coefficients is trivial in both the cases. The map
$X\to X/\mathbb{Z}_2\to B\mathbb{Z}_2 $, respectively. Note that the system of local coefficients is trivial in both the cases. The map ![]() $ f^*=i^*\otimes id: E_2^{r,s}\to \tilde{E}_2^{r,s} $ commutes with the differentials. As discussed above, the only possible non-zero differentials are given by
$ f^*=i^*\otimes id: E_2^{r,s}\to \tilde{E}_2^{r,s} $ commutes with the differentials. As discussed above, the only possible non-zero differentials are given by ![]() $ d_2(a_i) $ and
$ d_2(a_i) $ and ![]() $ \tilde{d}_2(a_i) $. For the latter, observe that
$ \tilde{d}_2(a_i) $. For the latter, observe that
 \begin{equation*}
\tilde{d}_2(a_i)=\tilde{d}_2(f^*(a_i))= f^*(d_2(a_i))=0.
\end{equation*}
\begin{equation*}
\tilde{d}_2(a_i)=\tilde{d}_2(f^*(a_i))= f^*(d_2(a_i))=0.
\end{equation*} Therefore, the spectral sequence ![]() $ \tilde{E}^{r,s}_n $ collapses at the E 2 page. This contradicts the fact that
$ \tilde{E}^{r,s}_n $ collapses at the E 2 page. This contradicts the fact that ![]() $ H^*(X/\mathbb{Z}_2;\mathbb{Z}_2) $ is finite dimensional. Hence, the degree 2 polynomials x i cannot have a non-trivial common zero. Then part (a) of the theorem follows from Proposition 4.16.
$ H^*(X/\mathbb{Z}_2;\mathbb{Z}_2) $ is finite dimensional. Hence, the degree 2 polynomials x i cannot have a non-trivial common zero. Then part (a) of the theorem follows from Proposition 4.16.
For part (b), we observe that if all of the odd m i’s are of the form ![]() $ 4k_i+1 $, then the ideal
$ 4k_i+1 $, then the ideal ![]() $ \mathcal{I} $ is invariant under the action of Steenrod algebra by Proposition 4.19. Since the polynomials x i cannot have a non-trivial common zero, applying Proposition 4.18, we obtain the result.
$ \mathcal{I} $ is invariant under the action of Steenrod algebra by Proposition 4.19. Since the polynomials x i cannot have a non-trivial common zero, applying Proposition 4.18, we obtain the result.
4.3. Concluding remarks
We would like to conclude with the following natural questions.
When both ![]() $ m, n\equiv 3\pmod 4 $,
$ m, n\equiv 3\pmod 4 $, ![]() $ \mathbb{Z}_2\oplus \mathbb{Z}_2 $ acts freely on
$ \mathbb{Z}_2\oplus \mathbb{Z}_2 $ acts freely on ![]() $ P(m, n) $ (see
$ P(m, n) $ (see ![]() $\S$ 3). However, in contrast with
$\S$ 3). However, in contrast with ![]() $ \mathbb{R}P^m\times \mathbb{C}P^n $, there seems to be no obvious way to construct a free action of
$ \mathbb{R}P^m\times \mathbb{C}P^n $, there seems to be no obvious way to construct a free action of ![]() $ (\mathbb{Z}_2)^3 $ on
$ (\mathbb{Z}_2)^3 $ on ![]() $ P(m,n) $. Similarly, for
$ P(m,n) $. Similarly, for ![]() $ m,n\equiv 1\pmod 4 $, there seems to be no obvious free action of
$ m,n\equiv 1\pmod 4 $, there seems to be no obvious free action of ![]() $ (\mathbb{Z}_2)^2 $ on
$ (\mathbb{Z}_2)^2 $ on ![]() $ P(m,n) $, whereas there is an immediate free action of
$ P(m,n) $, whereas there is an immediate free action of ![]() $ (\mathbb{Z}_2)^2 $ on
$ (\mathbb{Z}_2)^2 $ on ![]() $ \mathbb{R}P^m\times \mathbb{C}P^n $. Of course, there may exist some free involutions that are exotic in nature. Hence, further investigations on the free rank of products of Dold manifolds might be interesting.
$ \mathbb{R}P^m\times \mathbb{C}P^n $. Of course, there may exist some free involutions that are exotic in nature. Hence, further investigations on the free rank of products of Dold manifolds might be interesting.
A first step towards determining the free rank of  $ \prod^k_{i=1}P(m_i,n_i) $ may be the following:
$ \prod^k_{i=1}P(m_i,n_i) $ may be the following:
Question 1: Determine the maximum value of r such that ![]() $ G=({\mathbb{Z}_2})^r $ acts freely on products of equidimensional Dold manifolds,
$ G=({\mathbb{Z}_2})^r $ acts freely on products of equidimensional Dold manifolds, ![]() $ X=(P(m,n))^k $ with a trivial action on
$ X=(P(m,n))^k $ with a trivial action on ![]() $ {\mathbb{Z}_2} $ cohomology.
$ {\mathbb{Z}_2} $ cohomology.
Associated with this action of G, we have the following extension
Investigating along the line of arguments used in [Reference Yalçin33], for example, Propositions 2.3 and 3.4, might shed some light. For this, a crucial step will be to understand the rank of ![]() $ \pi_1(X/G) $.
$ \pi_1(X/G) $.
Wall manifolds ![]() $ Q(m,n) $ [Reference Wall31] exhibit a close connection to that of Dold and Milnor manifolds in the sense that for certain values of m and n, they serve as generators for the unoriented cobordism algebra. So we may ask the following natural question.
$ Q(m,n) $ [Reference Wall31] exhibit a close connection to that of Dold and Milnor manifolds in the sense that for certain values of m and n, they serve as generators for the unoriented cobordism algebra. So we may ask the following natural question.
Question-2: Determine the free rank of symmetry of products of Wall manifolds.
The cohomology ring structure of ![]() $ Q(m, n) $ with
$ Q(m, n) $ with ![]() $ \mathbb{Z}_2 $ coefficient was determined in [Reference Wall31, Lemma 4]. In a recent work [Reference Paiva and dos Santos26], the authors have investigated group actions on Wall manifolds, where the authors determined the
$ \mathbb{Z}_2 $ coefficient was determined in [Reference Wall31, Lemma 4]. In a recent work [Reference Paiva and dos Santos26], the authors have investigated group actions on Wall manifolds, where the authors determined the ![]() $ \mathbb{Z}_2 $-cohomology algebra of orbit spaces of free involutions on some Wall manifolds.
$ \mathbb{Z}_2 $-cohomology algebra of orbit spaces of free involutions on some Wall manifolds.
Acknowledgements
This article is a part of author’s PhD thesis. The author would like to thank her supervisor Prof. Mahender Singh for some valuable discussions and for his continuous support. The author is indebted to Prof. Samik Basu for pointing out some crucial observations and for his help with some of the arguments. The author wishes to thank the anonymous referees most warmly for pointing out a gap in the previous version of the paper and for many valuable suggestions.
Competing Interests
The author declares none.