Hostname: page-component-8448b6f56d-c4f8m Total loading time: 0 Render date: 2024-04-23T23:46:16.877Z Has data issue: false hasContentIssue false
  • English
  • Français

Jump phenomena of the n-th eigenvalue of discrete Sturm–Liouville problems with application to the continuous case

Part of: Ordinary differential operators Difference equations Boundary value problems Difference and functional equations

Published online by Cambridge University Press:  02 March 2022

Guojing Ren  and
Hao Zhu
Guojing Ren
Affiliation:
School of Mathematics and Quantitative Economics, Shandong University of Finance and Economics, Jinan, Shandong 250014, P. R. China (gjren@sdufe.edu.cn)
Hao Zhu
Affiliation:
Department of Mathematics, Nanjing University, 210093 Nanjing, P. R. China (haozhu@nju.edu.cn)
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we characterize jump phenomena of the $n$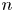-th eigenvalue of self-adjoint discrete Sturm–Liouville problems in any dimension. For a fixed Sturm–Liouville equation, we completely characterize jump phenomena of the $n$-th eigenvalue. For a fixed boundary condition, unlike in the continuous case, the $n$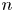-th eigenvalue exhibits jump phenomena and we describe the singularity under a non-degenerate assumption. Compared with the continuous case in Hu et al. (2019, J. Differ. Equ. 266, 4106–4136) and Kong et al. (1999, J. Differ. Equ. 156, 328–354), the jump set here is involved with coefficients of the Sturm–Liouville equations. This, along with arbitrariness of the dimension, causes difficulty when dividing the jump areas. We study the singularity by partitioning and analysing the local coordinate systems, and provide a Hermitian matrix which can determine the areas’ division. To prove the asymptotic behaviour of the $n$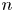-th eigenvalue, we generalize the method developed in Zhu and Shi (2016, J. Differ. Equ. 260, 5987–6016) to any dimension. As an application, by transforming the continuous Sturm–Liouville problem of Atkinson type to a discrete one, we determine the number of eigenvalues and obtain complete characterization of jump phenomena of the $n$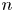-th eigenvalue for the Atkinson type.

Keywords

Sturm–Liouville problemthe n-th eigenvaluejumpasymptotic behaviour

MSC classification

Primary: 34B24: Sturm-Liouville theory
Secondary: 39A12: Discrete version of topics in analysis 34L15: Eigenvalues, estimation of eigenvalues, upper and lower bounds
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 153 , Issue 2 , April 2023 , pp. 619 - 653
Check for updates
Copyright
Copyright © The Author(s), 2022. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ao, J., Sun, J. and Zettl, A.. Finite spectrum of $2n$th order boundary value problems. Appl. Math. Lett. 42 (2015), 1–8.CrossRefGoogle Scholar
Arnold, V. I.. Characteristic class entering in quantization conditions. Funct. Anal. Appl. 1 (1967), 1–13.CrossRefGoogle Scholar
Arnold, V. I.. The complex Lagrangian Grassmannian. Funktsional. Anal. i Prilozhen. 34 (2000), 63–65 (in Russian) translation in Funct. Anal. Appl. 34 (2000), 208–210.CrossRefGoogle Scholar
Atkinson, F. V.. Discrete and Continuous Boundary Problems (Academic Press, New York, 1964).Google Scholar
Bohner, M., Došlý, O. and Kratz, W.. Sturmian and spectral theory for discrete symplectic systems. Trans. Am. Math. Soc. 361 (2009), 3109–3123.CrossRefGoogle Scholar
Clark, S.. A spectral analysis for self-adjoint operators generated by a class of second order difference equations. J. Math. Anal. Appl. 197 (1996), 267–285.CrossRefGoogle Scholar
Everitt, W. N., Möller, M. and Zettl, A., Discontinuous dependence of the n-th Sturm–Liouville eigenvalues, In: General Inequalities (Birkhäuser, Basel, 1997).CrossRefGoogle Scholar
Hu, X., Liu, L., Wu, L. and Zhu, H.. Singularity of the $n$-th eigenvalue of high dimensional Sturm–Liouville problems. J. Differ. Equ. 266 (2019), 4106–4136.CrossRefGoogle Scholar
Jirari, A.. Second-order Sturm–Liouville difference equations and orthogonal polynomials. Mem. Am. Math. Soc. 113 (1995), x+138.Google Scholar
Kato, T.. Perturbation theory for linear operators, 2nd ed. (Springer-Verlag, Berlin/ Heidelberg/New York/Tokyo, 1984).Google Scholar
Knopp, K.. Theory of functions (English translation) Part II (Dover, New York, 1947).Google Scholar
Kong, Q., Volkmer, H. and Zettl, A.. Matrix representations of Sturm–Liouville problems with finite spectrum. Res. Math. 54 (2009), 103–116.CrossRefGoogle Scholar
Kong, Q., Wu, H. and Zettl, A.. Dependence of the $n$-th Sturm–Liouville eigenvalue on the problem. J. Differ. Equ. 156 (1999), 328–354.CrossRefGoogle Scholar
Kong, Q., Wu, H. and Zettl, A.. Geometric aspects of Sturm–Liouville problems, I. Structures on spaces of boundary conditions. Proc. R. Soc. Edin. Sect. A 130 (2000), 561–589.CrossRefGoogle Scholar
Kong, Q., Wu, H. and Zettl, A.. Sturm–Liouville problems with finite spectrum. J. Math. Anal. Appl. 263 (2001), 748–762.CrossRefGoogle Scholar
Kong, Q. and Zettl, A.. Dependence of eigenvalues of Sturm–Liouville problems on the boundary. J. Differ. Equ. 126 (1996), 389–407.CrossRefGoogle Scholar
Long, Y., Index Theory for Symplectic Paths with Applications, Progr. Math., Vol. 207 (Birkhäuser, Basel, 2002).CrossRefGoogle Scholar
Rellich, F.. Störungstheorie der Spektralzerlegung. Proc. Int. Congress Math. 1 (1950), 606–613.Google Scholar
Shi, Y. and Chen, S.. Spectral theory of second-order vector difference equations. J. Math. Anal. Appl. 239 (1999), 195–212.CrossRefGoogle Scholar
Zettl, A., Sturm–Liouville Theory, Math. Surv. and Monographs, Vol. 121 (Amer. Math. Soc., RI, 2005).Google Scholar
Zhu, H.. A note on eigenvalues of a class of singular continuous and discrete linear Hamiltonian systems. Linear Algebra Appl. 570 (2019), 46–60.CrossRefGoogle Scholar
Zhu, H. and Shi, Y.. Continuous dependence of the $n$-th eigenvalue of self-adjoint discrete Sturm–Liouville problems on the problem. J. Differ. Equ. 260 (2016), 5987–6016.Google Scholar
Zhu, H., Sun, S., Shi, Y. and Wu, H.. Dependence of eigenvalues of certain closely discrete Sturm–Liouville problems. Complex Anal. Oper. Theory 10 (2016), 667–702.CrossRefGoogle Scholar