Hostname: page-component-8448b6f56d-42gr6 Total loading time: 0 Render date: 2024-04-23T07:51:43.827Z Has data issue: false hasContentIssue false
  • English
  • Français

AN UNCERTAINTY PRINCIPLE FOR SOLUTIONS OF THE SCHRÖDINGER EQUATION ON 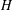$H$-TYPE GROUPS

Part of: Abstract harmonic analysis Parabolic equations and systems Lie groups

Published online by Cambridge University Press:  02 April 2020

AINGERU FERNÁNDEZ-BERTOLIN Open the ORCID record for AINGERU FERNÁNDEZ-BERTOLIN [Opens in a new window] ,
PHILIPPE JAMING Open the ORCID record for PHILIPPE JAMING [Opens in a new window]  and
SALVADOR PÉREZ-ESTEVA
AINGERU FERNÁNDEZ-BERTOLIN
Affiliation:
Departamento de Matemáticas, Universidad del País Vasco UPV/EHU, Apartado 644, 48080Bilbao, Spain e-mail: aingeru.fernandez@ehu.eus
PHILIPPE JAMING*
Affiliation:
Univ. Bordeaux, IMB, UMR 5251, F-33400Talence, France CNRS, IMB, UMR 5251, F-33400Talence, France
SALVADOR PÉREZ-ESTEVA
Affiliation:
Instituto de Matemáticas, Unidad Cuernavaca, Universidad Nacional Autónoma de México, Cuernavaca, Morelos 62251, Mexico e-mail: salvador@matcuer.unam.mx
*
e-mail: Philippe.Jaming@math.u-bordeaux.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper we consider uncertainty principles for solutions of certain partial differential equations on 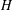$H$-type groups. We first prove that, on 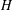$H$-type groups, the heat kernel is an average of Gaussians in the central variable, so that it does not satisfy a certain reformulation of Hardy’s uncertainty principle. We then prove the analogue of Hardy’s uncertainty principle for solutions of the Schrödinger equation with potential on 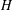$H$-type groups. This extends the free case considered by Ben Saïd et al. [‘Uniqueness of solutions to Schrödinger equations on H-type groups’, J. Aust. Math. Soc. (3)95 (2013), 297–314] and by Ludwig and Müller [‘Uniqueness of solutions to Schrödinger equations on 2-step nilpotent Lie groups’, Proc. Amer. Math. Soc.142 (2014), 2101–2118].

Keywords

uncertainty principleH-type groupSchrödinger equationheat kernel

MSC classification

Primary: 22E30: Analysis on real and complex Lie groups
Secondary: 43A80: Analysis on other specific Lie groups 35K08: Heat kernel
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 111 , Issue 1 , August 2021 , pp. 1 - 16
Check for updates
Copyright
© 2020 Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Communicated by C. Meaney

This study has been carried out with financial support from the French state, managed by the French National Research Agency (ANR) in the frame of the ‘Investments for the Future’ Programme IdEx Bordeaux—CPU (ANR-10-IDEX-03-02). A.F.-B. acknowledges financial support from ERCEA Advanced Grant 2014 669689—HADE, the MINECO project MTM2014-53145-P and the Basque Government project IT-641-13. P.J. acknowledges financial support from the French ANR program ANR-12-BS01-0001 (Aventures) from the Austrian–French AMADEUS project 35598VB—ChargeDisq and from the Tunisian–French CMCU/Utique project 15G1504. S.P.E. acknowledges financial support from the Mexican Grant PAPIIT-UNAM IN106418.

References

Baklouti, A. and Kaniuth, E., ‘On Hardy’s uncertainty principle for connected nilpotent Lie groups’, Math. Z. 259 (2008), 233–247.Google Scholar
Baklouti, A. and Thangavelu, S., ‘Variants of Miyachi’s theorem for nilpotent Lie groups’, J. Aust. Math. Soc. 88 (2010), 1–17.Google Scholar
Barceló, J. A., Fanelli, L., Gutiérrez, S., Ruiz, A. and Vilela, M. C., ‘Hardy uncertainty principle and unique continuation properties of covariant Schrödinger flows’, J. Funct. Anal. 264(10) (2013), 2386–2425.Google Scholar
Beals, R., Gaveau, B. and Greiner, P. C., ‘Hamilton-Jacobi theory and the heat kernel on Heisenberg groups’, J. Math. Pures Appl. 79(9) (2000), 633–689.Google Scholar
Ben Saïd, S., Thangavelu, S. and Dogga, V. N., ‘Uniqueness of solutions to Schrödinger equations on H-type groups’, J. Aust. Math. Soc. (3) 95 (2013), 297–314.Google Scholar
Bonfiglioli, A., Lanconelli, E. and Uguzzoni, F., Stratified Lie Groups and Potential Theory for Their Sub-Laplacians, Springer Monographs in Mathematics (Springer, Berlin, 2007).Google Scholar
Cassano, B. and Fanelli, L., ‘Sharp Hardy uncertainty principle and Gaussian profiles of covariant Schrödinger evolutions’, Trans. Amer. Math. Soc. 367 (2015), 2213–2233.Google Scholar
Cassano, B. and Fanelli, L., ‘Gaussian decay of harmonic oscillators and related models’, J. Math. Anal. Appl. 456 (2017), 214–228.Google Scholar
Chanillo, S., ‘Uniqueness of solutions to Schrödinger equations on complex semi-simple Lie groups’, Proc. Indian Acad. Sci. Math. Sci. 117 (2007), 325–331.Google Scholar
Davies, E. B., Heat Kernel and Spectral Theory (Cambridge University Press, Cambridge, 1989).Google Scholar
Davies, E. B. and Pang, M. M. H., ‘Sharp heat kernel bounds for some Laplace operators’, Quart. J. Math. Oxford Ser. (2) 40 (1989), 281–290.Google Scholar
Eldredge, N., ‘Precise estimates for the subelliptic heat kernel on H-type groups’, J. Math. Pures Appl. 92 (2009), 52–85.Google Scholar
Escauriaza, L., Kenig, C. E., Ponce, G. and Vega, L., ‘Hardy’s uncertainty principle, convexity and Schrödinger evolutions’, J. Eur. Math. Soc. 10 (2008), 883–907.Google Scholar
Escauriaza, L., Kenig, C. E., Ponce, G. and Vega, L., ‘The sharp Hardy uncertainty principle for Schrödinger evolutions’, Duke Math. J. 155 (2010), 163–187.Google Scholar
Folland, G. B. and Sitaram, A., ‘The uncertainty principle: a mathematical survey’, J. Fourier Anal. Appl. 3 (1997), 207–238.Google Scholar
Gaveau, B., ‘Principe de moindre action, propagation de la chaleur et estimées sous-elliptiques sur certains groupes nilpotents’, Acta Math. 139 (1977), 95–153.Google Scholar
Hardy, G. H., ‘A theorem concerning Fourier transforms’, J. Lond. Math. Soc. 8 (1933), 227–231.Google Scholar
Hulanicki, A., ‘The distribution of energy in the Brownian motion in the Gaussian field and analytic-hypoellipticity of certain subelliptic operators on the Heisenberg group’, Studia Math. 56 (1976), 165–173.Google Scholar
Jaming, Ph., Uncertainty principles for orthonormal bases, Séminaire d’équations aux dérivées partielles, 2006, Palayseau, France. Exposé XV, 21 February.Google Scholar
Jaming, Ph., Matolcsi, M. and Révész, S., ‘On the extremal rays of the cone of positive, positive definite functions’, J. Fourier Anal. Appl. 15 (2009), 561–582.Google Scholar
Kaniuth, E. and Kumar, A., ‘Hardy’s theorem for simply connected nilpotent Lie groups’, Math. Proc. Cambridge Philos. Soc. 131 (2001), 487–494.Google Scholar
Kaplan, A., ‘Fundamental solutions for a class of hypoelliptic PDE generated by composition of quadratic forms’, Trans. Amer. Math. Soc. 258 (1980), 147–153.Google Scholar
Klingler, A., ‘New derivation of the Heisenberg kernel’, Comm. Partial Differential Equations 22 (1997), 2051–2060.Google Scholar
Lévy, P., ‘Wiener’s random function, and other Laplacian random functions’, in: Proceedings of the Second Berkeley Symposium on Mathematical Statistics and Probability, 1950 (University of California Press, Berkeley, 1951), 171–187.Google Scholar
Ludwig, J. and Müller, D., ‘Uniqueness of solutions to Schrödinger equations on 2-step nilpotent Lie groups’, Proc. Amer. Math. Soc. 142 (2014), 2101–2118.Google Scholar
Parui, S. and Thangavelu, S., ‘On theorems of Beurling and Hardy for certain step two nilpotent groups’, Integral Transforms Spec. Funct. 20 (2009), 127–145.Google Scholar
Randall, J., ‘The heat kernel for generalized Heisenberg groups’, J. Geom. Anal. 6 (1996), 287–316.Google Scholar
Schoenberg, I. J., ‘Metric spaces and completely monotone functions’, Ann. of Math. 39 (1938), 811–841.Google Scholar
Sitaram, A., Sundari, M. and Thangavelu, S., ‘Uncertainty principles on certain Lie groups’, Proc. Indian Acad. Sci. Math. Sci. 105 (1995), 135–151.Google Scholar
Steerneman, A. G. M. and van Perlo-ten Kleij, F., ‘Spherical distributions: Schoenberg (1938) revisited’, Expo. Math. 23 (2005), 281–287.Google Scholar
Taylor, T., ‘A parametrix for step-two hypoelliptic diffusion equations’, Trans. Amer. Math. Soc. 296 (1986), 191–215.Google Scholar
Thangavelu, S., ‘An analogue of Hardy’s theorem for the Heisenberg group’, Colloq. Math. 87 (2001), 137–145.Google Scholar
Thangavelu, S., ‘Revisiting Hardy’s theorem for the Heisenberg group’, Math. Z. 242 (2002), 761–779.Google Scholar
Thangavelu, S., An Introduction to the Uncertainty Principle. Hardy’s Theorem on Lie Groups. With a Foreword by Gerald B. Folland, Progress in Mathematics, 217 (Birkhauser, Boston, 2004).Google Scholar
Varopoulos, N. Th., Saloff-Coste, L. and Coulhon, T., Analysis and Geometry on Groups, Cambridge Tracts in Mathematics, 100 (Cambridge University Press, Cambridge, 1992).Google Scholar