Let A2 be the axiomatic system of second order arithmetic as described in [2]. One of the models of A2 is the “principal model” Mpr consisting of all integers and all sets of integers. Obviously there exist many denumerable ω-models elementarily equivalent to Mpr and we shall deal in this paper with some questions pertaining to this family which we denote by .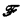

In §1 we define a rather natural relation ε between two denumerable families of sets of integers. From the upward Skolem-Löwenheim theorem it follows easily that there exists a family ordered by ε in the type ω1, but it is not immediately obvious whether there exist a subfamily of 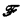not well-ordered by ε. In the present paper we construct such a family of type η. ω1 where η is the order type of rationals and indicate some applications to hyperdegrees.

We adopt the terminology and notation of [2], with the only change that we adjoin to the language of A2 the constants ν0, ν1, … for the consecutive numerals 0, 1, 2, … and axioms which characterise them:

Also we modify the axioms of A2 given in [2] by prefixing them by general quantifiers bounded either to S or to N.