Hostname: page-component-8448b6f56d-cfpbc Total loading time: 0 Render date: 2024-04-19T00:39:46.274Z Has data issue: false hasContentIssue false
  • English
  • Français

Scott's problem for Proper Scott sets

Published online by Cambridge University Press:  12 March 2014

Victoria Gitman
Victoria Gitman*
Affiliation:
New York City College of Technology (Cuny), Mathematics, 300 Jay Street, Brooklyn, NY 11201, USA, E-mail: vgitman@nylogic.org
Get access Rights & Permissions [Opens in a new window]

Abstract

Some 40 years ago, Dana Scott proved that every countable Scott set is the standard system of a model of PA. Two decades later, Knight and Nadel extended his result to Scott sets of size ω1. Here, I show that assuming the Proper Forcing Axiom (PFA), every A-proper Scott set is the standard system of a model of PA. I define that a Scott set 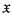is proper if the quotient Boolean algebra 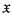/Fin is a proper partial order and A-proper if 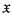is additionally arithmetically closed. I also investigate the question of the existence of proper Scott sets.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 73 , Issue 3 , September 2008 , pp. 845 - 860
Copyright
Copyright © Association for Symbolic Logic 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Baumgartner, J. E., Applications of the proper forcing axiom, Handbook of set theoretic topology (Kunen, K. and Vaughan, J., editors), North-Holland, Amsterdam, 1984, pp. 913–959.CrossRefGoogle Scholar
[2]Blass, A., On certain types and models for arithmetic, this Journal, vol. 39 (1974), pp. 151–162.Google Scholar
[3]Enayat, A., From bounded arithmetic to second order arithmetic via automorphisms, Logic in Tehran (Enayat, A., Kalantari, I., and Moniri, M., editors), Lecture Notes in Logic, vol. 26, Association for Symbolic Logic, 2006, pp. 87–113.CrossRefGoogle Scholar
[4]Enayat, A., Uncountable expansions of the standard model of Peano arithmetic, preprint, 2006.Google Scholar
[5]Engström, F., Expansions, omitting types, and standard systems, Ph.D. thesis, Chalmers University of Technology and Goteborg University, 2004.Google Scholar
[6]Gaifman, H., Models and types of Peano's arithmetic, Annals of Mathematical Logic, vol. 9 (1976), no. 3, pp. 223–306.CrossRefGoogle Scholar
[7]Gitman, V., Applications of the Proper Forcing Axiom to models of Peano Arithmetic, Ph.D. thesis, The Graduate Center of the City University of New York, 2007.Google Scholar
[8]Gitman, V., Proper and piecewise proper families of reals, 2007, preprint.Google Scholar
[9]Jech, T., Set theory, third ed., Springer Monographs in Mathematics, Springer-Verlag, New York, 2003.Google Scholar
[10]Johnstone, T. and Hamkins, J. D., The proper and semi-proper forcing axioms for forcing notions that preserve ℵ2 or ℵ3, (2007), preprint.Google Scholar
[11]Kaye, R., Models of Peano arithmetic, Oxford Logic Guides, vol. 15, Oxford University Press, New York, 1991.CrossRefGoogle Scholar
[12]Kirby, L. and Paris, J. B., Initial segments of models of Peano's axioms, Set theory and hierarchy theory, V (Proceedings of the third conference, Bierutowice, 1976), Lecture Notes in Mathematics, vol. 619, Springer-Verlag, Berlin, 1977, pp. 211–226.Google Scholar
[13]Knight, J. and Nadel, M., Models of Peano arithmetic and closed ideals, this Journal, vol. 47 (1982), no. 4, pp. 833–840.Google Scholar
[14]Kossak, R. and Schmerl, J. H., The structureof models of Peanoarithmetic, Oxford Logic Guides, vol. 50, Oxford University Press, New York, 2006.CrossRefGoogle Scholar
[15]Scott, D., Algebras of sets binumerable in complete extensions of arithmetic, Proceedings of the symposium on pure mathematics vol. V, American Mathematical Society, Providence, R.I., 1962, pp. 117–121.Google Scholar
[16]Shelah, S., Proper and improper forcing, second ed., Perspectives in Mathematical Logic, Springer-Verlag, New York, 1998.CrossRefGoogle Scholar
[17]Smoryński, S., Lectures on nonstandard models of arithmetic, Logic colloquium '82 (Florence, 1982), Studies in Logic and the Foundations of Mathematics, vol. 112, North-Holland, Amsterdam, 1984, pp. 1–70.CrossRefGoogle Scholar
[18]Veličković, B., Forcing axioms and stationary sets, Advances in Mathematics, vol. 94 (1992), no. 2, pp. 256–284.CrossRefGoogle Scholar
[19]Villaveces, A., Chains of end elementary extensions of models of set theory, this Journal, vol. 63 (1998), no. 3, pp. 1116–1136.Google Scholar