Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-26T15:17:21.032Z Has data issue: false hasContentIssue false
  • English
  • Français

Wing sweep effects on laminar separated flows

Published online by Cambridge University Press:  26 October 2022

Jean Hélder Marques Ribeiro Open the ORCID record for Jean Hélder Marques Ribeiro [Opens in a new window] ,
Chi-An Yeh Open the ORCID record for Chi-An Yeh [Opens in a new window] ,
Kai Zhang Open the ORCID record for Kai Zhang [Opens in a new window]  and
Kunihiko Taira Open the ORCID record for Kunihiko Taira [Opens in a new window]
Jean Hélder Marques Ribeiro*
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA 90095, USA
Chi-An Yeh
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA 90095, USA Department of Mechanical and Aerospace Engineering, North Carolina State University, Raleigh, NC 27695, USA
Kai Zhang
Affiliation:
Department of Mechanical and Aerospace Engineering, Rutgers University, Piscataway, NJ 08854, USA
Kunihiko Taira
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA 90095, USA
*
†Email address for correspondence: jeanmarques@g.ucla.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

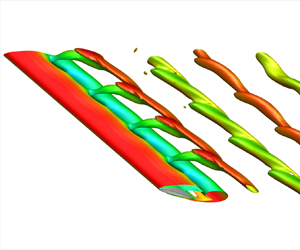
We reveal the effects of sweep on the wake dynamics around NACA 0015 wings at high angles of attack using direct numerical simulations and resolvent analysis. The influence of sweep on the wake dynamics is considered for sweep angles from $0^\circ$ to $45^\circ$ and angles of attack from $16^\circ$ to $30^\circ$ for a spanwise periodic wing at a chord-based Reynolds number of $400$ and a Mach number of $0.1$. Wing sweep affects the wake dynamics, especially in terms of stability and spanwise fluctuations with implications on the development of three-dimensional (3-D) wakes. We observe that wing sweep attenuates spanwise fluctuations. Even as the sweep angle influences the wake, force and pressure coefficients can be collapsed for low angles of attack when examined in wall-normal and wingspan-normal independent flow components. Some small deviations at high sweep and incidence angles are attributed to vortical wake structures that impose secondary aerodynamic loads, revealed through the force element analysis. Furthermore, we conduct global resolvent analysis to uncover oblique modes with high disturbance amplification. The resolvent analysis also reveals the presence of wavemakers in the shear-dominated region associated with the emergence of 3-D wakes at high angles of attack. For flows at high sweep angles, the optimal convection speed of the response modes is shown to be faster than the optimal wavemakers speed suggesting a mechanism for the attenuation of perturbations. The present findings serve as a fundamental stepping stone to understanding separated flows at higher Reynolds numbers.

JFM classification

Wakes/Jets: Separated flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 950 , 10 November 2022 , A23
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

‡

Current affiliation: School of Naval Architecture, Ocean and Civil Engineering, Shanghai Jiao Tong University, Shanghai 200240, PR China.

References

Anderson, J.D. 2010 Fundamentals of Aerodynamics. McGraw-Hill.Google Scholar
Braza, M., Faghani, D. & Persillon, H. 2001 Successive stages and the role of natural vortex dislocations in three-dimensional wake transition. J. Fluid Mech. 439, 1–41.CrossRefGoogle Scholar
Brès, G.A., Ham, F.E., Nichols, J.W. & Lele, S.K. 2017 Unstructured large-eddy simulations of supersonic jets. AIAA J. 55 (4), 1164–1184.CrossRefGoogle Scholar
Chang, C.-C. 1992 Potential flow and forces for incompressible viscous flow. Proc. R. Soc. Lond. A 437 (1901), 517–525.Google Scholar
Chu, B.-T. 1965 On the energy transfer to small disturbances in fluid flow (part I). Acta Mech. 1 (3), 215–234.CrossRefGoogle Scholar
Coleman, G.N., Rumsey, C.L. & Spalart, P.R. 2019 Numerical study of a turbulent separation bubble with sweep. J. Fluid Mech. 880, 684–706.CrossRefGoogle Scholar
Crouch, J.D., Garbaruk, A. & Strelets, M. 2019 Global instability in the onset of transonic-wing buffet. J. Fluid Mech. 881, 3–22.CrossRefGoogle Scholar
Farrell, B.F. & Ioannou, P.J. 1994 Variance maintained by stochastic forcing of non-normal dynamical systems associated with linearly stable shear flows. Phys. Rev. Lett. 72 (8), 1188–1191.CrossRefGoogle ScholarPubMed
Fosas de Pando, M. & Schmid, P.J. 2017 Optimal frequency-response sensitivity of compressible flow over roughness elements. J. Turbul. 18 (4), 338–351.CrossRefGoogle Scholar
Fosas de Pando, M., Schmid, P.J. & Sipp, D. 2017 On the receptivity of aerofoil tonal noise: an adjoint analysis. J. Fluid Mech. 812, 771–791.CrossRefGoogle Scholar
Freund, J.B. 1997 Proposed inflow/outflow boundary condition for direct computation of aerodynamic sound. AIAA J. 35 (4), 740–742.CrossRefGoogle Scholar
Garmann, D.J. & Visbal, M.R. 2020 Examination of pitch-plunge equivalence for dynamic stall over swept finite wings. AIAA Paper 2020–1759.CrossRefGoogle Scholar
Gaster, M. 1967 The structure and behaviour of laminar separation bubbles. AGARD CP-4, 813–854.Google Scholar
Giannetti, F., Camarri, S. & Luchini, P. 2010 Structural sensitivity of the secondary instability in the wake of a circular cylinder. J. Fluid Mech. 651, 319–337.CrossRefGoogle Scholar
Giannetti, F. & Luchini, P. 2007 Structural sensitivity of the first instability of the cylinder wake. J. Fluid Mech. 581 (1), 167–197.CrossRefGoogle Scholar
Gómez, F., Blackburn, H.M., Rudman, M., Sharma, A.S. & McKeon, B.J. 2016 A reduced-order model of three-dimensional unsteady flow in a cavity based on the resolvent operator. J. Fluid Mech. 798, R2.CrossRefGoogle Scholar
Harper, C.W & Maki, R.L 1964 A review of the stall characteristics of swept wings. NASA Tech. Rep. TN D-2373.Google Scholar
He, W. & Timme, S. 2021 Triglobal infinite-wing shock-buffet study. J. Fluid Mech. 925, A27.CrossRefGoogle Scholar
Hoarau, Y., Braza, M., Ventikos, Y., Faghani, D. & Tzabiras, G. 2003 Organized modes and the three-dimensional transition to turbulence in the incompressible flow around a NACA 0012 wing. J. Fluid Mech. 496, 63–72.CrossRefGoogle Scholar
Horton, H.P. 1968 Laminar separation bubbles in two and three dimensional incompressible flow. PhD thesis, Queen Mary University of London.Google Scholar
Huang, R.F., Wu, J.Y., Jeng, J.H. & Chen, R.C. 2001 Surface flow and vortex shedding of an impulsively started wing. J. Fluid Mech. 441, 265–292.CrossRefGoogle Scholar
Jovanović, M.R. 2004 Modeling, Analysis, and Control of Spatially Distributed Systems. University of California at Santa Barbara.Google Scholar
Jovanović, M.R. & Bamieh, B. 2005 Componentwise energy amplification in channel flows. J. Fluid Mech. 534, 145–183.CrossRefGoogle Scholar
Khalighi, Y., Ham, F., Nichols, J., Lele, S.K. & Moin, P. 2011 Unstructured large eddy simulation for prediction of noise issued from turbulent jets in various configurations. AIAA Paper 2011–2886.CrossRefGoogle Scholar
Kojima, Y., Yeh, C.-A., Taira, K. & Kameda, M. 2020 Resolvent analysis on the origin of two-dimensional transonic buffet. J. Fluid Mech. 885, R1.CrossRefGoogle Scholar
Liu, Q., Sun, Y., Yeh, C.-A., Ukeiley, L.S., Cattafesta, L.N. & Taira, K. 2021 Unsteady control of supersonic turbulent cavity flow based on resolvent analysis. J. Fluid Mech. 925, A5.CrossRefGoogle Scholar
McKeon, B.J. & Sharma, A.S. 2010 A critical-layer framework for turbulent pipe flow. J. Fluid Mech. 658, 336–382.CrossRefGoogle Scholar
Mittal, S., Pandi, J.S.S. & Hore, M. 2021 Cellular vortex shedding from a cylinder at low Reynolds number. J. Fluid Mech. 915, A74.CrossRefGoogle Scholar
Mittal, S. & Sidharth, G.S. 2014 Steady forces on a cylinder with oblique vortex shedding. J. Fluids Struct. 44, 310–315.CrossRefGoogle Scholar
Paladini, E., Beneddine, S., Dandois, J., Sipp, D. & Robinet, J.-C. 2019 Transonic buffet instability: from two-dimensional airfoils to three-dimensional swept wings. Phys. Rev. Fluids 4 (10), 103906.CrossRefGoogle Scholar
Pauley, L.L., Moin, P. & Reynolds, W.C. 1990 The structure of two-dimensional separation. J. Fluid Mech. 220, 397–411.CrossRefGoogle Scholar
Plante, F., Dandois, J., Beneddine, S., Laurendeau, É. & Sipp, D. 2021 Link between subsonic stall and transonic buffet on swept and unswept wings: from global stability analysis to nonlinear dynamics. J. Fluid Mech. 908, A16.CrossRefGoogle Scholar
Plante, F., Dandois, J. & Laurendeau, É. 2020 Similarities between cellular patterns occurring in transonic buffet and subsonic stall. AIAA J. 58 (1), 71–84.CrossRefGoogle Scholar
Reddy, S.C. & Henningson, D.S. 1993 Energy growth in viscous channel flows. J. Fluid Mech. 252, 209–238.CrossRefGoogle Scholar
Ribeiro, J.H.M., Yeh, C. -A. & Taira, K. 2020 Randomized resolvent analysis. Phys. Rev. Fluids 5 (3), 033902.CrossRefGoogle Scholar
Rossi, E., Colagrossi, A., Oger, G. & Le Touzé, D. 2018 Multiple bifurcations of the flow over stalled airfoils when changing the Reynolds number. J. Fluid Mech. 846, 356–391.CrossRefGoogle Scholar
Schmid, P.J. & Brandt, L. 2014 Analysis of fluid systems: stability, receptivity, sensitivity. Appl. Mech. Rev. 66 (2), 024803.CrossRefGoogle Scholar
Schmidt, O.T., Towne, A., Rigas, G., Colonius, T. & Brès, G.A. 2018 Spectral analysis of jet turbulence. J. Fluid Mech. 855, 953–982.CrossRefGoogle Scholar
Serpieri, J. & Kotsonis, M. 2016 Three-dimensional organisation of primary and secondary crossflow instability. J. Fluid Mech. 799, 200–245.CrossRefGoogle Scholar
Skene, C.S., Ribeiro, J.H.M. & Taira, K. 2022 csskene/linear-analysis-tools: initial release. Available at: https://doi.org/10.5281/zenodo.6550726.CrossRefGoogle Scholar
Skene, C.S. & Schmid, P.J. 2019 Adjoint-based parametric sensitivity analysis for swirling M-flames. J. Fluid Mech. 859, 516–542.CrossRefGoogle Scholar
Stewart, G.W. 2002 A Krylov–Schur algorithm for large eigenproblems. SIAM J. Matrix Anal. Applics. 23 (3), 601–614.CrossRefGoogle Scholar
Sun, Y., Taira, K., Cattafesta, L.N.III & Ukeiley, L.S. 2017 Biglobal instabilities of compressible open-cavity flows. J. Fluid Mech. 826, 270–301.CrossRefGoogle Scholar
Taira, K., Brunton, S.L., Dawson, S.T.M., Rowley, C.W., Colonius, T., McKeon, B.J., Schmidt, O.T., Gordeyev, S., Theofilis, V. & Ukeiley, L.S. 2017 Modal analysis of fluid flows: an overview. AIAA J. 55 (12), 4013–4041.CrossRefGoogle Scholar
Taira, K. & Colonius, T. 2009 Three-dimensional flows around low-aspect-ratio flat-plate wings at low Reynolds numbers. J. Fluid Mech. 623, 187–207.CrossRefGoogle Scholar
Taira, K., Hemati, M.S., Brunton, S.L., Sun, Y., Duraisamy, K., Bagheri, S., Dawson, S.T.M. & Yeh, C.-A. 2020 Modal analysis of fluid flows: applications and outlook. AIAA J. 58 (3), 998–1022.CrossRefGoogle Scholar
Tobak, M. & Peake, D.J. 1982 Topology of three-dimensional separated flows. Annu. Rev. Fluid Mech. 14 (1), 61–85.CrossRefGoogle Scholar
Trefethen, L.N. & Embree, M. 2005 Spectra and Pseudospectra. Princeton University Press.CrossRefGoogle Scholar
Trefethen, L.N., Trefethen, A.E., Reddy, S.C. & Driscoll, T.A. 1993 Hydrodynamic stability without eigenvalues. Science 261 (5121), 578–584.CrossRefGoogle ScholarPubMed
Videler, J.J., Stamhuis, E.J. & Povel, G.D.E. 2004 Leading-edge vortex lifts swifts. Science 306 (5703), 1960–1962.CrossRefGoogle ScholarPubMed
Visbal, M.R. & Garmann, D.J. 2019 Effect of sweep on dynamic stall of a pitching finite-aspect-ratio wing. AIAA J. 57 (8), 3274–3289.CrossRefGoogle Scholar
White, F.M. 1991 Viscous Fluid Flow. McGraw-Hill.Google Scholar
Williamson, C.H.K. & Roshko, A. 1988 Vortex formation in the wake of an oscillating cylinder. J. Fluids Struct. 2 (4), 355–381.CrossRefGoogle Scholar
Winkelman, A.E. & Barlow, J.B. 1980 Flowfield model for a rectangular planform wing beyond stall. AIAA J. 18 (8), 1006–1008.CrossRefGoogle Scholar
Wygnanski, I., Tewes, P., Kurz, H., Taubert, L. & Chen, C. 2011 The application of boundary layer independence principle to three-dimensional turbulent mixing layers. J. Fluid Mech. 675, 336–346.CrossRefGoogle Scholar
Wygnanski, I., Tewes, P. & Taubert, L. 2014 Applying the boundary-layer independence principle to turbulent flows. J. Aircraft 51 (1), 175–182.CrossRefGoogle Scholar
Yarusevych, S., Sullivan, P.E. & Kawall, J.G. 2009 On vortex shedding from an airfoil in low-Reynolds-number flows. J. Fluid Mech. 632, 245–271.CrossRefGoogle Scholar
Yeh, C.-A., Benton, S.I., Taira, K. & Garmann, D.J. 2020 Resolvent analysis of an airfoil laminar separation bubble at $Re = 500 000$. Phys. Rev. Fluids 5 (8), 083906.CrossRefGoogle Scholar
Yeh, C.-A. & Taira, K. 2019 Resolvent-analysis-based design of airfoil separation control. J. Fluid Mech. 867, 572–610.CrossRefGoogle Scholar
Yen, S.-C. & Hsu, C.M. 2007 Flow patterns and wake structure of a swept-back wing. AIAA J. 45 (1), 228–236.CrossRefGoogle Scholar
Zhang, K., Hayostek, S., Amitay, M., Burstev, A., Theofilis, V. & Taira, K. 2020 a Laminar separated flows over finite-aspect-ratio swept wings. J. Fluid Mech. 905, R1.CrossRefGoogle Scholar
Zhang, K., Hayostek, S., Amitay, M., He, W., Theofilis, V. & Taira, K. 2020 b On the formation of three-dimensional separated flows over wings under tip effects. J. Fluid Mech. 895, A9.CrossRefGoogle Scholar
Zhang, K. & Taira, K. 2022 Laminar vortex dynamics around forward-swept wings. Phys. Rev. Fluids 7 (2), 024704.CrossRefGoogle Scholar